Mechanical Engineering Exam > Mechanical Engineering Questions > 100 kW power is supplied to the machine throu...
Start Learning for Free
100 kW power is supplied to the machine through a gear box which uses an epicyclic gear train. The power is supplied at 100 rad/s. The speed of the output shaft of the gear box is 10 rad/s in a sense opposite to the input speed. What is the holding toque on the fixed gear of the train?
- a)8 kNm
- b)9 kNm
- c)10 kNm
- d)11 kNm
Correct answer is option 'B'. Can you explain this answer?
Verified Answer
100 kW power is supplied to the machine through a gear box which uses ...
Holding torque
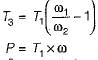
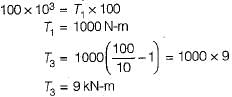
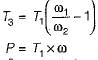
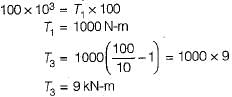
Most Upvoted Answer
100 kW power is supplied to the machine through a gear box which uses ...
To determine the holding torque on the fixed gear of the epicyclic gear train, we can use the power equation and the speed relationships of the gear train.
Given:
Power supplied = 100 kW
Input speed = 100 rad/s
Output speed = -10 rad/s (opposite to the input speed)
We can start by calculating the torque at the input shaft of the gear train using the power equation:
Power = Torque x Speed
Rearranging the equation:
Torque = Power / Speed
Substituting the given values:
Torque at input shaft = 100 kW / 100 rad/s = 1000 Nm
Next, we need to determine the speed ratio of the gear train. In an epicyclic gear train, the speed of the output shaft is related to the speeds of the input shaft and the carrier by the following equation:
Speed of output shaft = (Speed of input shaft + Speed of carrier) / Number of teeth on the output gear
Given that the speed of the output shaft is -10 rad/s and the speed of the input shaft is 100 rad/s, and assuming the number of teeth on the output gear is 1, we can solve for the speed of the carrier:
-10 = (100 + Speed of carrier) / 1
Speed of carrier = -110 rad/s
Since the speed of the carrier is negative, it means that the carrier is rotating in the opposite direction to the input shaft. This indicates that the fixed gear is the output gear.
Finally, we can determine the holding torque on the fixed gear by considering the torque relationships in an epicyclic gear train. The torque on the fixed gear is equal to the sum of the torques on the input gear and the carrier gear:
Holding torque on fixed gear = Torque on input gear + Torque on carrier gear
Holding torque on fixed gear = Torque at input shaft + (-Torque at input shaft) = 1000 Nm + (-1000 Nm) = 0 Nm
Therefore, the holding torque on the fixed gear of the epicyclic gear train is 0 Nm.
Given:
Power supplied = 100 kW
Input speed = 100 rad/s
Output speed = -10 rad/s (opposite to the input speed)
We can start by calculating the torque at the input shaft of the gear train using the power equation:
Power = Torque x Speed
Rearranging the equation:
Torque = Power / Speed
Substituting the given values:
Torque at input shaft = 100 kW / 100 rad/s = 1000 Nm
Next, we need to determine the speed ratio of the gear train. In an epicyclic gear train, the speed of the output shaft is related to the speeds of the input shaft and the carrier by the following equation:
Speed of output shaft = (Speed of input shaft + Speed of carrier) / Number of teeth on the output gear
Given that the speed of the output shaft is -10 rad/s and the speed of the input shaft is 100 rad/s, and assuming the number of teeth on the output gear is 1, we can solve for the speed of the carrier:
-10 = (100 + Speed of carrier) / 1
Speed of carrier = -110 rad/s
Since the speed of the carrier is negative, it means that the carrier is rotating in the opposite direction to the input shaft. This indicates that the fixed gear is the output gear.
Finally, we can determine the holding torque on the fixed gear by considering the torque relationships in an epicyclic gear train. The torque on the fixed gear is equal to the sum of the torques on the input gear and the carrier gear:
Holding torque on fixed gear = Torque on input gear + Torque on carrier gear
Holding torque on fixed gear = Torque at input shaft + (-Torque at input shaft) = 1000 Nm + (-1000 Nm) = 0 Nm
Therefore, the holding torque on the fixed gear of the epicyclic gear train is 0 Nm.

|
Explore Courses for Mechanical Engineering exam
|

|
Similar Mechanical Engineering Doubts
100 kW power is supplied to the machine through a gear box which uses an epicyclic gear train. The power is supplied at 100 rad/s. The speed of the output shaft of the gear box is 10 rad/s in a sense opposite to the input speed. What is the holding toque on the fixed gear of the train?a)8 kNmb)9 kNmc)10 kNmd)11 kNmCorrect answer is option 'B'. Can you explain this answer?
Question Description
100 kW power is supplied to the machine through a gear box which uses an epicyclic gear train. The power is supplied at 100 rad/s. The speed of the output shaft of the gear box is 10 rad/s in a sense opposite to the input speed. What is the holding toque on the fixed gear of the train?a)8 kNmb)9 kNmc)10 kNmd)11 kNmCorrect answer is option 'B'. Can you explain this answer? for Mechanical Engineering 2025 is part of Mechanical Engineering preparation. The Question and answers have been prepared according to the Mechanical Engineering exam syllabus. Information about 100 kW power is supplied to the machine through a gear box which uses an epicyclic gear train. The power is supplied at 100 rad/s. The speed of the output shaft of the gear box is 10 rad/s in a sense opposite to the input speed. What is the holding toque on the fixed gear of the train?a)8 kNmb)9 kNmc)10 kNmd)11 kNmCorrect answer is option 'B'. Can you explain this answer? covers all topics & solutions for Mechanical Engineering 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for 100 kW power is supplied to the machine through a gear box which uses an epicyclic gear train. The power is supplied at 100 rad/s. The speed of the output shaft of the gear box is 10 rad/s in a sense opposite to the input speed. What is the holding toque on the fixed gear of the train?a)8 kNmb)9 kNmc)10 kNmd)11 kNmCorrect answer is option 'B'. Can you explain this answer?.
100 kW power is supplied to the machine through a gear box which uses an epicyclic gear train. The power is supplied at 100 rad/s. The speed of the output shaft of the gear box is 10 rad/s in a sense opposite to the input speed. What is the holding toque on the fixed gear of the train?a)8 kNmb)9 kNmc)10 kNmd)11 kNmCorrect answer is option 'B'. Can you explain this answer? for Mechanical Engineering 2025 is part of Mechanical Engineering preparation. The Question and answers have been prepared according to the Mechanical Engineering exam syllabus. Information about 100 kW power is supplied to the machine through a gear box which uses an epicyclic gear train. The power is supplied at 100 rad/s. The speed of the output shaft of the gear box is 10 rad/s in a sense opposite to the input speed. What is the holding toque on the fixed gear of the train?a)8 kNmb)9 kNmc)10 kNmd)11 kNmCorrect answer is option 'B'. Can you explain this answer? covers all topics & solutions for Mechanical Engineering 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for 100 kW power is supplied to the machine through a gear box which uses an epicyclic gear train. The power is supplied at 100 rad/s. The speed of the output shaft of the gear box is 10 rad/s in a sense opposite to the input speed. What is the holding toque on the fixed gear of the train?a)8 kNmb)9 kNmc)10 kNmd)11 kNmCorrect answer is option 'B'. Can you explain this answer?.
Solutions for 100 kW power is supplied to the machine through a gear box which uses an epicyclic gear train. The power is supplied at 100 rad/s. The speed of the output shaft of the gear box is 10 rad/s in a sense opposite to the input speed. What is the holding toque on the fixed gear of the train?a)8 kNmb)9 kNmc)10 kNmd)11 kNmCorrect answer is option 'B'. Can you explain this answer? in English & in Hindi are available as part of our courses for Mechanical Engineering.
Download more important topics, notes, lectures and mock test series for Mechanical Engineering Exam by signing up for free.
Here you can find the meaning of 100 kW power is supplied to the machine through a gear box which uses an epicyclic gear train. The power is supplied at 100 rad/s. The speed of the output shaft of the gear box is 10 rad/s in a sense opposite to the input speed. What is the holding toque on the fixed gear of the train?a)8 kNmb)9 kNmc)10 kNmd)11 kNmCorrect answer is option 'B'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
100 kW power is supplied to the machine through a gear box which uses an epicyclic gear train. The power is supplied at 100 rad/s. The speed of the output shaft of the gear box is 10 rad/s in a sense opposite to the input speed. What is the holding toque on the fixed gear of the train?a)8 kNmb)9 kNmc)10 kNmd)11 kNmCorrect answer is option 'B'. Can you explain this answer?, a detailed solution for 100 kW power is supplied to the machine through a gear box which uses an epicyclic gear train. The power is supplied at 100 rad/s. The speed of the output shaft of the gear box is 10 rad/s in a sense opposite to the input speed. What is the holding toque on the fixed gear of the train?a)8 kNmb)9 kNmc)10 kNmd)11 kNmCorrect answer is option 'B'. Can you explain this answer? has been provided alongside types of 100 kW power is supplied to the machine through a gear box which uses an epicyclic gear train. The power is supplied at 100 rad/s. The speed of the output shaft of the gear box is 10 rad/s in a sense opposite to the input speed. What is the holding toque on the fixed gear of the train?a)8 kNmb)9 kNmc)10 kNmd)11 kNmCorrect answer is option 'B'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice 100 kW power is supplied to the machine through a gear box which uses an epicyclic gear train. The power is supplied at 100 rad/s. The speed of the output shaft of the gear box is 10 rad/s in a sense opposite to the input speed. What is the holding toque on the fixed gear of the train?a)8 kNmb)9 kNmc)10 kNmd)11 kNmCorrect answer is option 'B'. Can you explain this answer? tests, examples and also practice Mechanical Engineering tests.

|
Explore Courses for Mechanical Engineering exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


























