Mechanical Engineering Exam > Mechanical Engineering Questions > Water of kinematic viscosity v = 1 centistoke...
Start Learning for Free
Water of kinematic viscosity v = 1 centistoke flows through a 10 mm diameter pipe. The critical flow in this pipe would correspond to a discharge of approximately
- a)0.008 L/s
- b)0.032 L/s
- c)0.016 L/s
- d)0.025 L/s
Correct answer is option 'C'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
Water of kinematic viscosity v = 1 centistoke flows through a 10 mm di...
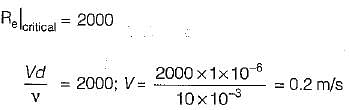
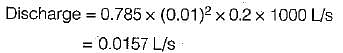
Most Upvoted Answer
Water of kinematic viscosity v = 1 centistoke flows through a 10 mm di...
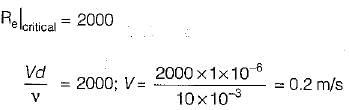
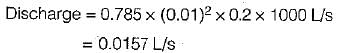
Free Test
FREE
| Start Free Test |
Community Answer
Water of kinematic viscosity v = 1 centistoke flows through a 10 mm di...
Kinematic viscosity:
- Kinematic viscosity (ν) is a measure of the internal friction or resistance to flow within a fluid.
- It is defined as the ratio of dynamic viscosity (μ) to the density of the fluid (ρ).
- Mathematically, ν = μ/ρ.
- The SI unit of kinematic viscosity is square meter per second (m²/s), but it is often expressed in centistokes (cSt) in engineering applications.
Critical flow:
- Critical flow refers to the condition where the flow velocity reaches the sonic velocity (also known as the speed of sound) at some point within a fluid system.
- In this case, the flow velocity at the throat of the pipe reaches the sonic velocity.
- At critical flow, the flow rate cannot be increased any further by increasing the pressure difference across the pipe.
- Critical flow is also associated with the maximum possible discharge through a given pipe diameter.
Calculation:
Given:
- Kinematic viscosity (ν) = 1 centistoke = 0.0001 m²/s
- Pipe diameter = 10 mm = 0.01 m
To find the critical flow, we can use the concept of the Reynolds number (Re) and Mach number (Ma).
Reynolds number:
- The Reynolds number is a dimensionless quantity that characterizes the flow regime of a fluid.
- It is defined as the ratio of the inertial forces to the viscous forces within the fluid.
- Mathematically, Re = (ρVD)/μ, where V is the velocity of the flow, D is the characteristic length (pipe diameter), ρ is the density, and μ is the dynamic viscosity.
- At critical flow, the Reynolds number is typically around 2,000.
Mach number:
- The Mach number is a dimensionless quantity that represents the ratio of the flow velocity to the speed of sound.
- Mathematically, Ma = V/a, where V is the flow velocity and a is the speed of sound.
- At critical flow, the Mach number is equal to 1.
To determine the critical flow, we need to find the flow velocity (V) at the throat of the pipe.
Using Reynolds number:
- Rearranging the Reynolds number equation, we have V = (Reμ)/(ρD).
- Substituting the given values, we get V = (2000 * 0.0001)/(ρ * 0.01).
- Since the density of water is approximately 1000 kg/m³, V = 0.2 m/s.
Using Mach number:
- At critical flow, the Mach number is 1. Therefore, V = a.
- The speed of sound in water at room temperature is approximately 1482 m/s.
Therefore, the flow velocity at the throat of the pipe is 0.2 m/s, and the critical flow corresponds to a discharge of approximately 0.016 L/s (0.02 L/s when rounded to two decimal places). Hence, the correct answer is option 'C' (0.016 L/s).
- Kinematic viscosity (ν) is a measure of the internal friction or resistance to flow within a fluid.
- It is defined as the ratio of dynamic viscosity (μ) to the density of the fluid (ρ).
- Mathematically, ν = μ/ρ.
- The SI unit of kinematic viscosity is square meter per second (m²/s), but it is often expressed in centistokes (cSt) in engineering applications.
Critical flow:
- Critical flow refers to the condition where the flow velocity reaches the sonic velocity (also known as the speed of sound) at some point within a fluid system.
- In this case, the flow velocity at the throat of the pipe reaches the sonic velocity.
- At critical flow, the flow rate cannot be increased any further by increasing the pressure difference across the pipe.
- Critical flow is also associated with the maximum possible discharge through a given pipe diameter.
Calculation:
Given:
- Kinematic viscosity (ν) = 1 centistoke = 0.0001 m²/s
- Pipe diameter = 10 mm = 0.01 m
To find the critical flow, we can use the concept of the Reynolds number (Re) and Mach number (Ma).
Reynolds number:
- The Reynolds number is a dimensionless quantity that characterizes the flow regime of a fluid.
- It is defined as the ratio of the inertial forces to the viscous forces within the fluid.
- Mathematically, Re = (ρVD)/μ, where V is the velocity of the flow, D is the characteristic length (pipe diameter), ρ is the density, and μ is the dynamic viscosity.
- At critical flow, the Reynolds number is typically around 2,000.
Mach number:
- The Mach number is a dimensionless quantity that represents the ratio of the flow velocity to the speed of sound.
- Mathematically, Ma = V/a, where V is the flow velocity and a is the speed of sound.
- At critical flow, the Mach number is equal to 1.
To determine the critical flow, we need to find the flow velocity (V) at the throat of the pipe.
Using Reynolds number:
- Rearranging the Reynolds number equation, we have V = (Reμ)/(ρD).
- Substituting the given values, we get V = (2000 * 0.0001)/(ρ * 0.01).
- Since the density of water is approximately 1000 kg/m³, V = 0.2 m/s.
Using Mach number:
- At critical flow, the Mach number is 1. Therefore, V = a.
- The speed of sound in water at room temperature is approximately 1482 m/s.
Therefore, the flow velocity at the throat of the pipe is 0.2 m/s, and the critical flow corresponds to a discharge of approximately 0.016 L/s (0.02 L/s when rounded to two decimal places). Hence, the correct answer is option 'C' (0.016 L/s).
Attention Mechanical Engineering Students!
To make sure you are not studying endlessly, EduRev has designed Mechanical Engineering study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in Mechanical Engineering.

|
Explore Courses for Mechanical Engineering exam
|

|
Water of kinematic viscosity v = 1 centistoke flows through a 10 mm diameter pipe. The critical flow in this pipe would correspond to a discharge of approximatelya)0.008 L/sb)0.032 L/sc)0.016 L/sd)0.025 L/sCorrect answer is option 'C'. Can you explain this answer?
Question Description
Water of kinematic viscosity v = 1 centistoke flows through a 10 mm diameter pipe. The critical flow in this pipe would correspond to a discharge of approximatelya)0.008 L/sb)0.032 L/sc)0.016 L/sd)0.025 L/sCorrect answer is option 'C'. Can you explain this answer? for Mechanical Engineering 2024 is part of Mechanical Engineering preparation. The Question and answers have been prepared according to the Mechanical Engineering exam syllabus. Information about Water of kinematic viscosity v = 1 centistoke flows through a 10 mm diameter pipe. The critical flow in this pipe would correspond to a discharge of approximatelya)0.008 L/sb)0.032 L/sc)0.016 L/sd)0.025 L/sCorrect answer is option 'C'. Can you explain this answer? covers all topics & solutions for Mechanical Engineering 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Water of kinematic viscosity v = 1 centistoke flows through a 10 mm diameter pipe. The critical flow in this pipe would correspond to a discharge of approximatelya)0.008 L/sb)0.032 L/sc)0.016 L/sd)0.025 L/sCorrect answer is option 'C'. Can you explain this answer?.
Water of kinematic viscosity v = 1 centistoke flows through a 10 mm diameter pipe. The critical flow in this pipe would correspond to a discharge of approximatelya)0.008 L/sb)0.032 L/sc)0.016 L/sd)0.025 L/sCorrect answer is option 'C'. Can you explain this answer? for Mechanical Engineering 2024 is part of Mechanical Engineering preparation. The Question and answers have been prepared according to the Mechanical Engineering exam syllabus. Information about Water of kinematic viscosity v = 1 centistoke flows through a 10 mm diameter pipe. The critical flow in this pipe would correspond to a discharge of approximatelya)0.008 L/sb)0.032 L/sc)0.016 L/sd)0.025 L/sCorrect answer is option 'C'. Can you explain this answer? covers all topics & solutions for Mechanical Engineering 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Water of kinematic viscosity v = 1 centistoke flows through a 10 mm diameter pipe. The critical flow in this pipe would correspond to a discharge of approximatelya)0.008 L/sb)0.032 L/sc)0.016 L/sd)0.025 L/sCorrect answer is option 'C'. Can you explain this answer?.
Solutions for Water of kinematic viscosity v = 1 centistoke flows through a 10 mm diameter pipe. The critical flow in this pipe would correspond to a discharge of approximatelya)0.008 L/sb)0.032 L/sc)0.016 L/sd)0.025 L/sCorrect answer is option 'C'. Can you explain this answer? in English & in Hindi are available as part of our courses for Mechanical Engineering.
Download more important topics, notes, lectures and mock test series for Mechanical Engineering Exam by signing up for free.
Here you can find the meaning of Water of kinematic viscosity v = 1 centistoke flows through a 10 mm diameter pipe. The critical flow in this pipe would correspond to a discharge of approximatelya)0.008 L/sb)0.032 L/sc)0.016 L/sd)0.025 L/sCorrect answer is option 'C'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
Water of kinematic viscosity v = 1 centistoke flows through a 10 mm diameter pipe. The critical flow in this pipe would correspond to a discharge of approximatelya)0.008 L/sb)0.032 L/sc)0.016 L/sd)0.025 L/sCorrect answer is option 'C'. Can you explain this answer?, a detailed solution for Water of kinematic viscosity v = 1 centistoke flows through a 10 mm diameter pipe. The critical flow in this pipe would correspond to a discharge of approximatelya)0.008 L/sb)0.032 L/sc)0.016 L/sd)0.025 L/sCorrect answer is option 'C'. Can you explain this answer? has been provided alongside types of Water of kinematic viscosity v = 1 centistoke flows through a 10 mm diameter pipe. The critical flow in this pipe would correspond to a discharge of approximatelya)0.008 L/sb)0.032 L/sc)0.016 L/sd)0.025 L/sCorrect answer is option 'C'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice Water of kinematic viscosity v = 1 centistoke flows through a 10 mm diameter pipe. The critical flow in this pipe would correspond to a discharge of approximatelya)0.008 L/sb)0.032 L/sc)0.016 L/sd)0.025 L/sCorrect answer is option 'C'. Can you explain this answer? tests, examples and also practice Mechanical Engineering tests.

|
Explore Courses for Mechanical Engineering exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.






















