Civil Engineering (CE) Exam > Civil Engineering (CE) Questions > An isosceles triangular plate of base 3 m and...
Start Learning for Free
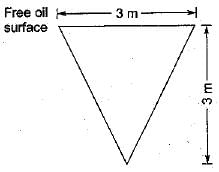
An isosceles triangular plate of base 3 m and altitude 3 m is immersed vertically in an oil of specific gravity 0.8. The base of the plate coincides with the free surface of oil. The centre of pressure will lie at a distance of (from free surface)
- a)2.5 m
- b)2 m
- c)1.5 m
- d)1 m
Correct answer is option 'C'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
An isosceles triangular plate of base 3 m and altitude 3 m is immersed...
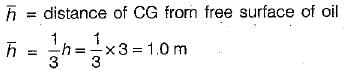
Depth of pressure (h*) from free oil surface is given by,
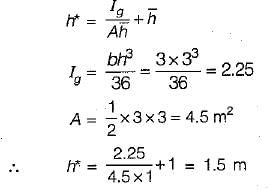
Most Upvoted Answer
An isosceles triangular plate of base 3 m and altitude 3 m is immersed...
Given:
- Base of the isosceles triangular plate = 3 m
- Altitude of the isosceles triangular plate = 3 m
- Specific gravity of oil = 0.8
To find: Distance of the center of pressure from the free surface of oil
Concepts used:
- Center of pressure
- Moment of fluid force
- Hydrostatic pressure
Solution:
1. We can assume that the isosceles triangular plate is divided into two right triangles with base and height both equal to 3/2 m.
2. Let's consider the left triangle and find the horizontal distance from the centroid of the triangle to the vertical line passing through the centroid of the triangle.
- Centroid of the left triangle will be at a height of 2/3 times the altitude from the base of the triangle.
- Therefore, h = 2/3 × (3/2) = 1 m (height of centroid from the base).
- The horizontal distance from the centroid to the vertical line passing through the centroid will be half the base of the triangle.
- Therefore, b/2 = 3/4 m (base of triangle divided by 2).
- Using Pythagoras theorem, the horizontal distance d can be found as follows:
d² = (3/4)² + 1²
d² = 9/16 + 1
d² = 25/16
d = 5/4 m
3. Now, let's find the vertical location of the center of pressure.
- The hydrostatic pressure at any depth is given by P = γh, where γ is the specific weight (weight per unit volume) of the fluid and h is the depth.
- The pressure distribution on the left triangle will be triangular, with the pressure at the base being zero and increasing linearly with depth.
- The average pressure on the left triangle can be found as follows:
Average pressure = (P1 + P2 + P3)/3, where P1, P2, and P3 are the pressures at one-third depth, two-third depth, and full depth, respectively.
- P3 = γh = 0.8 × 3 = 2.4 kN/m²
- P1 = γh/3 = 0.8 × 1 = 0.8 kN/m²
- P2 = γh × 2/3 = 0.8 × 2 = 1.6 kN/m²
- Average pressure = (0.8 + 1.6 + 2.4)/3 = 1.6 kN/m²
4. The moment of fluid force about any point is given by the product of the force and the perpendicular distance from the point to the line of action of the force.
- Let's consider the left triangle and find the moment of fluid force about the vertical line passing through the centroid of the triangle.
- The force on the left triangle can be found by integrating the pressure distribution over the area of the triangle.
- Force = ∫PdA = ∫(0.8h)bdh, where h varies from 0 to 3/2 m and b is the base of the triangle (3/2 m).
- Force = 0.8b∫h^2dh = 0.8 × (3/
- Base of the isosceles triangular plate = 3 m
- Altitude of the isosceles triangular plate = 3 m
- Specific gravity of oil = 0.8
To find: Distance of the center of pressure from the free surface of oil
Concepts used:
- Center of pressure
- Moment of fluid force
- Hydrostatic pressure
Solution:
1. We can assume that the isosceles triangular plate is divided into two right triangles with base and height both equal to 3/2 m.
2. Let's consider the left triangle and find the horizontal distance from the centroid of the triangle to the vertical line passing through the centroid of the triangle.
- Centroid of the left triangle will be at a height of 2/3 times the altitude from the base of the triangle.
- Therefore, h = 2/3 × (3/2) = 1 m (height of centroid from the base).
- The horizontal distance from the centroid to the vertical line passing through the centroid will be half the base of the triangle.
- Therefore, b/2 = 3/4 m (base of triangle divided by 2).
- Using Pythagoras theorem, the horizontal distance d can be found as follows:
d² = (3/4)² + 1²
d² = 9/16 + 1
d² = 25/16
d = 5/4 m
3. Now, let's find the vertical location of the center of pressure.
- The hydrostatic pressure at any depth is given by P = γh, where γ is the specific weight (weight per unit volume) of the fluid and h is the depth.
- The pressure distribution on the left triangle will be triangular, with the pressure at the base being zero and increasing linearly with depth.
- The average pressure on the left triangle can be found as follows:
Average pressure = (P1 + P2 + P3)/3, where P1, P2, and P3 are the pressures at one-third depth, two-third depth, and full depth, respectively.
- P3 = γh = 0.8 × 3 = 2.4 kN/m²
- P1 = γh/3 = 0.8 × 1 = 0.8 kN/m²
- P2 = γh × 2/3 = 0.8 × 2 = 1.6 kN/m²
- Average pressure = (0.8 + 1.6 + 2.4)/3 = 1.6 kN/m²
4. The moment of fluid force about any point is given by the product of the force and the perpendicular distance from the point to the line of action of the force.
- Let's consider the left triangle and find the moment of fluid force about the vertical line passing through the centroid of the triangle.
- The force on the left triangle can be found by integrating the pressure distribution over the area of the triangle.
- Force = ∫PdA = ∫(0.8h)bdh, where h varies from 0 to 3/2 m and b is the base of the triangle (3/2 m).
- Force = 0.8b∫h^2dh = 0.8 × (3/
Free Test
FREE
| Start Free Test |
Community Answer
An isosceles triangular plate of base 3 m and altitude 3 m is immersed...
Brian and Faith are students from Kenya Water Institute. After spending sometime carrying out a research, they discovered that there was need to analyze the hydrostatics law. Outline how they can determine; i. The pressure at a depth of 15m below the free surface of water in a reservoir. (2 marks) ii. Determine the height of water column corresponding to a pressure of 54KN/m2
Attention Civil Engineering (CE) Students!
To make sure you are not studying endlessly, EduRev has designed Civil Engineering (CE) study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in Civil Engineering (CE).

|
Explore Courses for Civil Engineering (CE) exam
|

|
Similar Civil Engineering (CE) Doubts
An isosceles triangular plate of base 3 m and altitude 3 m is immersed vertically in an oil of specific gravity 0.8. The base of the plate coincides with the free surface of oil. The centre of pressure will lie at a distance of (from free surface)a)2.5 mb)2 mc)1.5 md)1 mCorrect answer is option 'C'. Can you explain this answer?
Question Description
An isosceles triangular plate of base 3 m and altitude 3 m is immersed vertically in an oil of specific gravity 0.8. The base of the plate coincides with the free surface of oil. The centre of pressure will lie at a distance of (from free surface)a)2.5 mb)2 mc)1.5 md)1 mCorrect answer is option 'C'. Can you explain this answer? for Civil Engineering (CE) 2024 is part of Civil Engineering (CE) preparation. The Question and answers have been prepared according to the Civil Engineering (CE) exam syllabus. Information about An isosceles triangular plate of base 3 m and altitude 3 m is immersed vertically in an oil of specific gravity 0.8. The base of the plate coincides with the free surface of oil. The centre of pressure will lie at a distance of (from free surface)a)2.5 mb)2 mc)1.5 md)1 mCorrect answer is option 'C'. Can you explain this answer? covers all topics & solutions for Civil Engineering (CE) 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for An isosceles triangular plate of base 3 m and altitude 3 m is immersed vertically in an oil of specific gravity 0.8. The base of the plate coincides with the free surface of oil. The centre of pressure will lie at a distance of (from free surface)a)2.5 mb)2 mc)1.5 md)1 mCorrect answer is option 'C'. Can you explain this answer?.
An isosceles triangular plate of base 3 m and altitude 3 m is immersed vertically in an oil of specific gravity 0.8. The base of the plate coincides with the free surface of oil. The centre of pressure will lie at a distance of (from free surface)a)2.5 mb)2 mc)1.5 md)1 mCorrect answer is option 'C'. Can you explain this answer? for Civil Engineering (CE) 2024 is part of Civil Engineering (CE) preparation. The Question and answers have been prepared according to the Civil Engineering (CE) exam syllabus. Information about An isosceles triangular plate of base 3 m and altitude 3 m is immersed vertically in an oil of specific gravity 0.8. The base of the plate coincides with the free surface of oil. The centre of pressure will lie at a distance of (from free surface)a)2.5 mb)2 mc)1.5 md)1 mCorrect answer is option 'C'. Can you explain this answer? covers all topics & solutions for Civil Engineering (CE) 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for An isosceles triangular plate of base 3 m and altitude 3 m is immersed vertically in an oil of specific gravity 0.8. The base of the plate coincides with the free surface of oil. The centre of pressure will lie at a distance of (from free surface)a)2.5 mb)2 mc)1.5 md)1 mCorrect answer is option 'C'. Can you explain this answer?.
Solutions for An isosceles triangular plate of base 3 m and altitude 3 m is immersed vertically in an oil of specific gravity 0.8. The base of the plate coincides with the free surface of oil. The centre of pressure will lie at a distance of (from free surface)a)2.5 mb)2 mc)1.5 md)1 mCorrect answer is option 'C'. Can you explain this answer? in English & in Hindi are available as part of our courses for Civil Engineering (CE).
Download more important topics, notes, lectures and mock test series for Civil Engineering (CE) Exam by signing up for free.
Here you can find the meaning of An isosceles triangular plate of base 3 m and altitude 3 m is immersed vertically in an oil of specific gravity 0.8. The base of the plate coincides with the free surface of oil. The centre of pressure will lie at a distance of (from free surface)a)2.5 mb)2 mc)1.5 md)1 mCorrect answer is option 'C'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
An isosceles triangular plate of base 3 m and altitude 3 m is immersed vertically in an oil of specific gravity 0.8. The base of the plate coincides with the free surface of oil. The centre of pressure will lie at a distance of (from free surface)a)2.5 mb)2 mc)1.5 md)1 mCorrect answer is option 'C'. Can you explain this answer?, a detailed solution for An isosceles triangular plate of base 3 m and altitude 3 m is immersed vertically in an oil of specific gravity 0.8. The base of the plate coincides with the free surface of oil. The centre of pressure will lie at a distance of (from free surface)a)2.5 mb)2 mc)1.5 md)1 mCorrect answer is option 'C'. Can you explain this answer? has been provided alongside types of An isosceles triangular plate of base 3 m and altitude 3 m is immersed vertically in an oil of specific gravity 0.8. The base of the plate coincides with the free surface of oil. The centre of pressure will lie at a distance of (from free surface)a)2.5 mb)2 mc)1.5 md)1 mCorrect answer is option 'C'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice An isosceles triangular plate of base 3 m and altitude 3 m is immersed vertically in an oil of specific gravity 0.8. The base of the plate coincides with the free surface of oil. The centre of pressure will lie at a distance of (from free surface)a)2.5 mb)2 mc)1.5 md)1 mCorrect answer is option 'C'. Can you explain this answer? tests, examples and also practice Civil Engineering (CE) tests.

|
Explore Courses for Civil Engineering (CE) exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
























