Mathematics Exam > Mathematics Questions > The point of intersection of the lines drawn ...
Start Learning for Free
The point of intersection of the lines drawn from the vertices of any tetrahedron to the centroids of the opposite faces divides the distance from each vertex to the opposite face in which of the following ratios?
- a)3:1
- b)3:4
- c)2:1
- d)2:3
Correct answer is option 'A'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
The point of intersection of the lines drawn from the vertices of any ...
About Direction Cosines and Direction Ratios.
1. If a given line makes angles α, β, γ with positive directions of the axes of x, y and x respectively, then cosα, cosβ, cosγ are called the direction cosines (in short d.c.’s) of the given line.
Direction cosines of a line are generally denoted by l, m, n and are written as [l, m, n]
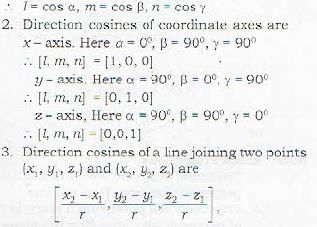
where r is the distance between the given points.
In particular, the d.c.'s of a line joining a point (a1, y1, z1) to the origin are
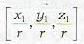
4. Direction Cosines satisfy
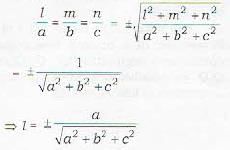
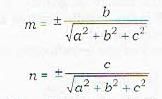
Thus if the d.r.’s of a line are a,b, c, then the direction cosines of the line are
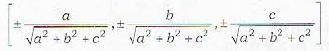
1. If a given line makes angles α, β, γ with positive directions of the axes of x, y and x respectively, then cosα, cosβ, cosγ are called the direction cosines (in short d.c.’s) of the given line.
Direction cosines of a line are generally denoted by l, m, n and are written as [l, m, n]
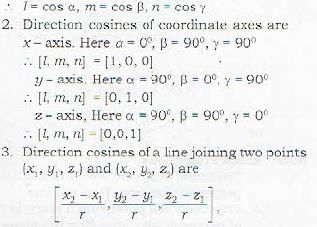
where r is the distance between the given points.
In particular, the d.c.'s of a line joining a point (a1, y1, z1) to the origin are
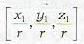
4. Direction Cosines satisfy
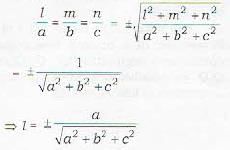
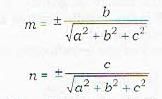
Thus if the d.r.’s of a line are a,b, c, then the direction cosines of the line are
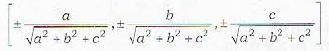
Most Upvoted Answer
The point of intersection of the lines drawn from the vertices of any ...
Explanation:
To understand the solution to this problem, let's first define some terms:
- Let ABCD be a tetrahedron with vertices A, B, C, and D.
- Let E, F, G, and H be the centroids of the opposite faces of the tetrahedron.
- Let P be the point of intersection of the lines AE, BF, CG, and DH.
Using Centroid Theorem:
- The centroid of a triangle divides the median in the ratio 2:1.
- Each of the lines AE, BF, CG, and DH can be considered as medians of the triangles AEF, BFG, CGH, and DHE, respectively.
- Therefore, the point P divides each of these medians in the ratio 2:1.
Dividing each line segment:
- Let's consider the line segment AP.
- Since P divides the line AE in the ratio 2:1, we can say that AP:PE = 2:1.
- Similarly, we can say that BP:PF = 2:1, CP:PG = 2:1, and DP:PH = 2:1.
Using Triangle Similarity:
- Let's consider the triangle ABC.
- Since P divides the line AE in the ratio 2:1, we can say that the triangles APE and ABC are similar.
- By the property of similar triangles, we can say that the ratios of corresponding sides are equal.
- Therefore, AP:AB = PE:BC = 2:1.
- Similarly, we can prove that BP:BC = 2:1, CP:CA = 2:1, and DP:DA = 2:1.
Conclusion:
- From the above analysis, we can conclude that the point P divides each line segment from the vertices of the tetrahedron to the centroids of the opposite faces in the ratio 2:1.
- Therefore, the correct answer is option A) 2:1.
To understand the solution to this problem, let's first define some terms:
- Let ABCD be a tetrahedron with vertices A, B, C, and D.
- Let E, F, G, and H be the centroids of the opposite faces of the tetrahedron.
- Let P be the point of intersection of the lines AE, BF, CG, and DH.
Using Centroid Theorem:
- The centroid of a triangle divides the median in the ratio 2:1.
- Each of the lines AE, BF, CG, and DH can be considered as medians of the triangles AEF, BFG, CGH, and DHE, respectively.
- Therefore, the point P divides each of these medians in the ratio 2:1.
Dividing each line segment:
- Let's consider the line segment AP.
- Since P divides the line AE in the ratio 2:1, we can say that AP:PE = 2:1.
- Similarly, we can say that BP:PF = 2:1, CP:PG = 2:1, and DP:PH = 2:1.
Using Triangle Similarity:
- Let's consider the triangle ABC.
- Since P divides the line AE in the ratio 2:1, we can say that the triangles APE and ABC are similar.
- By the property of similar triangles, we can say that the ratios of corresponding sides are equal.
- Therefore, AP:AB = PE:BC = 2:1.
- Similarly, we can prove that BP:BC = 2:1, CP:CA = 2:1, and DP:DA = 2:1.
Conclusion:
- From the above analysis, we can conclude that the point P divides each line segment from the vertices of the tetrahedron to the centroids of the opposite faces in the ratio 2:1.
- Therefore, the correct answer is option A) 2:1.
Free Test
FREE
| Start Free Test |
Community Answer
The point of intersection of the lines drawn from the vertices of any ...
About Direction Cosines and Direction Ratios.
1. If a given line makes angles α, β, γ with positive directions of the axes of x, y and x respectively, then cosα, cosβ, cosγ are called the direction cosines (in short d.c.’s) of the given line.
Direction cosines of a line are generally denoted by l, m, n and are written as [l, m, n]
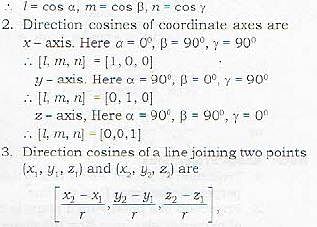
where r is the distance between the given points.
In particular, the d.c.'s of a line joining a point (a1, y1, z1) to the origin are
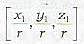
4. Direction Cosines satisfy
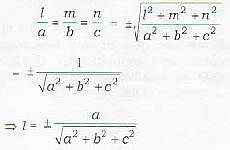
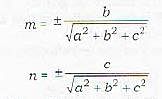
Thus if the d.r.’s of a line are a,b, c, then the direction cosines of the line are
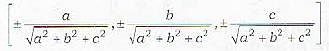
1. If a given line makes angles α, β, γ with positive directions of the axes of x, y and x respectively, then cosα, cosβ, cosγ are called the direction cosines (in short d.c.’s) of the given line.
Direction cosines of a line are generally denoted by l, m, n and are written as [l, m, n]
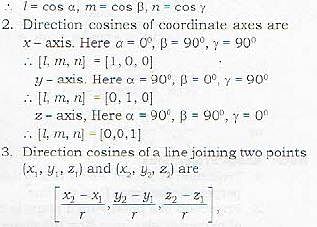
where r is the distance between the given points.
In particular, the d.c.'s of a line joining a point (a1, y1, z1) to the origin are
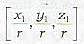
4. Direction Cosines satisfy
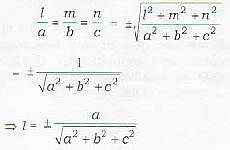
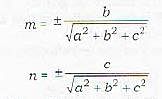
Thus if the d.r.’s of a line are a,b, c, then the direction cosines of the line are
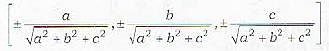

|
Explore Courses for Mathematics exam
|

|
Similar Mathematics Doubts
The point of intersection of the lines drawn from the vertices of any tetrahedron to the centroids of the opposite faces divides the distance from each vertex to the opposite face in which of the following ratios?a)3:1b)3:4c)2:1d)2:3Correct answer is option 'A'. Can you explain this answer?
Question Description
The point of intersection of the lines drawn from the vertices of any tetrahedron to the centroids of the opposite faces divides the distance from each vertex to the opposite face in which of the following ratios?a)3:1b)3:4c)2:1d)2:3Correct answer is option 'A'. Can you explain this answer? for Mathematics 2024 is part of Mathematics preparation. The Question and answers have been prepared according to the Mathematics exam syllabus. Information about The point of intersection of the lines drawn from the vertices of any tetrahedron to the centroids of the opposite faces divides the distance from each vertex to the opposite face in which of the following ratios?a)3:1b)3:4c)2:1d)2:3Correct answer is option 'A'. Can you explain this answer? covers all topics & solutions for Mathematics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The point of intersection of the lines drawn from the vertices of any tetrahedron to the centroids of the opposite faces divides the distance from each vertex to the opposite face in which of the following ratios?a)3:1b)3:4c)2:1d)2:3Correct answer is option 'A'. Can you explain this answer?.
The point of intersection of the lines drawn from the vertices of any tetrahedron to the centroids of the opposite faces divides the distance from each vertex to the opposite face in which of the following ratios?a)3:1b)3:4c)2:1d)2:3Correct answer is option 'A'. Can you explain this answer? for Mathematics 2024 is part of Mathematics preparation. The Question and answers have been prepared according to the Mathematics exam syllabus. Information about The point of intersection of the lines drawn from the vertices of any tetrahedron to the centroids of the opposite faces divides the distance from each vertex to the opposite face in which of the following ratios?a)3:1b)3:4c)2:1d)2:3Correct answer is option 'A'. Can you explain this answer? covers all topics & solutions for Mathematics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The point of intersection of the lines drawn from the vertices of any tetrahedron to the centroids of the opposite faces divides the distance from each vertex to the opposite face in which of the following ratios?a)3:1b)3:4c)2:1d)2:3Correct answer is option 'A'. Can you explain this answer?.
Solutions for The point of intersection of the lines drawn from the vertices of any tetrahedron to the centroids of the opposite faces divides the distance from each vertex to the opposite face in which of the following ratios?a)3:1b)3:4c)2:1d)2:3Correct answer is option 'A'. Can you explain this answer? in English & in Hindi are available as part of our courses for Mathematics.
Download more important topics, notes, lectures and mock test series for Mathematics Exam by signing up for free.
Here you can find the meaning of The point of intersection of the lines drawn from the vertices of any tetrahedron to the centroids of the opposite faces divides the distance from each vertex to the opposite face in which of the following ratios?a)3:1b)3:4c)2:1d)2:3Correct answer is option 'A'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
The point of intersection of the lines drawn from the vertices of any tetrahedron to the centroids of the opposite faces divides the distance from each vertex to the opposite face in which of the following ratios?a)3:1b)3:4c)2:1d)2:3Correct answer is option 'A'. Can you explain this answer?, a detailed solution for The point of intersection of the lines drawn from the vertices of any tetrahedron to the centroids of the opposite faces divides the distance from each vertex to the opposite face in which of the following ratios?a)3:1b)3:4c)2:1d)2:3Correct answer is option 'A'. Can you explain this answer? has been provided alongside types of The point of intersection of the lines drawn from the vertices of any tetrahedron to the centroids of the opposite faces divides the distance from each vertex to the opposite face in which of the following ratios?a)3:1b)3:4c)2:1d)2:3Correct answer is option 'A'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice The point of intersection of the lines drawn from the vertices of any tetrahedron to the centroids of the opposite faces divides the distance from each vertex to the opposite face in which of the following ratios?a)3:1b)3:4c)2:1d)2:3Correct answer is option 'A'. Can you explain this answer? tests, examples and also practice Mathematics tests.

|
Explore Courses for Mathematics exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















