Mechanical Engineering Exam > Mechanical Engineering Questions > A circular annular area of inner and outer di...
Start Learning for Free
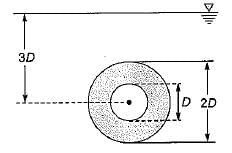
A circular annular area of inner and outer diameter, D and 2D respectively is immersed vertically in water with the center of the area at 3D below the water surface . The location of the center of pressure is
- a)3.05 D
- b)3.07 D
- c)3.1 D
- d)3.14 D
Correct answer is option 'C'. Can you explain this answer?
Verified Answer
A circular annular area of inner and outer diameter, D and 2D respecti...
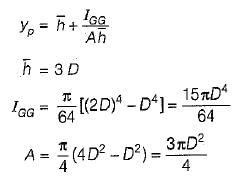
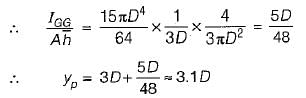
Most Upvoted Answer
A circular annular area of inner and outer diameter, D and 2D respecti...
Given data:
- Inner diameter of the annular area = D
- Outer diameter of the annular area = 2D
- Center of the annular area is located at a depth of 3D below the water surface
To find: The location of the center of pressure
Understanding the concept:
- When an object is immersed in a fluid, it experiences an upward force called the buoyant force.
- The magnitude of the buoyant force is equal to the weight of the fluid displaced by the object.
- The center of pressure is the point through which the resultant force of the fluid acts on the submerged surface.
Steps to determine the center of pressure:
1. Find the area of the annular surface:
- The area of the annular surface can be calculated as the difference between the areas of the outer and inner circles.
- Area of outer circle = π(2D/2)^2 = πD^2
- Area of inner circle = π(D/2)^2 = πD^2/4
- Area of annular surface = πD^2 - πD^2/4 = 3πD^2/4
2. Find the magnitude of the resultant force:
- The magnitude of the resultant force can be calculated as the product of the pressure and the area of the annular surface.
- Pressure = ρgh, where ρ is the density of water, g is the acceleration due to gravity, and h is the depth of the center of the annular surface below the water surface.
- Resultant force = Pressure x Area of annular surface
= (ρgh)(3πD^2/4)
= (3ρgπD^2h)/4
3. Find the moment of the resultant force:
- The moment of the resultant force about any point is given by the product of the magnitude of the resultant force and the perpendicular distance between the point and the line of action of the resultant force.
- In this case, the moment of the resultant force about the center of the annular surface is zero, as the center of pressure coincides with the center of the annular surface.
4. Find the location of the center of pressure:
- The center of pressure is located at a distance from the center of the annular surface, such that the moment of the resultant force about the center is zero.
- The location of the center of pressure can be calculated using the principle of moments.
- In this case, the moment of the resultant force about the center of the annular surface is zero, which implies that the center of pressure is also at the center of the annular surface.
Therefore, the location of the center of pressure is 3.1D (option C).
- Inner diameter of the annular area = D
- Outer diameter of the annular area = 2D
- Center of the annular area is located at a depth of 3D below the water surface
To find: The location of the center of pressure
Understanding the concept:
- When an object is immersed in a fluid, it experiences an upward force called the buoyant force.
- The magnitude of the buoyant force is equal to the weight of the fluid displaced by the object.
- The center of pressure is the point through which the resultant force of the fluid acts on the submerged surface.
Steps to determine the center of pressure:
1. Find the area of the annular surface:
- The area of the annular surface can be calculated as the difference between the areas of the outer and inner circles.
- Area of outer circle = π(2D/2)^2 = πD^2
- Area of inner circle = π(D/2)^2 = πD^2/4
- Area of annular surface = πD^2 - πD^2/4 = 3πD^2/4
2. Find the magnitude of the resultant force:
- The magnitude of the resultant force can be calculated as the product of the pressure and the area of the annular surface.
- Pressure = ρgh, where ρ is the density of water, g is the acceleration due to gravity, and h is the depth of the center of the annular surface below the water surface.
- Resultant force = Pressure x Area of annular surface
= (ρgh)(3πD^2/4)
= (3ρgπD^2h)/4
3. Find the moment of the resultant force:
- The moment of the resultant force about any point is given by the product of the magnitude of the resultant force and the perpendicular distance between the point and the line of action of the resultant force.
- In this case, the moment of the resultant force about the center of the annular surface is zero, as the center of pressure coincides with the center of the annular surface.
4. Find the location of the center of pressure:
- The center of pressure is located at a distance from the center of the annular surface, such that the moment of the resultant force about the center is zero.
- The location of the center of pressure can be calculated using the principle of moments.
- In this case, the moment of the resultant force about the center of the annular surface is zero, which implies that the center of pressure is also at the center of the annular surface.
Therefore, the location of the center of pressure is 3.1D (option C).

|
Explore Courses for Mechanical Engineering exam
|

|
Similar Mechanical Engineering Doubts
A circular annular area of inner and outer diameter, D and 2D respectively is immersed vertically in water with the center of the area at 3D below the water surface . The location of the center of pressure isa)3.05 Db)3.07 Dc)3.1 Dd)3.14 DCorrect answer is option 'C'. Can you explain this answer?
Question Description
A circular annular area of inner and outer diameter, D and 2D respectively is immersed vertically in water with the center of the area at 3D below the water surface . The location of the center of pressure isa)3.05 Db)3.07 Dc)3.1 Dd)3.14 DCorrect answer is option 'C'. Can you explain this answer? for Mechanical Engineering 2025 is part of Mechanical Engineering preparation. The Question and answers have been prepared according to the Mechanical Engineering exam syllabus. Information about A circular annular area of inner and outer diameter, D and 2D respectively is immersed vertically in water with the center of the area at 3D below the water surface . The location of the center of pressure isa)3.05 Db)3.07 Dc)3.1 Dd)3.14 DCorrect answer is option 'C'. Can you explain this answer? covers all topics & solutions for Mechanical Engineering 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A circular annular area of inner and outer diameter, D and 2D respectively is immersed vertically in water with the center of the area at 3D below the water surface . The location of the center of pressure isa)3.05 Db)3.07 Dc)3.1 Dd)3.14 DCorrect answer is option 'C'. Can you explain this answer?.
A circular annular area of inner and outer diameter, D and 2D respectively is immersed vertically in water with the center of the area at 3D below the water surface . The location of the center of pressure isa)3.05 Db)3.07 Dc)3.1 Dd)3.14 DCorrect answer is option 'C'. Can you explain this answer? for Mechanical Engineering 2025 is part of Mechanical Engineering preparation. The Question and answers have been prepared according to the Mechanical Engineering exam syllabus. Information about A circular annular area of inner and outer diameter, D and 2D respectively is immersed vertically in water with the center of the area at 3D below the water surface . The location of the center of pressure isa)3.05 Db)3.07 Dc)3.1 Dd)3.14 DCorrect answer is option 'C'. Can you explain this answer? covers all topics & solutions for Mechanical Engineering 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A circular annular area of inner and outer diameter, D and 2D respectively is immersed vertically in water with the center of the area at 3D below the water surface . The location of the center of pressure isa)3.05 Db)3.07 Dc)3.1 Dd)3.14 DCorrect answer is option 'C'. Can you explain this answer?.
Solutions for A circular annular area of inner and outer diameter, D and 2D respectively is immersed vertically in water with the center of the area at 3D below the water surface . The location of the center of pressure isa)3.05 Db)3.07 Dc)3.1 Dd)3.14 DCorrect answer is option 'C'. Can you explain this answer? in English & in Hindi are available as part of our courses for Mechanical Engineering.
Download more important topics, notes, lectures and mock test series for Mechanical Engineering Exam by signing up for free.
Here you can find the meaning of A circular annular area of inner and outer diameter, D and 2D respectively is immersed vertically in water with the center of the area at 3D below the water surface . The location of the center of pressure isa)3.05 Db)3.07 Dc)3.1 Dd)3.14 DCorrect answer is option 'C'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A circular annular area of inner and outer diameter, D and 2D respectively is immersed vertically in water with the center of the area at 3D below the water surface . The location of the center of pressure isa)3.05 Db)3.07 Dc)3.1 Dd)3.14 DCorrect answer is option 'C'. Can you explain this answer?, a detailed solution for A circular annular area of inner and outer diameter, D and 2D respectively is immersed vertically in water with the center of the area at 3D below the water surface . The location of the center of pressure isa)3.05 Db)3.07 Dc)3.1 Dd)3.14 DCorrect answer is option 'C'. Can you explain this answer? has been provided alongside types of A circular annular area of inner and outer diameter, D and 2D respectively is immersed vertically in water with the center of the area at 3D below the water surface . The location of the center of pressure isa)3.05 Db)3.07 Dc)3.1 Dd)3.14 DCorrect answer is option 'C'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A circular annular area of inner and outer diameter, D and 2D respectively is immersed vertically in water with the center of the area at 3D below the water surface . The location of the center of pressure isa)3.05 Db)3.07 Dc)3.1 Dd)3.14 DCorrect answer is option 'C'. Can you explain this answer? tests, examples and also practice Mechanical Engineering tests.

|
Explore Courses for Mechanical Engineering exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


























