Class 6 Exam > Class 6 Questions > 27 : x : : 63 : 84 Related: Examples: Ratios...
Start Learning for Free
27 : x : : 63 : 84
?Most Upvoted Answer
27 : x : : 63 : 84 Related: Examples: Ratios Proportion (Part- 3)?
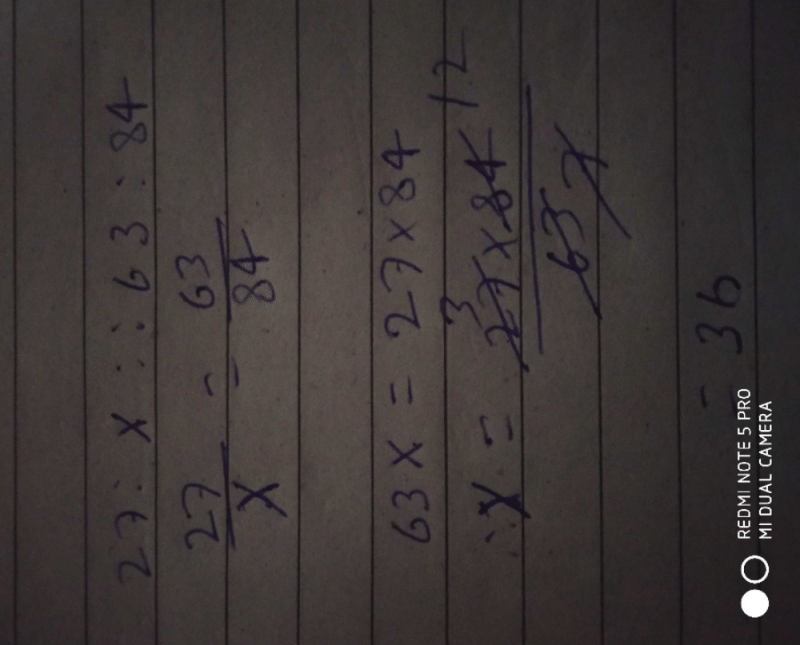
Community Answer
27 : x : : 63 : 84 Related: Examples: Ratios Proportion (Part- 3)?
Ratio:
A ratio is a comparison of two or more quantities. It shows how much one quantity is in relation to another. Ratios can be expressed in different ways, such as using numbers, fractions, or in the form of a proportion.
Proportion:
A proportion is a statement that two ratios are equal. It states that two ratios are equivalent and can be solved using cross-multiplication. In a proportion, the product of the means is equal to the product of the extremes.
Example:
Let's solve the given proportion: 27 : x :: 63 : 84
To find the value of 'x', we can set up the proportion as follows:
27/x = 63/84
Cross Multiplication:
To solve the proportion, we can use cross multiplication. This involves multiplying the numerator of one ratio with the denominator of the other ratio.
27 * 84 = 63 * x
Solve for x:
Now, we can solve for 'x' by dividing both sides of the equation by 63.
(27 * 84) / 63 = x
3584 / 63 = x
x = 56.79 (approx)
Therefore, the value of 'x' in the given proportion is approximately 56.79.
Conclusion:
In this example, we used the concept of ratios and proportions to find the value of 'x' in a given proportion. Ratios and proportions are important mathematical tools that help us compare quantities and solve problems involving unknown values. It is essential to understand the relationship between ratios and proportions to solve such problems effectively. Practicing more examples and understanding the underlying concepts will further strengthen your skills in this area.
A ratio is a comparison of two or more quantities. It shows how much one quantity is in relation to another. Ratios can be expressed in different ways, such as using numbers, fractions, or in the form of a proportion.
Proportion:
A proportion is a statement that two ratios are equal. It states that two ratios are equivalent and can be solved using cross-multiplication. In a proportion, the product of the means is equal to the product of the extremes.
Example:
Let's solve the given proportion: 27 : x :: 63 : 84
To find the value of 'x', we can set up the proportion as follows:
27/x = 63/84
Cross Multiplication:
To solve the proportion, we can use cross multiplication. This involves multiplying the numerator of one ratio with the denominator of the other ratio.
27 * 84 = 63 * x
Solve for x:
Now, we can solve for 'x' by dividing both sides of the equation by 63.
(27 * 84) / 63 = x
3584 / 63 = x
x = 56.79 (approx)
Therefore, the value of 'x' in the given proportion is approximately 56.79.
Conclusion:
In this example, we used the concept of ratios and proportions to find the value of 'x' in a given proportion. Ratios and proportions are important mathematical tools that help us compare quantities and solve problems involving unknown values. It is essential to understand the relationship between ratios and proportions to solve such problems effectively. Practicing more examples and understanding the underlying concepts will further strengthen your skills in this area.

|
Explore Courses for Class 6 exam
|

|
27 : x : : 63 : 84 Related: Examples: Ratios Proportion (Part- 3)?
Question Description
27 : x : : 63 : 84 Related: Examples: Ratios Proportion (Part- 3)? for Class 6 2025 is part of Class 6 preparation. The Question and answers have been prepared according to the Class 6 exam syllabus. Information about 27 : x : : 63 : 84 Related: Examples: Ratios Proportion (Part- 3)? covers all topics & solutions for Class 6 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for 27 : x : : 63 : 84 Related: Examples: Ratios Proportion (Part- 3)?.
27 : x : : 63 : 84 Related: Examples: Ratios Proportion (Part- 3)? for Class 6 2025 is part of Class 6 preparation. The Question and answers have been prepared according to the Class 6 exam syllabus. Information about 27 : x : : 63 : 84 Related: Examples: Ratios Proportion (Part- 3)? covers all topics & solutions for Class 6 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for 27 : x : : 63 : 84 Related: Examples: Ratios Proportion (Part- 3)?.
Solutions for 27 : x : : 63 : 84 Related: Examples: Ratios Proportion (Part- 3)? in English & in Hindi are available as part of our courses for Class 6.
Download more important topics, notes, lectures and mock test series for Class 6 Exam by signing up for free.
Here you can find the meaning of 27 : x : : 63 : 84 Related: Examples: Ratios Proportion (Part- 3)? defined & explained in the simplest way possible. Besides giving the explanation of
27 : x : : 63 : 84 Related: Examples: Ratios Proportion (Part- 3)?, a detailed solution for 27 : x : : 63 : 84 Related: Examples: Ratios Proportion (Part- 3)? has been provided alongside types of 27 : x : : 63 : 84 Related: Examples: Ratios Proportion (Part- 3)? theory, EduRev gives you an
ample number of questions to practice 27 : x : : 63 : 84 Related: Examples: Ratios Proportion (Part- 3)? tests, examples and also practice Class 6 tests.

|
Explore Courses for Class 6 exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


























