Class 12 Exam > Class 12 Questions > A bar magnet of magnetic moment 1.44am2 is p...
Start Learning for Free
A bar magnet of magnetic moment 1.44am2 is placed on a horizontal table with its north pole pointing towards north of the horizontal component of earth magnetic field is 18micro tesla then the neutral point is at distance (from the magnet) equal to
Verified Answer
A bar magnet of magnetic moment 1.44am2 is placed on a horizontal ta...
Earth’s magnetic field is horizontal only at the magnetic equator.
A point where magnetic field induction (B) due to a bar magnet is equal in magnitude and opposite in direction to the horizontal component of earth’s magnetic field (B0) is called neutral point.
If North Pole of the bar magnet faces to the geographic north pole of the earth, the two neutral points lie on the equatorial line of the bar magnet such that they equidistance from the centre of the magnet.
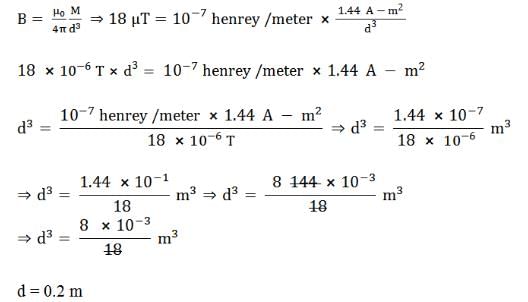
If the neutral points lie on the equatorial line of a bar magnet at a distance d from the centre of the bar magnet we can express the relation
As the following:
 This question is part of UPSC exam. View all Class 12 courses
This question is part of UPSC exam. View all Class 12 courses
Most Upvoted Answer
A bar magnet of magnetic moment 1.44am2 is placed on a horizontal ta...
The Neutral Point in a Magnetic Field
A bar magnet placed on a horizontal table experiences the influence of both the horizontal component of Earth's magnetic field and its own magnetic moment. The neutral point refers to the location where the magnetic field due to the magnet and the Earth's magnetic field cancel each other out, resulting in no net magnetic field at that point.
Magnetic Moment of the Bar Magnet
The magnetic moment of a bar magnet is a measure of its strength and is given by the product of its pole strength and the distance between its poles. It is denoted by the symbol 'm' and has the unit A.m² (ampere meter squared). In this case, the magnetic moment of the bar magnet is given as 1.44 A.m².
Earth's Magnetic Field
Earth has its own magnetic field, which can be approximated as a dipole field. The horizontal component of Earth's magnetic field is the component that is parallel to the surface of the Earth. In this case, the magnitude of the horizontal component is given as 18 μT (microtesla).
Neutral Point Calculation
To determine the distance of the neutral point from the magnet, we need to consider the balance of magnetic forces acting on the magnet. At the neutral point, the magnetic field due to the magnet and the Earth's magnetic field must cancel each other out.
The magnetic field due to a bar magnet at a point on its axial line is given by the formula:
B = (μ₀/4π) * (2m/d³)
Where:
B is the magnetic field at the point
μ₀ is the permeability of free space (4π × 10⁻⁷ T.m/A)
m is the magnetic moment of the bar magnet
d is the distance of the point from the center of the magnet
To find the neutral point, we equate the magnetic field due to the magnet to the Earth's magnetic field and solve for d.
(μ₀/4π) * (2m/d³) = B_earth
Substituting the given values:
(4π × 10⁻⁷ T.m/A) * (2 * 1.44 A.m² / d³) = 18 × 10⁻⁶ T
Simplifying the equation:
2.88 × 10⁻⁷ / d³ = 18 × 10⁻⁶ / (4π × 10⁻⁷)
Further simplification:
d³ = (4π × 10⁻⁷ * 18 × 10⁻⁶) / (2.88 × 10⁻⁷)
d³ = 28.274
Taking the cube root of both sides:
d ≈ 3.04 cm
Therefore, the neutral point is approximately 3.04 cm away from the magnet.
A bar magnet placed on a horizontal table experiences the influence of both the horizontal component of Earth's magnetic field and its own magnetic moment. The neutral point refers to the location where the magnetic field due to the magnet and the Earth's magnetic field cancel each other out, resulting in no net magnetic field at that point.
Magnetic Moment of the Bar Magnet
The magnetic moment of a bar magnet is a measure of its strength and is given by the product of its pole strength and the distance between its poles. It is denoted by the symbol 'm' and has the unit A.m² (ampere meter squared). In this case, the magnetic moment of the bar magnet is given as 1.44 A.m².
Earth's Magnetic Field
Earth has its own magnetic field, which can be approximated as a dipole field. The horizontal component of Earth's magnetic field is the component that is parallel to the surface of the Earth. In this case, the magnitude of the horizontal component is given as 18 μT (microtesla).
Neutral Point Calculation
To determine the distance of the neutral point from the magnet, we need to consider the balance of magnetic forces acting on the magnet. At the neutral point, the magnetic field due to the magnet and the Earth's magnetic field must cancel each other out.
The magnetic field due to a bar magnet at a point on its axial line is given by the formula:
B = (μ₀/4π) * (2m/d³)
Where:
B is the magnetic field at the point
μ₀ is the permeability of free space (4π × 10⁻⁷ T.m/A)
m is the magnetic moment of the bar magnet
d is the distance of the point from the center of the magnet
To find the neutral point, we equate the magnetic field due to the magnet to the Earth's magnetic field and solve for d.
(μ₀/4π) * (2m/d³) = B_earth
Substituting the given values:
(4π × 10⁻⁷ T.m/A) * (2 * 1.44 A.m² / d³) = 18 × 10⁻⁶ T
Simplifying the equation:
2.88 × 10⁻⁷ / d³ = 18 × 10⁻⁶ / (4π × 10⁻⁷)
Further simplification:
d³ = (4π × 10⁻⁷ * 18 × 10⁻⁶) / (2.88 × 10⁻⁷)
d³ = 28.274
Taking the cube root of both sides:
d ≈ 3.04 cm
Therefore, the neutral point is approximately 3.04 cm away from the magnet.

|
Explore Courses for Class 12 exam
|

|
Similar Class 12 Doubts
A bar magnet of magnetic moment 1.44am2 is placed on a horizontal table with its north pole pointing towards north of the horizontal component of earth magnetic field is 18micro tesla then the neutral point is at distance (from the magnet) equal to
Question Description
A bar magnet of magnetic moment 1.44am2 is placed on a horizontal table with its north pole pointing towards north of the horizontal component of earth magnetic field is 18micro tesla then the neutral point is at distance (from the magnet) equal to for Class 12 2025 is part of Class 12 preparation. The Question and answers have been prepared according to the Class 12 exam syllabus. Information about A bar magnet of magnetic moment 1.44am2 is placed on a horizontal table with its north pole pointing towards north of the horizontal component of earth magnetic field is 18micro tesla then the neutral point is at distance (from the magnet) equal to covers all topics & solutions for Class 12 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A bar magnet of magnetic moment 1.44am2 is placed on a horizontal table with its north pole pointing towards north of the horizontal component of earth magnetic field is 18micro tesla then the neutral point is at distance (from the magnet) equal to .
A bar magnet of magnetic moment 1.44am2 is placed on a horizontal table with its north pole pointing towards north of the horizontal component of earth magnetic field is 18micro tesla then the neutral point is at distance (from the magnet) equal to for Class 12 2025 is part of Class 12 preparation. The Question and answers have been prepared according to the Class 12 exam syllabus. Information about A bar magnet of magnetic moment 1.44am2 is placed on a horizontal table with its north pole pointing towards north of the horizontal component of earth magnetic field is 18micro tesla then the neutral point is at distance (from the magnet) equal to covers all topics & solutions for Class 12 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A bar magnet of magnetic moment 1.44am2 is placed on a horizontal table with its north pole pointing towards north of the horizontal component of earth magnetic field is 18micro tesla then the neutral point is at distance (from the magnet) equal to .
Solutions for A bar magnet of magnetic moment 1.44am2 is placed on a horizontal table with its north pole pointing towards north of the horizontal component of earth magnetic field is 18micro tesla then the neutral point is at distance (from the magnet) equal to in English & in Hindi are available as part of our courses for Class 12.
Download more important topics, notes, lectures and mock test series for Class 12 Exam by signing up for free.
Here you can find the meaning of A bar magnet of magnetic moment 1.44am2 is placed on a horizontal table with its north pole pointing towards north of the horizontal component of earth magnetic field is 18micro tesla then the neutral point is at distance (from the magnet) equal to defined & explained in the simplest way possible. Besides giving the explanation of
A bar magnet of magnetic moment 1.44am2 is placed on a horizontal table with its north pole pointing towards north of the horizontal component of earth magnetic field is 18micro tesla then the neutral point is at distance (from the magnet) equal to , a detailed solution for A bar magnet of magnetic moment 1.44am2 is placed on a horizontal table with its north pole pointing towards north of the horizontal component of earth magnetic field is 18micro tesla then the neutral point is at distance (from the magnet) equal to has been provided alongside types of A bar magnet of magnetic moment 1.44am2 is placed on a horizontal table with its north pole pointing towards north of the horizontal component of earth magnetic field is 18micro tesla then the neutral point is at distance (from the magnet) equal to theory, EduRev gives you an
ample number of questions to practice A bar magnet of magnetic moment 1.44am2 is placed on a horizontal table with its north pole pointing towards north of the horizontal component of earth magnetic field is 18micro tesla then the neutral point is at distance (from the magnet) equal to tests, examples and also practice Class 12 tests.

|
Explore Courses for Class 12 exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.





















