Mathematics Exam > Mathematics Questions > Find the total no. of all non - abelian group...
Start Learning for Free
Find the total no. of all non - abelian groups of order 6.
Correct answer is '1'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
Find the total no. of all non - abelian groups of order 6.Correct answ...
Let G be a non - abelian group of order 6 , then by Cauchy's theorem
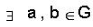
Such that O(a) = 3 , O(b) = 2 .
Let H = < a > then 0(H) = 0(a) = 3.
Since index of H in G is 2 , Hence H is normal in G.
if b ∈ H then 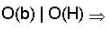 2 |3 which is contradiction, so b ∈ H
2 |3 which is contradiction, so b ∈ H
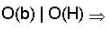 2 |3 which is contradiction, so b ∈ H
2 |3 which is contradiction, so b ∈ HH and Hb are distinct right cosets of H in G.
Hence 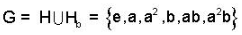
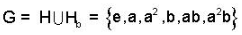
also H is normal in 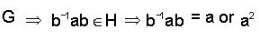
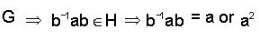
If b-1ab = a > then as (0(a) , 0(b)) = 1 , we get O(a b) = 0(a) 0(b) = 6 i.e. G is cyclic and so , abelian, which is not so ,
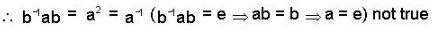
So , there is only one non - abelian group G of order 6 , namely
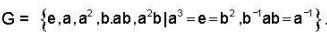
Most Upvoted Answer
Find the total no. of all non - abelian groups of order 6.Correct answ...
Non-abelian Groups of Order 6
To find the total number of non-abelian groups of order 6, we need to consider all the possible groups of this order and determine which ones are non-abelian.
Possible Groups of Order 6
There are several possible groups of order 6. Let's consider each of them and determine whether they are abelian or non-abelian.
1. Trivial Group: The trivial group is the group containing only the identity element. It is abelian.
2. Cyclic Group of Order 6: The cyclic group of order 6, denoted by C6, is generated by a single element and follows the operation of addition modulo 6. It is abelian.
3. Direct Product of Cyclic Groups: The direct product of two cyclic groups of order 2 and 3, denoted by C2 x C3, contains elements (0,0), (0,1), (1,0), (1,1), (0,2), (1,2) and follows the operation of component-wise addition modulo 2 and 3. It is abelian.
4. Symmetric Group of 3 Elements: The symmetric group of 3 elements, denoted by S3, consists of all possible permutations of three elements. It is non-abelian.
5. Dihedral Group of Order 6: The dihedral group of order 6, denoted by D6, consists of all possible rotations and reflections of a regular hexagon. It is non-abelian.
Conclusion
Out of the possible groups of order 6, only the symmetric group of 3 elements (S3) and the dihedral group of order 6 (D6) are non-abelian. Therefore, the total number of non-abelian groups of order 6 is 1.
Summary:
- Trivial Group: Abelian
- Cyclic Group of Order 6: Abelian
- Direct Product of Cyclic Groups: Abelian
- Symmetric Group of 3 Elements (S3): Non-abelian
- Dihedral Group of Order 6 (D6): Non-abelian
Hence, there is only 1 non-abelian group of order 6.
To find the total number of non-abelian groups of order 6, we need to consider all the possible groups of this order and determine which ones are non-abelian.
Possible Groups of Order 6
There are several possible groups of order 6. Let's consider each of them and determine whether they are abelian or non-abelian.
1. Trivial Group: The trivial group is the group containing only the identity element. It is abelian.
2. Cyclic Group of Order 6: The cyclic group of order 6, denoted by C6, is generated by a single element and follows the operation of addition modulo 6. It is abelian.
3. Direct Product of Cyclic Groups: The direct product of two cyclic groups of order 2 and 3, denoted by C2 x C3, contains elements (0,0), (0,1), (1,0), (1,1), (0,2), (1,2) and follows the operation of component-wise addition modulo 2 and 3. It is abelian.
4. Symmetric Group of 3 Elements: The symmetric group of 3 elements, denoted by S3, consists of all possible permutations of three elements. It is non-abelian.
5. Dihedral Group of Order 6: The dihedral group of order 6, denoted by D6, consists of all possible rotations and reflections of a regular hexagon. It is non-abelian.
Conclusion
Out of the possible groups of order 6, only the symmetric group of 3 elements (S3) and the dihedral group of order 6 (D6) are non-abelian. Therefore, the total number of non-abelian groups of order 6 is 1.
Summary:
- Trivial Group: Abelian
- Cyclic Group of Order 6: Abelian
- Direct Product of Cyclic Groups: Abelian
- Symmetric Group of 3 Elements (S3): Non-abelian
- Dihedral Group of Order 6 (D6): Non-abelian
Hence, there is only 1 non-abelian group of order 6.

|
Explore Courses for Mathematics exam
|

|
Similar Mathematics Doubts
Find the total no. of all non - abelian groups of order 6.Correct answer is '1'. Can you explain this answer?
Question Description
Find the total no. of all non - abelian groups of order 6.Correct answer is '1'. Can you explain this answer? for Mathematics 2024 is part of Mathematics preparation. The Question and answers have been prepared according to the Mathematics exam syllabus. Information about Find the total no. of all non - abelian groups of order 6.Correct answer is '1'. Can you explain this answer? covers all topics & solutions for Mathematics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Find the total no. of all non - abelian groups of order 6.Correct answer is '1'. Can you explain this answer?.
Find the total no. of all non - abelian groups of order 6.Correct answer is '1'. Can you explain this answer? for Mathematics 2024 is part of Mathematics preparation. The Question and answers have been prepared according to the Mathematics exam syllabus. Information about Find the total no. of all non - abelian groups of order 6.Correct answer is '1'. Can you explain this answer? covers all topics & solutions for Mathematics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Find the total no. of all non - abelian groups of order 6.Correct answer is '1'. Can you explain this answer?.
Solutions for Find the total no. of all non - abelian groups of order 6.Correct answer is '1'. Can you explain this answer? in English & in Hindi are available as part of our courses for Mathematics.
Download more important topics, notes, lectures and mock test series for Mathematics Exam by signing up for free.
Here you can find the meaning of Find the total no. of all non - abelian groups of order 6.Correct answer is '1'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
Find the total no. of all non - abelian groups of order 6.Correct answer is '1'. Can you explain this answer?, a detailed solution for Find the total no. of all non - abelian groups of order 6.Correct answer is '1'. Can you explain this answer? has been provided alongside types of Find the total no. of all non - abelian groups of order 6.Correct answer is '1'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice Find the total no. of all non - abelian groups of order 6.Correct answer is '1'. Can you explain this answer? tests, examples and also practice Mathematics tests.

|
Explore Courses for Mathematics exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















