Defence Exam > Defence Questions > A train moving at a cover a rate of 90 kmph. ...
Start Learning for Free
A train moving at a cover a rate of 90 kmph. Crosses a tunnel in 36 seconds. and other train which is 100 metres shorter moving at a cover a rate of 45 kmph. How much time will it take to go through the tunnel?
- a)54 seconds
- b)64 seconds
- c)50 seconds
- d)58 seconds
Correct answer is option 'B'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
A train moving at a cover a rate of 90 kmph. Crosses a tunnel in 36 se...
Speed of 1st train = 90 km/hr 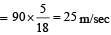
Time taken by 1st train = 36 seconds
∴ Distance = speed × time
= 25 × 36 = 900m
Now
2nd train is 100 meters shorter, then
∴ Distance (900 – 100) = 800m
Speed of 2nd train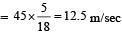
∴ Time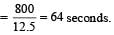
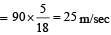
Time taken by 1st train = 36 seconds
∴ Distance = speed × time
= 25 × 36 = 900m
Now
2nd train is 100 meters shorter, then
∴ Distance (900 – 100) = 800m
Speed of 2nd train
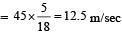
∴ Time
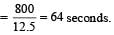
Most Upvoted Answer
A train moving at a cover a rate of 90 kmph. Crosses a tunnel in 36 se...
Problem Statement:
A train moving at a cover a rate of 90 kmph. Crosses a tunnel in 36 seconds. and other train which is 100 metres shorter moving at a cover a rate of 45 kmph. How much time will it take to go through the tunnel?
Solution:
To solve this problem, we need to first find the length of the tunnel. Let's assume that the length of the tunnel is 'x' meters.
Step 1: Find the speed of the first train in meters per second.
Given, the speed of the first train = 90 kmph
Converting kmph to m/s, we get:
90 kmph = (90*1000)/3600 m/s = 25 m/s
Step 2: Find the length of the first train in meters.
We know that the first train crosses the tunnel in 36 seconds. Therefore, the distance covered by the first train in 36 seconds is equal to the length of the tunnel plus the length of the train. Mathematically, we can write:
Distance covered by the first train in 36 seconds = x + length of the first train
We can also write:
Distance = Speed * Time
Therefore, we have:
x + length of the first train = 25 * 36
x + length of the first train = 900
As we don't know the length of the first train, we cannot solve for 'x' at this point.
Step 3: Find the speed of the second train in meters per second.
Given, the speed of the second train = 45 kmph
Converting kmph to m/s, we get:
45 kmph = (45*1000)/3600 m/s = 12.5 m/s
Step 4: Find the length of the second train in meters.
We know that the second train is 100 meters shorter than the first train. Therefore, the length of the second train can be written as:
Length of the second train = Length of the first train - 100 meters
Substituting this in Step 2, we get:
x + (length of the first train - 100) = 12.5 * t
where 't' is the time taken by the second train to cross the tunnel.
Step 5: Solve for 'x' and 't'.
We have two equations:
x + length of the first train = 900
x + (length of the first train - 100) = 12.5 * t
Subtracting the second equation from the first, we get:
100 = 12.5 * t
t = 8 seconds
Therefore, the time taken by the second train to go through the tunnel is 8 seconds.
Final Answer:
Option (B) 64 seconds.
A train moving at a cover a rate of 90 kmph. Crosses a tunnel in 36 seconds. and other train which is 100 metres shorter moving at a cover a rate of 45 kmph. How much time will it take to go through the tunnel?
Solution:
To solve this problem, we need to first find the length of the tunnel. Let's assume that the length of the tunnel is 'x' meters.
Step 1: Find the speed of the first train in meters per second.
Given, the speed of the first train = 90 kmph
Converting kmph to m/s, we get:
90 kmph = (90*1000)/3600 m/s = 25 m/s
Step 2: Find the length of the first train in meters.
We know that the first train crosses the tunnel in 36 seconds. Therefore, the distance covered by the first train in 36 seconds is equal to the length of the tunnel plus the length of the train. Mathematically, we can write:
Distance covered by the first train in 36 seconds = x + length of the first train
We can also write:
Distance = Speed * Time
Therefore, we have:
x + length of the first train = 25 * 36
x + length of the first train = 900
As we don't know the length of the first train, we cannot solve for 'x' at this point.
Step 3: Find the speed of the second train in meters per second.
Given, the speed of the second train = 45 kmph
Converting kmph to m/s, we get:
45 kmph = (45*1000)/3600 m/s = 12.5 m/s
Step 4: Find the length of the second train in meters.
We know that the second train is 100 meters shorter than the first train. Therefore, the length of the second train can be written as:
Length of the second train = Length of the first train - 100 meters
Substituting this in Step 2, we get:
x + (length of the first train - 100) = 12.5 * t
where 't' is the time taken by the second train to cross the tunnel.
Step 5: Solve for 'x' and 't'.
We have two equations:
x + length of the first train = 900
x + (length of the first train - 100) = 12.5 * t
Subtracting the second equation from the first, we get:
100 = 12.5 * t
t = 8 seconds
Therefore, the time taken by the second train to go through the tunnel is 8 seconds.
Final Answer:
Option (B) 64 seconds.

|
Explore Courses for Defence exam
|

|
Similar Defence Doubts
A train moving at a cover a rate of 90 kmph. Crosses a tunnel in 36 seconds. and other train which is 100 metres shorter moving at a cover a rate of 45 kmph. How much time will it take to go through the tunnel?a)54 secondsb)64 secondsc)50 secondsd)58 secondsCorrect answer is option 'B'. Can you explain this answer?
Question Description
A train moving at a cover a rate of 90 kmph. Crosses a tunnel in 36 seconds. and other train which is 100 metres shorter moving at a cover a rate of 45 kmph. How much time will it take to go through the tunnel?a)54 secondsb)64 secondsc)50 secondsd)58 secondsCorrect answer is option 'B'. Can you explain this answer? for Defence 2024 is part of Defence preparation. The Question and answers have been prepared according to the Defence exam syllabus. Information about A train moving at a cover a rate of 90 kmph. Crosses a tunnel in 36 seconds. and other train which is 100 metres shorter moving at a cover a rate of 45 kmph. How much time will it take to go through the tunnel?a)54 secondsb)64 secondsc)50 secondsd)58 secondsCorrect answer is option 'B'. Can you explain this answer? covers all topics & solutions for Defence 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A train moving at a cover a rate of 90 kmph. Crosses a tunnel in 36 seconds. and other train which is 100 metres shorter moving at a cover a rate of 45 kmph. How much time will it take to go through the tunnel?a)54 secondsb)64 secondsc)50 secondsd)58 secondsCorrect answer is option 'B'. Can you explain this answer?.
A train moving at a cover a rate of 90 kmph. Crosses a tunnel in 36 seconds. and other train which is 100 metres shorter moving at a cover a rate of 45 kmph. How much time will it take to go through the tunnel?a)54 secondsb)64 secondsc)50 secondsd)58 secondsCorrect answer is option 'B'. Can you explain this answer? for Defence 2024 is part of Defence preparation. The Question and answers have been prepared according to the Defence exam syllabus. Information about A train moving at a cover a rate of 90 kmph. Crosses a tunnel in 36 seconds. and other train which is 100 metres shorter moving at a cover a rate of 45 kmph. How much time will it take to go through the tunnel?a)54 secondsb)64 secondsc)50 secondsd)58 secondsCorrect answer is option 'B'. Can you explain this answer? covers all topics & solutions for Defence 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A train moving at a cover a rate of 90 kmph. Crosses a tunnel in 36 seconds. and other train which is 100 metres shorter moving at a cover a rate of 45 kmph. How much time will it take to go through the tunnel?a)54 secondsb)64 secondsc)50 secondsd)58 secondsCorrect answer is option 'B'. Can you explain this answer?.
Solutions for A train moving at a cover a rate of 90 kmph. Crosses a tunnel in 36 seconds. and other train which is 100 metres shorter moving at a cover a rate of 45 kmph. How much time will it take to go through the tunnel?a)54 secondsb)64 secondsc)50 secondsd)58 secondsCorrect answer is option 'B'. Can you explain this answer? in English & in Hindi are available as part of our courses for Defence.
Download more important topics, notes, lectures and mock test series for Defence Exam by signing up for free.
Here you can find the meaning of A train moving at a cover a rate of 90 kmph. Crosses a tunnel in 36 seconds. and other train which is 100 metres shorter moving at a cover a rate of 45 kmph. How much time will it take to go through the tunnel?a)54 secondsb)64 secondsc)50 secondsd)58 secondsCorrect answer is option 'B'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A train moving at a cover a rate of 90 kmph. Crosses a tunnel in 36 seconds. and other train which is 100 metres shorter moving at a cover a rate of 45 kmph. How much time will it take to go through the tunnel?a)54 secondsb)64 secondsc)50 secondsd)58 secondsCorrect answer is option 'B'. Can you explain this answer?, a detailed solution for A train moving at a cover a rate of 90 kmph. Crosses a tunnel in 36 seconds. and other train which is 100 metres shorter moving at a cover a rate of 45 kmph. How much time will it take to go through the tunnel?a)54 secondsb)64 secondsc)50 secondsd)58 secondsCorrect answer is option 'B'. Can you explain this answer? has been provided alongside types of A train moving at a cover a rate of 90 kmph. Crosses a tunnel in 36 seconds. and other train which is 100 metres shorter moving at a cover a rate of 45 kmph. How much time will it take to go through the tunnel?a)54 secondsb)64 secondsc)50 secondsd)58 secondsCorrect answer is option 'B'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A train moving at a cover a rate of 90 kmph. Crosses a tunnel in 36 seconds. and other train which is 100 metres shorter moving at a cover a rate of 45 kmph. How much time will it take to go through the tunnel?a)54 secondsb)64 secondsc)50 secondsd)58 secondsCorrect answer is option 'B'. Can you explain this answer? tests, examples and also practice Defence tests.

|
Explore Courses for Defence exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















