Physics Exam > Physics Questions > Calculate the percentage contraction of a rod...
Start Learning for Free
Calculate the percentage contraction of a rod moving with a velocity 0.8c in a direction inclined at 60o to its own length.
- a)8%
- b)9%
- c)6%
- d)7%
Correct answer is option 'B'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
Calculate the percentage contraction of a rod moving with a velocity 0...
Here's the solution to your question:
We know the observed length in moving frame
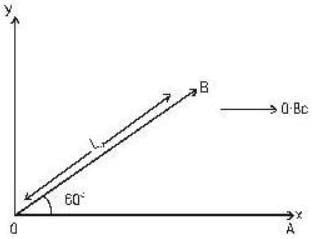
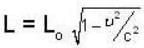
So,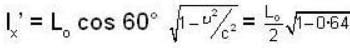
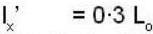
Similarly ly’ = L0 Sin 60�
So, L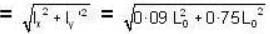
L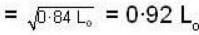
So, precentral Contraction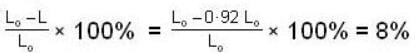

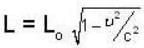
So,
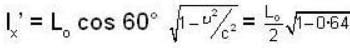
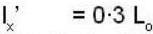
Similarly ly’ = L0 Sin 60�
So, L
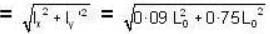
L
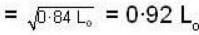
So, precentral Contraction
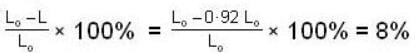
Hence, the correct answer is Option A
You can learn all the concepts of IIT JAM Physics through the link:
You can learn all the concepts of IIT JAM Physics through the link:
Most Upvoted Answer
Calculate the percentage contraction of a rod moving with a velocity 0...
To calculate the percentage contraction of a rod moving with a velocity of 0.8c (where c is the speed of light) in a direction inclined at 60 degrees to its own length, we can use the concept of length contraction.
Length contraction is a phenomenon predicted by Einstein's theory of special relativity. According to this theory, objects moving at relativistic speeds will appear contracted along the direction of motion when observed from a stationary reference frame.
Let's break down the solution into steps:
Step 1: Determine the velocity of the rod relative to the observer
The given velocity is 0.8c, where c is the speed of light. This means the rod is moving at 0.8 times the speed of light relative to the observer.
Step 2: Calculate the Lorentz factor (γ)
The Lorentz factor is given by the equation γ = 1 / √(1 - (v^2 / c^2)), where v is the velocity of the object and c is the speed of light.
In this case, v = 0.8c. Plugging in the values, we get:
γ = 1 / √(1 - (0.8c)^2 / c^2)
= 1 / √(1 - 0.64)
= 1 / √(0.36)
= 1 / 0.6
= 1.67
Step 3: Calculate the contraction factor (β)
The contraction factor is given by the equation β = √(1 - (1/γ^2))
Plugging in the value of γ, we get:
β = √(1 - (1/1.67^2))
= √(1 - 1/2.7889)
= √(1 - 0.358)
= √(0.642)
= 0.802
Step 4: Calculate the percentage contraction
The percentage contraction is given by the equation percentage contraction = (1 - β) * 100
Plugging in the value of β, we get:
percentage contraction = (1 - 0.802) * 100
= 0.198 * 100
= 19.8%
Therefore, the percentage contraction of the rod moving with a velocity of 0.8c in a direction inclined at 60 degrees to its own length is approximately 19.8%.
Length contraction is a phenomenon predicted by Einstein's theory of special relativity. According to this theory, objects moving at relativistic speeds will appear contracted along the direction of motion when observed from a stationary reference frame.
Let's break down the solution into steps:
Step 1: Determine the velocity of the rod relative to the observer
The given velocity is 0.8c, where c is the speed of light. This means the rod is moving at 0.8 times the speed of light relative to the observer.
Step 2: Calculate the Lorentz factor (γ)
The Lorentz factor is given by the equation γ = 1 / √(1 - (v^2 / c^2)), where v is the velocity of the object and c is the speed of light.
In this case, v = 0.8c. Plugging in the values, we get:
γ = 1 / √(1 - (0.8c)^2 / c^2)
= 1 / √(1 - 0.64)
= 1 / √(0.36)
= 1 / 0.6
= 1.67
Step 3: Calculate the contraction factor (β)
The contraction factor is given by the equation β = √(1 - (1/γ^2))
Plugging in the value of γ, we get:
β = √(1 - (1/1.67^2))
= √(1 - 1/2.7889)
= √(1 - 0.358)
= √(0.642)
= 0.802
Step 4: Calculate the percentage contraction
The percentage contraction is given by the equation percentage contraction = (1 - β) * 100
Plugging in the value of β, we get:
percentage contraction = (1 - 0.802) * 100
= 0.198 * 100
= 19.8%
Therefore, the percentage contraction of the rod moving with a velocity of 0.8c in a direction inclined at 60 degrees to its own length is approximately 19.8%.

|
Explore Courses for Physics exam
|

|
Similar Physics Doubts
Calculate the percentage contraction of a rod moving with a velocity 0.8c in a direction inclined at 60o to its own length.a)8%b)9%c)6%d)7%Correct answer is option 'B'. Can you explain this answer?
Question Description
Calculate the percentage contraction of a rod moving with a velocity 0.8c in a direction inclined at 60o to its own length.a)8%b)9%c)6%d)7%Correct answer is option 'B'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about Calculate the percentage contraction of a rod moving with a velocity 0.8c in a direction inclined at 60o to its own length.a)8%b)9%c)6%d)7%Correct answer is option 'B'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Calculate the percentage contraction of a rod moving with a velocity 0.8c in a direction inclined at 60o to its own length.a)8%b)9%c)6%d)7%Correct answer is option 'B'. Can you explain this answer?.
Calculate the percentage contraction of a rod moving with a velocity 0.8c in a direction inclined at 60o to its own length.a)8%b)9%c)6%d)7%Correct answer is option 'B'. Can you explain this answer? for Physics 2024 is part of Physics preparation. The Question and answers have been prepared according to the Physics exam syllabus. Information about Calculate the percentage contraction of a rod moving with a velocity 0.8c in a direction inclined at 60o to its own length.a)8%b)9%c)6%d)7%Correct answer is option 'B'. Can you explain this answer? covers all topics & solutions for Physics 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Calculate the percentage contraction of a rod moving with a velocity 0.8c in a direction inclined at 60o to its own length.a)8%b)9%c)6%d)7%Correct answer is option 'B'. Can you explain this answer?.
Solutions for Calculate the percentage contraction of a rod moving with a velocity 0.8c in a direction inclined at 60o to its own length.a)8%b)9%c)6%d)7%Correct answer is option 'B'. Can you explain this answer? in English & in Hindi are available as part of our courses for Physics.
Download more important topics, notes, lectures and mock test series for Physics Exam by signing up for free.
Here you can find the meaning of Calculate the percentage contraction of a rod moving with a velocity 0.8c in a direction inclined at 60o to its own length.a)8%b)9%c)6%d)7%Correct answer is option 'B'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
Calculate the percentage contraction of a rod moving with a velocity 0.8c in a direction inclined at 60o to its own length.a)8%b)9%c)6%d)7%Correct answer is option 'B'. Can you explain this answer?, a detailed solution for Calculate the percentage contraction of a rod moving with a velocity 0.8c in a direction inclined at 60o to its own length.a)8%b)9%c)6%d)7%Correct answer is option 'B'. Can you explain this answer? has been provided alongside types of Calculate the percentage contraction of a rod moving with a velocity 0.8c in a direction inclined at 60o to its own length.a)8%b)9%c)6%d)7%Correct answer is option 'B'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice Calculate the percentage contraction of a rod moving with a velocity 0.8c in a direction inclined at 60o to its own length.a)8%b)9%c)6%d)7%Correct answer is option 'B'. Can you explain this answer? tests, examples and also practice Physics tests.

|
Explore Courses for Physics exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.



















