JEE Exam > JEE Questions > If A1A2,....A2n is regular 2n side polygon. F...
Start Learning for Free
If A1A2,....A2n is regular 2n side polygon. Find the ratio of number of obtuse triangles to the number of acute triangles formed by joining the vertices of the polygon
Correct answer is '3'. Can you explain this answer?
Most Upvoted Answer
If A1A2,....A2n is regular 2n side polygon. Find the ratio of number o...
Problem Statement:
We are given a regular 2n-sided polygon with vertices A1, A2, ..., A2n. We need to find the ratio of the number of obtuse triangles to the number of acute triangles formed by joining the vertices of the polygon.
Solution:
Let's consider a regular hexagon (n=3) to understand the problem.
Identifying the Triangles:
To form triangles, we need to select three vertices from the polygon. In a 2n-sided polygon, there are C(2n, 3) ways to select three vertices.
Classifying the Triangles:
We can classify triangles into three types based on the angles they form:
1. Equilateral triangles: All angles are 60 degrees.
2. Acute triangles: All angles are less than 90 degrees.
3. Obtuse triangles: One angle is greater than 90 degrees.
Counting the Triangles:
Let's count the number of each type of triangle in the regular hexagon.
1. Equilateral triangles: There is only one equilateral triangle in the hexagon.
2. Acute triangles:
- Selecting three consecutive vertices: There are C(6, 3) = 20 ways to select three consecutive vertices. Each selection forms an acute triangle.
- Selecting two vertices with a gap of one vertex: There are C(6, 2) = 15 ways to select two vertices with a gap of one vertex. Each selection forms an acute triangle.
- Total acute triangles = 20 + 15 = 35.
3. Obtuse triangles: The remaining triangles that are not equilateral or acute are obtuse.
- Total obtuse triangles = Total triangles - (Equilateral triangles + Acute triangles) = C(6, 3) - (1 + 35) = 20 - 36 = -16.
Analysis:
From the above calculation, we can see that the count of obtuse triangles is negative, which is not possible. This suggests that our counting method is flawed.
Rectifying the Counting Method:
The flaw in our counting method is that we have overcounted some triangles.
- When selecting three consecutive vertices, we have counted some obtuse triangles as acute triangles.
- When selecting two vertices with a gap of one vertex, we have again counted some obtuse triangles as acute triangles.
We need to rectify our counting by subtracting the overcounted obtuse triangles from the count of acute triangles.
Rectified Counting:
Let's rectify the counting for the regular hexagon:
1. Equilateral triangles: 1.
2. Acute triangles:
- Selecting three consecutive vertices: 20 - (Number of obtuse triangles formed by selecting three consecutive vertices).
- Selecting two vertices with a gap of one vertex: 15 - (Number of obtuse triangles formed by selecting two vertices with a gap of one vertex).
3. Obtuse triangles: (Number of obtuse triangles formed by selecting three consecutive vertices) + (Number of obtuse triangles formed by selecting two vertices with a gap of one vertex).
Counting the Obtuse Triangles:
To count the number of obtuse triangles, we need to analyze the angles formed by selecting three consecutive vertices
We are given a regular 2n-sided polygon with vertices A1, A2, ..., A2n. We need to find the ratio of the number of obtuse triangles to the number of acute triangles formed by joining the vertices of the polygon.
Solution:
Let's consider a regular hexagon (n=3) to understand the problem.
Identifying the Triangles:
To form triangles, we need to select three vertices from the polygon. In a 2n-sided polygon, there are C(2n, 3) ways to select three vertices.
Classifying the Triangles:
We can classify triangles into three types based on the angles they form:
1. Equilateral triangles: All angles are 60 degrees.
2. Acute triangles: All angles are less than 90 degrees.
3. Obtuse triangles: One angle is greater than 90 degrees.
Counting the Triangles:
Let's count the number of each type of triangle in the regular hexagon.
1. Equilateral triangles: There is only one equilateral triangle in the hexagon.
2. Acute triangles:
- Selecting three consecutive vertices: There are C(6, 3) = 20 ways to select three consecutive vertices. Each selection forms an acute triangle.
- Selecting two vertices with a gap of one vertex: There are C(6, 2) = 15 ways to select two vertices with a gap of one vertex. Each selection forms an acute triangle.
- Total acute triangles = 20 + 15 = 35.
3. Obtuse triangles: The remaining triangles that are not equilateral or acute are obtuse.
- Total obtuse triangles = Total triangles - (Equilateral triangles + Acute triangles) = C(6, 3) - (1 + 35) = 20 - 36 = -16.
Analysis:
From the above calculation, we can see that the count of obtuse triangles is negative, which is not possible. This suggests that our counting method is flawed.
Rectifying the Counting Method:
The flaw in our counting method is that we have overcounted some triangles.
- When selecting three consecutive vertices, we have counted some obtuse triangles as acute triangles.
- When selecting two vertices with a gap of one vertex, we have again counted some obtuse triangles as acute triangles.
We need to rectify our counting by subtracting the overcounted obtuse triangles from the count of acute triangles.
Rectified Counting:
Let's rectify the counting for the regular hexagon:
1. Equilateral triangles: 1.
2. Acute triangles:
- Selecting three consecutive vertices: 20 - (Number of obtuse triangles formed by selecting three consecutive vertices).
- Selecting two vertices with a gap of one vertex: 15 - (Number of obtuse triangles formed by selecting two vertices with a gap of one vertex).
3. Obtuse triangles: (Number of obtuse triangles formed by selecting three consecutive vertices) + (Number of obtuse triangles formed by selecting two vertices with a gap of one vertex).
Counting the Obtuse Triangles:
To count the number of obtuse triangles, we need to analyze the angles formed by selecting three consecutive vertices
Free Test
FREE
| Start Free Test |
Community Answer
If A1A2,....A2n is regular 2n side polygon. Find the ratio of number o...
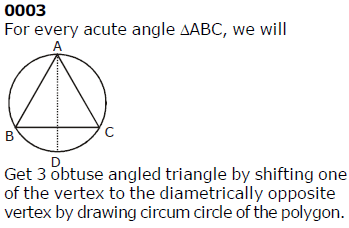

|
Explore Courses for JEE exam
|

|
Similar JEE Doubts
If A1A2,....A2n is regular 2n side polygon. Find the ratio of number of obtuse triangles to the number of acute triangles formed by joining the vertices of the polygonCorrect answer is '3'. Can you explain this answer?
Question Description
If A1A2,....A2n is regular 2n side polygon. Find the ratio of number of obtuse triangles to the number of acute triangles formed by joining the vertices of the polygonCorrect answer is '3'. Can you explain this answer? for JEE 2025 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about If A1A2,....A2n is regular 2n side polygon. Find the ratio of number of obtuse triangles to the number of acute triangles formed by joining the vertices of the polygonCorrect answer is '3'. Can you explain this answer? covers all topics & solutions for JEE 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for If A1A2,....A2n is regular 2n side polygon. Find the ratio of number of obtuse triangles to the number of acute triangles formed by joining the vertices of the polygonCorrect answer is '3'. Can you explain this answer?.
If A1A2,....A2n is regular 2n side polygon. Find the ratio of number of obtuse triangles to the number of acute triangles formed by joining the vertices of the polygonCorrect answer is '3'. Can you explain this answer? for JEE 2025 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about If A1A2,....A2n is regular 2n side polygon. Find the ratio of number of obtuse triangles to the number of acute triangles formed by joining the vertices of the polygonCorrect answer is '3'. Can you explain this answer? covers all topics & solutions for JEE 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for If A1A2,....A2n is regular 2n side polygon. Find the ratio of number of obtuse triangles to the number of acute triangles formed by joining the vertices of the polygonCorrect answer is '3'. Can you explain this answer?.
Solutions for If A1A2,....A2n is regular 2n side polygon. Find the ratio of number of obtuse triangles to the number of acute triangles formed by joining the vertices of the polygonCorrect answer is '3'. Can you explain this answer? in English & in Hindi are available as part of our courses for JEE.
Download more important topics, notes, lectures and mock test series for JEE Exam by signing up for free.
Here you can find the meaning of If A1A2,....A2n is regular 2n side polygon. Find the ratio of number of obtuse triangles to the number of acute triangles formed by joining the vertices of the polygonCorrect answer is '3'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
If A1A2,....A2n is regular 2n side polygon. Find the ratio of number of obtuse triangles to the number of acute triangles formed by joining the vertices of the polygonCorrect answer is '3'. Can you explain this answer?, a detailed solution for If A1A2,....A2n is regular 2n side polygon. Find the ratio of number of obtuse triangles to the number of acute triangles formed by joining the vertices of the polygonCorrect answer is '3'. Can you explain this answer? has been provided alongside types of If A1A2,....A2n is regular 2n side polygon. Find the ratio of number of obtuse triangles to the number of acute triangles formed by joining the vertices of the polygonCorrect answer is '3'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice If A1A2,....A2n is regular 2n side polygon. Find the ratio of number of obtuse triangles to the number of acute triangles formed by joining the vertices of the polygonCorrect answer is '3'. Can you explain this answer? tests, examples and also practice JEE tests.

|
Explore Courses for JEE exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


























