Electrical Engineering (EE) Exam > Electrical Engineering (EE) Questions > The peak overshoot of step-input response of ...
Start Learning for Free
The peak overshoot of step-input response of an underdamped second-order system is an indication of
- a)rise time of the system
- b)settling time of the system
- c)damping ratio of the system
- d)natural frequency of the system
Correct answer is option 'C'. Can you explain this answer?
Verified Answer
The peak overshoot of step-input response of an underdamped second-ord...
If damping of system increases, peak overshoot Mp decreases and vice-versa.
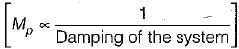
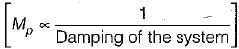
Most Upvoted Answer
The peak overshoot of step-input response of an underdamped second-ord...
Introduction:
In control systems, the step-input response is a common method to analyze the behavior of a system. The response of a second-order system to a step input is characterized by certain parameters, one of which is the peak overshoot. The peak overshoot represents the maximum deviation of the response from its final steady-state value. The magnitude of the peak overshoot is influenced by various factors, including the damping ratio of the system.
Damping Ratio:
The damping ratio, denoted by ζ (zeta), is a dimensionless parameter that quantifies the level of damping in a second-order system. It describes the behavior of the system in relation to its natural frequency and provides insight into the system's response characteristics. The damping ratio can take values between 0 and 1, representing different types of damping.
Relationship with Peak Overshoot:
The peak overshoot of a step-input response is directly related to the damping ratio of the system. Here's how the two are connected:
1. Underdamped System: An underdamped system has a damping ratio less than 1 (0 < ζ="" />< 1)="" and="" exhibits="" oscillatory="" behavior="" in="" its="" response.="" this="" means="" that="" the="" system="" overshoots="" its="" steady-state="" value="" before="" settling="" down.="" the="" magnitude="" of="" the="" peak="" overshoot="" is="" directly="" proportional="" to="" the="" damping="" />
2. Overdamped System: An overdamped system has a damping ratio greater than 1 (ζ > 1) and does not exhibit oscillatory behavior. Instead, it approaches its steady-state value without overshooting. In this case, the peak overshoot is zero.
3. Critically Damped System: A critically damped system has a damping ratio equal to 1 (ζ = 1). It also does not exhibit overshoot and approaches its steady-state value in the fastest possible time.
Conclusion:
From the above discussion, it is evident that the peak overshoot of the step-input response of an underdamped second-order system is an indication of the damping ratio of the system. The damping ratio determines the level of oscillation and overshoot in the system's response. Higher damping ratios result in smaller peak overshoots, while lower damping ratios lead to larger peak overshoots. Therefore, by analyzing the magnitude of the peak overshoot, one can infer the damping ratio and gain insights into the system's dynamic behavior.
In control systems, the step-input response is a common method to analyze the behavior of a system. The response of a second-order system to a step input is characterized by certain parameters, one of which is the peak overshoot. The peak overshoot represents the maximum deviation of the response from its final steady-state value. The magnitude of the peak overshoot is influenced by various factors, including the damping ratio of the system.
Damping Ratio:
The damping ratio, denoted by ζ (zeta), is a dimensionless parameter that quantifies the level of damping in a second-order system. It describes the behavior of the system in relation to its natural frequency and provides insight into the system's response characteristics. The damping ratio can take values between 0 and 1, representing different types of damping.
Relationship with Peak Overshoot:
The peak overshoot of a step-input response is directly related to the damping ratio of the system. Here's how the two are connected:
1. Underdamped System: An underdamped system has a damping ratio less than 1 (0 < ζ="" />< 1)="" and="" exhibits="" oscillatory="" behavior="" in="" its="" response.="" this="" means="" that="" the="" system="" overshoots="" its="" steady-state="" value="" before="" settling="" down.="" the="" magnitude="" of="" the="" peak="" overshoot="" is="" directly="" proportional="" to="" the="" damping="" />
2. Overdamped System: An overdamped system has a damping ratio greater than 1 (ζ > 1) and does not exhibit oscillatory behavior. Instead, it approaches its steady-state value without overshooting. In this case, the peak overshoot is zero.
3. Critically Damped System: A critically damped system has a damping ratio equal to 1 (ζ = 1). It also does not exhibit overshoot and approaches its steady-state value in the fastest possible time.
Conclusion:
From the above discussion, it is evident that the peak overshoot of the step-input response of an underdamped second-order system is an indication of the damping ratio of the system. The damping ratio determines the level of oscillation and overshoot in the system's response. Higher damping ratios result in smaller peak overshoots, while lower damping ratios lead to larger peak overshoots. Therefore, by analyzing the magnitude of the peak overshoot, one can infer the damping ratio and gain insights into the system's dynamic behavior.

|
Explore Courses for Electrical Engineering (EE) exam
|

|
Question Description
The peak overshoot of step-input response of an underdamped second-order system is an indication ofa)rise time of the systemb)settling time of the systemc)damping ratio of the systemd)natural frequency of the systemCorrect answer is option 'C'. Can you explain this answer? for Electrical Engineering (EE) 2025 is part of Electrical Engineering (EE) preparation. The Question and answers have been prepared according to the Electrical Engineering (EE) exam syllabus. Information about The peak overshoot of step-input response of an underdamped second-order system is an indication ofa)rise time of the systemb)settling time of the systemc)damping ratio of the systemd)natural frequency of the systemCorrect answer is option 'C'. Can you explain this answer? covers all topics & solutions for Electrical Engineering (EE) 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The peak overshoot of step-input response of an underdamped second-order system is an indication ofa)rise time of the systemb)settling time of the systemc)damping ratio of the systemd)natural frequency of the systemCorrect answer is option 'C'. Can you explain this answer?.
The peak overshoot of step-input response of an underdamped second-order system is an indication ofa)rise time of the systemb)settling time of the systemc)damping ratio of the systemd)natural frequency of the systemCorrect answer is option 'C'. Can you explain this answer? for Electrical Engineering (EE) 2025 is part of Electrical Engineering (EE) preparation. The Question and answers have been prepared according to the Electrical Engineering (EE) exam syllabus. Information about The peak overshoot of step-input response of an underdamped second-order system is an indication ofa)rise time of the systemb)settling time of the systemc)damping ratio of the systemd)natural frequency of the systemCorrect answer is option 'C'. Can you explain this answer? covers all topics & solutions for Electrical Engineering (EE) 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The peak overshoot of step-input response of an underdamped second-order system is an indication ofa)rise time of the systemb)settling time of the systemc)damping ratio of the systemd)natural frequency of the systemCorrect answer is option 'C'. Can you explain this answer?.
Solutions for The peak overshoot of step-input response of an underdamped second-order system is an indication ofa)rise time of the systemb)settling time of the systemc)damping ratio of the systemd)natural frequency of the systemCorrect answer is option 'C'. Can you explain this answer? in English & in Hindi are available as part of our courses for Electrical Engineering (EE).
Download more important topics, notes, lectures and mock test series for Electrical Engineering (EE) Exam by signing up for free.
Here you can find the meaning of The peak overshoot of step-input response of an underdamped second-order system is an indication ofa)rise time of the systemb)settling time of the systemc)damping ratio of the systemd)natural frequency of the systemCorrect answer is option 'C'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
The peak overshoot of step-input response of an underdamped second-order system is an indication ofa)rise time of the systemb)settling time of the systemc)damping ratio of the systemd)natural frequency of the systemCorrect answer is option 'C'. Can you explain this answer?, a detailed solution for The peak overshoot of step-input response of an underdamped second-order system is an indication ofa)rise time of the systemb)settling time of the systemc)damping ratio of the systemd)natural frequency of the systemCorrect answer is option 'C'. Can you explain this answer? has been provided alongside types of The peak overshoot of step-input response of an underdamped second-order system is an indication ofa)rise time of the systemb)settling time of the systemc)damping ratio of the systemd)natural frequency of the systemCorrect answer is option 'C'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice The peak overshoot of step-input response of an underdamped second-order system is an indication ofa)rise time of the systemb)settling time of the systemc)damping ratio of the systemd)natural frequency of the systemCorrect answer is option 'C'. Can you explain this answer? tests, examples and also practice Electrical Engineering (EE) tests.

|
Explore Courses for Electrical Engineering (EE) exam
|

|
Signup to solve all Doubts
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.





















