JEE Exam > JEE Questions > In a chemical reaction a substance changes in...
Start Learning for Free
In a chemical reaction a substance changes into another such that the rate of decomposition of a chemical substance x present at instant t is proportional to x itself i.e. amount of unchanged substance still present . If half of the substance present initially has been converted at the end of 1 minute then the time at the end of which 99 % of the substance will have changed is
- a)log2 10 minute
- b)log2 99 minute
- c)log2 50 minute
- d)log2 100 minute
Correct answer is option 'D'. Can you explain this answer?
Verified Answer
In a chemical reaction a substance changes into another such that the ...
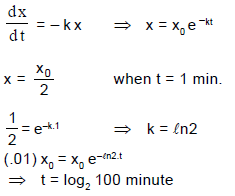
Most Upvoted Answer
In a chemical reaction a substance changes into another such that the ...
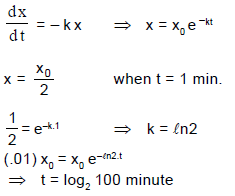
Free Test
FREE
| Start Free Test |
Community Answer
In a chemical reaction a substance changes into another such that the ...
Given information:
A chemical reaction follows first-order kinetics, where the rate of decomposition of a chemical substance x at any instant t is proportional to the amount of unchanged substance still present.
At the end of 1 minute, half of the substance present initially has been converted.
Understanding the problem:
We need to find the time at which 99% of the substance will have changed.
Let's assume the initial amount of substance x is X.
Given that at the end of 1 minute, half of the substance has been converted, we can express this as:
X/2 = X * e^(-k*1)
Where k is the rate constant.
Solving the problem:
We can rewrite the equation as:
e^(-k) = 1/2
Taking the natural logarithm on both sides, we get:
-k = ln(1/2)
k = -ln(1/2)
Now, we need to find the time at which 99% of the substance will have changed.
Let's assume the time required for 99% change is t.
At time t, the amount of substance x remaining will be X * e^(-k*t).
We can express this as:
X * e^(-k*t) = 0.01X
Dividing both sides by X, we get:
e^(-k*t) = 0.01
Taking the natural logarithm on both sides, we get:
-k*t = ln(0.01)
Substituting the value of k, we have:
-(-ln(1/2))*t = ln(0.01)
t = ln(0.01)/ln(1/2)
t = -ln(0.01)/ln(2)
t ≈ 6.64
Answer:
The time at which 99% of the substance will have changed is approximately log2 100 minutes (option D).

|
Explore Courses for JEE exam
|

|
Similar JEE Doubts
In a chemical reaction a substance changes into another such that the rate of decomposition of a chemical substance x present at instant t is proportional to x itself i.e. amount of unchanged substance still present . If half of the substance present initially has been converted at the end of 1 minute then the time at the end of which 99 % of the substance will have changed isa)log2 10 minuteb)log299 minutec)log2 50 minuted)log2 100 minuteCorrect answer is option 'D'. Can you explain this answer?
Question Description
In a chemical reaction a substance changes into another such that the rate of decomposition of a chemical substance x present at instant t is proportional to x itself i.e. amount of unchanged substance still present . If half of the substance present initially has been converted at the end of 1 minute then the time at the end of which 99 % of the substance will have changed isa)log2 10 minuteb)log299 minutec)log2 50 minuted)log2 100 minuteCorrect answer is option 'D'. Can you explain this answer? for JEE 2025 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about In a chemical reaction a substance changes into another such that the rate of decomposition of a chemical substance x present at instant t is proportional to x itself i.e. amount of unchanged substance still present . If half of the substance present initially has been converted at the end of 1 minute then the time at the end of which 99 % of the substance will have changed isa)log2 10 minuteb)log299 minutec)log2 50 minuted)log2 100 minuteCorrect answer is option 'D'. Can you explain this answer? covers all topics & solutions for JEE 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for In a chemical reaction a substance changes into another such that the rate of decomposition of a chemical substance x present at instant t is proportional to x itself i.e. amount of unchanged substance still present . If half of the substance present initially has been converted at the end of 1 minute then the time at the end of which 99 % of the substance will have changed isa)log2 10 minuteb)log299 minutec)log2 50 minuted)log2 100 minuteCorrect answer is option 'D'. Can you explain this answer?.
In a chemical reaction a substance changes into another such that the rate of decomposition of a chemical substance x present at instant t is proportional to x itself i.e. amount of unchanged substance still present . If half of the substance present initially has been converted at the end of 1 minute then the time at the end of which 99 % of the substance will have changed isa)log2 10 minuteb)log299 minutec)log2 50 minuted)log2 100 minuteCorrect answer is option 'D'. Can you explain this answer? for JEE 2025 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about In a chemical reaction a substance changes into another such that the rate of decomposition of a chemical substance x present at instant t is proportional to x itself i.e. amount of unchanged substance still present . If half of the substance present initially has been converted at the end of 1 minute then the time at the end of which 99 % of the substance will have changed isa)log2 10 minuteb)log299 minutec)log2 50 minuted)log2 100 minuteCorrect answer is option 'D'. Can you explain this answer? covers all topics & solutions for JEE 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for In a chemical reaction a substance changes into another such that the rate of decomposition of a chemical substance x present at instant t is proportional to x itself i.e. amount of unchanged substance still present . If half of the substance present initially has been converted at the end of 1 minute then the time at the end of which 99 % of the substance will have changed isa)log2 10 minuteb)log299 minutec)log2 50 minuted)log2 100 minuteCorrect answer is option 'D'. Can you explain this answer?.
Solutions for In a chemical reaction a substance changes into another such that the rate of decomposition of a chemical substance x present at instant t is proportional to x itself i.e. amount of unchanged substance still present . If half of the substance present initially has been converted at the end of 1 minute then the time at the end of which 99 % of the substance will have changed isa)log2 10 minuteb)log299 minutec)log2 50 minuted)log2 100 minuteCorrect answer is option 'D'. Can you explain this answer? in English & in Hindi are available as part of our courses for JEE.
Download more important topics, notes, lectures and mock test series for JEE Exam by signing up for free.
Here you can find the meaning of In a chemical reaction a substance changes into another such that the rate of decomposition of a chemical substance x present at instant t is proportional to x itself i.e. amount of unchanged substance still present . If half of the substance present initially has been converted at the end of 1 minute then the time at the end of which 99 % of the substance will have changed isa)log2 10 minuteb)log299 minutec)log2 50 minuted)log2 100 minuteCorrect answer is option 'D'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
In a chemical reaction a substance changes into another such that the rate of decomposition of a chemical substance x present at instant t is proportional to x itself i.e. amount of unchanged substance still present . If half of the substance present initially has been converted at the end of 1 minute then the time at the end of which 99 % of the substance will have changed isa)log2 10 minuteb)log299 minutec)log2 50 minuted)log2 100 minuteCorrect answer is option 'D'. Can you explain this answer?, a detailed solution for In a chemical reaction a substance changes into another such that the rate of decomposition of a chemical substance x present at instant t is proportional to x itself i.e. amount of unchanged substance still present . If half of the substance present initially has been converted at the end of 1 minute then the time at the end of which 99 % of the substance will have changed isa)log2 10 minuteb)log299 minutec)log2 50 minuted)log2 100 minuteCorrect answer is option 'D'. Can you explain this answer? has been provided alongside types of In a chemical reaction a substance changes into another such that the rate of decomposition of a chemical substance x present at instant t is proportional to x itself i.e. amount of unchanged substance still present . If half of the substance present initially has been converted at the end of 1 minute then the time at the end of which 99 % of the substance will have changed isa)log2 10 minuteb)log299 minutec)log2 50 minuted)log2 100 minuteCorrect answer is option 'D'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice In a chemical reaction a substance changes into another such that the rate of decomposition of a chemical substance x present at instant t is proportional to x itself i.e. amount of unchanged substance still present . If half of the substance present initially has been converted at the end of 1 minute then the time at the end of which 99 % of the substance will have changed isa)log2 10 minuteb)log299 minutec)log2 50 minuted)log2 100 minuteCorrect answer is option 'D'. Can you explain this answer? tests, examples and also practice JEE tests.

|
Explore Courses for JEE exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
























