Mechanical Engineering Exam > Mechanical Engineering Questions > A cantilever beam of uniform cross-section a...
Start Learning for Free
A cantilever beam of uniform cross-section and length L is subjected to upward force W at mid-span and downward force P at the free end. For the deflection to be zero at the free end. W and P are related by
- a)W = 2P
- b)W = 16P/5
- c)W = 3P
- d)W = 5P
Correct answer is option 'B'. Can you explain this answer?
Verified Answer
A cantilever beam of uniform cross-section and length L is subjected ...
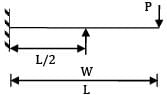
Deflection at free end due to load, p = 

Deflection at free end due to support load W
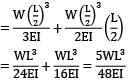
∴ The deflection at free end is zero
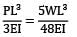
W = 16/5P
Most Upvoted Answer
A cantilever beam of uniform cross-section and length L is subjected ...
To determine the relationship between the upward force W and the downward force P for the deflection to be zero at the free end of the cantilever beam, we can analyze the equilibrium conditions and apply the principles of structural mechanics.
Let's break down the problem step by step:
1. Equilibrium conditions:
- The sum of all vertical forces acting on the beam must equal zero.
- The sum of all moments about any point on the beam must equal zero.
2. Deflection at the free end:
- If the deflection at the free end is zero, it means that the beam is in static equilibrium, and the downward force P is balanced by the upward force W.
3. Equilibrium equations:
- From the equilibrium conditions, we can write the following equations:
ΣFy = 0 (sum of vertical forces)
ΣM = 0 (sum of moments)
4. Vertical forces:
- There are two vertical forces acting on the beam: the upward force W and the downward force P.
- The upward force W is applied at the mid-span of the beam.
- The downward force P is applied at the free end of the beam.
- Therefore, the sum of vertical forces can be written as:
W - P = 0
5. Moments:
- To simplify the analysis, let's consider the mid-span of the beam as the reference point for calculating moments.
- The moment generated by the upward force W at the mid-span is:
Mw = W * (L/2)
- The moment generated by the downward force P at the free end is:
Mp = P * L
- The sum of moments can be written as:
Mw - Mp = 0
6. Solving the equations:
- From equation (4), we have W - P = 0, which implies W = P.
- Substituting W = P into equation (6), we have:
Mw - Mp = 0
W * (L/2) - P * L = 0
(L/2) * W - L * P = 0
- Dividing both sides of the equation by L, we get:
(1/2) * W - P = 0
- Rearranging the equation, we obtain:
W = 2P
Therefore, the relationship between the upward force W and the downward force P for the deflection to be zero at the free end of the cantilever beam is W = 2P. Hence, option 'B' is the correct answer.
Let's break down the problem step by step:
1. Equilibrium conditions:
- The sum of all vertical forces acting on the beam must equal zero.
- The sum of all moments about any point on the beam must equal zero.
2. Deflection at the free end:
- If the deflection at the free end is zero, it means that the beam is in static equilibrium, and the downward force P is balanced by the upward force W.
3. Equilibrium equations:
- From the equilibrium conditions, we can write the following equations:
ΣFy = 0 (sum of vertical forces)
ΣM = 0 (sum of moments)
4. Vertical forces:
- There are two vertical forces acting on the beam: the upward force W and the downward force P.
- The upward force W is applied at the mid-span of the beam.
- The downward force P is applied at the free end of the beam.
- Therefore, the sum of vertical forces can be written as:
W - P = 0
5. Moments:
- To simplify the analysis, let's consider the mid-span of the beam as the reference point for calculating moments.
- The moment generated by the upward force W at the mid-span is:
Mw = W * (L/2)
- The moment generated by the downward force P at the free end is:
Mp = P * L
- The sum of moments can be written as:
Mw - Mp = 0
6. Solving the equations:
- From equation (4), we have W - P = 0, which implies W = P.
- Substituting W = P into equation (6), we have:
Mw - Mp = 0
W * (L/2) - P * L = 0
(L/2) * W - L * P = 0
- Dividing both sides of the equation by L, we get:
(1/2) * W - P = 0
- Rearranging the equation, we obtain:
W = 2P
Therefore, the relationship between the upward force W and the downward force P for the deflection to be zero at the free end of the cantilever beam is W = 2P. Hence, option 'B' is the correct answer.

|
Explore Courses for Mechanical Engineering exam
|

|
Question Description
A cantilever beam of uniform cross-section and length L is subjected to upward force W at mid-span and downward force P at the free end. For the deflection to be zero at the free end. W and P are related bya) W = 2Pb) W = 16P/5c) W = 3Pd) W = 5PCorrect answer is option 'B'. Can you explain this answer? for Mechanical Engineering 2025 is part of Mechanical Engineering preparation. The Question and answers have been prepared according to the Mechanical Engineering exam syllabus. Information about A cantilever beam of uniform cross-section and length L is subjected to upward force W at mid-span and downward force P at the free end. For the deflection to be zero at the free end. W and P are related bya) W = 2Pb) W = 16P/5c) W = 3Pd) W = 5PCorrect answer is option 'B'. Can you explain this answer? covers all topics & solutions for Mechanical Engineering 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A cantilever beam of uniform cross-section and length L is subjected to upward force W at mid-span and downward force P at the free end. For the deflection to be zero at the free end. W and P are related bya) W = 2Pb) W = 16P/5c) W = 3Pd) W = 5PCorrect answer is option 'B'. Can you explain this answer?.
A cantilever beam of uniform cross-section and length L is subjected to upward force W at mid-span and downward force P at the free end. For the deflection to be zero at the free end. W and P are related bya) W = 2Pb) W = 16P/5c) W = 3Pd) W = 5PCorrect answer is option 'B'. Can you explain this answer? for Mechanical Engineering 2025 is part of Mechanical Engineering preparation. The Question and answers have been prepared according to the Mechanical Engineering exam syllabus. Information about A cantilever beam of uniform cross-section and length L is subjected to upward force W at mid-span and downward force P at the free end. For the deflection to be zero at the free end. W and P are related bya) W = 2Pb) W = 16P/5c) W = 3Pd) W = 5PCorrect answer is option 'B'. Can you explain this answer? covers all topics & solutions for Mechanical Engineering 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A cantilever beam of uniform cross-section and length L is subjected to upward force W at mid-span and downward force P at the free end. For the deflection to be zero at the free end. W and P are related bya) W = 2Pb) W = 16P/5c) W = 3Pd) W = 5PCorrect answer is option 'B'. Can you explain this answer?.
Solutions for A cantilever beam of uniform cross-section and length L is subjected to upward force W at mid-span and downward force P at the free end. For the deflection to be zero at the free end. W and P are related bya) W = 2Pb) W = 16P/5c) W = 3Pd) W = 5PCorrect answer is option 'B'. Can you explain this answer? in English & in Hindi are available as part of our courses for Mechanical Engineering.
Download more important topics, notes, lectures and mock test series for Mechanical Engineering Exam by signing up for free.
Here you can find the meaning of A cantilever beam of uniform cross-section and length L is subjected to upward force W at mid-span and downward force P at the free end. For the deflection to be zero at the free end. W and P are related bya) W = 2Pb) W = 16P/5c) W = 3Pd) W = 5PCorrect answer is option 'B'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A cantilever beam of uniform cross-section and length L is subjected to upward force W at mid-span and downward force P at the free end. For the deflection to be zero at the free end. W and P are related bya) W = 2Pb) W = 16P/5c) W = 3Pd) W = 5PCorrect answer is option 'B'. Can you explain this answer?, a detailed solution for A cantilever beam of uniform cross-section and length L is subjected to upward force W at mid-span and downward force P at the free end. For the deflection to be zero at the free end. W and P are related bya) W = 2Pb) W = 16P/5c) W = 3Pd) W = 5PCorrect answer is option 'B'. Can you explain this answer? has been provided alongside types of A cantilever beam of uniform cross-section and length L is subjected to upward force W at mid-span and downward force P at the free end. For the deflection to be zero at the free end. W and P are related bya) W = 2Pb) W = 16P/5c) W = 3Pd) W = 5PCorrect answer is option 'B'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A cantilever beam of uniform cross-section and length L is subjected to upward force W at mid-span and downward force P at the free end. For the deflection to be zero at the free end. W and P are related bya) W = 2Pb) W = 16P/5c) W = 3Pd) W = 5PCorrect answer is option 'B'. Can you explain this answer? tests, examples and also practice Mechanical Engineering tests.

|
Explore Courses for Mechanical Engineering exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















