CAT Exam > CAT Questions > Directions for Questions: Six players –...
Start Learning for Free
Directions for Questions: Six players – Tanzi, Umeza, Wangdu, Xyla, Yonita and Zeneca competed in an archery tournament. The tournament had three compulsory rounds, Rounds 1 to 3. In each round every player shot an arrow at a target. Hitting the centre of the target (called bull’s eye) fetched the highest score of 5. The only other possible scores that a player could achieve were 4, 3, 2 and 1. Every bull’s eye score in the first three rounds gave a player one additional chance to shoot in the bonus rounds, Rounds 4 to 6. The possible scores in Rounds 4 to 6 were identical to the first three.
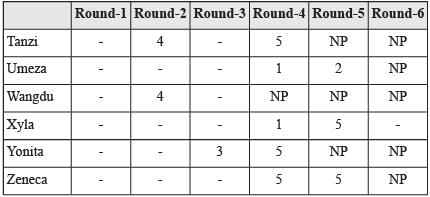
A player’s total score in the tournament was the sum of his/ her scores in all rounds played by him/her. The table below presents partial information on points scored by the players after completion of the tournament. In the table, NP means that the player did not participate in that round, while a hyphen means that the player participated in that round and the score information is missing.
The following facts are also known.
1. Tanzi, Umeza and Yonita had the same total score.
2. Total scores for all players, except one, were in multiples of three.
3. The highest total score was one more than double of the lowest total score.
4. The number of players hitting bull’s eye in Round 2 was double of that in Round 3.
5. Tanzi and Zeneca had the same score in Round 1 but different scores in Round 3.
A player’s total score in the tournament was the sum of his/ her scores in all rounds played by him/her. The table below presents partial information on points scored by the players after completion of the tournament. In the table, NP means that the player did not participate in that round, while a hyphen means that the player participated in that round and the score information is missing.

The following facts are also known.
1. Tanzi, Umeza and Yonita had the same total score.
2. Total scores for all players, except one, were in multiples of three.
3. The highest total score was one more than double of the lowest total score.
4. The number of players hitting bull’s eye in Round 2 was double of that in Round 3.
5. Tanzi and Zeneca had the same score in Round 1 but different scores in Round 3.
(2019)
Q. What was Tanzi’s score in Round 3?
- a)3
- b)5
- c)4
- d)1
Correct answer is option 'D'. Can you explain this answer?
Verified Answer
Directions for Questions: Six players – Tanzi, Umeza, Wangdu, Xy...
From given data, we get that total score of Tanzi = Umeza = Yonita Since Tanzi played another round, he/she must have hit bulls’eye in either Round 1 or Round 3. In the other round, let us say that Tanzi scored x. So Tanzi’s total score would be 14 + x.
Umeza played Round 4 and Round 5. This means Umeza have hit bull’s eye in two of the first three rounds. In the remaining round, let Umeza’s, score be y. Umeza’s total score would be 13 + y.
Yonita played round 4, he/she must have hit bull’s eye in round 1 or round 2. Let Yonita scored z in either of round 1 or 2, then, his/her total score = 5 + Z + 3 + 5 = 13 + Z.
Since the total score was not a multiple of 3 for only one person and Tanzi, Umeza and Yonita had the same total score 14 + x, 13 + y and 13 + z should be multiples of 3. 14 + x will be a multiple of 3 if x = 1 or 4. In that case the total score wil be 15 or 18. 13 + y will be multiple of 3 if y = 2 or 5. But if y = 5, then Umeza would had been played Round 6. Therefore, y = z = 2 and x = 1. The total score of Umeza. Tanzi and Yonita is 15.
Since Wangdu did not play any round after Round 3, the maximum score that Wangdu can get is 12 when he scores 4 in both round 1 and round 3.
Since Xyla played all the rounds, Xyla must have scored 5 in each of the first three rounds. So Xyla’s minimum total score is 22 and maximum total score is 26, based on Xyla scored (1 to 5) in round 6.
Zeneca played round 4 and round 5. So Zeneca must have hit bull’s eye and have scored 5 in two of the first three rounds.
So Zeneca’s minimum and maximum total scores are 21 and 24 respectively. Therefore, Wangdu had the lowest score.
If Wangdu scored 12, then the highest score would be 25. Only Xyla can score 25 (5 in the first three rounds and 4 in round 6).
If Wangdu scored 11, then the highest score would be 23. This is not possible because there will be two total scores that are not multiples of 3.
If Wangdu scored 10, then the highest score would be 21. But we know that Xyla’s minimum score is 22. Therefore, this is not possible.
Any score of Wangdu less than 10 would mean the highest score is less than 20 but we know that Xyla’s minimum score is 22. Therefore, Wangdu socred 12 and Xyla scored 25 is only possible value.
Xyla’s total score is 25, which is not a multiple of 3. Hence, Zeneca’s total score must be a multiple of 3, Zeneca would have scored 21 or 24.
Tanzi and Zeneca scored the same in round 1. Tanzi’s score in round 1 is either 1 or 5. If Tanzi socred 1 in round 1, then Zeneca would also have scored 1 in round 1. But in this case, both Zeneca and Tanzi would have scored 5 in round 3. But it is given that their scores in round 3 are different. Therfore, Tanze scored 5 in round 1 and 1 in round 3.
The number of players hitting bull’s eye in round 2 is either 2 or 4. If it is 2, then the toal number of 5 in round 2 and round 3 combined should be 3. Two of those 5s were scored by Xyla. Umeza and Zeneca would each have scored at least one 5 in roudns 2 and 3 combined but in this case, the number of 5s in round 2 and 3 combined would be at least 4, which is not possible. Therefore, the number of players hitting bull’s eye in round 2 are 4. Since Tanzi and Wangdu scored 4 in round 2, all the other players would have hit bull’s eye in round 2. This means that the number of players hitting bull’s eye in round 3 are 2. Xyla is one of them and the other one has to be either Umeza or Zeneca. But if Zeneca had scored 5 in Round 3, then Zeneca would have played round 6, which Zeneca didn’t. Therefore Umeza is the other person who scored 5 in round 3.
Since Umeza’s total score is 15, Umeza scored 2 in round 1.
Since Yonita’s total score is 15, Yonita scored 2 in round 1.
Zeneca’s total score cannot be 21 because in that case, both Zeneca and Tanzi would have scored the same in round 3, but they had different scores.
Therefore, Zeneca scored 4 in round 3.
After adding all, we get that table :
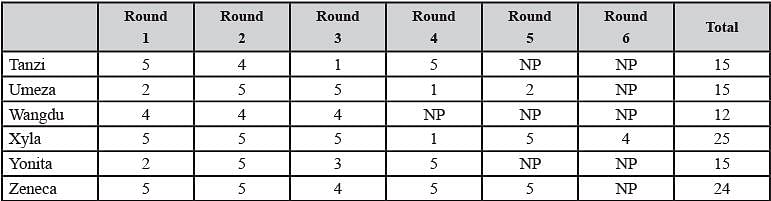
Tanzi’s score in Round 3 was 1.
Umeza played Round 4 and Round 5. This means Umeza have hit bull’s eye in two of the first three rounds. In the remaining round, let Umeza’s, score be y. Umeza’s total score would be 13 + y.
Yonita played round 4, he/she must have hit bull’s eye in round 1 or round 2. Let Yonita scored z in either of round 1 or 2, then, his/her total score = 5 + Z + 3 + 5 = 13 + Z.
Since the total score was not a multiple of 3 for only one person and Tanzi, Umeza and Yonita had the same total score 14 + x, 13 + y and 13 + z should be multiples of 3. 14 + x will be a multiple of 3 if x = 1 or 4. In that case the total score wil be 15 or 18. 13 + y will be multiple of 3 if y = 2 or 5. But if y = 5, then Umeza would had been played Round 6. Therefore, y = z = 2 and x = 1. The total score of Umeza. Tanzi and Yonita is 15.
Since Wangdu did not play any round after Round 3, the maximum score that Wangdu can get is 12 when he scores 4 in both round 1 and round 3.
Since Xyla played all the rounds, Xyla must have scored 5 in each of the first three rounds. So Xyla’s minimum total score is 22 and maximum total score is 26, based on Xyla scored (1 to 5) in round 6.
Zeneca played round 4 and round 5. So Zeneca must have hit bull’s eye and have scored 5 in two of the first three rounds.
So Zeneca’s minimum and maximum total scores are 21 and 24 respectively. Therefore, Wangdu had the lowest score.
If Wangdu scored 12, then the highest score would be 25. Only Xyla can score 25 (5 in the first three rounds and 4 in round 6).
If Wangdu scored 11, then the highest score would be 23. This is not possible because there will be two total scores that are not multiples of 3.
If Wangdu scored 10, then the highest score would be 21. But we know that Xyla’s minimum score is 22. Therefore, this is not possible.
Any score of Wangdu less than 10 would mean the highest score is less than 20 but we know that Xyla’s minimum score is 22. Therefore, Wangdu socred 12 and Xyla scored 25 is only possible value.
Xyla’s total score is 25, which is not a multiple of 3. Hence, Zeneca’s total score must be a multiple of 3, Zeneca would have scored 21 or 24.
Tanzi and Zeneca scored the same in round 1. Tanzi’s score in round 1 is either 1 or 5. If Tanzi socred 1 in round 1, then Zeneca would also have scored 1 in round 1. But in this case, both Zeneca and Tanzi would have scored 5 in round 3. But it is given that their scores in round 3 are different. Therfore, Tanze scored 5 in round 1 and 1 in round 3.
The number of players hitting bull’s eye in round 2 is either 2 or 4. If it is 2, then the toal number of 5 in round 2 and round 3 combined should be 3. Two of those 5s were scored by Xyla. Umeza and Zeneca would each have scored at least one 5 in roudns 2 and 3 combined but in this case, the number of 5s in round 2 and 3 combined would be at least 4, which is not possible. Therefore, the number of players hitting bull’s eye in round 2 are 4. Since Tanzi and Wangdu scored 4 in round 2, all the other players would have hit bull’s eye in round 2. This means that the number of players hitting bull’s eye in round 3 are 2. Xyla is one of them and the other one has to be either Umeza or Zeneca. But if Zeneca had scored 5 in Round 3, then Zeneca would have played round 6, which Zeneca didn’t. Therefore Umeza is the other person who scored 5 in round 3.
Since Umeza’s total score is 15, Umeza scored 2 in round 1.
Since Yonita’s total score is 15, Yonita scored 2 in round 1.
Zeneca’s total score cannot be 21 because in that case, both Zeneca and Tanzi would have scored the same in round 3, but they had different scores.
Therefore, Zeneca scored 4 in round 3.
After adding all, we get that table :

Tanzi’s score in Round 3 was 1.
Most Upvoted Answer
Directions for Questions: Six players – Tanzi, Umeza, Wangdu, Xy...
From given data, we get that total score of Tanzi = Umeza = Yonita Since Tanzi played another round, he/she must have hit bulls’eye in either Round 1 or Round 3. In the other round, let us say that Tanzi scored x. So Tanzi’s total score would be 14 + x.
Umeza played Round 4 and Round 5. This means Umeza have hit bull’s eye in two of the first three rounds. In the remaining round, let Umeza’s, score be y. Umeza’s total score would be 13 + y.
Yonita played round 4, he/she must have hit bull’s eye in round 1 or round 2. Let Yonita scored z in either of round 1 or 2, then, his/her total score = 5 + Z + 3 + 5 = 13 + Z.
Since the total score was not a multiple of 3 for only one person and Tanzi, Umeza and Yonita had the same total score 14 + x, 13 + y and 13 + z should be multiples of 3. 14 + x will be a multiple of 3 if x = 1 or 4. In that case the total score wil be 15 or 18. 13 + y will be multiple of 3 if y = 2 or 5. But if y = 5, then Umeza would had been played Round 6. Therefore, y = z = 2 and x = 1. The total score of Umeza. Tanzi and Yonita is 15.
Since Wangdu did not play any round after Round 3, the maximum score that Wangdu can get is 12 when he scores 4 in both round 1 and round 3.
Since Xyla played all the rounds, Xyla must have scored 5 in each of the first three rounds. So Xyla’s minimum total score is 22 and maximum total score is 26, based on Xyla scored (1 to 5) in round 6.
Zeneca played round 4 and round 5. So Zeneca must have hit bull’s eye and have scored 5 in two of the first three rounds.
So Zeneca’s minimum and maximum total scores are 21 and 24 respectively. Therefore, Wangdu had the lowest score.
If Wangdu scored 12, then the highest score would be 25. Only Xyla can score 25 (5 in the first three rounds and 4 in round 6).
If Wangdu scored 11, then the highest score would be 23. This is not possible because there will be two total scores that are not multiples of 3.
If Wangdu scored 10, then the highest score would be 21. But we know that Xyla’s minimum score is 22. Therefore, this is not possible.
Any score of Wangdu less than 10 would mean the highest score is less than 20 but we know that Xyla’s minimum score is 22. Therefore, Wangdu socred 12 and Xyla scored 25 is only possible value.
Xyla’s total score is 25, which is not a multiple of 3. Hence, Zeneca’s total score must be a multiple of 3, Zeneca would have scored 21 or 24.
Tanzi and Zeneca scored the same in round 1. Tanzi’s score in round 1 is either 1 or 5. If Tanzi socred 1 in round 1, then Zeneca would also have scored 1 in round 1. But in this case, both Zeneca and Tanzi would have scored 5 in round 3. But it is given that their scores in round 3 are different. Therfore, Tanze scored 5 in round 1 and 1 in round 3.
The number of players hitting bull’s eye in round 2 is either 2 or 4. If it is 2, then the toal number of 5 in round 2 and round 3 combined should be 3. Two of those 5s were scored by Xyla. Umeza and Zeneca would each have scored at least one 5 in roudns 2 and 3 combined but in this case, the number of 5s in round 2 and 3 combined would be at least 4, which is not possible. Therefore, the number of players hitting bull’s eye in round 2 are 4. Since Tanzi and Wangdu scored 4 in round 2, all the other players would have hit bull’s eye in round 2. This means that the number of players hitting bull’s eye in round 3 are 2. Xyla is one of them and the other one has to be either Umeza or Zeneca. But if Zeneca had scored 5 in Round 3, then Zeneca would have played round 6, which Zeneca didn’t. Therefore Umeza is the other person who scored 5 in round 3.
Since Umeza’s total score is 15, Umeza scored 2 in round 1.
Since Yonita’s total score is 15, Yonita scored 2 in round 1.
Zeneca’s total score cannot be 21 because in that case, both Zeneca and Tanzi would have scored the same in round 3, but they had different scores.
Therefore, Zeneca scored 4 in round 3.
After adding all, we get that table :
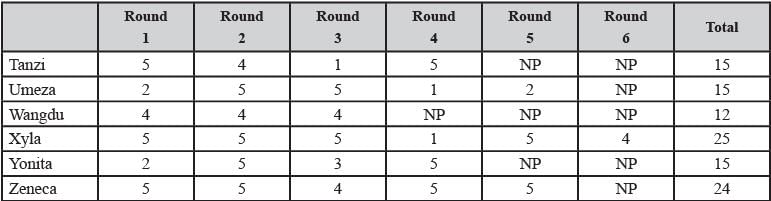
Tanzi’s score in Round 3 was 1.
Umeza played Round 4 and Round 5. This means Umeza have hit bull’s eye in two of the first three rounds. In the remaining round, let Umeza’s, score be y. Umeza’s total score would be 13 + y.
Yonita played round 4, he/she must have hit bull’s eye in round 1 or round 2. Let Yonita scored z in either of round 1 or 2, then, his/her total score = 5 + Z + 3 + 5 = 13 + Z.
Since the total score was not a multiple of 3 for only one person and Tanzi, Umeza and Yonita had the same total score 14 + x, 13 + y and 13 + z should be multiples of 3. 14 + x will be a multiple of 3 if x = 1 or 4. In that case the total score wil be 15 or 18. 13 + y will be multiple of 3 if y = 2 or 5. But if y = 5, then Umeza would had been played Round 6. Therefore, y = z = 2 and x = 1. The total score of Umeza. Tanzi and Yonita is 15.
Since Wangdu did not play any round after Round 3, the maximum score that Wangdu can get is 12 when he scores 4 in both round 1 and round 3.
Since Xyla played all the rounds, Xyla must have scored 5 in each of the first three rounds. So Xyla’s minimum total score is 22 and maximum total score is 26, based on Xyla scored (1 to 5) in round 6.
Zeneca played round 4 and round 5. So Zeneca must have hit bull’s eye and have scored 5 in two of the first three rounds.
So Zeneca’s minimum and maximum total scores are 21 and 24 respectively. Therefore, Wangdu had the lowest score.
If Wangdu scored 12, then the highest score would be 25. Only Xyla can score 25 (5 in the first three rounds and 4 in round 6).
If Wangdu scored 11, then the highest score would be 23. This is not possible because there will be two total scores that are not multiples of 3.
If Wangdu scored 10, then the highest score would be 21. But we know that Xyla’s minimum score is 22. Therefore, this is not possible.
Any score of Wangdu less than 10 would mean the highest score is less than 20 but we know that Xyla’s minimum score is 22. Therefore, Wangdu socred 12 and Xyla scored 25 is only possible value.
Xyla’s total score is 25, which is not a multiple of 3. Hence, Zeneca’s total score must be a multiple of 3, Zeneca would have scored 21 or 24.
Tanzi and Zeneca scored the same in round 1. Tanzi’s score in round 1 is either 1 or 5. If Tanzi socred 1 in round 1, then Zeneca would also have scored 1 in round 1. But in this case, both Zeneca and Tanzi would have scored 5 in round 3. But it is given that their scores in round 3 are different. Therfore, Tanze scored 5 in round 1 and 1 in round 3.
The number of players hitting bull’s eye in round 2 is either 2 or 4. If it is 2, then the toal number of 5 in round 2 and round 3 combined should be 3. Two of those 5s were scored by Xyla. Umeza and Zeneca would each have scored at least one 5 in roudns 2 and 3 combined but in this case, the number of 5s in round 2 and 3 combined would be at least 4, which is not possible. Therefore, the number of players hitting bull’s eye in round 2 are 4. Since Tanzi and Wangdu scored 4 in round 2, all the other players would have hit bull’s eye in round 2. This means that the number of players hitting bull’s eye in round 3 are 2. Xyla is one of them and the other one has to be either Umeza or Zeneca. But if Zeneca had scored 5 in Round 3, then Zeneca would have played round 6, which Zeneca didn’t. Therefore Umeza is the other person who scored 5 in round 3.
Since Umeza’s total score is 15, Umeza scored 2 in round 1.
Since Yonita’s total score is 15, Yonita scored 2 in round 1.
Zeneca’s total score cannot be 21 because in that case, both Zeneca and Tanzi would have scored the same in round 3, but they had different scores.
Therefore, Zeneca scored 4 in round 3.
After adding all, we get that table :

Tanzi’s score in Round 3 was 1.
Free Test
FREE
| Start Free Test |
Community Answer
Directions for Questions: Six players – Tanzi, Umeza, Wangdu, Xy...
From given data, we get that total score of Tanzi = Umeza = Yonita Since Tanzi played another round, he/she must have hit bulls’eye in either Round 1 or Round 3. In the other round, let us say that Tanzi scored x. So Tanzi’s total score would be 14 + x.
Umeza played Round 4 and Round 5. This means Umeza have hit bull’s eye in two of the first three rounds. In the remaining round, let Umeza’s, score be y. Umeza’s total score would be 13 + y.
Yonita played round 4, he/she must have hit bull’s eye in round 1 or round 2. Let Yonita scored z in either of round 1 or 2, then, his/her total score = 5 + Z + 3 + 5 = 13 + Z.
Since the total score was not a multiple of 3 for only one person and Tanzi, Umeza and Yonita had the same total score 14 + x, 13 + y and 13 + z should be multiples of 3. 14 + x will be a multiple of 3 if x = 1 or 4. In that case the total score wil be 15 or 18. 13 + y will be multiple of 3 if y = 2 or 5. But if y = 5, then Umeza would had been played Round 6. Therefore, y = z = 2 and x = 1. The total score of Umeza. Tanzi and Yonita is 15.
Since Wangdu did not play any round after Round 3, the maximum score that Wangdu can get is 12 when he scores 4 in both round 1 and round 3.
Since Xyla played all the rounds, Xyla must have scored 5 in each of the first three rounds. So Xyla’s minimum total score is 22 and maximum total score is 26, based on Xyla scored (1 to 5) in round 6.
Zeneca played round 4 and round 5. So Zeneca must have hit bull’s eye and have scored 5 in two of the first three rounds.
So Zeneca’s minimum and maximum total scores are 21 and 24 respectively. Therefore, Wangdu had the lowest score.
If Wangdu scored 12, then the highest score would be 25. Only Xyla can score 25 (5 in the first three rounds and 4 in round 6).
If Wangdu scored 11, then the highest score would be 23. This is not possible because there will be two total scores that are not multiples of 3.
If Wangdu scored 10, then the highest score would be 21. But we know that Xyla’s minimum score is 22. Therefore, this is not possible.
Any score of Wangdu less than 10 would mean the highest score is less than 20 but we know that Xyla’s minimum score is 22. Therefore, Wangdu socred 12 and Xyla scored 25 is only possible value.
Xyla’s total score is 25, which is not a multiple of 3. Hence, Zeneca’s total score must be a multiple of 3, Zeneca would have scored 21 or 24.
Tanzi and Zeneca scored the same in round 1. Tanzi’s score in round 1 is either 1 or 5. If Tanzi socred 1 in round 1, then Zeneca would also have scored 1 in round 1. But in this case, both Zeneca and Tanzi would have scored 5 in round 3. But it is given that their scores in round 3 are different. Therfore, Tanze scored 5 in round 1 and 1 in round 3.
The number of players hitting bull’s eye in round 2 is either 2 or 4. If it is 2, then the toal number of 5 in round 2 and round 3 combined should be 3. Two of those 5s were scored by Xyla. Umeza and Zeneca would each have scored at least one 5 in roudns 2 and 3 combined but in this case, the number of 5s in round 2 and 3 combined would be at least 4, which is not possible. Therefore, the number of players hitting bull’s eye in round 2 are 4. Since Tanzi and Wangdu scored 4 in round 2, all the other players would have hit bull’s eye in round 2. This means that the number of players hitting bull’s eye in round 3 are 2. Xyla is one of them and the other one has to be either Umeza or Zeneca. But if Zeneca had scored 5 in Round 3, then Zeneca would have played round 6, which Zeneca didn’t. Therefore Umeza is the other person who scored 5 in round 3.
Since Umeza’s total score is 15, Umeza scored 2 in round 1.
Since Yonita’s total score is 15, Yonita scored 2 in round 1.
Zeneca’s total score cannot be 21 because in that case, both Zeneca and Tanzi would have scored the same in round 3, but they had different scores.
Therefore, Zeneca scored 4 in round 3.
After adding all, we get that table :

Tanzi’s score in Round 3 was 1.
Umeza played Round 4 and Round 5. This means Umeza have hit bull’s eye in two of the first three rounds. In the remaining round, let Umeza’s, score be y. Umeza’s total score would be 13 + y.
Yonita played round 4, he/she must have hit bull’s eye in round 1 or round 2. Let Yonita scored z in either of round 1 or 2, then, his/her total score = 5 + Z + 3 + 5 = 13 + Z.
Since the total score was not a multiple of 3 for only one person and Tanzi, Umeza and Yonita had the same total score 14 + x, 13 + y and 13 + z should be multiples of 3. 14 + x will be a multiple of 3 if x = 1 or 4. In that case the total score wil be 15 or 18. 13 + y will be multiple of 3 if y = 2 or 5. But if y = 5, then Umeza would had been played Round 6. Therefore, y = z = 2 and x = 1. The total score of Umeza. Tanzi and Yonita is 15.
Since Wangdu did not play any round after Round 3, the maximum score that Wangdu can get is 12 when he scores 4 in both round 1 and round 3.
Since Xyla played all the rounds, Xyla must have scored 5 in each of the first three rounds. So Xyla’s minimum total score is 22 and maximum total score is 26, based on Xyla scored (1 to 5) in round 6.
Zeneca played round 4 and round 5. So Zeneca must have hit bull’s eye and have scored 5 in two of the first three rounds.
So Zeneca’s minimum and maximum total scores are 21 and 24 respectively. Therefore, Wangdu had the lowest score.
If Wangdu scored 12, then the highest score would be 25. Only Xyla can score 25 (5 in the first three rounds and 4 in round 6).
If Wangdu scored 11, then the highest score would be 23. This is not possible because there will be two total scores that are not multiples of 3.
If Wangdu scored 10, then the highest score would be 21. But we know that Xyla’s minimum score is 22. Therefore, this is not possible.
Any score of Wangdu less than 10 would mean the highest score is less than 20 but we know that Xyla’s minimum score is 22. Therefore, Wangdu socred 12 and Xyla scored 25 is only possible value.
Xyla’s total score is 25, which is not a multiple of 3. Hence, Zeneca’s total score must be a multiple of 3, Zeneca would have scored 21 or 24.
Tanzi and Zeneca scored the same in round 1. Tanzi’s score in round 1 is either 1 or 5. If Tanzi socred 1 in round 1, then Zeneca would also have scored 1 in round 1. But in this case, both Zeneca and Tanzi would have scored 5 in round 3. But it is given that their scores in round 3 are different. Therfore, Tanze scored 5 in round 1 and 1 in round 3.
The number of players hitting bull’s eye in round 2 is either 2 or 4. If it is 2, then the toal number of 5 in round 2 and round 3 combined should be 3. Two of those 5s were scored by Xyla. Umeza and Zeneca would each have scored at least one 5 in roudns 2 and 3 combined but in this case, the number of 5s in round 2 and 3 combined would be at least 4, which is not possible. Therefore, the number of players hitting bull’s eye in round 2 are 4. Since Tanzi and Wangdu scored 4 in round 2, all the other players would have hit bull’s eye in round 2. This means that the number of players hitting bull’s eye in round 3 are 2. Xyla is one of them and the other one has to be either Umeza or Zeneca. But if Zeneca had scored 5 in Round 3, then Zeneca would have played round 6, which Zeneca didn’t. Therefore Umeza is the other person who scored 5 in round 3.
Since Umeza’s total score is 15, Umeza scored 2 in round 1.
Since Yonita’s total score is 15, Yonita scored 2 in round 1.
Zeneca’s total score cannot be 21 because in that case, both Zeneca and Tanzi would have scored the same in round 3, but they had different scores.
Therefore, Zeneca scored 4 in round 3.
After adding all, we get that table :

Tanzi’s score in Round 3 was 1.

|
Explore Courses for CAT exam
|

|
Similar CAT Doubts
Directions for Questions: Six players – Tanzi, Umeza, Wangdu, Xyla, Yonita and Zeneca competed in an archery tournament. The tournament had three compulsory rounds, Rounds 1 to 3. In each round every player shot an arrow at a target. Hitting the centre of the target (called bull’s eye) fetched the highest score of 5. The only other possible scores that a player could achieve were 4, 3, 2 and 1. Every bull’s eye score in the first three rounds gave a player one additional chance to shoot in the bonus rounds, Rounds 4 to 6. The possible scores in Rounds 4 to 6 were identical to the first three.A player’s total score in the tournament was the sum of his/ her scores in all rounds played by him/her. The table below presents partial information on points scored by the players aftercompletion of the tournament. In the table, NP means that the player did not participate in that round, while a hyphen means that the player participated in that round and the score information is missing.The following facts are also known.1. Tanzi, Umeza and Yonita had the same total score.2. Total scores for all players, except one, were in multiples of three.3. The highest total score was one more than double of the lowest total score.4. The number of players hitting bull’s eye in Round 2 was double of that in Round 3.5. Tanzi and Zeneca had the same score in Round 1 but different scores in Round 3.(2019)Q. What was Tanzi’s score in Round 3?a)3b)5c)4d)1Correct answer is option 'D'. Can you explain this answer?
Question Description
Directions for Questions: Six players – Tanzi, Umeza, Wangdu, Xyla, Yonita and Zeneca competed in an archery tournament. The tournament had three compulsory rounds, Rounds 1 to 3. In each round every player shot an arrow at a target. Hitting the centre of the target (called bull’s eye) fetched the highest score of 5. The only other possible scores that a player could achieve were 4, 3, 2 and 1. Every bull’s eye score in the first three rounds gave a player one additional chance to shoot in the bonus rounds, Rounds 4 to 6. The possible scores in Rounds 4 to 6 were identical to the first three.A player’s total score in the tournament was the sum of his/ her scores in all rounds played by him/her. The table below presents partial information on points scored by the players aftercompletion of the tournament. In the table, NP means that the player did not participate in that round, while a hyphen means that the player participated in that round and the score information is missing.The following facts are also known.1. Tanzi, Umeza and Yonita had the same total score.2. Total scores for all players, except one, were in multiples of three.3. The highest total score was one more than double of the lowest total score.4. The number of players hitting bull’s eye in Round 2 was double of that in Round 3.5. Tanzi and Zeneca had the same score in Round 1 but different scores in Round 3.(2019)Q. What was Tanzi’s score in Round 3?a)3b)5c)4d)1Correct answer is option 'D'. Can you explain this answer? for CAT 2025 is part of CAT preparation. The Question and answers have been prepared according to the CAT exam syllabus. Information about Directions for Questions: Six players – Tanzi, Umeza, Wangdu, Xyla, Yonita and Zeneca competed in an archery tournament. The tournament had three compulsory rounds, Rounds 1 to 3. In each round every player shot an arrow at a target. Hitting the centre of the target (called bull’s eye) fetched the highest score of 5. The only other possible scores that a player could achieve were 4, 3, 2 and 1. Every bull’s eye score in the first three rounds gave a player one additional chance to shoot in the bonus rounds, Rounds 4 to 6. The possible scores in Rounds 4 to 6 were identical to the first three.A player’s total score in the tournament was the sum of his/ her scores in all rounds played by him/her. The table below presents partial information on points scored by the players aftercompletion of the tournament. In the table, NP means that the player did not participate in that round, while a hyphen means that the player participated in that round and the score information is missing.The following facts are also known.1. Tanzi, Umeza and Yonita had the same total score.2. Total scores for all players, except one, were in multiples of three.3. The highest total score was one more than double of the lowest total score.4. The number of players hitting bull’s eye in Round 2 was double of that in Round 3.5. Tanzi and Zeneca had the same score in Round 1 but different scores in Round 3.(2019)Q. What was Tanzi’s score in Round 3?a)3b)5c)4d)1Correct answer is option 'D'. Can you explain this answer? covers all topics & solutions for CAT 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Directions for Questions: Six players – Tanzi, Umeza, Wangdu, Xyla, Yonita and Zeneca competed in an archery tournament. The tournament had three compulsory rounds, Rounds 1 to 3. In each round every player shot an arrow at a target. Hitting the centre of the target (called bull’s eye) fetched the highest score of 5. The only other possible scores that a player could achieve were 4, 3, 2 and 1. Every bull’s eye score in the first three rounds gave a player one additional chance to shoot in the bonus rounds, Rounds 4 to 6. The possible scores in Rounds 4 to 6 were identical to the first three.A player’s total score in the tournament was the sum of his/ her scores in all rounds played by him/her. The table below presents partial information on points scored by the players aftercompletion of the tournament. In the table, NP means that the player did not participate in that round, while a hyphen means that the player participated in that round and the score information is missing.The following facts are also known.1. Tanzi, Umeza and Yonita had the same total score.2. Total scores for all players, except one, were in multiples of three.3. The highest total score was one more than double of the lowest total score.4. The number of players hitting bull’s eye in Round 2 was double of that in Round 3.5. Tanzi and Zeneca had the same score in Round 1 but different scores in Round 3.(2019)Q. What was Tanzi’s score in Round 3?a)3b)5c)4d)1Correct answer is option 'D'. Can you explain this answer?.
Directions for Questions: Six players – Tanzi, Umeza, Wangdu, Xyla, Yonita and Zeneca competed in an archery tournament. The tournament had three compulsory rounds, Rounds 1 to 3. In each round every player shot an arrow at a target. Hitting the centre of the target (called bull’s eye) fetched the highest score of 5. The only other possible scores that a player could achieve were 4, 3, 2 and 1. Every bull’s eye score in the first three rounds gave a player one additional chance to shoot in the bonus rounds, Rounds 4 to 6. The possible scores in Rounds 4 to 6 were identical to the first three.A player’s total score in the tournament was the sum of his/ her scores in all rounds played by him/her. The table below presents partial information on points scored by the players aftercompletion of the tournament. In the table, NP means that the player did not participate in that round, while a hyphen means that the player participated in that round and the score information is missing.The following facts are also known.1. Tanzi, Umeza and Yonita had the same total score.2. Total scores for all players, except one, were in multiples of three.3. The highest total score was one more than double of the lowest total score.4. The number of players hitting bull’s eye in Round 2 was double of that in Round 3.5. Tanzi and Zeneca had the same score in Round 1 but different scores in Round 3.(2019)Q. What was Tanzi’s score in Round 3?a)3b)5c)4d)1Correct answer is option 'D'. Can you explain this answer? for CAT 2025 is part of CAT preparation. The Question and answers have been prepared according to the CAT exam syllabus. Information about Directions for Questions: Six players – Tanzi, Umeza, Wangdu, Xyla, Yonita and Zeneca competed in an archery tournament. The tournament had three compulsory rounds, Rounds 1 to 3. In each round every player shot an arrow at a target. Hitting the centre of the target (called bull’s eye) fetched the highest score of 5. The only other possible scores that a player could achieve were 4, 3, 2 and 1. Every bull’s eye score in the first three rounds gave a player one additional chance to shoot in the bonus rounds, Rounds 4 to 6. The possible scores in Rounds 4 to 6 were identical to the first three.A player’s total score in the tournament was the sum of his/ her scores in all rounds played by him/her. The table below presents partial information on points scored by the players aftercompletion of the tournament. In the table, NP means that the player did not participate in that round, while a hyphen means that the player participated in that round and the score information is missing.The following facts are also known.1. Tanzi, Umeza and Yonita had the same total score.2. Total scores for all players, except one, were in multiples of three.3. The highest total score was one more than double of the lowest total score.4. The number of players hitting bull’s eye in Round 2 was double of that in Round 3.5. Tanzi and Zeneca had the same score in Round 1 but different scores in Round 3.(2019)Q. What was Tanzi’s score in Round 3?a)3b)5c)4d)1Correct answer is option 'D'. Can you explain this answer? covers all topics & solutions for CAT 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Directions for Questions: Six players – Tanzi, Umeza, Wangdu, Xyla, Yonita and Zeneca competed in an archery tournament. The tournament had three compulsory rounds, Rounds 1 to 3. In each round every player shot an arrow at a target. Hitting the centre of the target (called bull’s eye) fetched the highest score of 5. The only other possible scores that a player could achieve were 4, 3, 2 and 1. Every bull’s eye score in the first three rounds gave a player one additional chance to shoot in the bonus rounds, Rounds 4 to 6. The possible scores in Rounds 4 to 6 were identical to the first three.A player’s total score in the tournament was the sum of his/ her scores in all rounds played by him/her. The table below presents partial information on points scored by the players aftercompletion of the tournament. In the table, NP means that the player did not participate in that round, while a hyphen means that the player participated in that round and the score information is missing.The following facts are also known.1. Tanzi, Umeza and Yonita had the same total score.2. Total scores for all players, except one, were in multiples of three.3. The highest total score was one more than double of the lowest total score.4. The number of players hitting bull’s eye in Round 2 was double of that in Round 3.5. Tanzi and Zeneca had the same score in Round 1 but different scores in Round 3.(2019)Q. What was Tanzi’s score in Round 3?a)3b)5c)4d)1Correct answer is option 'D'. Can you explain this answer?.
Solutions for Directions for Questions: Six players – Tanzi, Umeza, Wangdu, Xyla, Yonita and Zeneca competed in an archery tournament. The tournament had three compulsory rounds, Rounds 1 to 3. In each round every player shot an arrow at a target. Hitting the centre of the target (called bull’s eye) fetched the highest score of 5. The only other possible scores that a player could achieve were 4, 3, 2 and 1. Every bull’s eye score in the first three rounds gave a player one additional chance to shoot in the bonus rounds, Rounds 4 to 6. The possible scores in Rounds 4 to 6 were identical to the first three.A player’s total score in the tournament was the sum of his/ her scores in all rounds played by him/her. The table below presents partial information on points scored by the players aftercompletion of the tournament. In the table, NP means that the player did not participate in that round, while a hyphen means that the player participated in that round and the score information is missing.The following facts are also known.1. Tanzi, Umeza and Yonita had the same total score.2. Total scores for all players, except one, were in multiples of three.3. The highest total score was one more than double of the lowest total score.4. The number of players hitting bull’s eye in Round 2 was double of that in Round 3.5. Tanzi and Zeneca had the same score in Round 1 but different scores in Round 3.(2019)Q. What was Tanzi’s score in Round 3?a)3b)5c)4d)1Correct answer is option 'D'. Can you explain this answer? in English & in Hindi are available as part of our courses for CAT.
Download more important topics, notes, lectures and mock test series for CAT Exam by signing up for free.
Here you can find the meaning of Directions for Questions: Six players – Tanzi, Umeza, Wangdu, Xyla, Yonita and Zeneca competed in an archery tournament. The tournament had three compulsory rounds, Rounds 1 to 3. In each round every player shot an arrow at a target. Hitting the centre of the target (called bull’s eye) fetched the highest score of 5. The only other possible scores that a player could achieve were 4, 3, 2 and 1. Every bull’s eye score in the first three rounds gave a player one additional chance to shoot in the bonus rounds, Rounds 4 to 6. The possible scores in Rounds 4 to 6 were identical to the first three.A player’s total score in the tournament was the sum of his/ her scores in all rounds played by him/her. The table below presents partial information on points scored by the players aftercompletion of the tournament. In the table, NP means that the player did not participate in that round, while a hyphen means that the player participated in that round and the score information is missing.The following facts are also known.1. Tanzi, Umeza and Yonita had the same total score.2. Total scores for all players, except one, were in multiples of three.3. The highest total score was one more than double of the lowest total score.4. The number of players hitting bull’s eye in Round 2 was double of that in Round 3.5. Tanzi and Zeneca had the same score in Round 1 but different scores in Round 3.(2019)Q. What was Tanzi’s score in Round 3?a)3b)5c)4d)1Correct answer is option 'D'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
Directions for Questions: Six players – Tanzi, Umeza, Wangdu, Xyla, Yonita and Zeneca competed in an archery tournament. The tournament had three compulsory rounds, Rounds 1 to 3. In each round every player shot an arrow at a target. Hitting the centre of the target (called bull’s eye) fetched the highest score of 5. The only other possible scores that a player could achieve were 4, 3, 2 and 1. Every bull’s eye score in the first three rounds gave a player one additional chance to shoot in the bonus rounds, Rounds 4 to 6. The possible scores in Rounds 4 to 6 were identical to the first three.A player’s total score in the tournament was the sum of his/ her scores in all rounds played by him/her. The table below presents partial information on points scored by the players aftercompletion of the tournament. In the table, NP means that the player did not participate in that round, while a hyphen means that the player participated in that round and the score information is missing.The following facts are also known.1. Tanzi, Umeza and Yonita had the same total score.2. Total scores for all players, except one, were in multiples of three.3. The highest total score was one more than double of the lowest total score.4. The number of players hitting bull’s eye in Round 2 was double of that in Round 3.5. Tanzi and Zeneca had the same score in Round 1 but different scores in Round 3.(2019)Q. What was Tanzi’s score in Round 3?a)3b)5c)4d)1Correct answer is option 'D'. Can you explain this answer?, a detailed solution for Directions for Questions: Six players – Tanzi, Umeza, Wangdu, Xyla, Yonita and Zeneca competed in an archery tournament. The tournament had three compulsory rounds, Rounds 1 to 3. In each round every player shot an arrow at a target. Hitting the centre of the target (called bull’s eye) fetched the highest score of 5. The only other possible scores that a player could achieve were 4, 3, 2 and 1. Every bull’s eye score in the first three rounds gave a player one additional chance to shoot in the bonus rounds, Rounds 4 to 6. The possible scores in Rounds 4 to 6 were identical to the first three.A player’s total score in the tournament was the sum of his/ her scores in all rounds played by him/her. The table below presents partial information on points scored by the players aftercompletion of the tournament. In the table, NP means that the player did not participate in that round, while a hyphen means that the player participated in that round and the score information is missing.The following facts are also known.1. Tanzi, Umeza and Yonita had the same total score.2. Total scores for all players, except one, were in multiples of three.3. The highest total score was one more than double of the lowest total score.4. The number of players hitting bull’s eye in Round 2 was double of that in Round 3.5. Tanzi and Zeneca had the same score in Round 1 but different scores in Round 3.(2019)Q. What was Tanzi’s score in Round 3?a)3b)5c)4d)1Correct answer is option 'D'. Can you explain this answer? has been provided alongside types of Directions for Questions: Six players – Tanzi, Umeza, Wangdu, Xyla, Yonita and Zeneca competed in an archery tournament. The tournament had three compulsory rounds, Rounds 1 to 3. In each round every player shot an arrow at a target. Hitting the centre of the target (called bull’s eye) fetched the highest score of 5. The only other possible scores that a player could achieve were 4, 3, 2 and 1. Every bull’s eye score in the first three rounds gave a player one additional chance to shoot in the bonus rounds, Rounds 4 to 6. The possible scores in Rounds 4 to 6 were identical to the first three.A player’s total score in the tournament was the sum of his/ her scores in all rounds played by him/her. The table below presents partial information on points scored by the players aftercompletion of the tournament. In the table, NP means that the player did not participate in that round, while a hyphen means that the player participated in that round and the score information is missing.The following facts are also known.1. Tanzi, Umeza and Yonita had the same total score.2. Total scores for all players, except one, were in multiples of three.3. The highest total score was one more than double of the lowest total score.4. The number of players hitting bull’s eye in Round 2 was double of that in Round 3.5. Tanzi and Zeneca had the same score in Round 1 but different scores in Round 3.(2019)Q. What was Tanzi’s score in Round 3?a)3b)5c)4d)1Correct answer is option 'D'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice Directions for Questions: Six players – Tanzi, Umeza, Wangdu, Xyla, Yonita and Zeneca competed in an archery tournament. The tournament had three compulsory rounds, Rounds 1 to 3. In each round every player shot an arrow at a target. Hitting the centre of the target (called bull’s eye) fetched the highest score of 5. The only other possible scores that a player could achieve were 4, 3, 2 and 1. Every bull’s eye score in the first three rounds gave a player one additional chance to shoot in the bonus rounds, Rounds 4 to 6. The possible scores in Rounds 4 to 6 were identical to the first three.A player’s total score in the tournament was the sum of his/ her scores in all rounds played by him/her. The table below presents partial information on points scored by the players aftercompletion of the tournament. In the table, NP means that the player did not participate in that round, while a hyphen means that the player participated in that round and the score information is missing.The following facts are also known.1. Tanzi, Umeza and Yonita had the same total score.2. Total scores for all players, except one, were in multiples of three.3. The highest total score was one more than double of the lowest total score.4. The number of players hitting bull’s eye in Round 2 was double of that in Round 3.5. Tanzi and Zeneca had the same score in Round 1 but different scores in Round 3.(2019)Q. What was Tanzi’s score in Round 3?a)3b)5c)4d)1Correct answer is option 'D'. Can you explain this answer? tests, examples and also practice CAT tests.

|
Explore Courses for CAT exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


























