Civil Engineering (CE) Exam > Civil Engineering (CE) Questions > A simply supported beam ABC of length L carri...
Start Learning for Free
A simply supported beam ABC of length L carries a concentrated load P at an intermediate point B . If slope at A is 0.75 times the slope at C, then length of portion AB is equal to ?
Most Upvoted Answer
A simply supported beam ABC of length L carries a concentrated load P ...
Solution:
Given,
Length of beam = L
Concentrated load at point B = P
Slope at point A = 0.75 times the slope at point C
To find,
Length of portion AB
Assumptions:
Beam is homogeneous, isotropic and prismatic.
Load is applied at the mid-span of the beam.
The beam is subjected to pure bending.
The beam is loaded within its elastic limit.
The beam is simply supported.
Analysis:
The bending moment diagram of the simply supported beam with a concentrated load at point B is shown below:
From the diagram, we can see that the bending moment at point A is zero and at point C is P(L/2). Therefore, the slope at point C can be calculated as:
θC = MC / EI
where,
MC = maximum bending moment at point C = P(L/2)
E = modulus of elasticity of beam material
I = moment of inertia of the beam
The slope at point A can be calculated as:
θA = 0.75 × θC
Therefore, the bending moment at point B can be calculated as:
MB = P(L/4)
The slope at point B can be calculated as:
θB = MB / EI
Now, we can apply the moment-area method to find the length of portion AB.
Moment-Area Method:
The moment-area method is based on the principle that the area of the moment diagram between any two points is equal to the change in slope of the beam between those two points.
Using this principle, we can calculate the change in slope of the beam between points A and B as follows:
Change in slope between A and B = area of moment diagram between A and B / EI
From the bending moment diagram, we can see that the moment at point B is P(L/4) and the moment at point A is zero. Therefore, the area of the moment diagram between points A and B can be calculated as:
Area of moment diagram between A and B = (P L / 4) × (L / 4)
= PL2 / 16
Therefore, the change in slope between points A and B can be calculated as:
Change in slope between A and B = (PL2 / 16) / EI
Similarly, we can calculate the change in slope between points B and C as follows:
Change in slope between B and C = (PL2 / 16) / EI
Since the total change in slope between points A and C is equal to θC - θA, we can write:
θC - θA = Change in slope between A and B + Change in slope between B and C
Substituting the values of θC, θA, and the changes in slope, we get:
P(L2 / 16EI) = 2 × (PL2 / 16EI)
Simplifying this equation, we get:
L / 2 = AB
Therefore, the length of portion AB is equal to half the length of the beam, i.e., L/2.
Conclusion:
The length of portion AB in a simply supported beam carrying a concentrated load at an intermediate point B and with the slope at point A being 0.75 times the slope at point C is equal to half the length of the beam, i.e., L/2.
Given,
Length of beam = L
Concentrated load at point B = P
Slope at point A = 0.75 times the slope at point C
To find,
Length of portion AB
Assumptions:
Beam is homogeneous, isotropic and prismatic.
Load is applied at the mid-span of the beam.
The beam is subjected to pure bending.
The beam is loaded within its elastic limit.
The beam is simply supported.
Analysis:
The bending moment diagram of the simply supported beam with a concentrated load at point B is shown below:
From the diagram, we can see that the bending moment at point A is zero and at point C is P(L/2). Therefore, the slope at point C can be calculated as:
θC = MC / EI
where,
MC = maximum bending moment at point C = P(L/2)
E = modulus of elasticity of beam material
I = moment of inertia of the beam
The slope at point A can be calculated as:
θA = 0.75 × θC
Therefore, the bending moment at point B can be calculated as:
MB = P(L/4)
The slope at point B can be calculated as:
θB = MB / EI
Now, we can apply the moment-area method to find the length of portion AB.
Moment-Area Method:
The moment-area method is based on the principle that the area of the moment diagram between any two points is equal to the change in slope of the beam between those two points.
Using this principle, we can calculate the change in slope of the beam between points A and B as follows:
Change in slope between A and B = area of moment diagram between A and B / EI
From the bending moment diagram, we can see that the moment at point B is P(L/4) and the moment at point A is zero. Therefore, the area of the moment diagram between points A and B can be calculated as:
Area of moment diagram between A and B = (P L / 4) × (L / 4)
= PL2 / 16
Therefore, the change in slope between points A and B can be calculated as:
Change in slope between A and B = (PL2 / 16) / EI
Similarly, we can calculate the change in slope between points B and C as follows:
Change in slope between B and C = (PL2 / 16) / EI
Since the total change in slope between points A and C is equal to θC - θA, we can write:
θC - θA = Change in slope between A and B + Change in slope between B and C
Substituting the values of θC, θA, and the changes in slope, we get:
P(L2 / 16EI) = 2 × (PL2 / 16EI)
Simplifying this equation, we get:
L / 2 = AB
Therefore, the length of portion AB is equal to half the length of the beam, i.e., L/2.
Conclusion:
The length of portion AB in a simply supported beam carrying a concentrated load at an intermediate point B and with the slope at point A being 0.75 times the slope at point C is equal to half the length of the beam, i.e., L/2.
Community Answer
A simply supported beam ABC of length L carries a concentrated load P ...
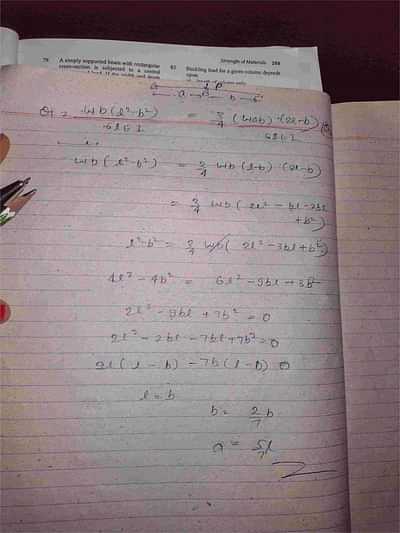

|
Explore Courses for Civil Engineering (CE) exam
|

|
Similar Civil Engineering (CE) Doubts
A simply supported beam ABC of length L carries a concentrated load P at an intermediate point B . If slope at A is 0.75 times the slope at C, then length of portion AB is equal to ?
Question Description
A simply supported beam ABC of length L carries a concentrated load P at an intermediate point B . If slope at A is 0.75 times the slope at C, then length of portion AB is equal to ? for Civil Engineering (CE) 2025 is part of Civil Engineering (CE) preparation. The Question and answers have been prepared according to the Civil Engineering (CE) exam syllabus. Information about A simply supported beam ABC of length L carries a concentrated load P at an intermediate point B . If slope at A is 0.75 times the slope at C, then length of portion AB is equal to ? covers all topics & solutions for Civil Engineering (CE) 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A simply supported beam ABC of length L carries a concentrated load P at an intermediate point B . If slope at A is 0.75 times the slope at C, then length of portion AB is equal to ?.
A simply supported beam ABC of length L carries a concentrated load P at an intermediate point B . If slope at A is 0.75 times the slope at C, then length of portion AB is equal to ? for Civil Engineering (CE) 2025 is part of Civil Engineering (CE) preparation. The Question and answers have been prepared according to the Civil Engineering (CE) exam syllabus. Information about A simply supported beam ABC of length L carries a concentrated load P at an intermediate point B . If slope at A is 0.75 times the slope at C, then length of portion AB is equal to ? covers all topics & solutions for Civil Engineering (CE) 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A simply supported beam ABC of length L carries a concentrated load P at an intermediate point B . If slope at A is 0.75 times the slope at C, then length of portion AB is equal to ?.
Solutions for A simply supported beam ABC of length L carries a concentrated load P at an intermediate point B . If slope at A is 0.75 times the slope at C, then length of portion AB is equal to ? in English & in Hindi are available as part of our courses for Civil Engineering (CE).
Download more important topics, notes, lectures and mock test series for Civil Engineering (CE) Exam by signing up for free.
Here you can find the meaning of A simply supported beam ABC of length L carries a concentrated load P at an intermediate point B . If slope at A is 0.75 times the slope at C, then length of portion AB is equal to ? defined & explained in the simplest way possible. Besides giving the explanation of
A simply supported beam ABC of length L carries a concentrated load P at an intermediate point B . If slope at A is 0.75 times the slope at C, then length of portion AB is equal to ?, a detailed solution for A simply supported beam ABC of length L carries a concentrated load P at an intermediate point B . If slope at A is 0.75 times the slope at C, then length of portion AB is equal to ? has been provided alongside types of A simply supported beam ABC of length L carries a concentrated load P at an intermediate point B . If slope at A is 0.75 times the slope at C, then length of portion AB is equal to ? theory, EduRev gives you an
ample number of questions to practice A simply supported beam ABC of length L carries a concentrated load P at an intermediate point B . If slope at A is 0.75 times the slope at C, then length of portion AB is equal to ? tests, examples and also practice Civil Engineering (CE) tests.

|
Explore Courses for Civil Engineering (CE) exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


























