JEE Exam > JEE Questions > Direction: Read the following text and answe...
Start Learning for Free
Direction: Read the following text and answer the following questions on the basis of the same:
Polio drops are delivered to 50K children in a district. The rate at which polio drops are given is directly proportional to the number of children who have not been administered the drops. By the end of 2nd week half the children have been given the polio drops. How many will have been given the drops by the end of 3rd week can be estimated using the solution to the differential equation dy/dx = k(50 - y) where x denotes the number of weeks and y the number of children who have been given the drops.
Q. Which of the following solutions may be used to find the number of children who have been given the polio drops?
- a)y = 50 – ekx
- b)y = 50 – e–kx
- c)y = 50(1 – e–kx)
- d)y = 50(e–kx – 1)
Correct answer is option 'C'. Can you explain this answer?
Most Upvoted Answer
Direction: Read the following text and answer the following questions...
We have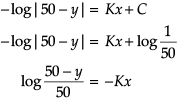
Free Test
FREE
| Start Free Test |
Community Answer
Direction: Read the following text and answer the following questions...
Solution:
Given:
- Polio drops are delivered to 50K children in a district.
- The rate at which polio drops are given is directly proportional to the number of children who have not been administered the drops.
- By the end of the 2nd week, half the children have been given the polio drops.
To find:
- The number of children who will have been given the drops by the end of the 3rd week.
Let's analyze the given information and solve the differential equation to find the solution.
1. Analyzing the given information:
- We are given that the rate at which polio drops are given is directly proportional to the number of children who have not been administered the drops.
- This means that the rate of administration of drops is proportional to the number of children remaining without drops, which can be represented as dy/dx = k(50 - y), where x denotes the number of weeks and y denotes the number of children who have been given the drops.
- By the end of the 2nd week, half the children have been given the polio drops. This means y = 50K/2 = 25K.
2. Solving the differential equation:
To solve the differential equation dy/dx = k(50 - y), we need to separate variables and integrate both sides.
dy/(50 - y) = k dx
Integrating both sides:
∫(1/(50 - y)) dy = ∫k dx
ln|50 - y| = kx + C1, where C1 is the constant of integration.
Now, let's solve for y:
|50 - y| = e^(kx + C1)
|50 - y| = e^(kx) * e^(C1)
Since e^(C1) is just another constant, let's denote it as C2:
|50 - y| = C2 * e^(kx)
3. Applying the initial condition:
By the end of the 2nd week, half the children have been given the polio drops, which means y = 25K. Substituting this value into the equation:
|50 - 25K| = C2 * e^(2k)
Since C2 is just a constant, let's denote it as C:
|50 - 25K| = C * e^(2k)
4. Simplifying the equation:
The absolute value expression can be written as two separate equations:
50 - 25K = C * e^(2k) (when 50 - 25K ≥ 0)
25K - 50 = C * e^(2k) (when 50 - 25K < />
5. Finding the value of C:
Since we know that y = 25K by the end of the 2nd week, we can substitute this value into one of the equations to find C:
25K - 50 = C * e^(2k)
25K = C * e^(2k) + 50
25K = C * 1 + 50 (since e^0 = 1)
25K = C + 50
C = 25K - 50
6. Writing the solution:
Substituting the value of C back into the equation:
|50 - 25K| = (25K - 50) * e
Given:
- Polio drops are delivered to 50K children in a district.
- The rate at which polio drops are given is directly proportional to the number of children who have not been administered the drops.
- By the end of the 2nd week, half the children have been given the polio drops.
To find:
- The number of children who will have been given the drops by the end of the 3rd week.
Let's analyze the given information and solve the differential equation to find the solution.
1. Analyzing the given information:
- We are given that the rate at which polio drops are given is directly proportional to the number of children who have not been administered the drops.
- This means that the rate of administration of drops is proportional to the number of children remaining without drops, which can be represented as dy/dx = k(50 - y), where x denotes the number of weeks and y denotes the number of children who have been given the drops.
- By the end of the 2nd week, half the children have been given the polio drops. This means y = 50K/2 = 25K.
2. Solving the differential equation:
To solve the differential equation dy/dx = k(50 - y), we need to separate variables and integrate both sides.
dy/(50 - y) = k dx
Integrating both sides:
∫(1/(50 - y)) dy = ∫k dx
ln|50 - y| = kx + C1, where C1 is the constant of integration.
Now, let's solve for y:
|50 - y| = e^(kx + C1)
|50 - y| = e^(kx) * e^(C1)
Since e^(C1) is just another constant, let's denote it as C2:
|50 - y| = C2 * e^(kx)
3. Applying the initial condition:
By the end of the 2nd week, half the children have been given the polio drops, which means y = 25K. Substituting this value into the equation:
|50 - 25K| = C2 * e^(2k)
Since C2 is just a constant, let's denote it as C:
|50 - 25K| = C * e^(2k)
4. Simplifying the equation:
The absolute value expression can be written as two separate equations:
50 - 25K = C * e^(2k) (when 50 - 25K ≥ 0)
25K - 50 = C * e^(2k) (when 50 - 25K < />
5. Finding the value of C:
Since we know that y = 25K by the end of the 2nd week, we can substitute this value into one of the equations to find C:
25K - 50 = C * e^(2k)
25K = C * e^(2k) + 50
25K = C * 1 + 50 (since e^0 = 1)
25K = C + 50
C = 25K - 50
6. Writing the solution:
Substituting the value of C back into the equation:
|50 - 25K| = (25K - 50) * e

|
Explore Courses for JEE exam
|

|
Similar JEE Doubts
Question Description
Direction: Read the following text and answer the following questions on the basis of the same:Polio drops are delivered to 50K children in a district. The rate at which polio drops are given is directly proportional to the number of children who have not been administered the drops. By the end of 2nd week half the children have been given the polio drops. How many will have been given the drops by the end of 3rd week can be estimated using the solution to the differential equation dy/dx = k(50 - y) where x denotes the number of weeks and y the number of children who have been given the drops.Q. Which of the following solutions may be used to find the number of children who have been given the polio drops?a)y = 50 – ekxb)y = 50 – e–kxc)y = 50(1 – e–kx)d)y = 50(e–kx – 1)Correct answer is option 'C'. Can you explain this answer? for JEE 2025 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about Direction: Read the following text and answer the following questions on the basis of the same:Polio drops are delivered to 50K children in a district. The rate at which polio drops are given is directly proportional to the number of children who have not been administered the drops. By the end of 2nd week half the children have been given the polio drops. How many will have been given the drops by the end of 3rd week can be estimated using the solution to the differential equation dy/dx = k(50 - y) where x denotes the number of weeks and y the number of children who have been given the drops.Q. Which of the following solutions may be used to find the number of children who have been given the polio drops?a)y = 50 – ekxb)y = 50 – e–kxc)y = 50(1 – e–kx)d)y = 50(e–kx – 1)Correct answer is option 'C'. Can you explain this answer? covers all topics & solutions for JEE 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Direction: Read the following text and answer the following questions on the basis of the same:Polio drops are delivered to 50K children in a district. The rate at which polio drops are given is directly proportional to the number of children who have not been administered the drops. By the end of 2nd week half the children have been given the polio drops. How many will have been given the drops by the end of 3rd week can be estimated using the solution to the differential equation dy/dx = k(50 - y) where x denotes the number of weeks and y the number of children who have been given the drops.Q. Which of the following solutions may be used to find the number of children who have been given the polio drops?a)y = 50 – ekxb)y = 50 – e–kxc)y = 50(1 – e–kx)d)y = 50(e–kx – 1)Correct answer is option 'C'. Can you explain this answer?.
Direction: Read the following text and answer the following questions on the basis of the same:Polio drops are delivered to 50K children in a district. The rate at which polio drops are given is directly proportional to the number of children who have not been administered the drops. By the end of 2nd week half the children have been given the polio drops. How many will have been given the drops by the end of 3rd week can be estimated using the solution to the differential equation dy/dx = k(50 - y) where x denotes the number of weeks and y the number of children who have been given the drops.Q. Which of the following solutions may be used to find the number of children who have been given the polio drops?a)y = 50 – ekxb)y = 50 – e–kxc)y = 50(1 – e–kx)d)y = 50(e–kx – 1)Correct answer is option 'C'. Can you explain this answer? for JEE 2025 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about Direction: Read the following text and answer the following questions on the basis of the same:Polio drops are delivered to 50K children in a district. The rate at which polio drops are given is directly proportional to the number of children who have not been administered the drops. By the end of 2nd week half the children have been given the polio drops. How many will have been given the drops by the end of 3rd week can be estimated using the solution to the differential equation dy/dx = k(50 - y) where x denotes the number of weeks and y the number of children who have been given the drops.Q. Which of the following solutions may be used to find the number of children who have been given the polio drops?a)y = 50 – ekxb)y = 50 – e–kxc)y = 50(1 – e–kx)d)y = 50(e–kx – 1)Correct answer is option 'C'. Can you explain this answer? covers all topics & solutions for JEE 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Direction: Read the following text and answer the following questions on the basis of the same:Polio drops are delivered to 50K children in a district. The rate at which polio drops are given is directly proportional to the number of children who have not been administered the drops. By the end of 2nd week half the children have been given the polio drops. How many will have been given the drops by the end of 3rd week can be estimated using the solution to the differential equation dy/dx = k(50 - y) where x denotes the number of weeks and y the number of children who have been given the drops.Q. Which of the following solutions may be used to find the number of children who have been given the polio drops?a)y = 50 – ekxb)y = 50 – e–kxc)y = 50(1 – e–kx)d)y = 50(e–kx – 1)Correct answer is option 'C'. Can you explain this answer?.
Solutions for Direction: Read the following text and answer the following questions on the basis of the same:Polio drops are delivered to 50K children in a district. The rate at which polio drops are given is directly proportional to the number of children who have not been administered the drops. By the end of 2nd week half the children have been given the polio drops. How many will have been given the drops by the end of 3rd week can be estimated using the solution to the differential equation dy/dx = k(50 - y) where x denotes the number of weeks and y the number of children who have been given the drops.Q. Which of the following solutions may be used to find the number of children who have been given the polio drops?a)y = 50 – ekxb)y = 50 – e–kxc)y = 50(1 – e–kx)d)y = 50(e–kx – 1)Correct answer is option 'C'. Can you explain this answer? in English & in Hindi are available as part of our courses for JEE.
Download more important topics, notes, lectures and mock test series for JEE Exam by signing up for free.
Here you can find the meaning of Direction: Read the following text and answer the following questions on the basis of the same:Polio drops are delivered to 50K children in a district. The rate at which polio drops are given is directly proportional to the number of children who have not been administered the drops. By the end of 2nd week half the children have been given the polio drops. How many will have been given the drops by the end of 3rd week can be estimated using the solution to the differential equation dy/dx = k(50 - y) where x denotes the number of weeks and y the number of children who have been given the drops.Q. Which of the following solutions may be used to find the number of children who have been given the polio drops?a)y = 50 – ekxb)y = 50 – e–kxc)y = 50(1 – e–kx)d)y = 50(e–kx – 1)Correct answer is option 'C'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
Direction: Read the following text and answer the following questions on the basis of the same:Polio drops are delivered to 50K children in a district. The rate at which polio drops are given is directly proportional to the number of children who have not been administered the drops. By the end of 2nd week half the children have been given the polio drops. How many will have been given the drops by the end of 3rd week can be estimated using the solution to the differential equation dy/dx = k(50 - y) where x denotes the number of weeks and y the number of children who have been given the drops.Q. Which of the following solutions may be used to find the number of children who have been given the polio drops?a)y = 50 – ekxb)y = 50 – e–kxc)y = 50(1 – e–kx)d)y = 50(e–kx – 1)Correct answer is option 'C'. Can you explain this answer?, a detailed solution for Direction: Read the following text and answer the following questions on the basis of the same:Polio drops are delivered to 50K children in a district. The rate at which polio drops are given is directly proportional to the number of children who have not been administered the drops. By the end of 2nd week half the children have been given the polio drops. How many will have been given the drops by the end of 3rd week can be estimated using the solution to the differential equation dy/dx = k(50 - y) where x denotes the number of weeks and y the number of children who have been given the drops.Q. Which of the following solutions may be used to find the number of children who have been given the polio drops?a)y = 50 – ekxb)y = 50 – e–kxc)y = 50(1 – e–kx)d)y = 50(e–kx – 1)Correct answer is option 'C'. Can you explain this answer? has been provided alongside types of Direction: Read the following text and answer the following questions on the basis of the same:Polio drops are delivered to 50K children in a district. The rate at which polio drops are given is directly proportional to the number of children who have not been administered the drops. By the end of 2nd week half the children have been given the polio drops. How many will have been given the drops by the end of 3rd week can be estimated using the solution to the differential equation dy/dx = k(50 - y) where x denotes the number of weeks and y the number of children who have been given the drops.Q. Which of the following solutions may be used to find the number of children who have been given the polio drops?a)y = 50 – ekxb)y = 50 – e–kxc)y = 50(1 – e–kx)d)y = 50(e–kx – 1)Correct answer is option 'C'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice Direction: Read the following text and answer the following questions on the basis of the same:Polio drops are delivered to 50K children in a district. The rate at which polio drops are given is directly proportional to the number of children who have not been administered the drops. By the end of 2nd week half the children have been given the polio drops. How many will have been given the drops by the end of 3rd week can be estimated using the solution to the differential equation dy/dx = k(50 - y) where x denotes the number of weeks and y the number of children who have been given the drops.Q. Which of the following solutions may be used to find the number of children who have been given the polio drops?a)y = 50 – ekxb)y = 50 – e–kxc)y = 50(1 – e–kx)d)y = 50(e–kx – 1)Correct answer is option 'C'. Can you explain this answer? tests, examples and also practice JEE tests.

|
Explore Courses for JEE exam
|

|
Signup to solve all Doubts
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.





















