JEE Exam > JEE Questions > In a parallel plate air capacitor, the dista...
Start Learning for Free
In a parallel plate air capacitor, the distance between plates is reduced to one fourth and the space between them is filled with a dielectric medium of constant 2. If the initial capacity of the capacitor is 4μF. then its new capacity is
- a)32 μF
- b)18 μF
- c)8 μF
- d)44 μF
Correct answer is option 'A'. Can you explain this answer?
Most Upvoted Answer
In a parallel plate air capacitor, the distance between plates is red...
The capacitance of a parallel plate air capacitor is given by C0 = ε0A/d …(i)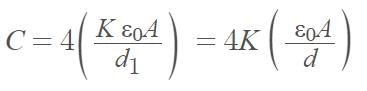
Whore, ε0 = permittivity of the medium,
A = area of plates
and d = distance between the plates
When the distance between plates is reduced and a dielectric slab is introduced, then capacitance becomes
C = Kε0A/d1 …(ii)
where, K = dielectric constant of the medium.
Here, K = 2, d1 =d/4 and C0 = 4μF = 4 x 10-6F
From Eq. (ii), we get
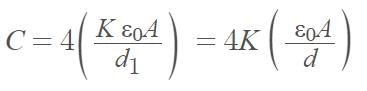
= 4KC0 …(iii) [from Eq. (i)]
Substituting given values in Eq. (iii), we get C = 4 x 2 x 4 = 32 μF
Community Answer
In a parallel plate air capacitor, the distance between plates is red...
Explanation:
Given, initial capacitance of the parallel plate air capacitor, C = 4 μF
The distance between the plates is reduced to one fourth, i.e., d' = d/4
The dielectric constant of the medium filled between the plates is 2.
We know that the capacitance of a parallel plate capacitor is given by:
C = ε0A/d
where ε0 is the permittivity of free space, A is the area of the plates and d is the distance between the plates.
New Capacitance:
The new capacitance of the capacitor can be found using the formula:
C' = ε0A/d'
where d' is the new distance between the plates.
Since the area of the plates and the permittivity of free space remain constant, we have:
C' = C(d/d')
Substituting the given values, we get:
C' = 4(4/1) = 16 μF
Effect of dielectric medium:
When a dielectric material is introduced between the plates of a capacitor, the capacitance increases. The capacitance of the capacitor with a dielectric medium can be given by:
C' = kε0A/d'
where k is the dielectric constant of the material and ε0 is the permittivity of free space.
Substituting the given values, we get:
C'' = 2ε0A/(d/4) = 8ε0A/d
Therefore, the new capacitance of the capacitor with the dielectric medium is 8 times the initial capacitance.
Final Answer:
The new capacitance of the capacitor when the distance between plates is reduced to one fourth and the space between them is filled with a dielectric medium of constant 2 is:
C'' = 16 x 8 = 32 μF
Hence, the correct answer is option (a) 32 μF.
Given, initial capacitance of the parallel plate air capacitor, C = 4 μF
The distance between the plates is reduced to one fourth, i.e., d' = d/4
The dielectric constant of the medium filled between the plates is 2.
We know that the capacitance of a parallel plate capacitor is given by:
C = ε0A/d
where ε0 is the permittivity of free space, A is the area of the plates and d is the distance between the plates.
New Capacitance:
The new capacitance of the capacitor can be found using the formula:
C' = ε0A/d'
where d' is the new distance between the plates.
Since the area of the plates and the permittivity of free space remain constant, we have:
C' = C(d/d')
Substituting the given values, we get:
C' = 4(4/1) = 16 μF
Effect of dielectric medium:
When a dielectric material is introduced between the plates of a capacitor, the capacitance increases. The capacitance of the capacitor with a dielectric medium can be given by:
C' = kε0A/d'
where k is the dielectric constant of the material and ε0 is the permittivity of free space.
Substituting the given values, we get:
C'' = 2ε0A/(d/4) = 8ε0A/d
Therefore, the new capacitance of the capacitor with the dielectric medium is 8 times the initial capacitance.
Final Answer:
The new capacitance of the capacitor when the distance between plates is reduced to one fourth and the space between them is filled with a dielectric medium of constant 2 is:
C'' = 16 x 8 = 32 μF
Hence, the correct answer is option (a) 32 μF.

|
Explore Courses for JEE exam
|

|
Similar JEE Doubts
In a parallel plate air capacitor, the distance between plates is reduced to one fourth and the space between them is filled with a dielectric medium of constant 2. If the initial capacity of the capacitor is 4μF. then its new capacity isa)32 μFb)18 μFc)8 μFd)44 μFCorrect answer is option 'A'. Can you explain this answer?
Question Description
In a parallel plate air capacitor, the distance between plates is reduced to one fourth and the space between them is filled with a dielectric medium of constant 2. If the initial capacity of the capacitor is 4μF. then its new capacity isa)32 μFb)18 μFc)8 μFd)44 μFCorrect answer is option 'A'. Can you explain this answer? for JEE 2025 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about In a parallel plate air capacitor, the distance between plates is reduced to one fourth and the space between them is filled with a dielectric medium of constant 2. If the initial capacity of the capacitor is 4μF. then its new capacity isa)32 μFb)18 μFc)8 μFd)44 μFCorrect answer is option 'A'. Can you explain this answer? covers all topics & solutions for JEE 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for In a parallel plate air capacitor, the distance between plates is reduced to one fourth and the space between them is filled with a dielectric medium of constant 2. If the initial capacity of the capacitor is 4μF. then its new capacity isa)32 μFb)18 μFc)8 μFd)44 μFCorrect answer is option 'A'. Can you explain this answer?.
In a parallel plate air capacitor, the distance between plates is reduced to one fourth and the space between them is filled with a dielectric medium of constant 2. If the initial capacity of the capacitor is 4μF. then its new capacity isa)32 μFb)18 μFc)8 μFd)44 μFCorrect answer is option 'A'. Can you explain this answer? for JEE 2025 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about In a parallel plate air capacitor, the distance between plates is reduced to one fourth and the space between them is filled with a dielectric medium of constant 2. If the initial capacity of the capacitor is 4μF. then its new capacity isa)32 μFb)18 μFc)8 μFd)44 μFCorrect answer is option 'A'. Can you explain this answer? covers all topics & solutions for JEE 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for In a parallel plate air capacitor, the distance between plates is reduced to one fourth and the space between them is filled with a dielectric medium of constant 2. If the initial capacity of the capacitor is 4μF. then its new capacity isa)32 μFb)18 μFc)8 μFd)44 μFCorrect answer is option 'A'. Can you explain this answer?.
Solutions for In a parallel plate air capacitor, the distance between plates is reduced to one fourth and the space between them is filled with a dielectric medium of constant 2. If the initial capacity of the capacitor is 4μF. then its new capacity isa)32 μFb)18 μFc)8 μFd)44 μFCorrect answer is option 'A'. Can you explain this answer? in English & in Hindi are available as part of our courses for JEE.
Download more important topics, notes, lectures and mock test series for JEE Exam by signing up for free.
Here you can find the meaning of In a parallel plate air capacitor, the distance between plates is reduced to one fourth and the space between them is filled with a dielectric medium of constant 2. If the initial capacity of the capacitor is 4μF. then its new capacity isa)32 μFb)18 μFc)8 μFd)44 μFCorrect answer is option 'A'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
In a parallel plate air capacitor, the distance between plates is reduced to one fourth and the space between them is filled with a dielectric medium of constant 2. If the initial capacity of the capacitor is 4μF. then its new capacity isa)32 μFb)18 μFc)8 μFd)44 μFCorrect answer is option 'A'. Can you explain this answer?, a detailed solution for In a parallel plate air capacitor, the distance between plates is reduced to one fourth and the space between them is filled with a dielectric medium of constant 2. If the initial capacity of the capacitor is 4μF. then its new capacity isa)32 μFb)18 μFc)8 μFd)44 μFCorrect answer is option 'A'. Can you explain this answer? has been provided alongside types of In a parallel plate air capacitor, the distance between plates is reduced to one fourth and the space between them is filled with a dielectric medium of constant 2. If the initial capacity of the capacitor is 4μF. then its new capacity isa)32 μFb)18 μFc)8 μFd)44 μFCorrect answer is option 'A'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice In a parallel plate air capacitor, the distance between plates is reduced to one fourth and the space between them is filled with a dielectric medium of constant 2. If the initial capacity of the capacitor is 4μF. then its new capacity isa)32 μFb)18 μFc)8 μFd)44 μFCorrect answer is option 'A'. Can you explain this answer? tests, examples and also practice JEE tests.

|
Explore Courses for JEE exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


























