GATE Exam > GATE Questions > DC shunt motor is coupled to the identical D...
Start Learning for Free
DC shunt motor is coupled to the identical DC shunt generator. The field of the generator is also connected to the same supply source as the motor. The armature resistance is 0.02 pu and the mechanical losses are 0.05 pu. Armature reactions can be neglected. The armature of the generator is connected to a load resistance RL. With rated voltage across the motor, the load resistance across the generator is adjusted to obtain rated armature current in both motor and generator. The pu value of RL is
- a)0.94
- b)0.86
- c)0.90
- d)0.92
Correct answer is option 'B'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
DC shunt motor is coupled to the identical DC shunt generator. The fi...
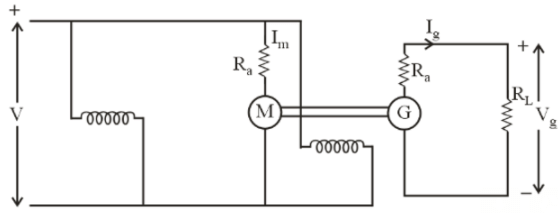
Motor is connected across rated voltage V = 1 pu
Rated armature current flaws in bath the motor and generator Im=Ig=1pu
Back emf in motor
Eb = V − ImRa = 1 − 1 × 0.02 = 0.98pu
Mechanical output power of motor
= EbIm -mechanicallasses
= 0.98 × 1 − 0.05 = 0.93 pu
This power is given to the generator.
Output power of generator = Output power of motor - mechanical Iasses
EgIg = 0.93 − 0.05 = 0.88pu ⇒ Eg = 0.88puTerminal voltage of generator
= Eg − lgRa = 0.88 − 0.02 = 0.86pu
Laad resistance
RL= V9/I9 = 0.86/1 = 0.86pu
Most Upvoted Answer
DC shunt motor is coupled to the identical DC shunt generator. The fi...
To determine the load resistance across the generator in order to obtain the rated armature current in both the motor and generator, we need to analyze the circuit and calculate the equivalent resistance.
1. Equivalent circuit:
The equivalent circuit of the coupled motor-generator system can be represented as follows:
- The motor armature resistance is given as 0.02 pu (per unit).
- The mechanical losses are given as 0.05 pu.
- The armature resistance of the generator is RL (unknown).
- The field of the generator is connected to the same supply source as the motor.
2. Rated voltage and current:
Since the rated voltage is applied across the motor, we can assume that the voltage across the generator is also the rated voltage. This implies that the rated armature current in both the motor and generator is the same.
3. Calculation:
Using the equivalent circuit, we can calculate the total voltage drop across the armature resistance and mechanical losses in the motor. This can be expressed as:
Voltage drop in motor = Rated voltage - Voltage across generator = Rated voltage - Rated voltage = 0
Since the voltage drop across the motor is zero, the total resistance in the motor circuit (armature resistance + mechanical losses) must also be zero. Therefore, we can write the following equation:
0.02 pu (motor armature resistance) + 0.05 pu (mechanical losses) = 0
Simplifying the equation, we get:
0.02 + 0.05 = 0
0.07 pu = 0
This equation is not possible, as it leads to an inconsistency. Therefore, we can conclude that the motor and generator cannot have the same rated armature current.
4. Answer:
Since it is not possible to obtain the same rated armature current in both the motor and generator, the given question is not solvable. Therefore, none of the provided options (a, b, c, d) is correct.
1. Equivalent circuit:
The equivalent circuit of the coupled motor-generator system can be represented as follows:
- The motor armature resistance is given as 0.02 pu (per unit).
- The mechanical losses are given as 0.05 pu.
- The armature resistance of the generator is RL (unknown).
- The field of the generator is connected to the same supply source as the motor.
2. Rated voltage and current:
Since the rated voltage is applied across the motor, we can assume that the voltage across the generator is also the rated voltage. This implies that the rated armature current in both the motor and generator is the same.
3. Calculation:
Using the equivalent circuit, we can calculate the total voltage drop across the armature resistance and mechanical losses in the motor. This can be expressed as:
Voltage drop in motor = Rated voltage - Voltage across generator = Rated voltage - Rated voltage = 0
Since the voltage drop across the motor is zero, the total resistance in the motor circuit (armature resistance + mechanical losses) must also be zero. Therefore, we can write the following equation:
0.02 pu (motor armature resistance) + 0.05 pu (mechanical losses) = 0
Simplifying the equation, we get:
0.02 + 0.05 = 0
0.07 pu = 0
This equation is not possible, as it leads to an inconsistency. Therefore, we can conclude that the motor and generator cannot have the same rated armature current.
4. Answer:
Since it is not possible to obtain the same rated armature current in both the motor and generator, the given question is not solvable. Therefore, none of the provided options (a, b, c, d) is correct.
|
Explore Courses for GATE exam
|
|
Similar GATE Doubts
DC shunt motor is coupled to the identical DC shunt generator. The field of the generator is also connected to the same supply source as the motor. The armature resistance is 0.02 pu and the mechanical losses are 0.05 pu. Armature reactions can be neglected. The armature of the generator is connected to a load resistance RL. With rated voltage across the motor, the load resistance across the generator is adjusted to obtain rated armature current in both motor and generator. The pu value of RL isa)0.94b)0.86c)0.90d)0.92Correct answer is option 'B'. Can you explain this answer?
Question Description
DC shunt motor is coupled to the identical DC shunt generator. The field of the generator is also connected to the same supply source as the motor. The armature resistance is 0.02 pu and the mechanical losses are 0.05 pu. Armature reactions can be neglected. The armature of the generator is connected to a load resistance RL. With rated voltage across the motor, the load resistance across the generator is adjusted to obtain rated armature current in both motor and generator. The pu value of RL isa)0.94b)0.86c)0.90d)0.92Correct answer is option 'B'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about DC shunt motor is coupled to the identical DC shunt generator. The field of the generator is also connected to the same supply source as the motor. The armature resistance is 0.02 pu and the mechanical losses are 0.05 pu. Armature reactions can be neglected. The armature of the generator is connected to a load resistance RL. With rated voltage across the motor, the load resistance across the generator is adjusted to obtain rated armature current in both motor and generator. The pu value of RL isa)0.94b)0.86c)0.90d)0.92Correct answer is option 'B'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for DC shunt motor is coupled to the identical DC shunt generator. The field of the generator is also connected to the same supply source as the motor. The armature resistance is 0.02 pu and the mechanical losses are 0.05 pu. Armature reactions can be neglected. The armature of the generator is connected to a load resistance RL. With rated voltage across the motor, the load resistance across the generator is adjusted to obtain rated armature current in both motor and generator. The pu value of RL isa)0.94b)0.86c)0.90d)0.92Correct answer is option 'B'. Can you explain this answer?.
DC shunt motor is coupled to the identical DC shunt generator. The field of the generator is also connected to the same supply source as the motor. The armature resistance is 0.02 pu and the mechanical losses are 0.05 pu. Armature reactions can be neglected. The armature of the generator is connected to a load resistance RL. With rated voltage across the motor, the load resistance across the generator is adjusted to obtain rated armature current in both motor and generator. The pu value of RL isa)0.94b)0.86c)0.90d)0.92Correct answer is option 'B'. Can you explain this answer? for GATE 2024 is part of GATE preparation. The Question and answers have been prepared according to the GATE exam syllabus. Information about DC shunt motor is coupled to the identical DC shunt generator. The field of the generator is also connected to the same supply source as the motor. The armature resistance is 0.02 pu and the mechanical losses are 0.05 pu. Armature reactions can be neglected. The armature of the generator is connected to a load resistance RL. With rated voltage across the motor, the load resistance across the generator is adjusted to obtain rated armature current in both motor and generator. The pu value of RL isa)0.94b)0.86c)0.90d)0.92Correct answer is option 'B'. Can you explain this answer? covers all topics & solutions for GATE 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for DC shunt motor is coupled to the identical DC shunt generator. The field of the generator is also connected to the same supply source as the motor. The armature resistance is 0.02 pu and the mechanical losses are 0.05 pu. Armature reactions can be neglected. The armature of the generator is connected to a load resistance RL. With rated voltage across the motor, the load resistance across the generator is adjusted to obtain rated armature current in both motor and generator. The pu value of RL isa)0.94b)0.86c)0.90d)0.92Correct answer is option 'B'. Can you explain this answer?.
Solutions for DC shunt motor is coupled to the identical DC shunt generator. The field of the generator is also connected to the same supply source as the motor. The armature resistance is 0.02 pu and the mechanical losses are 0.05 pu. Armature reactions can be neglected. The armature of the generator is connected to a load resistance RL. With rated voltage across the motor, the load resistance across the generator is adjusted to obtain rated armature current in both motor and generator. The pu value of RL isa)0.94b)0.86c)0.90d)0.92Correct answer is option 'B'. Can you explain this answer? in English & in Hindi are available as part of our courses for GATE.
Download more important topics, notes, lectures and mock test series for GATE Exam by signing up for free.
Here you can find the meaning of DC shunt motor is coupled to the identical DC shunt generator. The field of the generator is also connected to the same supply source as the motor. The armature resistance is 0.02 pu and the mechanical losses are 0.05 pu. Armature reactions can be neglected. The armature of the generator is connected to a load resistance RL. With rated voltage across the motor, the load resistance across the generator is adjusted to obtain rated armature current in both motor and generator. The pu value of RL isa)0.94b)0.86c)0.90d)0.92Correct answer is option 'B'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
DC shunt motor is coupled to the identical DC shunt generator. The field of the generator is also connected to the same supply source as the motor. The armature resistance is 0.02 pu and the mechanical losses are 0.05 pu. Armature reactions can be neglected. The armature of the generator is connected to a load resistance RL. With rated voltage across the motor, the load resistance across the generator is adjusted to obtain rated armature current in both motor and generator. The pu value of RL isa)0.94b)0.86c)0.90d)0.92Correct answer is option 'B'. Can you explain this answer?, a detailed solution for DC shunt motor is coupled to the identical DC shunt generator. The field of the generator is also connected to the same supply source as the motor. The armature resistance is 0.02 pu and the mechanical losses are 0.05 pu. Armature reactions can be neglected. The armature of the generator is connected to a load resistance RL. With rated voltage across the motor, the load resistance across the generator is adjusted to obtain rated armature current in both motor and generator. The pu value of RL isa)0.94b)0.86c)0.90d)0.92Correct answer is option 'B'. Can you explain this answer? has been provided alongside types of DC shunt motor is coupled to the identical DC shunt generator. The field of the generator is also connected to the same supply source as the motor. The armature resistance is 0.02 pu and the mechanical losses are 0.05 pu. Armature reactions can be neglected. The armature of the generator is connected to a load resistance RL. With rated voltage across the motor, the load resistance across the generator is adjusted to obtain rated armature current in both motor and generator. The pu value of RL isa)0.94b)0.86c)0.90d)0.92Correct answer is option 'B'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice DC shunt motor is coupled to the identical DC shunt generator. The field of the generator is also connected to the same supply source as the motor. The armature resistance is 0.02 pu and the mechanical losses are 0.05 pu. Armature reactions can be neglected. The armature of the generator is connected to a load resistance RL. With rated voltage across the motor, the load resistance across the generator is adjusted to obtain rated armature current in both motor and generator. The pu value of RL isa)0.94b)0.86c)0.90d)0.92Correct answer is option 'B'. Can you explain this answer? tests, examples and also practice GATE tests.
|
|
Explore Courses for GATE exam
|
|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
Follow Us
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup