Civil Engineering (CE) Exam > Civil Engineering (CE) Questions > If f (z) = u + iv is an analytic function, th...
Start Learning for Free
If f (z) = u + iv is an analytic function, then
- a)u is harmonic function
- b)v is harmonic function
- c)Both u and v are harmonic functions
- d)Both u and v are not harmonic functions
Correct answer is option 'C'. Can you explain this answer?
Most Upvoted Answer
If f (z) = u + iv is an analytic function, thena)u is harmonic functio...
Explanation:
To understand why option C is the correct answer, let's first define what it means for a function to be analytic.
An analytic function is a complex-valued function that is differentiable at every point within its domain. In other words, the function has a derivative at each point within its domain.
Given that f(z) = u + iv is an analytic function, we can conclude the following:
Harmonic Functions:
A harmonic function is a real-valued function that satisfies Laplace's equation, which states that the sum of the second-order partial derivatives of the function is equal to zero. In other words, if u is a harmonic function, then it satisfies ∇²u = 0, where ∇² is the Laplacian operator.
Similarly, if v is a harmonic function, then it satisfies ∇²v = 0.
Derivatives of Analytic Functions:
Since f(z) is an analytic function, it means that both u and v have continuous first-order partial derivatives. This allows us to use the Cauchy-Riemann equations.
The Cauchy-Riemann equations relate the partial derivatives of u and v. They state that if f(z) = u + iv is an analytic function, then the following conditions must hold:
1. ∂u/∂x = ∂v/∂y
2. ∂u/∂y = -∂v/∂x
From these equations, we can see that the real part (u) and the imaginary part (v) of an analytic function are related to each other.
Conclusion:
Based on the above information, we can conclude that both u and v are harmonic functions. This is because if u and v are related to each other through the Cauchy-Riemann equations, and f(z) is an analytic function, then both u and v must satisfy Laplace's equation.
Therefore, the correct answer is option C: Both u and v are harmonic functions.
To understand why option C is the correct answer, let's first define what it means for a function to be analytic.
An analytic function is a complex-valued function that is differentiable at every point within its domain. In other words, the function has a derivative at each point within its domain.
Given that f(z) = u + iv is an analytic function, we can conclude the following:
Harmonic Functions:
A harmonic function is a real-valued function that satisfies Laplace's equation, which states that the sum of the second-order partial derivatives of the function is equal to zero. In other words, if u is a harmonic function, then it satisfies ∇²u = 0, where ∇² is the Laplacian operator.
Similarly, if v is a harmonic function, then it satisfies ∇²v = 0.
Derivatives of Analytic Functions:
Since f(z) is an analytic function, it means that both u and v have continuous first-order partial derivatives. This allows us to use the Cauchy-Riemann equations.
The Cauchy-Riemann equations relate the partial derivatives of u and v. They state that if f(z) = u + iv is an analytic function, then the following conditions must hold:
1. ∂u/∂x = ∂v/∂y
2. ∂u/∂y = -∂v/∂x
From these equations, we can see that the real part (u) and the imaginary part (v) of an analytic function are related to each other.
Conclusion:
Based on the above information, we can conclude that both u and v are harmonic functions. This is because if u and v are related to each other through the Cauchy-Riemann equations, and f(z) is an analytic function, then both u and v must satisfy Laplace's equation.
Therefore, the correct answer is option C: Both u and v are harmonic functions.
Free Test
FREE
| Start Free Test |
Community Answer
If f (z) = u + iv is an analytic function, thena)u is harmonic functio...
Concept:
If a function f satisfies Laplace's equation ∇2f = 0, then f is said to be a harmonic function.
Calculation:
If f (z) = u + iv is an analytic function, then
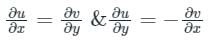
Now
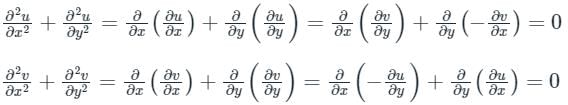
Both u and v are satisfying Laplace’s equation (∇2f = 0).
∴ Both u and v are harmonic functions.
If a function f satisfies Laplace's equation ∇2f = 0, then f is said to be a harmonic function.
Calculation:
If f (z) = u + iv is an analytic function, then
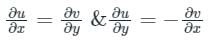
Now
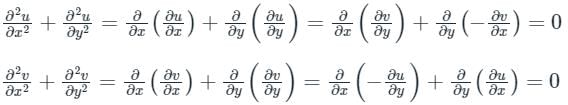
Both u and v are satisfying Laplace’s equation (∇2f = 0).
∴ Both u and v are harmonic functions.

|
Explore Courses for Civil Engineering (CE) exam
|

|
Question Description
If f (z) = u + iv is an analytic function, thena)u is harmonic functionb)v is harmonic functionc)Both u and v are harmonic functionsd)Both u and v are not harmonic functionsCorrect answer is option 'C'. Can you explain this answer? for Civil Engineering (CE) 2025 is part of Civil Engineering (CE) preparation. The Question and answers have been prepared according to the Civil Engineering (CE) exam syllabus. Information about If f (z) = u + iv is an analytic function, thena)u is harmonic functionb)v is harmonic functionc)Both u and v are harmonic functionsd)Both u and v are not harmonic functionsCorrect answer is option 'C'. Can you explain this answer? covers all topics & solutions for Civil Engineering (CE) 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for If f (z) = u + iv is an analytic function, thena)u is harmonic functionb)v is harmonic functionc)Both u and v are harmonic functionsd)Both u and v are not harmonic functionsCorrect answer is option 'C'. Can you explain this answer?.
If f (z) = u + iv is an analytic function, thena)u is harmonic functionb)v is harmonic functionc)Both u and v are harmonic functionsd)Both u and v are not harmonic functionsCorrect answer is option 'C'. Can you explain this answer? for Civil Engineering (CE) 2025 is part of Civil Engineering (CE) preparation. The Question and answers have been prepared according to the Civil Engineering (CE) exam syllabus. Information about If f (z) = u + iv is an analytic function, thena)u is harmonic functionb)v is harmonic functionc)Both u and v are harmonic functionsd)Both u and v are not harmonic functionsCorrect answer is option 'C'. Can you explain this answer? covers all topics & solutions for Civil Engineering (CE) 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for If f (z) = u + iv is an analytic function, thena)u is harmonic functionb)v is harmonic functionc)Both u and v are harmonic functionsd)Both u and v are not harmonic functionsCorrect answer is option 'C'. Can you explain this answer?.
Solutions for If f (z) = u + iv is an analytic function, thena)u is harmonic functionb)v is harmonic functionc)Both u and v are harmonic functionsd)Both u and v are not harmonic functionsCorrect answer is option 'C'. Can you explain this answer? in English & in Hindi are available as part of our courses for Civil Engineering (CE).
Download more important topics, notes, lectures and mock test series for Civil Engineering (CE) Exam by signing up for free.
Here you can find the meaning of If f (z) = u + iv is an analytic function, thena)u is harmonic functionb)v is harmonic functionc)Both u and v are harmonic functionsd)Both u and v are not harmonic functionsCorrect answer is option 'C'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
If f (z) = u + iv is an analytic function, thena)u is harmonic functionb)v is harmonic functionc)Both u and v are harmonic functionsd)Both u and v are not harmonic functionsCorrect answer is option 'C'. Can you explain this answer?, a detailed solution for If f (z) = u + iv is an analytic function, thena)u is harmonic functionb)v is harmonic functionc)Both u and v are harmonic functionsd)Both u and v are not harmonic functionsCorrect answer is option 'C'. Can you explain this answer? has been provided alongside types of If f (z) = u + iv is an analytic function, thena)u is harmonic functionb)v is harmonic functionc)Both u and v are harmonic functionsd)Both u and v are not harmonic functionsCorrect answer is option 'C'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice If f (z) = u + iv is an analytic function, thena)u is harmonic functionb)v is harmonic functionc)Both u and v are harmonic functionsd)Both u and v are not harmonic functionsCorrect answer is option 'C'. Can you explain this answer? tests, examples and also practice Civil Engineering (CE) tests.

|
Explore Courses for Civil Engineering (CE) exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


















