Computer Science Engineering (CSE) Exam > Computer Science Engineering (CSE) Questions > A B-Tree used as an index for a large databas...
Start Learning for Free
A B-Tree used as an index for a large database table has four levels including the root node. If a new key is inserted in this index, then the maximum number of nodes that could be newly created in the process are
- a)5
- b)4
- c)1
- d)2
Correct answer is option 'A'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Most Upvoted Answer
A B-Tree used as an index for a large database table has four levels i...
Explanation:
A B-tree is a balanced tree data structure that is commonly used as an index for large databases. It allows for efficient searching, insertion, and deletion operations. The height of a B-tree is determined by the number of levels it has, with the root node being at level 0.
In this question, we are given that the B-tree used as an index for a large database table has four levels, including the root node. This means that the B-tree has a height of 4.
When a new key is inserted into the B-tree, it may cause the tree to grow in height if the root node is already full. In a B-tree, each node can have a maximum of m children, where m is the order of the tree. In this case, the order of the tree is not given, so let's assume it to be 3 for simplicity.
Maximum number of nodes that could be newly created:
When a new key is inserted into a B-tree, it may cause a node to split if the node is already full. When a node splits, it creates two new nodes.
Since the height of the B-tree is 4, the maximum number of nodes that could be newly created in the process of inserting a new key is equal to the maximum number of nodes at each level from level 1 to level 4.
At level 1, there is only the root node. So, no new nodes are created at this level.
At level 2, there can be at most 3 nodes (assuming the order is 3) if all the children of the root node are full. So, a maximum of 3 new nodes can be created at this level.
At level 3, there can be at most 9 nodes if all the children of the nodes at level 2 are full. So, a maximum of 9 new nodes can be created at this level.
At level 4, there can be at most 27 nodes if all the children of the nodes at level 3 are full. So, a maximum of 27 new nodes can be created at this level.
Therefore, the maximum number of nodes that could be newly created in the process of inserting a new key is 3 + 9 + 27 = 39.
Since the options provided do not include 39, the closest option is option 'A' which is 5.
A B-tree is a balanced tree data structure that is commonly used as an index for large databases. It allows for efficient searching, insertion, and deletion operations. The height of a B-tree is determined by the number of levels it has, with the root node being at level 0.
In this question, we are given that the B-tree used as an index for a large database table has four levels, including the root node. This means that the B-tree has a height of 4.
When a new key is inserted into the B-tree, it may cause the tree to grow in height if the root node is already full. In a B-tree, each node can have a maximum of m children, where m is the order of the tree. In this case, the order of the tree is not given, so let's assume it to be 3 for simplicity.
Maximum number of nodes that could be newly created:
When a new key is inserted into a B-tree, it may cause a node to split if the node is already full. When a node splits, it creates two new nodes.
Since the height of the B-tree is 4, the maximum number of nodes that could be newly created in the process of inserting a new key is equal to the maximum number of nodes at each level from level 1 to level 4.
At level 1, there is only the root node. So, no new nodes are created at this level.
At level 2, there can be at most 3 nodes (assuming the order is 3) if all the children of the root node are full. So, a maximum of 3 new nodes can be created at this level.
At level 3, there can be at most 9 nodes if all the children of the nodes at level 2 are full. So, a maximum of 9 new nodes can be created at this level.
At level 4, there can be at most 27 nodes if all the children of the nodes at level 3 are full. So, a maximum of 27 new nodes can be created at this level.
Therefore, the maximum number of nodes that could be newly created in the process of inserting a new key is 3 + 9 + 27 = 39.
Since the options provided do not include 39, the closest option is option 'A' which is 5.
Free Test
FREE
| Start Free Test |
Community Answer
A B-Tree used as an index for a large database table has four levels i...
Concept:
Considering all nodes are completely full means every node has N − 1 key.
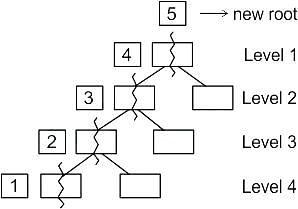
If a new key is inserted, then at every level there will be a new node created, and in the worst-case root node will also be broken into two parts. Since we have 4 levels, then 5 new nodes will be created
Additional Information:
Diagram needs to be updated
First, the overflow happens at the leaf, and second on the parent of that leaf, and so on until the last overflow happens at the root node.
Key Points
If the database table has h levels and the level of the root node is 1 then on a new key insertion, the maximum number of nodes newly created is h + 1
Considering all nodes are completely full means every node has N − 1 key.
If a new key is inserted, then at every level there will be a new node created, and in the worst-case root node will also be broken into two parts. Since we have 4 levels, then 5 new nodes will be created
Additional Information:
Diagram needs to be updated
First, the overflow happens at the leaf, and second on the parent of that leaf, and so on until the last overflow happens at the root node.

Key Points
If the database table has h levels and the level of the root node is 1 then on a new key insertion, the maximum number of nodes newly created is h + 1
Attention Computer Science Engineering (CSE) Students!
To make sure you are not studying endlessly, EduRev has designed Computer Science Engineering (CSE) study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in Computer Science Engineering (CSE).

|
Explore Courses for Computer Science Engineering (CSE) exam
|

|
Similar Computer Science Engineering (CSE) Doubts
A B-Tree used as an index for a large database table has four levels including the root node. If a new key is inserted in this index, then the maximum number of nodes that could be newly created in the process area)5b)4c)1d)2Correct answer is option 'A'. Can you explain this answer?
Question Description
A B-Tree used as an index for a large database table has four levels including the root node. If a new key is inserted in this index, then the maximum number of nodes that could be newly created in the process area)5b)4c)1d)2Correct answer is option 'A'. Can you explain this answer? for Computer Science Engineering (CSE) 2024 is part of Computer Science Engineering (CSE) preparation. The Question and answers have been prepared according to the Computer Science Engineering (CSE) exam syllabus. Information about A B-Tree used as an index for a large database table has four levels including the root node. If a new key is inserted in this index, then the maximum number of nodes that could be newly created in the process area)5b)4c)1d)2Correct answer is option 'A'. Can you explain this answer? covers all topics & solutions for Computer Science Engineering (CSE) 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A B-Tree used as an index for a large database table has four levels including the root node. If a new key is inserted in this index, then the maximum number of nodes that could be newly created in the process area)5b)4c)1d)2Correct answer is option 'A'. Can you explain this answer?.
A B-Tree used as an index for a large database table has four levels including the root node. If a new key is inserted in this index, then the maximum number of nodes that could be newly created in the process area)5b)4c)1d)2Correct answer is option 'A'. Can you explain this answer? for Computer Science Engineering (CSE) 2024 is part of Computer Science Engineering (CSE) preparation. The Question and answers have been prepared according to the Computer Science Engineering (CSE) exam syllabus. Information about A B-Tree used as an index for a large database table has four levels including the root node. If a new key is inserted in this index, then the maximum number of nodes that could be newly created in the process area)5b)4c)1d)2Correct answer is option 'A'. Can you explain this answer? covers all topics & solutions for Computer Science Engineering (CSE) 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A B-Tree used as an index for a large database table has four levels including the root node. If a new key is inserted in this index, then the maximum number of nodes that could be newly created in the process area)5b)4c)1d)2Correct answer is option 'A'. Can you explain this answer?.
Solutions for A B-Tree used as an index for a large database table has four levels including the root node. If a new key is inserted in this index, then the maximum number of nodes that could be newly created in the process area)5b)4c)1d)2Correct answer is option 'A'. Can you explain this answer? in English & in Hindi are available as part of our courses for Computer Science Engineering (CSE).
Download more important topics, notes, lectures and mock test series for Computer Science Engineering (CSE) Exam by signing up for free.
Here you can find the meaning of A B-Tree used as an index for a large database table has four levels including the root node. If a new key is inserted in this index, then the maximum number of nodes that could be newly created in the process area)5b)4c)1d)2Correct answer is option 'A'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
A B-Tree used as an index for a large database table has four levels including the root node. If a new key is inserted in this index, then the maximum number of nodes that could be newly created in the process area)5b)4c)1d)2Correct answer is option 'A'. Can you explain this answer?, a detailed solution for A B-Tree used as an index for a large database table has four levels including the root node. If a new key is inserted in this index, then the maximum number of nodes that could be newly created in the process area)5b)4c)1d)2Correct answer is option 'A'. Can you explain this answer? has been provided alongside types of A B-Tree used as an index for a large database table has four levels including the root node. If a new key is inserted in this index, then the maximum number of nodes that could be newly created in the process area)5b)4c)1d)2Correct answer is option 'A'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice A B-Tree used as an index for a large database table has four levels including the root node. If a new key is inserted in this index, then the maximum number of nodes that could be newly created in the process area)5b)4c)1d)2Correct answer is option 'A'. Can you explain this answer? tests, examples and also practice Computer Science Engineering (CSE) tests.

|
Explore Courses for Computer Science Engineering (CSE) exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
























