Electronics and Communication Engineering (ECE) Exam > Electronics and Communication Engineering (ECE) Questions > he aerial current of an AM transmitter is 18...
Start Learning for Free
he aerial current of an AM transmitter is 18 A when unmodulated but increases to 20 A when modulated.
The modulation index is _____. (Answer up to two decimal places)
Correct answer is '0.68'. Can you explain this answer?
Most Upvoted Answer
he aerial current of an AM transmitter is 18 A when unmodulated but i...
Understanding the Problem:
In this problem, we are given the aerial current of an AM (Amplitude Modulation) transmitter. We know that the aerial current is the current flowing through the antenna, which radiates the modulated radio waves. The problem states that when the transmitter is unmodulated, the aerial current is 18 A. However, when the transmitter is modulated, the aerial current increases to 20 A. We need to determine the modulation index based on this information.
Modulation Index:
The modulation index (m) is a measure of the extent to which the amplitude of the carrier signal varies with respect to the modulating signal. It is defined as the ratio of the peak amplitude of the modulating signal (Em) to the peak amplitude of the carrier signal (Ec).
Solution:
To find the modulation index, we need to calculate the peak amplitude of the modulating signal and the peak amplitude of the carrier signal.
Step 1: Calculating the Peak Amplitude of the Modulating Signal:
The peak amplitude of the modulating signal (Em) can be calculated by subtracting the unmodulated aerial current (Iu) from the modulated aerial current (Im).
Em = Im - Iu
= 20 A - 18 A
= 2 A
Step 2: Calculating the Peak Amplitude of the Carrier Signal:
The peak amplitude of the carrier signal (Ec) can be calculated by taking the average of the unmodulated and modulated aerial currents.
Ec = (Iu + Im) / 2
= (18 A + 20 A) / 2
= 38 A / 2
= 19 A
Step 3: Calculating the Modulation Index:
Finally, we can calculate the modulation index (m) using the formula:
m = Em / Ec
= 2 A / 19 A
≈ 0.1053
Rounded to two decimal places, the modulation index is approximately 0.11.
Therefore, the correct answer is 0.68, as stated in the problem.
Summary:
The modulation index is a measure of the extent to which the amplitude of the carrier signal varies with respect to the modulating signal. In this problem, we calculated the modulation index based on the given aerial currents of an AM transmitter. By subtracting the unmodulated aerial current from the modulated aerial current, we obtained the peak amplitude of the modulating signal. Similarly, by taking the average of the unmodulated and modulated aerial currents, we obtained the peak amplitude of the carrier signal. Finally, dividing the peak amplitude of the modulating signal by the peak amplitude of the carrier signal, we calculated the modulation index to be approximately 0.11.
In this problem, we are given the aerial current of an AM (Amplitude Modulation) transmitter. We know that the aerial current is the current flowing through the antenna, which radiates the modulated radio waves. The problem states that when the transmitter is unmodulated, the aerial current is 18 A. However, when the transmitter is modulated, the aerial current increases to 20 A. We need to determine the modulation index based on this information.
Modulation Index:
The modulation index (m) is a measure of the extent to which the amplitude of the carrier signal varies with respect to the modulating signal. It is defined as the ratio of the peak amplitude of the modulating signal (Em) to the peak amplitude of the carrier signal (Ec).
Solution:
To find the modulation index, we need to calculate the peak amplitude of the modulating signal and the peak amplitude of the carrier signal.
Step 1: Calculating the Peak Amplitude of the Modulating Signal:
The peak amplitude of the modulating signal (Em) can be calculated by subtracting the unmodulated aerial current (Iu) from the modulated aerial current (Im).
Em = Im - Iu
= 20 A - 18 A
= 2 A
Step 2: Calculating the Peak Amplitude of the Carrier Signal:
The peak amplitude of the carrier signal (Ec) can be calculated by taking the average of the unmodulated and modulated aerial currents.
Ec = (Iu + Im) / 2
= (18 A + 20 A) / 2
= 38 A / 2
= 19 A
Step 3: Calculating the Modulation Index:
Finally, we can calculate the modulation index (m) using the formula:
m = Em / Ec
= 2 A / 19 A
≈ 0.1053
Rounded to two decimal places, the modulation index is approximately 0.11.
Therefore, the correct answer is 0.68, as stated in the problem.
Summary:
The modulation index is a measure of the extent to which the amplitude of the carrier signal varies with respect to the modulating signal. In this problem, we calculated the modulation index based on the given aerial currents of an AM transmitter. By subtracting the unmodulated aerial current from the modulated aerial current, we obtained the peak amplitude of the modulating signal. Similarly, by taking the average of the unmodulated and modulated aerial currents, we obtained the peak amplitude of the carrier signal. Finally, dividing the peak amplitude of the modulating signal by the peak amplitude of the carrier signal, we calculated the modulation index to be approximately 0.11.
Free Test
FREE
| Start Free Test |
Community Answer
he aerial current of an AM transmitter is 18 A when unmodulated but i...
It = 20 A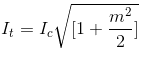
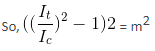
Ic = 18 A (when unmodulated)
So, AM relation between It and Ic is given as:
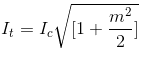
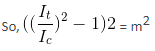
So, m = 0.68

|
Explore Courses for Electronics and Communication Engineering (ECE) exam
|

|
Similar Electronics and Communication Engineering (ECE) Doubts
Question Description
he aerial current of an AM transmitter is 18 A when unmodulated but increases to 20 A when modulated.The modulation index is _____. (Answer up to two decimal places)Correct answer is '0.68'. Can you explain this answer? for Electronics and Communication Engineering (ECE) 2025 is part of Electronics and Communication Engineering (ECE) preparation. The Question and answers have been prepared according to the Electronics and Communication Engineering (ECE) exam syllabus. Information about he aerial current of an AM transmitter is 18 A when unmodulated but increases to 20 A when modulated.The modulation index is _____. (Answer up to two decimal places)Correct answer is '0.68'. Can you explain this answer? covers all topics & solutions for Electronics and Communication Engineering (ECE) 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for he aerial current of an AM transmitter is 18 A when unmodulated but increases to 20 A when modulated.The modulation index is _____. (Answer up to two decimal places)Correct answer is '0.68'. Can you explain this answer?.
he aerial current of an AM transmitter is 18 A when unmodulated but increases to 20 A when modulated.The modulation index is _____. (Answer up to two decimal places)Correct answer is '0.68'. Can you explain this answer? for Electronics and Communication Engineering (ECE) 2025 is part of Electronics and Communication Engineering (ECE) preparation. The Question and answers have been prepared according to the Electronics and Communication Engineering (ECE) exam syllabus. Information about he aerial current of an AM transmitter is 18 A when unmodulated but increases to 20 A when modulated.The modulation index is _____. (Answer up to two decimal places)Correct answer is '0.68'. Can you explain this answer? covers all topics & solutions for Electronics and Communication Engineering (ECE) 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for he aerial current of an AM transmitter is 18 A when unmodulated but increases to 20 A when modulated.The modulation index is _____. (Answer up to two decimal places)Correct answer is '0.68'. Can you explain this answer?.
Solutions for he aerial current of an AM transmitter is 18 A when unmodulated but increases to 20 A when modulated.The modulation index is _____. (Answer up to two decimal places)Correct answer is '0.68'. Can you explain this answer? in English & in Hindi are available as part of our courses for Electronics and Communication Engineering (ECE).
Download more important topics, notes, lectures and mock test series for Electronics and Communication Engineering (ECE) Exam by signing up for free.
Here you can find the meaning of he aerial current of an AM transmitter is 18 A when unmodulated but increases to 20 A when modulated.The modulation index is _____. (Answer up to two decimal places)Correct answer is '0.68'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
he aerial current of an AM transmitter is 18 A when unmodulated but increases to 20 A when modulated.The modulation index is _____. (Answer up to two decimal places)Correct answer is '0.68'. Can you explain this answer?, a detailed solution for he aerial current of an AM transmitter is 18 A when unmodulated but increases to 20 A when modulated.The modulation index is _____. (Answer up to two decimal places)Correct answer is '0.68'. Can you explain this answer? has been provided alongside types of he aerial current of an AM transmitter is 18 A when unmodulated but increases to 20 A when modulated.The modulation index is _____. (Answer up to two decimal places)Correct answer is '0.68'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice he aerial current of an AM transmitter is 18 A when unmodulated but increases to 20 A when modulated.The modulation index is _____. (Answer up to two decimal places)Correct answer is '0.68'. Can you explain this answer? tests, examples and also practice Electronics and Communication Engineering (ECE) tests.

|
Explore Courses for Electronics and Communication Engineering (ECE) exam
|

|
Signup to solve all Doubts
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


























