Civil Engineering (CE) Exam > Civil Engineering (CE) Questions > The shapes of the bending moment diagram for ...
Start Learning for Free
The shapes of the bending moment diagram for a uniform cantilever beam carrying a uniformly distributed load over its length is
- a)A straight line
- b)An ellipse
- c)A hyperbola
- d)A parabola
Correct answer is option 'D'. Can you explain this answer?
Most Upvoted Answer
The shapes of the bending moment diagram for a uniform cantilever beam...
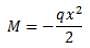
is the equation of parabola.

Free Test
FREE
| Start Free Test |
Community Answer
The shapes of the bending moment diagram for a uniform cantilever beam...
Shape of Bending Moment Diagram for a Uniform Cantilever Beam carrying a Uniformly Distributed Load
The bending moment diagram for a beam represents how the bending moment varies along the length of the beam. In the case of a uniform cantilever beam carrying a uniformly distributed load over its length, the shape of the bending moment diagram is a parabola.
Explanation:
- A cantilever beam is a type of beam that is fixed at one end and free at the other end. It is supported only at one point, and the load is applied at the free end.
- When a uniform load is applied to a cantilever beam, it means that the load is distributed evenly over the entire length of the beam.
- The bending moment at any point along the beam is given by the equation M = w*x^2/2, where M is the bending moment, w is the uniformly distributed load per unit length, and x is the distance from the fixed end.
- As we can see from the equation, the bending moment is directly proportional to the square of the distance from the fixed end. This means that the bending moment increases quadratically as we move away from the fixed end.
- Therefore, the shape of the bending moment diagram for a uniform cantilever beam carrying a uniformly distributed load is a parabola.
- The vertex of the parabola represents the maximum bending moment, which occurs at the fixed end of the beam.
- The bending moment gradually decreases as we move towards the free end of the beam, resulting in a downward-sloping parabolic shape.
Conclusion:
In conclusion, the shape of the bending moment diagram for a uniform cantilever beam carrying a uniformly distributed load over its length is a parabola. This is because the bending moment varies quadratically with the distance from the fixed end of the beam. Understanding the shape of the bending moment diagram is important in structural analysis as it helps engineers design beams and determine the maximum bending moment and shear forces acting on the beam.
The bending moment diagram for a beam represents how the bending moment varies along the length of the beam. In the case of a uniform cantilever beam carrying a uniformly distributed load over its length, the shape of the bending moment diagram is a parabola.
Explanation:
- A cantilever beam is a type of beam that is fixed at one end and free at the other end. It is supported only at one point, and the load is applied at the free end.
- When a uniform load is applied to a cantilever beam, it means that the load is distributed evenly over the entire length of the beam.
- The bending moment at any point along the beam is given by the equation M = w*x^2/2, where M is the bending moment, w is the uniformly distributed load per unit length, and x is the distance from the fixed end.
- As we can see from the equation, the bending moment is directly proportional to the square of the distance from the fixed end. This means that the bending moment increases quadratically as we move away from the fixed end.
- Therefore, the shape of the bending moment diagram for a uniform cantilever beam carrying a uniformly distributed load is a parabola.
- The vertex of the parabola represents the maximum bending moment, which occurs at the fixed end of the beam.
- The bending moment gradually decreases as we move towards the free end of the beam, resulting in a downward-sloping parabolic shape.
Conclusion:
In conclusion, the shape of the bending moment diagram for a uniform cantilever beam carrying a uniformly distributed load over its length is a parabola. This is because the bending moment varies quadratically with the distance from the fixed end of the beam. Understanding the shape of the bending moment diagram is important in structural analysis as it helps engineers design beams and determine the maximum bending moment and shear forces acting on the beam.

|
Explore Courses for Civil Engineering (CE) exam
|

|
Similar Civil Engineering (CE) Doubts
The shapes of the bending moment diagram for a uniform cantilever beam carrying a uniformly distributed load over its length isa)A straight lineb)An ellipsec)A hyperbolad)A parabolaCorrect answer is option 'D'. Can you explain this answer?
Question Description
The shapes of the bending moment diagram for a uniform cantilever beam carrying a uniformly distributed load over its length isa)A straight lineb)An ellipsec)A hyperbolad)A parabolaCorrect answer is option 'D'. Can you explain this answer? for Civil Engineering (CE) 2025 is part of Civil Engineering (CE) preparation. The Question and answers have been prepared according to the Civil Engineering (CE) exam syllabus. Information about The shapes of the bending moment diagram for a uniform cantilever beam carrying a uniformly distributed load over its length isa)A straight lineb)An ellipsec)A hyperbolad)A parabolaCorrect answer is option 'D'. Can you explain this answer? covers all topics & solutions for Civil Engineering (CE) 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The shapes of the bending moment diagram for a uniform cantilever beam carrying a uniformly distributed load over its length isa)A straight lineb)An ellipsec)A hyperbolad)A parabolaCorrect answer is option 'D'. Can you explain this answer?.
The shapes of the bending moment diagram for a uniform cantilever beam carrying a uniformly distributed load over its length isa)A straight lineb)An ellipsec)A hyperbolad)A parabolaCorrect answer is option 'D'. Can you explain this answer? for Civil Engineering (CE) 2025 is part of Civil Engineering (CE) preparation. The Question and answers have been prepared according to the Civil Engineering (CE) exam syllabus. Information about The shapes of the bending moment diagram for a uniform cantilever beam carrying a uniformly distributed load over its length isa)A straight lineb)An ellipsec)A hyperbolad)A parabolaCorrect answer is option 'D'. Can you explain this answer? covers all topics & solutions for Civil Engineering (CE) 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The shapes of the bending moment diagram for a uniform cantilever beam carrying a uniformly distributed load over its length isa)A straight lineb)An ellipsec)A hyperbolad)A parabolaCorrect answer is option 'D'. Can you explain this answer?.
Solutions for The shapes of the bending moment diagram for a uniform cantilever beam carrying a uniformly distributed load over its length isa)A straight lineb)An ellipsec)A hyperbolad)A parabolaCorrect answer is option 'D'. Can you explain this answer? in English & in Hindi are available as part of our courses for Civil Engineering (CE).
Download more important topics, notes, lectures and mock test series for Civil Engineering (CE) Exam by signing up for free.
Here you can find the meaning of The shapes of the bending moment diagram for a uniform cantilever beam carrying a uniformly distributed load over its length isa)A straight lineb)An ellipsec)A hyperbolad)A parabolaCorrect answer is option 'D'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
The shapes of the bending moment diagram for a uniform cantilever beam carrying a uniformly distributed load over its length isa)A straight lineb)An ellipsec)A hyperbolad)A parabolaCorrect answer is option 'D'. Can you explain this answer?, a detailed solution for The shapes of the bending moment diagram for a uniform cantilever beam carrying a uniformly distributed load over its length isa)A straight lineb)An ellipsec)A hyperbolad)A parabolaCorrect answer is option 'D'. Can you explain this answer? has been provided alongside types of The shapes of the bending moment diagram for a uniform cantilever beam carrying a uniformly distributed load over its length isa)A straight lineb)An ellipsec)A hyperbolad)A parabolaCorrect answer is option 'D'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice The shapes of the bending moment diagram for a uniform cantilever beam carrying a uniformly distributed load over its length isa)A straight lineb)An ellipsec)A hyperbolad)A parabolaCorrect answer is option 'D'. Can you explain this answer? tests, examples and also practice Civil Engineering (CE) tests.

|
Explore Courses for Civil Engineering (CE) exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


























