JEE Exam > JEE Questions > In an LCR series circuit, an inductor 30 mH a...
Start Learning for Free
In an LCR series circuit, an inductor 30 mH and a resistor 1 Ω are connected to an AC source of angular frequency 300 rad/s. The value of capacitance for which the current leads the voltage by 45° is 1/x × 10-3 F. Then the value of x is
Correct answer is '3'. Can you explain this answer?
Most Upvoted Answer
In an LCR series circuit, an inductor 30 mH and a resistor 1 Ωar...
In an LCR series circuit, an inductor 30 mH and a resistor 1 Ω are connected in series with a capacitor. The circuit is powered by an AC voltage source.
The behavior of the circuit depends on the frequency of the AC voltage source. At low frequencies, the inductor dominates and the circuit behaves as an inductive circuit. At high frequencies, the capacitor dominates and the circuit behaves as a capacitive circuit.
At the resonant frequency, the inductive and capacitive reactances cancel each other out, resulting in a purely resistive circuit. This is the frequency at which the circuit has the maximum impedance.
The resonant frequency can be calculated using the formula:
fr = 1 / (2π√(LC))
Where fr is the resonant frequency, L is the inductance in henries, and C is the capacitance in farads.
In this circuit, the inductance is given as 30 mH, which is equal to 0.03 H. Let's assume the capacitance is C farads.
Using the formula, we can calculate the resonant frequency:
fr = 1 / (2π√(0.03C))
At the resonant frequency, the inductive and capacitive reactances are equal in magnitude but opposite in sign. Therefore, the impedance of the circuit is purely resistive and can be calculated using Ohm's Law:
Z = √(R^2 + (XL - XC)^2)
Where Z is the impedance, R is the resistance in ohms, XL is the inductive reactance in ohms, and XC is the capacitive reactance in ohms.
Since the resistance is given as 1 Ω, XL can be calculated using the formula:
XL = 2πfL
Where f is the frequency in hertz.
Similarly, XC can be calculated using the formula:
XC = 1 / (2πfC)
At the resonant frequency, XL and XC are equal in magnitude but opposite in sign, resulting in a purely resistive impedance.
The behavior of the circuit depends on the frequency of the AC voltage source. At low frequencies, the inductor dominates and the circuit behaves as an inductive circuit. At high frequencies, the capacitor dominates and the circuit behaves as a capacitive circuit.
At the resonant frequency, the inductive and capacitive reactances cancel each other out, resulting in a purely resistive circuit. This is the frequency at which the circuit has the maximum impedance.
The resonant frequency can be calculated using the formula:
fr = 1 / (2π√(LC))
Where fr is the resonant frequency, L is the inductance in henries, and C is the capacitance in farads.
In this circuit, the inductance is given as 30 mH, which is equal to 0.03 H. Let's assume the capacitance is C farads.
Using the formula, we can calculate the resonant frequency:
fr = 1 / (2π√(0.03C))
At the resonant frequency, the inductive and capacitive reactances are equal in magnitude but opposite in sign. Therefore, the impedance of the circuit is purely resistive and can be calculated using Ohm's Law:
Z = √(R^2 + (XL - XC)^2)
Where Z is the impedance, R is the resistance in ohms, XL is the inductive reactance in ohms, and XC is the capacitive reactance in ohms.
Since the resistance is given as 1 Ω, XL can be calculated using the formula:
XL = 2πfL
Where f is the frequency in hertz.
Similarly, XC can be calculated using the formula:
XC = 1 / (2πfC)
At the resonant frequency, XL and XC are equal in magnitude but opposite in sign, resulting in a purely resistive impedance.
Free Test
FREE
| Start Free Test |
Community Answer
In an LCR series circuit, an inductor 30 mH and a resistor 1 Ωar...
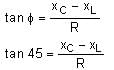
XC - XL = R
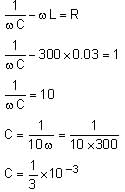
X = 3
|
Explore Courses for JEE exam
|
|
Similar JEE Doubts
Top Courses for JEEView all
In an LCR series circuit, an inductor 30 mH and a resistor 1 Ωare connected to an AC source of angular frequency 300 rad/s. The value of capacitance for which the current leads the voltage by 45° is 1/x × 10-3 F. Then the value of x isCorrect answer is '3'. Can you explain this answer?
Question Description
In an LCR series circuit, an inductor 30 mH and a resistor 1 Ωare connected to an AC source of angular frequency 300 rad/s. The value of capacitance for which the current leads the voltage by 45° is 1/x × 10-3 F. Then the value of x isCorrect answer is '3'. Can you explain this answer? for JEE 2025 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about In an LCR series circuit, an inductor 30 mH and a resistor 1 Ωare connected to an AC source of angular frequency 300 rad/s. The value of capacitance for which the current leads the voltage by 45° is 1/x × 10-3 F. Then the value of x isCorrect answer is '3'. Can you explain this answer? covers all topics & solutions for JEE 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for In an LCR series circuit, an inductor 30 mH and a resistor 1 Ωare connected to an AC source of angular frequency 300 rad/s. The value of capacitance for which the current leads the voltage by 45° is 1/x × 10-3 F. Then the value of x isCorrect answer is '3'. Can you explain this answer?.
In an LCR series circuit, an inductor 30 mH and a resistor 1 Ωare connected to an AC source of angular frequency 300 rad/s. The value of capacitance for which the current leads the voltage by 45° is 1/x × 10-3 F. Then the value of x isCorrect answer is '3'. Can you explain this answer? for JEE 2025 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about In an LCR series circuit, an inductor 30 mH and a resistor 1 Ωare connected to an AC source of angular frequency 300 rad/s. The value of capacitance for which the current leads the voltage by 45° is 1/x × 10-3 F. Then the value of x isCorrect answer is '3'. Can you explain this answer? covers all topics & solutions for JEE 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for In an LCR series circuit, an inductor 30 mH and a resistor 1 Ωare connected to an AC source of angular frequency 300 rad/s. The value of capacitance for which the current leads the voltage by 45° is 1/x × 10-3 F. Then the value of x isCorrect answer is '3'. Can you explain this answer?.
Solutions for In an LCR series circuit, an inductor 30 mH and a resistor 1 Ωare connected to an AC source of angular frequency 300 rad/s. The value of capacitance for which the current leads the voltage by 45° is 1/x × 10-3 F. Then the value of x isCorrect answer is '3'. Can you explain this answer? in English & in Hindi are available as part of our courses for JEE.
Download more important topics, notes, lectures and mock test series for JEE Exam by signing up for free.
Here you can find the meaning of In an LCR series circuit, an inductor 30 mH and a resistor 1 Ωare connected to an AC source of angular frequency 300 rad/s. The value of capacitance for which the current leads the voltage by 45° is 1/x × 10-3 F. Then the value of x isCorrect answer is '3'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
In an LCR series circuit, an inductor 30 mH and a resistor 1 Ωare connected to an AC source of angular frequency 300 rad/s. The value of capacitance for which the current leads the voltage by 45° is 1/x × 10-3 F. Then the value of x isCorrect answer is '3'. Can you explain this answer?, a detailed solution for In an LCR series circuit, an inductor 30 mH and a resistor 1 Ωare connected to an AC source of angular frequency 300 rad/s. The value of capacitance for which the current leads the voltage by 45° is 1/x × 10-3 F. Then the value of x isCorrect answer is '3'. Can you explain this answer? has been provided alongside types of In an LCR series circuit, an inductor 30 mH and a resistor 1 Ωare connected to an AC source of angular frequency 300 rad/s. The value of capacitance for which the current leads the voltage by 45° is 1/x × 10-3 F. Then the value of x isCorrect answer is '3'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice In an LCR series circuit, an inductor 30 mH and a resistor 1 Ωare connected to an AC source of angular frequency 300 rad/s. The value of capacitance for which the current leads the voltage by 45° is 1/x × 10-3 F. Then the value of x isCorrect answer is '3'. Can you explain this answer? tests, examples and also practice JEE tests.
|
|
Explore Courses for JEE exam
|
|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup