The chainages (m) and corresponding perpendicular offsets(m) taken from a chain line to an irregular boundary were (0, 0), (30, 2.65), (60, 3.80), (90, 3.75), (120, 4.65), (150, 3.60), (180, 5.00) and (210, 5.80). The area (m2) between the chain line and the irregular boundary by Simpsons rule isa)681b)767.825c)781d)790.5Correct answer is option 'C'. Can you explain this answer?

Question

Answer

Given data:
Chainages (m) and corresponding perpendicular offsets (m) taken from a chain line to an irregular boundary are as follows:

(0, 0), (30, 2.65), (60, 3.80), (90, 3.75), (120, 4.65), (150, 3.60), (180, 5.00), and (210, 5.80)

To find:
The area (m²) between the chain line and the irregular boundary using Simpson's rule.

Explanation:
Simpson's rule is a numerical integration method used to approximate the area under a curve. It provides a better approximation compared to other methods like the trapezoidal rule when the curve is not linear.

The formula for Simpson's rule is:
Area = (h/3) * [y0 + 4y1 + 2y2 + 4y3 + 2y4 + ... + 2yn-2 + 4yn-1 + yn]

Where:
h = step size (difference between consecutive chainages)
y0, y1, y2, ..., yn = perpendicular offsets at respective chainages

Step 1: Calculate the step size (h)
h = (210 - 0) / (n-1)
= 210 / (8-1)
= 210 / 7
= 30

Step 2: Calculate the sum of perpendicular offsets based on the given data:
Sum of even offsets = y2 + y4 + y6
= 3.80 + 4.65 + 5.00
= 13.45

Sum of odd offsets = y1 + y3 + y5 + y7
= 2.65 + 3.75 + 3.60 + 5.80
= 15.80

Step 3: Calculate the area using Simpson's rule:
Area = (h/3) * [y0 + 4y1 + 2y2 + 4y3 + 2y4 + 4y5 + 2y6 + 4y7 + yn]
= (30/3) * [0 + 4(2.65) + 2(3.80) + 4(3.75) + 2(4.65) + 4(3.60) + 2(5.00) + 4(5.80) + 0]
= 10 * [0 + 10.60 + 7.60 + 15.00 + 9.30 + 14.40 + 10.00 + 23.20 + 0]
= 10 * 90.10
= 901

Step 4: Calculate the adjusted area by multiplying by the step size (h/2):
Adjusted area = Area * (h/2)
= 901 * (30/2)
= 901 * 15
= 13515

Step 5: Round the final result to the nearest whole number:
Final area = 13515 (rounded to the nearest whole number)
=

Answer

Simpson's Rule:
In this rule, the boundaries between the ends of ordinates are assumed to form an arc of a parabola. Hence, Simpson's rule is sometimes called the parabolic rule.
The rule may be stated as follows,
To the sum of the first and the last ordinate, four times the sum of even ordinates and twice the sum of the remaining odd ordinates are added. This total sum is multiplied by the common distance. One-third of this product is the required area.
The area is given by Simpson's rule:

where O₁, O₂, O₃, .........O_n is the offset
Limitation:
This rule is applicable only when the number of divisions is even, i.e., the number of ordinates is odd.
Calculation

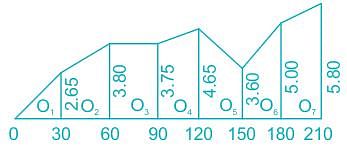
Simpson's Rule :- If this rule is to be applied, the number of ordinates must be odd. But here the number of ordinate is even (eight)
so, Simpson's rule is applied from 0₁ to 0₇ and the area between 0₇ and 0₈ is found out by trapezoidal rule.

A₁ = 10 × (5 + 40 + 16.9)
A₁ = 619 m²
A₂= 30/2(5.00+5.80)
A₂ = 162 m²
∴ Total area = A₁ + A₂
= 619 + 162 = 781 m²

Similar Civil Engineering (CE) Doubts

The value of abscissa (x) and ordinate (y) of curve are as follows:By Simpsons 1/3rd rule, the area under the curve (round off to two decimal places) is _______ Correct answer is '20.67'. Can you explain this answer?

Top Courses for Civil Engineering (CE)View all

General Aptitude for GATE

GATE Civil Engineering (CE) 2025 Mock Test Series

Fluid Mechanics for Civil Engineering

General Awareness & Knowledge

Topicwise Question Bank for Civil Engineering

Top Courses for Civil Engineering (CE)

Top Courses for Civil Engineering (CE)

Suggested Free Tests

Related Content

Mock Test Series

Subject-wise Courses

Subject-wise Courses

Other Engg Branches

Company