SAT Exam > SAT Questions > Question based on the following passage and s...
Start Learning for Free
Question based on the following passage and supplementary material.
This passage is adapted from Brian Greene, “How the Higgs Boson Was Found.” ©2013 by Smithsonian Institution. The Higgs boson is an elementary particle associated with the Higgs field. Experiments conducted in 2012–2013 tentatively confirmed the existence of the Higgs boson and thus of the Higgs field.
This passage is adapted from Brian Greene, “How the Higgs Boson Was Found.” ©2013 by Smithsonian Institution. The Higgs boson is an elementary particle associated with the Higgs field. Experiments conducted in 2012–2013 tentatively confirmed the existence of the Higgs boson and thus of the Higgs field.
Nearly a half-century ago, Peter Higgs and a
handful of other physicists were trying to understand
the origin of a basic physical feature: mass. You can
think of mass as an object’s heft or, a little more
(5) precisely, as the resistance it offers to having its
motion changed. Push on a freight train (or a
feather) to increase its speed, and the resistance you
feel reflects its mass. At a microscopic level, the
freight train’s mass comes from its constituent
(10) molecules and atoms, which are themselves built
from fundamental particles, electrons and quarks.
But where do the masses of these and other
fundamental particles come from?
When physicists in the 1960s modeled the
(15) behavior of these particles using equations rooted in
quantum physics, they encountered a puzzle. If they
imagined that the particles were all massless, then
each term in the equations clicked into a perfectly
symmetric pattern, like the tips of a perfect
(20) snowflake. And this symmetry was not just
mathematically elegant. It explained patterns evident
in the experimental data. But—and here’s the
puzzle—physicists knew that the particles did have
mass, and when they modified the equations to
(25) account for this fact, the mathematical harmony was
spoiled. The equations became complex and
unwieldy and, worse still, inconsistent.
What to do? Here’s the idea put forward by Higgs.
Don’t shove the particles’ masses down the throat of
(30) the beautiful equations. Instead, keep the equations
pristine and symmetric, but consider them operating
within a peculiar environment. Imagine that all of
space is uniformly filled with an invisible
substance—now called the Higgs field—that exerts a
(35) drag force on particles when they accelerate through
it. Push on a fundamental particle in an effort to
increase its speed and, according to Higgs, you would
feel this drag force as a resistance. Justifiably, you
would interpret the resistance as the particle’s mass.
(40) For a mental toehold, think of a ping-pong ball
submerged in water. When you push on the
ping-pong ball, it will feel much more massive than it
does outside of water. Its interaction with the watery
environment has the effect of endowing it with mass.
(45) So with particles submerged in the Higgs field.
In 1964, Higgs submitted a paper to a prominent
physics journal in which he formulated this idea
mathematically. The paper was rejected. Not because
it contained a technical error, but because the
(50) premise of an invisible something permeating space,
interacting with particles to provide their mass, well,
it all just seemed like heaps of overwrought
speculation. The editors of the journal deemed it “of
no obvious relevance to physics.”
(55) But Higgs persevered (and his revised paper
appeared later that year in another journal), and
physicists who took the time to study the proposal
gradually realized that his idea was a stroke of genius,
one that allowed them to have their cake and eat it
(60) too. In Higgs’s scheme, the fundamental equations
can retain their pristine form because the dirty work
of providing the particles’ masses is relegated to the
environment.
While I wasn’t around to witness the initial
(65) rejection of Higgs’s proposal in 1964 (well, I was
around, but only barely), I can attest that by the
mid-1980s, the assessment had changed. The physics
community had, for the most part, fully bought into
the idea that there was a Higgs field permeating
(70) space. In fact, in a graduate course I took that
covered what’s known as the Standard Model of
Particle Physics (the quantum equations physicists
have assembled to describe the particles of matter
and the dominant forces by which they influence
(75) each other), the professor presented the Higgs field
with such certainty that for a long while I had no idea
it had yet to be established experimentally.
On occasion, that happens in physics. Mathematical
equations can sometimes tell such a convincing tale,
(80) they can seemingly radiate reality so strongly, that
they become entrenched in the vernacular of
working physicists, even before there’s data to
confirm them.
handful of other physicists were trying to understand
the origin of a basic physical feature: mass. You can
think of mass as an object’s heft or, a little more
(5) precisely, as the resistance it offers to having its
motion changed. Push on a freight train (or a
feather) to increase its speed, and the resistance you
feel reflects its mass. At a microscopic level, the
freight train’s mass comes from its constituent
(10) molecules and atoms, which are themselves built
from fundamental particles, electrons and quarks.
But where do the masses of these and other
fundamental particles come from?
When physicists in the 1960s modeled the
(15) behavior of these particles using equations rooted in
quantum physics, they encountered a puzzle. If they
imagined that the particles were all massless, then
each term in the equations clicked into a perfectly
symmetric pattern, like the tips of a perfect
(20) snowflake. And this symmetry was not just
mathematically elegant. It explained patterns evident
in the experimental data. But—and here’s the
puzzle—physicists knew that the particles did have
mass, and when they modified the equations to
(25) account for this fact, the mathematical harmony was
spoiled. The equations became complex and
unwieldy and, worse still, inconsistent.
What to do? Here’s the idea put forward by Higgs.
Don’t shove the particles’ masses down the throat of
(30) the beautiful equations. Instead, keep the equations
pristine and symmetric, but consider them operating
within a peculiar environment. Imagine that all of
space is uniformly filled with an invisible
substance—now called the Higgs field—that exerts a
(35) drag force on particles when they accelerate through
it. Push on a fundamental particle in an effort to
increase its speed and, according to Higgs, you would
feel this drag force as a resistance. Justifiably, you
would interpret the resistance as the particle’s mass.
(40) For a mental toehold, think of a ping-pong ball
submerged in water. When you push on the
ping-pong ball, it will feel much more massive than it
does outside of water. Its interaction with the watery
environment has the effect of endowing it with mass.
(45) So with particles submerged in the Higgs field.
In 1964, Higgs submitted a paper to a prominent
physics journal in which he formulated this idea
mathematically. The paper was rejected. Not because
it contained a technical error, but because the
(50) premise of an invisible something permeating space,
interacting with particles to provide their mass, well,
it all just seemed like heaps of overwrought
speculation. The editors of the journal deemed it “of
no obvious relevance to physics.”
(55) But Higgs persevered (and his revised paper
appeared later that year in another journal), and
physicists who took the time to study the proposal
gradually realized that his idea was a stroke of genius,
one that allowed them to have their cake and eat it
(60) too. In Higgs’s scheme, the fundamental equations
can retain their pristine form because the dirty work
of providing the particles’ masses is relegated to the
environment.
While I wasn’t around to witness the initial
(65) rejection of Higgs’s proposal in 1964 (well, I was
around, but only barely), I can attest that by the
mid-1980s, the assessment had changed. The physics
community had, for the most part, fully bought into
the idea that there was a Higgs field permeating
(70) space. In fact, in a graduate course I took that
covered what’s known as the Standard Model of
Particle Physics (the quantum equations physicists
have assembled to describe the particles of matter
and the dominant forces by which they influence
(75) each other), the professor presented the Higgs field
with such certainty that for a long while I had no idea
it had yet to be established experimentally.
On occasion, that happens in physics. Mathematical
equations can sometimes tell such a convincing tale,
(80) they can seemingly radiate reality so strongly, that
they become entrenched in the vernacular of
working physicists, even before there’s data to
confirm them.
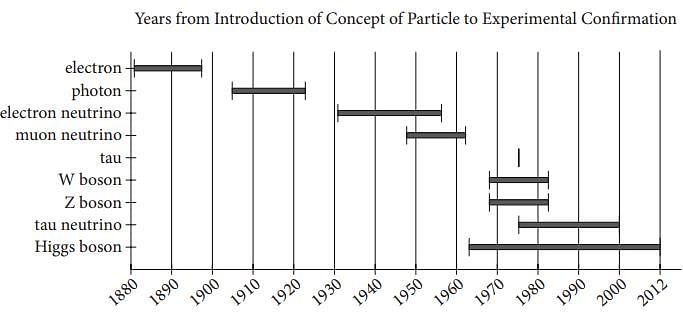
Q. Which statement is best supported by the data presented in the graph?
- a)The W boson and the Z boson were proposed and experimentally confirmed at about the same time.
- b)The Higgs boson was experimentally confirmed more quickly than were most other particles.
- c)The tau neutrino was experimentally confirmed at about the same time as the tau.
- d)The muon neutrino took longer to experimentally confirm than did the electron neutrino.
Correct answer is option 'A'. Can you explain this answer?
Most Upvoted Answer
Question based on the following passage and supplementary material.Thi...
Choice A is the best answer. Both the W boson and Z boson were introduced in the late 1960s and experimentally confirmed in the early 1980s. It is therefore accurate to say that they were both proposed and proven at about the same time.
Choice B is incorrect because the graph shows that it took more than forty years for the Higgs boson to be experimentally confirmed, while all the other particles were confirmed in a significantly shorter period of time than that. Choice C is incorrect because the graph shows that the tau neutrino was experimentally confirmed in 2000, while tau itself was experimentally confirmed in approximately 1975. Choice D is incorrect because the muon neutrino took approximately fifteen years to be confirmed, while the electron neutrino took well over twenty years.
Choice B is incorrect because the graph shows that it took more than forty years for the Higgs boson to be experimentally confirmed, while all the other particles were confirmed in a significantly shorter period of time than that. Choice C is incorrect because the graph shows that the tau neutrino was experimentally confirmed in 2000, while tau itself was experimentally confirmed in approximately 1975. Choice D is incorrect because the muon neutrino took approximately fifteen years to be confirmed, while the electron neutrino took well over twenty years.

|
Explore Courses for SAT exam
|

|
Question based on the following passage and supplementary material.This passage is adapted from Brian Greene, “How the Higgs Boson Was Found.” ©2013 by Smithsonian Institution. The Higgs boson is an elementary particle associated with the Higgs field. Experiments conducted in 2012–2013 tentatively confirmed the existence of the Higgs boson and thus of the Higgs field.Nearly a half-century ago, Peter Higgs and ahandful of other physicists were trying to understandthe origin of a basic physical feature: mass. You canthink of mass as an object’s heft or, a little more(5) precisely, as the resistance it offers to having itsmotion changed. Push on a freight train (or afeather) to increase its speed, and the resistance youfeel reflects its mass. At a microscopic level, thefreight train’s mass comes from its constituent(10) molecules and atoms, which are themselves builtfrom fundamental particles, electrons and quarks.But where do the masses of these and otherfundamental particles come from?When physicists in the 1960s modeled the(15) behavior of these particles using equations rooted inquantum physics, they encountered a puzzle. If theyimagined that the particles were all massless, theneach term in the equations clicked into a perfectlysymmetric pattern, like the tips of a perfect(20) snowflake. And this symmetry was not justmathematically elegant. It explained patterns evidentin the experimental data. But—and here’s thepuzzle—physicists knew that the particles did havemass, and when they modified the equations to(25) account for this fact, the mathematical harmony wasspoiled. The equations became complex andunwieldy and, worse still, inconsistent.What to do? Here’s the idea put forward by Higgs.Don’t shove the particles’ masses down the throat of(30) the beautiful equations. Instead, keep the equationspristine and symmetric, but consider them operatingwithin a peculiar environment. Imagine that all ofspace is uniformly filled with an invisiblesubstance—now called the Higgs field—that exerts a(35) drag force on particles when they accelerate throughit. Push on a fundamental particle in an effort toincrease its speed and, according to Higgs, you wouldfeel this drag force as a resistance. Justifiably, youwould interpret the resistance as the particle’s mass.(40) For a mental toehold, think of a ping-pong ballsubmerged in water. When you push on theping-pong ball, it will feel much more massive than itdoes outside of water. Its interaction with the wateryenvironment has the effect of endowing it with mass.(45) So with particles submerged in the Higgs field.In 1964, Higgs submitted a paper to a prominentphysics journal in which he formulated this ideamathematically. The paper was rejected. Not becauseit contained a technical error, but because the(50) premise of an invisible something permeating space,interacting with particles to provide their mass, well,it all just seemed like heaps of overwroughtspeculation. The editors of the journal deemed it “ofno obvious relevance to physics.”(55) But Higgs persevered (and his revised paperappeared later that year in another journal), andphysicists who took the time to study the proposalgradually realized that his idea was a stroke of genius,one that allowed them to have their cake and eat it(60) too. In Higgs’s scheme, the fundamental equationscan retain their pristine form because the dirty workof providing the particles’ masses is relegated to theenvironment.While I wasn’t around to witness the initial(65) rejection of Higgs’s proposal in 1964 (well, I wasaround, but only barely), I can attest that by themid-1980s, the assessment had changed. The physicscommunity had, for the most part, fully bought intothe idea that there was a Higgs field permeating(70) space. In fact, in a graduate course I took thatcovered what’s known as the Standard Model ofParticle Physics (the quantum equations physicistshave assembled to describe the particles of matterand the dominant forces by which they influence(75) each other), the professor presented the Higgs fieldwith such certainty that for a long while I had no ideait had yet to be established experimentally.On occasion, that happens in physics. Mathematicalequations can sometimes tell such a convincing tale,(80) they can seemingly radiate reality so strongly, thatthey become entrenched in the vernacular ofworking physicists, even before there’s data toconfirm them.Q.Which statement is best supported by the data presented in the graph?a)The W boson and the Z boson were proposed and experimentally confirmed at about the same time.b)The Higgs boson was experimentally confirmed more quickly than were most other particles.c)The tau neutrino was experimentally confirmed at about the same time as the tau.d)The muon neutrino took longer to experimentally confirm than did the electron neutrino.Correct answer is option 'A'. Can you explain this answer?
Question Description
Question based on the following passage and supplementary material.This passage is adapted from Brian Greene, “How the Higgs Boson Was Found.” ©2013 by Smithsonian Institution. The Higgs boson is an elementary particle associated with the Higgs field. Experiments conducted in 2012–2013 tentatively confirmed the existence of the Higgs boson and thus of the Higgs field.Nearly a half-century ago, Peter Higgs and ahandful of other physicists were trying to understandthe origin of a basic physical feature: mass. You canthink of mass as an object’s heft or, a little more(5) precisely, as the resistance it offers to having itsmotion changed. Push on a freight train (or afeather) to increase its speed, and the resistance youfeel reflects its mass. At a microscopic level, thefreight train’s mass comes from its constituent(10) molecules and atoms, which are themselves builtfrom fundamental particles, electrons and quarks.But where do the masses of these and otherfundamental particles come from?When physicists in the 1960s modeled the(15) behavior of these particles using equations rooted inquantum physics, they encountered a puzzle. If theyimagined that the particles were all massless, theneach term in the equations clicked into a perfectlysymmetric pattern, like the tips of a perfect(20) snowflake. And this symmetry was not justmathematically elegant. It explained patterns evidentin the experimental data. But—and here’s thepuzzle—physicists knew that the particles did havemass, and when they modified the equations to(25) account for this fact, the mathematical harmony wasspoiled. The equations became complex andunwieldy and, worse still, inconsistent.What to do? Here’s the idea put forward by Higgs.Don’t shove the particles’ masses down the throat of(30) the beautiful equations. Instead, keep the equationspristine and symmetric, but consider them operatingwithin a peculiar environment. Imagine that all ofspace is uniformly filled with an invisiblesubstance—now called the Higgs field—that exerts a(35) drag force on particles when they accelerate throughit. Push on a fundamental particle in an effort toincrease its speed and, according to Higgs, you wouldfeel this drag force as a resistance. Justifiably, youwould interpret the resistance as the particle’s mass.(40) For a mental toehold, think of a ping-pong ballsubmerged in water. When you push on theping-pong ball, it will feel much more massive than itdoes outside of water. Its interaction with the wateryenvironment has the effect of endowing it with mass.(45) So with particles submerged in the Higgs field.In 1964, Higgs submitted a paper to a prominentphysics journal in which he formulated this ideamathematically. The paper was rejected. Not becauseit contained a technical error, but because the(50) premise of an invisible something permeating space,interacting with particles to provide their mass, well,it all just seemed like heaps of overwroughtspeculation. The editors of the journal deemed it “ofno obvious relevance to physics.”(55) But Higgs persevered (and his revised paperappeared later that year in another journal), andphysicists who took the time to study the proposalgradually realized that his idea was a stroke of genius,one that allowed them to have their cake and eat it(60) too. In Higgs’s scheme, the fundamental equationscan retain their pristine form because the dirty workof providing the particles’ masses is relegated to theenvironment.While I wasn’t around to witness the initial(65) rejection of Higgs’s proposal in 1964 (well, I wasaround, but only barely), I can attest that by themid-1980s, the assessment had changed. The physicscommunity had, for the most part, fully bought intothe idea that there was a Higgs field permeating(70) space. In fact, in a graduate course I took thatcovered what’s known as the Standard Model ofParticle Physics (the quantum equations physicistshave assembled to describe the particles of matterand the dominant forces by which they influence(75) each other), the professor presented the Higgs fieldwith such certainty that for a long while I had no ideait had yet to be established experimentally.On occasion, that happens in physics. Mathematicalequations can sometimes tell such a convincing tale,(80) they can seemingly radiate reality so strongly, thatthey become entrenched in the vernacular ofworking physicists, even before there’s data toconfirm them.Q.Which statement is best supported by the data presented in the graph?a)The W boson and the Z boson were proposed and experimentally confirmed at about the same time.b)The Higgs boson was experimentally confirmed more quickly than were most other particles.c)The tau neutrino was experimentally confirmed at about the same time as the tau.d)The muon neutrino took longer to experimentally confirm than did the electron neutrino.Correct answer is option 'A'. Can you explain this answer? for SAT 2025 is part of SAT preparation. The Question and answers have been prepared according to the SAT exam syllabus. Information about Question based on the following passage and supplementary material.This passage is adapted from Brian Greene, “How the Higgs Boson Was Found.” ©2013 by Smithsonian Institution. The Higgs boson is an elementary particle associated with the Higgs field. Experiments conducted in 2012–2013 tentatively confirmed the existence of the Higgs boson and thus of the Higgs field.Nearly a half-century ago, Peter Higgs and ahandful of other physicists were trying to understandthe origin of a basic physical feature: mass. You canthink of mass as an object’s heft or, a little more(5) precisely, as the resistance it offers to having itsmotion changed. Push on a freight train (or afeather) to increase its speed, and the resistance youfeel reflects its mass. At a microscopic level, thefreight train’s mass comes from its constituent(10) molecules and atoms, which are themselves builtfrom fundamental particles, electrons and quarks.But where do the masses of these and otherfundamental particles come from?When physicists in the 1960s modeled the(15) behavior of these particles using equations rooted inquantum physics, they encountered a puzzle. If theyimagined that the particles were all massless, theneach term in the equations clicked into a perfectlysymmetric pattern, like the tips of a perfect(20) snowflake. And this symmetry was not justmathematically elegant. It explained patterns evidentin the experimental data. But—and here’s thepuzzle—physicists knew that the particles did havemass, and when they modified the equations to(25) account for this fact, the mathematical harmony wasspoiled. The equations became complex andunwieldy and, worse still, inconsistent.What to do? Here’s the idea put forward by Higgs.Don’t shove the particles’ masses down the throat of(30) the beautiful equations. Instead, keep the equationspristine and symmetric, but consider them operatingwithin a peculiar environment. Imagine that all ofspace is uniformly filled with an invisiblesubstance—now called the Higgs field—that exerts a(35) drag force on particles when they accelerate throughit. Push on a fundamental particle in an effort toincrease its speed and, according to Higgs, you wouldfeel this drag force as a resistance. Justifiably, youwould interpret the resistance as the particle’s mass.(40) For a mental toehold, think of a ping-pong ballsubmerged in water. When you push on theping-pong ball, it will feel much more massive than itdoes outside of water. Its interaction with the wateryenvironment has the effect of endowing it with mass.(45) So with particles submerged in the Higgs field.In 1964, Higgs submitted a paper to a prominentphysics journal in which he formulated this ideamathematically. The paper was rejected. Not becauseit contained a technical error, but because the(50) premise of an invisible something permeating space,interacting with particles to provide their mass, well,it all just seemed like heaps of overwroughtspeculation. The editors of the journal deemed it “ofno obvious relevance to physics.”(55) But Higgs persevered (and his revised paperappeared later that year in another journal), andphysicists who took the time to study the proposalgradually realized that his idea was a stroke of genius,one that allowed them to have their cake and eat it(60) too. In Higgs’s scheme, the fundamental equationscan retain their pristine form because the dirty workof providing the particles’ masses is relegated to theenvironment.While I wasn’t around to witness the initial(65) rejection of Higgs’s proposal in 1964 (well, I wasaround, but only barely), I can attest that by themid-1980s, the assessment had changed. The physicscommunity had, for the most part, fully bought intothe idea that there was a Higgs field permeating(70) space. In fact, in a graduate course I took thatcovered what’s known as the Standard Model ofParticle Physics (the quantum equations physicistshave assembled to describe the particles of matterand the dominant forces by which they influence(75) each other), the professor presented the Higgs fieldwith such certainty that for a long while I had no ideait had yet to be established experimentally.On occasion, that happens in physics. Mathematicalequations can sometimes tell such a convincing tale,(80) they can seemingly radiate reality so strongly, thatthey become entrenched in the vernacular ofworking physicists, even before there’s data toconfirm them.Q.Which statement is best supported by the data presented in the graph?a)The W boson and the Z boson were proposed and experimentally confirmed at about the same time.b)The Higgs boson was experimentally confirmed more quickly than were most other particles.c)The tau neutrino was experimentally confirmed at about the same time as the tau.d)The muon neutrino took longer to experimentally confirm than did the electron neutrino.Correct answer is option 'A'. Can you explain this answer? covers all topics & solutions for SAT 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Question based on the following passage and supplementary material.This passage is adapted from Brian Greene, “How the Higgs Boson Was Found.” ©2013 by Smithsonian Institution. The Higgs boson is an elementary particle associated with the Higgs field. Experiments conducted in 2012–2013 tentatively confirmed the existence of the Higgs boson and thus of the Higgs field.Nearly a half-century ago, Peter Higgs and ahandful of other physicists were trying to understandthe origin of a basic physical feature: mass. You canthink of mass as an object’s heft or, a little more(5) precisely, as the resistance it offers to having itsmotion changed. Push on a freight train (or afeather) to increase its speed, and the resistance youfeel reflects its mass. At a microscopic level, thefreight train’s mass comes from its constituent(10) molecules and atoms, which are themselves builtfrom fundamental particles, electrons and quarks.But where do the masses of these and otherfundamental particles come from?When physicists in the 1960s modeled the(15) behavior of these particles using equations rooted inquantum physics, they encountered a puzzle. If theyimagined that the particles were all massless, theneach term in the equations clicked into a perfectlysymmetric pattern, like the tips of a perfect(20) snowflake. And this symmetry was not justmathematically elegant. It explained patterns evidentin the experimental data. But—and here’s thepuzzle—physicists knew that the particles did havemass, and when they modified the equations to(25) account for this fact, the mathematical harmony wasspoiled. The equations became complex andunwieldy and, worse still, inconsistent.What to do? Here’s the idea put forward by Higgs.Don’t shove the particles’ masses down the throat of(30) the beautiful equations. Instead, keep the equationspristine and symmetric, but consider them operatingwithin a peculiar environment. Imagine that all ofspace is uniformly filled with an invisiblesubstance—now called the Higgs field—that exerts a(35) drag force on particles when they accelerate throughit. Push on a fundamental particle in an effort toincrease its speed and, according to Higgs, you wouldfeel this drag force as a resistance. Justifiably, youwould interpret the resistance as the particle’s mass.(40) For a mental toehold, think of a ping-pong ballsubmerged in water. When you push on theping-pong ball, it will feel much more massive than itdoes outside of water. Its interaction with the wateryenvironment has the effect of endowing it with mass.(45) So with particles submerged in the Higgs field.In 1964, Higgs submitted a paper to a prominentphysics journal in which he formulated this ideamathematically. The paper was rejected. Not becauseit contained a technical error, but because the(50) premise of an invisible something permeating space,interacting with particles to provide their mass, well,it all just seemed like heaps of overwroughtspeculation. The editors of the journal deemed it “ofno obvious relevance to physics.”(55) But Higgs persevered (and his revised paperappeared later that year in another journal), andphysicists who took the time to study the proposalgradually realized that his idea was a stroke of genius,one that allowed them to have their cake and eat it(60) too. In Higgs’s scheme, the fundamental equationscan retain their pristine form because the dirty workof providing the particles’ masses is relegated to theenvironment.While I wasn’t around to witness the initial(65) rejection of Higgs’s proposal in 1964 (well, I wasaround, but only barely), I can attest that by themid-1980s, the assessment had changed. The physicscommunity had, for the most part, fully bought intothe idea that there was a Higgs field permeating(70) space. In fact, in a graduate course I took thatcovered what’s known as the Standard Model ofParticle Physics (the quantum equations physicistshave assembled to describe the particles of matterand the dominant forces by which they influence(75) each other), the professor presented the Higgs fieldwith such certainty that for a long while I had no ideait had yet to be established experimentally.On occasion, that happens in physics. Mathematicalequations can sometimes tell such a convincing tale,(80) they can seemingly radiate reality so strongly, thatthey become entrenched in the vernacular ofworking physicists, even before there’s data toconfirm them.Q.Which statement is best supported by the data presented in the graph?a)The W boson and the Z boson were proposed and experimentally confirmed at about the same time.b)The Higgs boson was experimentally confirmed more quickly than were most other particles.c)The tau neutrino was experimentally confirmed at about the same time as the tau.d)The muon neutrino took longer to experimentally confirm than did the electron neutrino.Correct answer is option 'A'. Can you explain this answer?.
Question based on the following passage and supplementary material.This passage is adapted from Brian Greene, “How the Higgs Boson Was Found.” ©2013 by Smithsonian Institution. The Higgs boson is an elementary particle associated with the Higgs field. Experiments conducted in 2012–2013 tentatively confirmed the existence of the Higgs boson and thus of the Higgs field.Nearly a half-century ago, Peter Higgs and ahandful of other physicists were trying to understandthe origin of a basic physical feature: mass. You canthink of mass as an object’s heft or, a little more(5) precisely, as the resistance it offers to having itsmotion changed. Push on a freight train (or afeather) to increase its speed, and the resistance youfeel reflects its mass. At a microscopic level, thefreight train’s mass comes from its constituent(10) molecules and atoms, which are themselves builtfrom fundamental particles, electrons and quarks.But where do the masses of these and otherfundamental particles come from?When physicists in the 1960s modeled the(15) behavior of these particles using equations rooted inquantum physics, they encountered a puzzle. If theyimagined that the particles were all massless, theneach term in the equations clicked into a perfectlysymmetric pattern, like the tips of a perfect(20) snowflake. And this symmetry was not justmathematically elegant. It explained patterns evidentin the experimental data. But—and here’s thepuzzle—physicists knew that the particles did havemass, and when they modified the equations to(25) account for this fact, the mathematical harmony wasspoiled. The equations became complex andunwieldy and, worse still, inconsistent.What to do? Here’s the idea put forward by Higgs.Don’t shove the particles’ masses down the throat of(30) the beautiful equations. Instead, keep the equationspristine and symmetric, but consider them operatingwithin a peculiar environment. Imagine that all ofspace is uniformly filled with an invisiblesubstance—now called the Higgs field—that exerts a(35) drag force on particles when they accelerate throughit. Push on a fundamental particle in an effort toincrease its speed and, according to Higgs, you wouldfeel this drag force as a resistance. Justifiably, youwould interpret the resistance as the particle’s mass.(40) For a mental toehold, think of a ping-pong ballsubmerged in water. When you push on theping-pong ball, it will feel much more massive than itdoes outside of water. Its interaction with the wateryenvironment has the effect of endowing it with mass.(45) So with particles submerged in the Higgs field.In 1964, Higgs submitted a paper to a prominentphysics journal in which he formulated this ideamathematically. The paper was rejected. Not becauseit contained a technical error, but because the(50) premise of an invisible something permeating space,interacting with particles to provide their mass, well,it all just seemed like heaps of overwroughtspeculation. The editors of the journal deemed it “ofno obvious relevance to physics.”(55) But Higgs persevered (and his revised paperappeared later that year in another journal), andphysicists who took the time to study the proposalgradually realized that his idea was a stroke of genius,one that allowed them to have their cake and eat it(60) too. In Higgs’s scheme, the fundamental equationscan retain their pristine form because the dirty workof providing the particles’ masses is relegated to theenvironment.While I wasn’t around to witness the initial(65) rejection of Higgs’s proposal in 1964 (well, I wasaround, but only barely), I can attest that by themid-1980s, the assessment had changed. The physicscommunity had, for the most part, fully bought intothe idea that there was a Higgs field permeating(70) space. In fact, in a graduate course I took thatcovered what’s known as the Standard Model ofParticle Physics (the quantum equations physicistshave assembled to describe the particles of matterand the dominant forces by which they influence(75) each other), the professor presented the Higgs fieldwith such certainty that for a long while I had no ideait had yet to be established experimentally.On occasion, that happens in physics. Mathematicalequations can sometimes tell such a convincing tale,(80) they can seemingly radiate reality so strongly, thatthey become entrenched in the vernacular ofworking physicists, even before there’s data toconfirm them.Q.Which statement is best supported by the data presented in the graph?a)The W boson and the Z boson were proposed and experimentally confirmed at about the same time.b)The Higgs boson was experimentally confirmed more quickly than were most other particles.c)The tau neutrino was experimentally confirmed at about the same time as the tau.d)The muon neutrino took longer to experimentally confirm than did the electron neutrino.Correct answer is option 'A'. Can you explain this answer? for SAT 2025 is part of SAT preparation. The Question and answers have been prepared according to the SAT exam syllabus. Information about Question based on the following passage and supplementary material.This passage is adapted from Brian Greene, “How the Higgs Boson Was Found.” ©2013 by Smithsonian Institution. The Higgs boson is an elementary particle associated with the Higgs field. Experiments conducted in 2012–2013 tentatively confirmed the existence of the Higgs boson and thus of the Higgs field.Nearly a half-century ago, Peter Higgs and ahandful of other physicists were trying to understandthe origin of a basic physical feature: mass. You canthink of mass as an object’s heft or, a little more(5) precisely, as the resistance it offers to having itsmotion changed. Push on a freight train (or afeather) to increase its speed, and the resistance youfeel reflects its mass. At a microscopic level, thefreight train’s mass comes from its constituent(10) molecules and atoms, which are themselves builtfrom fundamental particles, electrons and quarks.But where do the masses of these and otherfundamental particles come from?When physicists in the 1960s modeled the(15) behavior of these particles using equations rooted inquantum physics, they encountered a puzzle. If theyimagined that the particles were all massless, theneach term in the equations clicked into a perfectlysymmetric pattern, like the tips of a perfect(20) snowflake. And this symmetry was not justmathematically elegant. It explained patterns evidentin the experimental data. But—and here’s thepuzzle—physicists knew that the particles did havemass, and when they modified the equations to(25) account for this fact, the mathematical harmony wasspoiled. The equations became complex andunwieldy and, worse still, inconsistent.What to do? Here’s the idea put forward by Higgs.Don’t shove the particles’ masses down the throat of(30) the beautiful equations. Instead, keep the equationspristine and symmetric, but consider them operatingwithin a peculiar environment. Imagine that all ofspace is uniformly filled with an invisiblesubstance—now called the Higgs field—that exerts a(35) drag force on particles when they accelerate throughit. Push on a fundamental particle in an effort toincrease its speed and, according to Higgs, you wouldfeel this drag force as a resistance. Justifiably, youwould interpret the resistance as the particle’s mass.(40) For a mental toehold, think of a ping-pong ballsubmerged in water. When you push on theping-pong ball, it will feel much more massive than itdoes outside of water. Its interaction with the wateryenvironment has the effect of endowing it with mass.(45) So with particles submerged in the Higgs field.In 1964, Higgs submitted a paper to a prominentphysics journal in which he formulated this ideamathematically. The paper was rejected. Not becauseit contained a technical error, but because the(50) premise of an invisible something permeating space,interacting with particles to provide their mass, well,it all just seemed like heaps of overwroughtspeculation. The editors of the journal deemed it “ofno obvious relevance to physics.”(55) But Higgs persevered (and his revised paperappeared later that year in another journal), andphysicists who took the time to study the proposalgradually realized that his idea was a stroke of genius,one that allowed them to have their cake and eat it(60) too. In Higgs’s scheme, the fundamental equationscan retain their pristine form because the dirty workof providing the particles’ masses is relegated to theenvironment.While I wasn’t around to witness the initial(65) rejection of Higgs’s proposal in 1964 (well, I wasaround, but only barely), I can attest that by themid-1980s, the assessment had changed. The physicscommunity had, for the most part, fully bought intothe idea that there was a Higgs field permeating(70) space. In fact, in a graduate course I took thatcovered what’s known as the Standard Model ofParticle Physics (the quantum equations physicistshave assembled to describe the particles of matterand the dominant forces by which they influence(75) each other), the professor presented the Higgs fieldwith such certainty that for a long while I had no ideait had yet to be established experimentally.On occasion, that happens in physics. Mathematicalequations can sometimes tell such a convincing tale,(80) they can seemingly radiate reality so strongly, thatthey become entrenched in the vernacular ofworking physicists, even before there’s data toconfirm them.Q.Which statement is best supported by the data presented in the graph?a)The W boson and the Z boson were proposed and experimentally confirmed at about the same time.b)The Higgs boson was experimentally confirmed more quickly than were most other particles.c)The tau neutrino was experimentally confirmed at about the same time as the tau.d)The muon neutrino took longer to experimentally confirm than did the electron neutrino.Correct answer is option 'A'. Can you explain this answer? covers all topics & solutions for SAT 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Question based on the following passage and supplementary material.This passage is adapted from Brian Greene, “How the Higgs Boson Was Found.” ©2013 by Smithsonian Institution. The Higgs boson is an elementary particle associated with the Higgs field. Experiments conducted in 2012–2013 tentatively confirmed the existence of the Higgs boson and thus of the Higgs field.Nearly a half-century ago, Peter Higgs and ahandful of other physicists were trying to understandthe origin of a basic physical feature: mass. You canthink of mass as an object’s heft or, a little more(5) precisely, as the resistance it offers to having itsmotion changed. Push on a freight train (or afeather) to increase its speed, and the resistance youfeel reflects its mass. At a microscopic level, thefreight train’s mass comes from its constituent(10) molecules and atoms, which are themselves builtfrom fundamental particles, electrons and quarks.But where do the masses of these and otherfundamental particles come from?When physicists in the 1960s modeled the(15) behavior of these particles using equations rooted inquantum physics, they encountered a puzzle. If theyimagined that the particles were all massless, theneach term in the equations clicked into a perfectlysymmetric pattern, like the tips of a perfect(20) snowflake. And this symmetry was not justmathematically elegant. It explained patterns evidentin the experimental data. But—and here’s thepuzzle—physicists knew that the particles did havemass, and when they modified the equations to(25) account for this fact, the mathematical harmony wasspoiled. The equations became complex andunwieldy and, worse still, inconsistent.What to do? Here’s the idea put forward by Higgs.Don’t shove the particles’ masses down the throat of(30) the beautiful equations. Instead, keep the equationspristine and symmetric, but consider them operatingwithin a peculiar environment. Imagine that all ofspace is uniformly filled with an invisiblesubstance—now called the Higgs field—that exerts a(35) drag force on particles when they accelerate throughit. Push on a fundamental particle in an effort toincrease its speed and, according to Higgs, you wouldfeel this drag force as a resistance. Justifiably, youwould interpret the resistance as the particle’s mass.(40) For a mental toehold, think of a ping-pong ballsubmerged in water. When you push on theping-pong ball, it will feel much more massive than itdoes outside of water. Its interaction with the wateryenvironment has the effect of endowing it with mass.(45) So with particles submerged in the Higgs field.In 1964, Higgs submitted a paper to a prominentphysics journal in which he formulated this ideamathematically. The paper was rejected. Not becauseit contained a technical error, but because the(50) premise of an invisible something permeating space,interacting with particles to provide their mass, well,it all just seemed like heaps of overwroughtspeculation. The editors of the journal deemed it “ofno obvious relevance to physics.”(55) But Higgs persevered (and his revised paperappeared later that year in another journal), andphysicists who took the time to study the proposalgradually realized that his idea was a stroke of genius,one that allowed them to have their cake and eat it(60) too. In Higgs’s scheme, the fundamental equationscan retain their pristine form because the dirty workof providing the particles’ masses is relegated to theenvironment.While I wasn’t around to witness the initial(65) rejection of Higgs’s proposal in 1964 (well, I wasaround, but only barely), I can attest that by themid-1980s, the assessment had changed. The physicscommunity had, for the most part, fully bought intothe idea that there was a Higgs field permeating(70) space. In fact, in a graduate course I took thatcovered what’s known as the Standard Model ofParticle Physics (the quantum equations physicistshave assembled to describe the particles of matterand the dominant forces by which they influence(75) each other), the professor presented the Higgs fieldwith such certainty that for a long while I had no ideait had yet to be established experimentally.On occasion, that happens in physics. Mathematicalequations can sometimes tell such a convincing tale,(80) they can seemingly radiate reality so strongly, thatthey become entrenched in the vernacular ofworking physicists, even before there’s data toconfirm them.Q.Which statement is best supported by the data presented in the graph?a)The W boson and the Z boson were proposed and experimentally confirmed at about the same time.b)The Higgs boson was experimentally confirmed more quickly than were most other particles.c)The tau neutrino was experimentally confirmed at about the same time as the tau.d)The muon neutrino took longer to experimentally confirm than did the electron neutrino.Correct answer is option 'A'. Can you explain this answer?.
Solutions for Question based on the following passage and supplementary material.This passage is adapted from Brian Greene, “How the Higgs Boson Was Found.” ©2013 by Smithsonian Institution. The Higgs boson is an elementary particle associated with the Higgs field. Experiments conducted in 2012–2013 tentatively confirmed the existence of the Higgs boson and thus of the Higgs field.Nearly a half-century ago, Peter Higgs and ahandful of other physicists were trying to understandthe origin of a basic physical feature: mass. You canthink of mass as an object’s heft or, a little more(5) precisely, as the resistance it offers to having itsmotion changed. Push on a freight train (or afeather) to increase its speed, and the resistance youfeel reflects its mass. At a microscopic level, thefreight train’s mass comes from its constituent(10) molecules and atoms, which are themselves builtfrom fundamental particles, electrons and quarks.But where do the masses of these and otherfundamental particles come from?When physicists in the 1960s modeled the(15) behavior of these particles using equations rooted inquantum physics, they encountered a puzzle. If theyimagined that the particles were all massless, theneach term in the equations clicked into a perfectlysymmetric pattern, like the tips of a perfect(20) snowflake. And this symmetry was not justmathematically elegant. It explained patterns evidentin the experimental data. But—and here’s thepuzzle—physicists knew that the particles did havemass, and when they modified the equations to(25) account for this fact, the mathematical harmony wasspoiled. The equations became complex andunwieldy and, worse still, inconsistent.What to do? Here’s the idea put forward by Higgs.Don’t shove the particles’ masses down the throat of(30) the beautiful equations. Instead, keep the equationspristine and symmetric, but consider them operatingwithin a peculiar environment. Imagine that all ofspace is uniformly filled with an invisiblesubstance—now called the Higgs field—that exerts a(35) drag force on particles when they accelerate throughit. Push on a fundamental particle in an effort toincrease its speed and, according to Higgs, you wouldfeel this drag force as a resistance. Justifiably, youwould interpret the resistance as the particle’s mass.(40) For a mental toehold, think of a ping-pong ballsubmerged in water. When you push on theping-pong ball, it will feel much more massive than itdoes outside of water. Its interaction with the wateryenvironment has the effect of endowing it with mass.(45) So with particles submerged in the Higgs field.In 1964, Higgs submitted a paper to a prominentphysics journal in which he formulated this ideamathematically. The paper was rejected. Not becauseit contained a technical error, but because the(50) premise of an invisible something permeating space,interacting with particles to provide their mass, well,it all just seemed like heaps of overwroughtspeculation. The editors of the journal deemed it “ofno obvious relevance to physics.”(55) But Higgs persevered (and his revised paperappeared later that year in another journal), andphysicists who took the time to study the proposalgradually realized that his idea was a stroke of genius,one that allowed them to have their cake and eat it(60) too. In Higgs’s scheme, the fundamental equationscan retain their pristine form because the dirty workof providing the particles’ masses is relegated to theenvironment.While I wasn’t around to witness the initial(65) rejection of Higgs’s proposal in 1964 (well, I wasaround, but only barely), I can attest that by themid-1980s, the assessment had changed. The physicscommunity had, for the most part, fully bought intothe idea that there was a Higgs field permeating(70) space. In fact, in a graduate course I took thatcovered what’s known as the Standard Model ofParticle Physics (the quantum equations physicistshave assembled to describe the particles of matterand the dominant forces by which they influence(75) each other), the professor presented the Higgs fieldwith such certainty that for a long while I had no ideait had yet to be established experimentally.On occasion, that happens in physics. Mathematicalequations can sometimes tell such a convincing tale,(80) they can seemingly radiate reality so strongly, thatthey become entrenched in the vernacular ofworking physicists, even before there’s data toconfirm them.Q.Which statement is best supported by the data presented in the graph?a)The W boson and the Z boson were proposed and experimentally confirmed at about the same time.b)The Higgs boson was experimentally confirmed more quickly than were most other particles.c)The tau neutrino was experimentally confirmed at about the same time as the tau.d)The muon neutrino took longer to experimentally confirm than did the electron neutrino.Correct answer is option 'A'. Can you explain this answer? in English & in Hindi are available as part of our courses for SAT.
Download more important topics, notes, lectures and mock test series for SAT Exam by signing up for free.
Here you can find the meaning of Question based on the following passage and supplementary material.This passage is adapted from Brian Greene, “How the Higgs Boson Was Found.” ©2013 by Smithsonian Institution. The Higgs boson is an elementary particle associated with the Higgs field. Experiments conducted in 2012–2013 tentatively confirmed the existence of the Higgs boson and thus of the Higgs field.Nearly a half-century ago, Peter Higgs and ahandful of other physicists were trying to understandthe origin of a basic physical feature: mass. You canthink of mass as an object’s heft or, a little more(5) precisely, as the resistance it offers to having itsmotion changed. Push on a freight train (or afeather) to increase its speed, and the resistance youfeel reflects its mass. At a microscopic level, thefreight train’s mass comes from its constituent(10) molecules and atoms, which are themselves builtfrom fundamental particles, electrons and quarks.But where do the masses of these and otherfundamental particles come from?When physicists in the 1960s modeled the(15) behavior of these particles using equations rooted inquantum physics, they encountered a puzzle. If theyimagined that the particles were all massless, theneach term in the equations clicked into a perfectlysymmetric pattern, like the tips of a perfect(20) snowflake. And this symmetry was not justmathematically elegant. It explained patterns evidentin the experimental data. But—and here’s thepuzzle—physicists knew that the particles did havemass, and when they modified the equations to(25) account for this fact, the mathematical harmony wasspoiled. The equations became complex andunwieldy and, worse still, inconsistent.What to do? Here’s the idea put forward by Higgs.Don’t shove the particles’ masses down the throat of(30) the beautiful equations. Instead, keep the equationspristine and symmetric, but consider them operatingwithin a peculiar environment. Imagine that all ofspace is uniformly filled with an invisiblesubstance—now called the Higgs field—that exerts a(35) drag force on particles when they accelerate throughit. Push on a fundamental particle in an effort toincrease its speed and, according to Higgs, you wouldfeel this drag force as a resistance. Justifiably, youwould interpret the resistance as the particle’s mass.(40) For a mental toehold, think of a ping-pong ballsubmerged in water. When you push on theping-pong ball, it will feel much more massive than itdoes outside of water. Its interaction with the wateryenvironment has the effect of endowing it with mass.(45) So with particles submerged in the Higgs field.In 1964, Higgs submitted a paper to a prominentphysics journal in which he formulated this ideamathematically. The paper was rejected. Not becauseit contained a technical error, but because the(50) premise of an invisible something permeating space,interacting with particles to provide their mass, well,it all just seemed like heaps of overwroughtspeculation. The editors of the journal deemed it “ofno obvious relevance to physics.”(55) But Higgs persevered (and his revised paperappeared later that year in another journal), andphysicists who took the time to study the proposalgradually realized that his idea was a stroke of genius,one that allowed them to have their cake and eat it(60) too. In Higgs’s scheme, the fundamental equationscan retain their pristine form because the dirty workof providing the particles’ masses is relegated to theenvironment.While I wasn’t around to witness the initial(65) rejection of Higgs’s proposal in 1964 (well, I wasaround, but only barely), I can attest that by themid-1980s, the assessment had changed. The physicscommunity had, for the most part, fully bought intothe idea that there was a Higgs field permeating(70) space. In fact, in a graduate course I took thatcovered what’s known as the Standard Model ofParticle Physics (the quantum equations physicistshave assembled to describe the particles of matterand the dominant forces by which they influence(75) each other), the professor presented the Higgs fieldwith such certainty that for a long while I had no ideait had yet to be established experimentally.On occasion, that happens in physics. Mathematicalequations can sometimes tell such a convincing tale,(80) they can seemingly radiate reality so strongly, thatthey become entrenched in the vernacular ofworking physicists, even before there’s data toconfirm them.Q.Which statement is best supported by the data presented in the graph?a)The W boson and the Z boson were proposed and experimentally confirmed at about the same time.b)The Higgs boson was experimentally confirmed more quickly than were most other particles.c)The tau neutrino was experimentally confirmed at about the same time as the tau.d)The muon neutrino took longer to experimentally confirm than did the electron neutrino.Correct answer is option 'A'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
Question based on the following passage and supplementary material.This passage is adapted from Brian Greene, “How the Higgs Boson Was Found.” ©2013 by Smithsonian Institution. The Higgs boson is an elementary particle associated with the Higgs field. Experiments conducted in 2012–2013 tentatively confirmed the existence of the Higgs boson and thus of the Higgs field.Nearly a half-century ago, Peter Higgs and ahandful of other physicists were trying to understandthe origin of a basic physical feature: mass. You canthink of mass as an object’s heft or, a little more(5) precisely, as the resistance it offers to having itsmotion changed. Push on a freight train (or afeather) to increase its speed, and the resistance youfeel reflects its mass. At a microscopic level, thefreight train’s mass comes from its constituent(10) molecules and atoms, which are themselves builtfrom fundamental particles, electrons and quarks.But where do the masses of these and otherfundamental particles come from?When physicists in the 1960s modeled the(15) behavior of these particles using equations rooted inquantum physics, they encountered a puzzle. If theyimagined that the particles were all massless, theneach term in the equations clicked into a perfectlysymmetric pattern, like the tips of a perfect(20) snowflake. And this symmetry was not justmathematically elegant. It explained patterns evidentin the experimental data. But—and here’s thepuzzle—physicists knew that the particles did havemass, and when they modified the equations to(25) account for this fact, the mathematical harmony wasspoiled. The equations became complex andunwieldy and, worse still, inconsistent.What to do? Here’s the idea put forward by Higgs.Don’t shove the particles’ masses down the throat of(30) the beautiful equations. Instead, keep the equationspristine and symmetric, but consider them operatingwithin a peculiar environment. Imagine that all ofspace is uniformly filled with an invisiblesubstance—now called the Higgs field—that exerts a(35) drag force on particles when they accelerate throughit. Push on a fundamental particle in an effort toincrease its speed and, according to Higgs, you wouldfeel this drag force as a resistance. Justifiably, youwould interpret the resistance as the particle’s mass.(40) For a mental toehold, think of a ping-pong ballsubmerged in water. When you push on theping-pong ball, it will feel much more massive than itdoes outside of water. Its interaction with the wateryenvironment has the effect of endowing it with mass.(45) So with particles submerged in the Higgs field.In 1964, Higgs submitted a paper to a prominentphysics journal in which he formulated this ideamathematically. The paper was rejected. Not becauseit contained a technical error, but because the(50) premise of an invisible something permeating space,interacting with particles to provide their mass, well,it all just seemed like heaps of overwroughtspeculation. The editors of the journal deemed it “ofno obvious relevance to physics.”(55) But Higgs persevered (and his revised paperappeared later that year in another journal), andphysicists who took the time to study the proposalgradually realized that his idea was a stroke of genius,one that allowed them to have their cake and eat it(60) too. In Higgs’s scheme, the fundamental equationscan retain their pristine form because the dirty workof providing the particles’ masses is relegated to theenvironment.While I wasn’t around to witness the initial(65) rejection of Higgs’s proposal in 1964 (well, I wasaround, but only barely), I can attest that by themid-1980s, the assessment had changed. The physicscommunity had, for the most part, fully bought intothe idea that there was a Higgs field permeating(70) space. In fact, in a graduate course I took thatcovered what’s known as the Standard Model ofParticle Physics (the quantum equations physicistshave assembled to describe the particles of matterand the dominant forces by which they influence(75) each other), the professor presented the Higgs fieldwith such certainty that for a long while I had no ideait had yet to be established experimentally.On occasion, that happens in physics. Mathematicalequations can sometimes tell such a convincing tale,(80) they can seemingly radiate reality so strongly, thatthey become entrenched in the vernacular ofworking physicists, even before there’s data toconfirm them.Q.Which statement is best supported by the data presented in the graph?a)The W boson and the Z boson were proposed and experimentally confirmed at about the same time.b)The Higgs boson was experimentally confirmed more quickly than were most other particles.c)The tau neutrino was experimentally confirmed at about the same time as the tau.d)The muon neutrino took longer to experimentally confirm than did the electron neutrino.Correct answer is option 'A'. Can you explain this answer?, a detailed solution for Question based on the following passage and supplementary material.This passage is adapted from Brian Greene, “How the Higgs Boson Was Found.” ©2013 by Smithsonian Institution. The Higgs boson is an elementary particle associated with the Higgs field. Experiments conducted in 2012–2013 tentatively confirmed the existence of the Higgs boson and thus of the Higgs field.Nearly a half-century ago, Peter Higgs and ahandful of other physicists were trying to understandthe origin of a basic physical feature: mass. You canthink of mass as an object’s heft or, a little more(5) precisely, as the resistance it offers to having itsmotion changed. Push on a freight train (or afeather) to increase its speed, and the resistance youfeel reflects its mass. At a microscopic level, thefreight train’s mass comes from its constituent(10) molecules and atoms, which are themselves builtfrom fundamental particles, electrons and quarks.But where do the masses of these and otherfundamental particles come from?When physicists in the 1960s modeled the(15) behavior of these particles using equations rooted inquantum physics, they encountered a puzzle. If theyimagined that the particles were all massless, theneach term in the equations clicked into a perfectlysymmetric pattern, like the tips of a perfect(20) snowflake. And this symmetry was not justmathematically elegant. It explained patterns evidentin the experimental data. But—and here’s thepuzzle—physicists knew that the particles did havemass, and when they modified the equations to(25) account for this fact, the mathematical harmony wasspoiled. The equations became complex andunwieldy and, worse still, inconsistent.What to do? Here’s the idea put forward by Higgs.Don’t shove the particles’ masses down the throat of(30) the beautiful equations. Instead, keep the equationspristine and symmetric, but consider them operatingwithin a peculiar environment. Imagine that all ofspace is uniformly filled with an invisiblesubstance—now called the Higgs field—that exerts a(35) drag force on particles when they accelerate throughit. Push on a fundamental particle in an effort toincrease its speed and, according to Higgs, you wouldfeel this drag force as a resistance. Justifiably, youwould interpret the resistance as the particle’s mass.(40) For a mental toehold, think of a ping-pong ballsubmerged in water. When you push on theping-pong ball, it will feel much more massive than itdoes outside of water. Its interaction with the wateryenvironment has the effect of endowing it with mass.(45) So with particles submerged in the Higgs field.In 1964, Higgs submitted a paper to a prominentphysics journal in which he formulated this ideamathematically. The paper was rejected. Not becauseit contained a technical error, but because the(50) premise of an invisible something permeating space,interacting with particles to provide their mass, well,it all just seemed like heaps of overwroughtspeculation. The editors of the journal deemed it “ofno obvious relevance to physics.”(55) But Higgs persevered (and his revised paperappeared later that year in another journal), andphysicists who took the time to study the proposalgradually realized that his idea was a stroke of genius,one that allowed them to have their cake and eat it(60) too. In Higgs’s scheme, the fundamental equationscan retain their pristine form because the dirty workof providing the particles’ masses is relegated to theenvironment.While I wasn’t around to witness the initial(65) rejection of Higgs’s proposal in 1964 (well, I wasaround, but only barely), I can attest that by themid-1980s, the assessment had changed. The physicscommunity had, for the most part, fully bought intothe idea that there was a Higgs field permeating(70) space. In fact, in a graduate course I took thatcovered what’s known as the Standard Model ofParticle Physics (the quantum equations physicistshave assembled to describe the particles of matterand the dominant forces by which they influence(75) each other), the professor presented the Higgs fieldwith such certainty that for a long while I had no ideait had yet to be established experimentally.On occasion, that happens in physics. Mathematicalequations can sometimes tell such a convincing tale,(80) they can seemingly radiate reality so strongly, thatthey become entrenched in the vernacular ofworking physicists, even before there’s data toconfirm them.Q.Which statement is best supported by the data presented in the graph?a)The W boson and the Z boson were proposed and experimentally confirmed at about the same time.b)The Higgs boson was experimentally confirmed more quickly than were most other particles.c)The tau neutrino was experimentally confirmed at about the same time as the tau.d)The muon neutrino took longer to experimentally confirm than did the electron neutrino.Correct answer is option 'A'. Can you explain this answer? has been provided alongside types of Question based on the following passage and supplementary material.This passage is adapted from Brian Greene, “How the Higgs Boson Was Found.” ©2013 by Smithsonian Institution. The Higgs boson is an elementary particle associated with the Higgs field. Experiments conducted in 2012–2013 tentatively confirmed the existence of the Higgs boson and thus of the Higgs field.Nearly a half-century ago, Peter Higgs and ahandful of other physicists were trying to understandthe origin of a basic physical feature: mass. You canthink of mass as an object’s heft or, a little more(5) precisely, as the resistance it offers to having itsmotion changed. Push on a freight train (or afeather) to increase its speed, and the resistance youfeel reflects its mass. At a microscopic level, thefreight train’s mass comes from its constituent(10) molecules and atoms, which are themselves builtfrom fundamental particles, electrons and quarks.But where do the masses of these and otherfundamental particles come from?When physicists in the 1960s modeled the(15) behavior of these particles using equations rooted inquantum physics, they encountered a puzzle. If theyimagined that the particles were all massless, theneach term in the equations clicked into a perfectlysymmetric pattern, like the tips of a perfect(20) snowflake. And this symmetry was not justmathematically elegant. It explained patterns evidentin the experimental data. But—and here’s thepuzzle—physicists knew that the particles did havemass, and when they modified the equations to(25) account for this fact, the mathematical harmony wasspoiled. The equations became complex andunwieldy and, worse still, inconsistent.What to do? Here’s the idea put forward by Higgs.Don’t shove the particles’ masses down the throat of(30) the beautiful equations. Instead, keep the equationspristine and symmetric, but consider them operatingwithin a peculiar environment. Imagine that all ofspace is uniformly filled with an invisiblesubstance—now called the Higgs field—that exerts a(35) drag force on particles when they accelerate throughit. Push on a fundamental particle in an effort toincrease its speed and, according to Higgs, you wouldfeel this drag force as a resistance. Justifiably, youwould interpret the resistance as the particle’s mass.(40) For a mental toehold, think of a ping-pong ballsubmerged in water. When you push on theping-pong ball, it will feel much more massive than itdoes outside of water. Its interaction with the wateryenvironment has the effect of endowing it with mass.(45) So with particles submerged in the Higgs field.In 1964, Higgs submitted a paper to a prominentphysics journal in which he formulated this ideamathematically. The paper was rejected. Not becauseit contained a technical error, but because the(50) premise of an invisible something permeating space,interacting with particles to provide their mass, well,it all just seemed like heaps of overwroughtspeculation. The editors of the journal deemed it “ofno obvious relevance to physics.”(55) But Higgs persevered (and his revised paperappeared later that year in another journal), andphysicists who took the time to study the proposalgradually realized that his idea was a stroke of genius,one that allowed them to have their cake and eat it(60) too. In Higgs’s scheme, the fundamental equationscan retain their pristine form because the dirty workof providing the particles’ masses is relegated to theenvironment.While I wasn’t around to witness the initial(65) rejection of Higgs’s proposal in 1964 (well, I wasaround, but only barely), I can attest that by themid-1980s, the assessment had changed. The physicscommunity had, for the most part, fully bought intothe idea that there was a Higgs field permeating(70) space. In fact, in a graduate course I took thatcovered what’s known as the Standard Model ofParticle Physics (the quantum equations physicistshave assembled to describe the particles of matterand the dominant forces by which they influence(75) each other), the professor presented the Higgs fieldwith such certainty that for a long while I had no ideait had yet to be established experimentally.On occasion, that happens in physics. Mathematicalequations can sometimes tell such a convincing tale,(80) they can seemingly radiate reality so strongly, thatthey become entrenched in the vernacular ofworking physicists, even before there’s data toconfirm them.Q.Which statement is best supported by the data presented in the graph?a)The W boson and the Z boson were proposed and experimentally confirmed at about the same time.b)The Higgs boson was experimentally confirmed more quickly than were most other particles.c)The tau neutrino was experimentally confirmed at about the same time as the tau.d)The muon neutrino took longer to experimentally confirm than did the electron neutrino.Correct answer is option 'A'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice Question based on the following passage and supplementary material.This passage is adapted from Brian Greene, “How the Higgs Boson Was Found.” ©2013 by Smithsonian Institution. The Higgs boson is an elementary particle associated with the Higgs field. Experiments conducted in 2012–2013 tentatively confirmed the existence of the Higgs boson and thus of the Higgs field.Nearly a half-century ago, Peter Higgs and ahandful of other physicists were trying to understandthe origin of a basic physical feature: mass. You canthink of mass as an object’s heft or, a little more(5) precisely, as the resistance it offers to having itsmotion changed. Push on a freight train (or afeather) to increase its speed, and the resistance youfeel reflects its mass. At a microscopic level, thefreight train’s mass comes from its constituent(10) molecules and atoms, which are themselves builtfrom fundamental particles, electrons and quarks.But where do the masses of these and otherfundamental particles come from?When physicists in the 1960s modeled the(15) behavior of these particles using equations rooted inquantum physics, they encountered a puzzle. If theyimagined that the particles were all massless, theneach term in the equations clicked into a perfectlysymmetric pattern, like the tips of a perfect(20) snowflake. And this symmetry was not justmathematically elegant. It explained patterns evidentin the experimental data. But—and here’s thepuzzle—physicists knew that the particles did havemass, and when they modified the equations to(25) account for this fact, the mathematical harmony wasspoiled. The equations became complex andunwieldy and, worse still, inconsistent.What to do? Here’s the idea put forward by Higgs.Don’t shove the particles’ masses down the throat of(30) the beautiful equations. Instead, keep the equationspristine and symmetric, but consider them operatingwithin a peculiar environment. Imagine that all ofspace is uniformly filled with an invisiblesubstance—now called the Higgs field—that exerts a(35) drag force on particles when they accelerate throughit. Push on a fundamental particle in an effort toincrease its speed and, according to Higgs, you wouldfeel this drag force as a resistance. Justifiably, youwould interpret the resistance as the particle’s mass.(40) For a mental toehold, think of a ping-pong ballsubmerged in water. When you push on theping-pong ball, it will feel much more massive than itdoes outside of water. Its interaction with the wateryenvironment has the effect of endowing it with mass.(45) So with particles submerged in the Higgs field.In 1964, Higgs submitted a paper to a prominentphysics journal in which he formulated this ideamathematically. The paper was rejected. Not becauseit contained a technical error, but because the(50) premise of an invisible something permeating space,interacting with particles to provide their mass, well,it all just seemed like heaps of overwroughtspeculation. The editors of the journal deemed it “ofno obvious relevance to physics.”(55) But Higgs persevered (and his revised paperappeared later that year in another journal), andphysicists who took the time to study the proposalgradually realized that his idea was a stroke of genius,one that allowed them to have their cake and eat it(60) too. In Higgs’s scheme, the fundamental equationscan retain their pristine form because the dirty workof providing the particles’ masses is relegated to theenvironment.While I wasn’t around to witness the initial(65) rejection of Higgs’s proposal in 1964 (well, I wasaround, but only barely), I can attest that by themid-1980s, the assessment had changed. The physicscommunity had, for the most part, fully bought intothe idea that there was a Higgs field permeating(70) space. In fact, in a graduate course I took thatcovered what’s known as the Standard Model ofParticle Physics (the quantum equations physicistshave assembled to describe the particles of matterand the dominant forces by which they influence(75) each other), the professor presented the Higgs fieldwith such certainty that for a long while I had no ideait had yet to be established experimentally.On occasion, that happens in physics. Mathematicalequations can sometimes tell such a convincing tale,(80) they can seemingly radiate reality so strongly, thatthey become entrenched in the vernacular ofworking physicists, even before there’s data toconfirm them.Q.Which statement is best supported by the data presented in the graph?a)The W boson and the Z boson were proposed and experimentally confirmed at about the same time.b)The Higgs boson was experimentally confirmed more quickly than were most other particles.c)The tau neutrino was experimentally confirmed at about the same time as the tau.d)The muon neutrino took longer to experimentally confirm than did the electron neutrino.Correct answer is option 'A'. Can you explain this answer? tests, examples and also practice SAT tests.

|
Explore Courses for SAT exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
























