JEE Exam > JEE Questions > Which of the following will be the correct sp...
Start Learning for Free
Which of the following will be the correct spin magnetic moment value (B.M.) for the compound Hg[Co(SCN)4]?
(Round off up to 2 decimal places)
(Round off up to 2 decimal places)
Correct answer is '3.87'. Can you explain this answer?
Verified Answer
Which of the following will be the correct spin magnetic moment value ...
The oxidation number of cobalt in the given complex is +2.
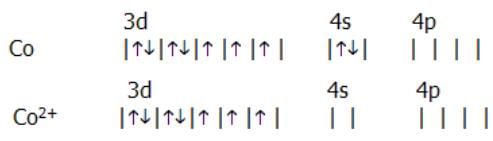
+23
Number of unpaired electrons (n) = 3
Spin magnetic moment (ms)
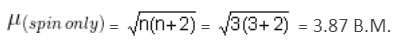
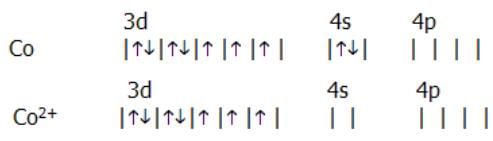
Co
in the complex is sp
hybridised and the complex has a tetrahedral geometry.
Number of unpaired electrons (n) = 3
Spin magnetic moment (ms)
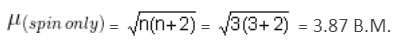
Most Upvoted Answer
Which of the following will be the correct spin magnetic moment value ...
Spin Magnetic Moment (B.M.)
The spin magnetic moment is a measure of the magnetic behavior of a compound or an atom. It is represented in units of Bohr magnetons (B.M.) and is calculated based on the number of unpaired electrons in the compound or atom.
Structure of Hg[Co(SCN)4]
To determine the spin magnetic moment of the compound Hg[Co(SCN)4], we first need to understand its structure.
Hg[Co(SCN)4] is a coordination compound where mercury (Hg) acts as a cation and forms coordination bonds with four thiocyanate (SCN) anions. The central cobalt (Co) atom is coordinated to four thiocyanate ligands, forming a tetrahedral geometry.
Determining the Number of Unpaired Electrons
To calculate the spin magnetic moment of Hg[Co(SCN)4], we need to determine the number of unpaired electrons in the compound. This can be done by considering the electronic configuration of the central cobalt atom.
The atomic number of cobalt (Co) is 27. Its electronic configuration is [Ar] 3d^7 4s^2. In a tetrahedral complex, the d orbitals split into two sets of different energy levels - the lower energy set (t2g) and the higher energy set (eg).
Since there are four ligands in the coordination sphere, the d orbitals are split into t2g (dxy, dxz, and dyz) and eg (dx^2-y^2 and dz^2).
Calculating the Number of Unpaired Electrons
To determine the number of unpaired electrons, we need to fill the t2g and eg orbitals with electrons based on Hund's rule. Hund's rule states that electrons occupy separate orbitals with parallel spins before pairing up.
In the case of Hg[Co(SCN)4], the electronic configuration of cobalt is [Ar] 3d^7. Since there are three t2g orbitals, three electrons will occupy these orbitals with parallel spins. Therefore, there will be one unpaired electron in the eg set of orbitals.
Calculating the Spin Magnetic Moment
The spin magnetic moment can be calculated using the formula:
Spin Magnetic Moment (B.M.) = √n(n+2)
Where n is the number of unpaired electrons.
In the case of Hg[Co(SCN)4], there is one unpaired electron (n = 1). Plugging this value into the formula, we get:
Spin Magnetic Moment (B.M.) = √1(1+2) = √3
Rounding off to two decimal places, the spin magnetic moment of Hg[Co(SCN)4] is 3.87 B.M.
Summary
- The spin magnetic moment of a compound or atom is a measure of its magnetic behavior.
- Hg[Co(SCN)4] is a coordination compound with a tetrahedral geometry.
- The electronic configuration of cobalt in Hg[Co(SCN)4] is [Ar] 3d^7.
- Based on the electronic configuration, there is one unpaired electron in the eg set of orbitals.
-
The spin magnetic moment is a measure of the magnetic behavior of a compound or an atom. It is represented in units of Bohr magnetons (B.M.) and is calculated based on the number of unpaired electrons in the compound or atom.
Structure of Hg[Co(SCN)4]
To determine the spin magnetic moment of the compound Hg[Co(SCN)4], we first need to understand its structure.
Hg[Co(SCN)4] is a coordination compound where mercury (Hg) acts as a cation and forms coordination bonds with four thiocyanate (SCN) anions. The central cobalt (Co) atom is coordinated to four thiocyanate ligands, forming a tetrahedral geometry.
Determining the Number of Unpaired Electrons
To calculate the spin magnetic moment of Hg[Co(SCN)4], we need to determine the number of unpaired electrons in the compound. This can be done by considering the electronic configuration of the central cobalt atom.
The atomic number of cobalt (Co) is 27. Its electronic configuration is [Ar] 3d^7 4s^2. In a tetrahedral complex, the d orbitals split into two sets of different energy levels - the lower energy set (t2g) and the higher energy set (eg).
Since there are four ligands in the coordination sphere, the d orbitals are split into t2g (dxy, dxz, and dyz) and eg (dx^2-y^2 and dz^2).
Calculating the Number of Unpaired Electrons
To determine the number of unpaired electrons, we need to fill the t2g and eg orbitals with electrons based on Hund's rule. Hund's rule states that electrons occupy separate orbitals with parallel spins before pairing up.
In the case of Hg[Co(SCN)4], the electronic configuration of cobalt is [Ar] 3d^7. Since there are three t2g orbitals, three electrons will occupy these orbitals with parallel spins. Therefore, there will be one unpaired electron in the eg set of orbitals.
Calculating the Spin Magnetic Moment
The spin magnetic moment can be calculated using the formula:
Spin Magnetic Moment (B.M.) = √n(n+2)
Where n is the number of unpaired electrons.
In the case of Hg[Co(SCN)4], there is one unpaired electron (n = 1). Plugging this value into the formula, we get:
Spin Magnetic Moment (B.M.) = √1(1+2) = √3
Rounding off to two decimal places, the spin magnetic moment of Hg[Co(SCN)4] is 3.87 B.M.
Summary
- The spin magnetic moment of a compound or atom is a measure of its magnetic behavior.
- Hg[Co(SCN)4] is a coordination compound with a tetrahedral geometry.
- The electronic configuration of cobalt in Hg[Co(SCN)4] is [Ar] 3d^7.
- Based on the electronic configuration, there is one unpaired electron in the eg set of orbitals.
-

|
Explore Courses for JEE exam
|

|
Question Description
Which of the following will be the correct spin magnetic moment value (B.M.) for the compound Hg[Co(SCN)4]?(Round off up to 2 decimal places)Correct answer is '3.87'. Can you explain this answer? for JEE 2025 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about Which of the following will be the correct spin magnetic moment value (B.M.) for the compound Hg[Co(SCN)4]?(Round off up to 2 decimal places)Correct answer is '3.87'. Can you explain this answer? covers all topics & solutions for JEE 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Which of the following will be the correct spin magnetic moment value (B.M.) for the compound Hg[Co(SCN)4]?(Round off up to 2 decimal places)Correct answer is '3.87'. Can you explain this answer?.
Which of the following will be the correct spin magnetic moment value (B.M.) for the compound Hg[Co(SCN)4]?(Round off up to 2 decimal places)Correct answer is '3.87'. Can you explain this answer? for JEE 2025 is part of JEE preparation. The Question and answers have been prepared according to the JEE exam syllabus. Information about Which of the following will be the correct spin magnetic moment value (B.M.) for the compound Hg[Co(SCN)4]?(Round off up to 2 decimal places)Correct answer is '3.87'. Can you explain this answer? covers all topics & solutions for JEE 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Which of the following will be the correct spin magnetic moment value (B.M.) for the compound Hg[Co(SCN)4]?(Round off up to 2 decimal places)Correct answer is '3.87'. Can you explain this answer?.
Solutions for Which of the following will be the correct spin magnetic moment value (B.M.) for the compound Hg[Co(SCN)4]?(Round off up to 2 decimal places)Correct answer is '3.87'. Can you explain this answer? in English & in Hindi are available as part of our courses for JEE.
Download more important topics, notes, lectures and mock test series for JEE Exam by signing up for free.
Here you can find the meaning of Which of the following will be the correct spin magnetic moment value (B.M.) for the compound Hg[Co(SCN)4]?(Round off up to 2 decimal places)Correct answer is '3.87'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
Which of the following will be the correct spin magnetic moment value (B.M.) for the compound Hg[Co(SCN)4]?(Round off up to 2 decimal places)Correct answer is '3.87'. Can you explain this answer?, a detailed solution for Which of the following will be the correct spin magnetic moment value (B.M.) for the compound Hg[Co(SCN)4]?(Round off up to 2 decimal places)Correct answer is '3.87'. Can you explain this answer? has been provided alongside types of Which of the following will be the correct spin magnetic moment value (B.M.) for the compound Hg[Co(SCN)4]?(Round off up to 2 decimal places)Correct answer is '3.87'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice Which of the following will be the correct spin magnetic moment value (B.M.) for the compound Hg[Co(SCN)4]?(Round off up to 2 decimal places)Correct answer is '3.87'. Can you explain this answer? tests, examples and also practice JEE tests.

|
Explore Courses for JEE exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.























