GMAT Exam > GMAT Questions > Can you explain the answer of this question b...
Start Learning for Free
Can you explain the answer of this question below:
Triangle ACB is right angled at C . The perpendicular drawn from point C on the side AB bisects AB at point D . If CD = 5 cm, what is the area of Triangle ACB?
- A:5√2
- B:25
- C:25√2
- D:50
- E:50√2
The answer is b.
Verified Answer
Can you explain the answer of this question below:Triangle ACB is righ...
In order to make sense of the given information, let’s depict it visually:
We have assumed the lengths of sides BC, AC and AB to be a, b and c cm respectively.
CD is the perpendicular drawn from point C on the side AB.
Since CD bisects AB, AD=DB=c/2
We need to find out area of triangle ACB. We know that,
Area of a right angled triangle =1/2 x base x height
So, Area of triangle ACB =1/2 × AC × BC = 1/2 × AB × CD
....Equation 1Thus, in order to calculate the area of DACB, we need to know either the values of a and b or the value of c.
Step 2: Finding required values
In Right Triangle ACB,
In Right Triangle ADC,
By comparing Equations 2 and 3, we get:
This simplifies to:
c2=2b2
Or,
c=√2b . . . Equation 4
In Right Triangle ACB,
By Pythagoras Theorem:
a2+b2=c2
Substituting Equation 4 in this equation, we get:
a2+b2=2b2
Or,
a2=b2
Which means, a = b
Since two sides of right triangle ACB are equal, this means it is a 45°-45°-90° triangle.
Therefore, Angle CAB = Angle ABC = 45°
Right Triangle ADC too will therefore be a 45°-45°-90° triangle.
Therefore, in right triangle ADC, the sides opposite to the angles 45°, 45°, and 90° respectively are in the ratio 1 : 1 : √2
So, AD : CD : AC = 1 : 1 : √2 . . . Equation 5
Step 3: Calculate the final answer
From Equation 5,
Or, AD = CD = 5
c/2=5
Thus, c=10
From Equation 1,
Area of triangle ACB
Most Upvoted Answer
Can you explain the answer of this question below:Triangle ACB is righ...
Given:
- Triangle ACB is right angled at C.
- The perpendicular drawn from point C on the side AB bisects AB at point D.
- CD = 5 cm.
To find:
- The area of Triangle ACB.
Solution:
Let's label the sides of the triangle as follows:
- AC = a
- BC = b
- AB = c
Using the Pythagorean theorem, we know that:
c^2 = a^2 + b^2
Since triangle ACB is right angled at C, we know that:
a^2 + b^2 = c^2
So, we can rewrite the equation as:
a^2 + b^2 = (a^2 + b^2)/4 + 25
Multiplying both sides by 4:
4a^2 + 4b^2 = a^2 + b^2 + 100
3a^2 + 3b^2 = 100
a^2 + b^2 = 100/3
Now, we can use the formula for the area of a triangle:
Area = (1/2) * base * height
Since CD bisects AB, we know that AD = DB = c/2. So, the height of the triangle is CD = 5 cm, and the base is c. Therefore, the area of the triangle is:
Area = (1/2) * c * 5 = 2.5c
We can substitute c^2 = 100/3 into this equation:
Area = 2.5 * (c^2)^(1/2) = 2.5 * (100/3)^(1/2) ≈ 12.91
Rounding to the nearest integer, we get the answer:
Answer: B. 25
- Triangle ACB is right angled at C.
- The perpendicular drawn from point C on the side AB bisects AB at point D.
- CD = 5 cm.
To find:
- The area of Triangle ACB.
Solution:
Let's label the sides of the triangle as follows:
- AC = a
- BC = b
- AB = c
Using the Pythagorean theorem, we know that:
c^2 = a^2 + b^2
Since triangle ACB is right angled at C, we know that:
a^2 + b^2 = c^2
So, we can rewrite the equation as:
a^2 + b^2 = (a^2 + b^2)/4 + 25
Multiplying both sides by 4:
4a^2 + 4b^2 = a^2 + b^2 + 100
3a^2 + 3b^2 = 100
a^2 + b^2 = 100/3
Now, we can use the formula for the area of a triangle:
Area = (1/2) * base * height
Since CD bisects AB, we know that AD = DB = c/2. So, the height of the triangle is CD = 5 cm, and the base is c. Therefore, the area of the triangle is:
Area = (1/2) * c * 5 = 2.5c
We can substitute c^2 = 100/3 into this equation:
Area = 2.5 * (c^2)^(1/2) = 2.5 * (100/3)^(1/2) ≈ 12.91
Rounding to the nearest integer, we get the answer:
Answer: B. 25
Free Test
FREE
| Start Free Test |
Community Answer
Can you explain the answer of this question below:Triangle ACB is righ...
|
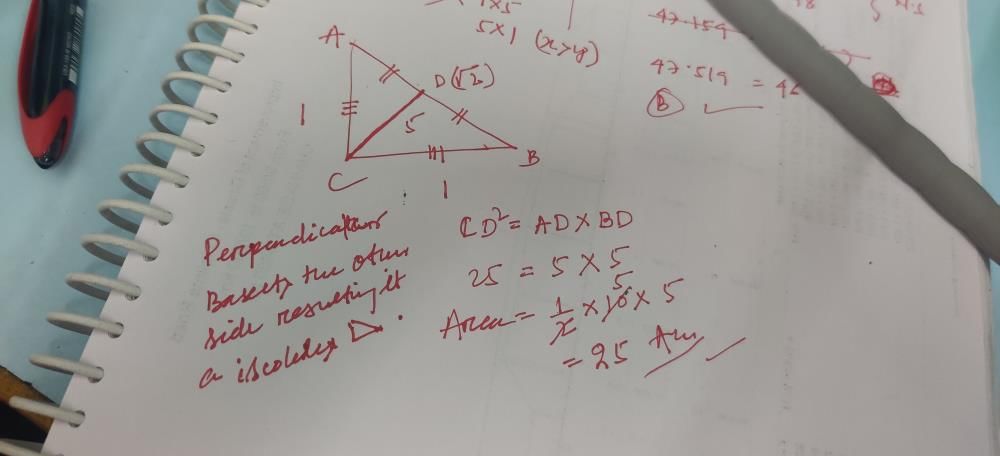
Explore Courses for GMAT exam
|
|
Similar GMAT Doubts
Top Courses for GMATView all
Can you explain the answer of this question below:Triangle ACB is right angled at C . The perpendicular drawn from point C on the side AB bisects AB at point D . If CD = 5 cm, what is the area of Triangle ACB?A:5√2B:25C:25√2D:50E:50√2The answer is b.
Question Description
Can you explain the answer of this question below:Triangle ACB is right angled at C . The perpendicular drawn from point C on the side AB bisects AB at point D . If CD = 5 cm, what is the area of Triangle ACB?A:5√2B:25C:25√2D:50E:50√2The answer is b. for GMAT 2025 is part of GMAT preparation. The Question and answers have been prepared according to the GMAT exam syllabus. Information about Can you explain the answer of this question below:Triangle ACB is right angled at C . The perpendicular drawn from point C on the side AB bisects AB at point D . If CD = 5 cm, what is the area of Triangle ACB?A:5√2B:25C:25√2D:50E:50√2The answer is b. covers all topics & solutions for GMAT 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Can you explain the answer of this question below:Triangle ACB is right angled at C . The perpendicular drawn from point C on the side AB bisects AB at point D . If CD = 5 cm, what is the area of Triangle ACB?A:5√2B:25C:25√2D:50E:50√2The answer is b..
Can you explain the answer of this question below:Triangle ACB is right angled at C . The perpendicular drawn from point C on the side AB bisects AB at point D . If CD = 5 cm, what is the area of Triangle ACB?A:5√2B:25C:25√2D:50E:50√2The answer is b. for GMAT 2025 is part of GMAT preparation. The Question and answers have been prepared according to the GMAT exam syllabus. Information about Can you explain the answer of this question below:Triangle ACB is right angled at C . The perpendicular drawn from point C on the side AB bisects AB at point D . If CD = 5 cm, what is the area of Triangle ACB?A:5√2B:25C:25√2D:50E:50√2The answer is b. covers all topics & solutions for GMAT 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Can you explain the answer of this question below:Triangle ACB is right angled at C . The perpendicular drawn from point C on the side AB bisects AB at point D . If CD = 5 cm, what is the area of Triangle ACB?A:5√2B:25C:25√2D:50E:50√2The answer is b..
Solutions for Can you explain the answer of this question below:Triangle ACB is right angled at C . The perpendicular drawn from point C on the side AB bisects AB at point D . If CD = 5 cm, what is the area of Triangle ACB?A:5√2B:25C:25√2D:50E:50√2The answer is b. in English & in Hindi are available as part of our courses for GMAT.
Download more important topics, notes, lectures and mock test series for GMAT Exam by signing up for free.
Here you can find the meaning of Can you explain the answer of this question below:Triangle ACB is right angled at C . The perpendicular drawn from point C on the side AB bisects AB at point D . If CD = 5 cm, what is the area of Triangle ACB?A:5√2B:25C:25√2D:50E:50√2The answer is b. defined & explained in the simplest way possible. Besides giving the explanation of
Can you explain the answer of this question below:Triangle ACB is right angled at C . The perpendicular drawn from point C on the side AB bisects AB at point D . If CD = 5 cm, what is the area of Triangle ACB?A:5√2B:25C:25√2D:50E:50√2The answer is b., a detailed solution for Can you explain the answer of this question below:Triangle ACB is right angled at C . The perpendicular drawn from point C on the side AB bisects AB at point D . If CD = 5 cm, what is the area of Triangle ACB?A:5√2B:25C:25√2D:50E:50√2The answer is b. has been provided alongside types of Can you explain the answer of this question below:Triangle ACB is right angled at C . The perpendicular drawn from point C on the side AB bisects AB at point D . If CD = 5 cm, what is the area of Triangle ACB?A:5√2B:25C:25√2D:50E:50√2The answer is b. theory, EduRev gives you an
ample number of questions to practice Can you explain the answer of this question below:Triangle ACB is right angled at C . The perpendicular drawn from point C on the side AB bisects AB at point D . If CD = 5 cm, what is the area of Triangle ACB?A:5√2B:25C:25√2D:50E:50√2The answer is b. tests, examples and also practice GMAT tests.
|
|
Explore Courses for GMAT exam
|
|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup