CAT Exam > CAT Questions > There are nine boxes arranged in a 3 × ...
Start Learning for Free
There are nine boxes arranged in a 3 × 3 array as shown in Tables 1 and 2. Each box contains three sacks. Each sack has a certain number of coins, between 1 and 9, both inclusive.
The average number of coins per sack in the boxes are all distinct integers. The total number of coins in each row is the same. The total number of coins in each column is also the same.
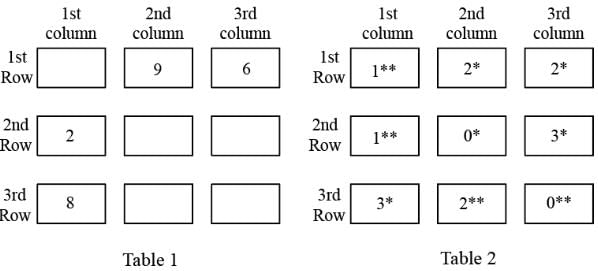
Table 1 gives information regarding the median of the numbers of coins in the three sacks in a box for some of the boxes. In Table 2 each box has a number which represents the number of sacks in that box having more than 5 coins. That number is followed by a * if the sacks in that box satisfy exactly one among the following three conditions, and it is followed by ** if two or more of these conditions are satisfied.
(i) The minimum among the numbers of coins in the three sacks in the box is 1.
(ii) The median of the numbers of coins in the three sacks is 1.
(iii) The maximum among the numbers of coins in the three sacks in the box is 9.
Table 1 gives information regarding the median of the numbers of coins in the three sacks in a box for some of the boxes. In Table 2 each box has a number which represents the number of sacks in that box having more than 5 coins. That number is followed by a * if the sacks in that box satisfy exactly one among the following three conditions, and it is followed by ** if two or more of these conditions are satisfied.
(i) The minimum among the numbers of coins in the three sacks in the box is 1.
(ii) The median of the numbers of coins in the three sacks is 1.
(iii) The maximum among the numbers of coins in the three sacks in the box is 9.
How many sacks have exactly one coin?
Correct answer is '9'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Most Upvoted Answer
There are nine boxes arranged in a 3 × 3 array as shown in Table...
We are given that each box contains three sacks. Each sack has a certain number of coins, between 1 and 9, both inclusive.
The average number of coins per sack in the boxes are all distinct integers. The total number of coins in each row is the same. The total number of coins in each column is also the same.
=> The total number of coins in a box range from 3 (1 + 1 + 1) to 27 (9 + 9 + 9)
Since, it is given that the average number of coins per sack in the boxes are all distinct integers => The total number of coins in a box would be 3, 6, 9, 12, 15, 18, 21, 24, 27 => averages of 1, 2, 3, 4,....,9 => Sum = 45.
=> Sum of averages coins in a box in a row or column = 45/3 = 15 [The total number of coins in each row is the same. The total number of coins in each column is also the same.] ==> (1)
Let us represent the final configuration of the sacks in boxes as follows:
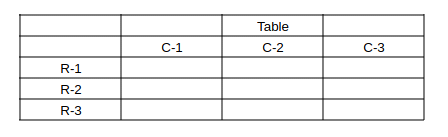
Also a bag (x,y) => bag in xth row and yth column.
We are given 2 clues => Table-1 & Table-2
Consider bag (3,1)
=> From Table-1 => Median = 8 & From Table-2 all 3 sacks have more than 5 coins. Also * => There is a 9 in one of the sacks.
=> c, 8, 9 are the coins in bag (3,1), now c > 5 & c + 8 + 9 should be a multiple of 3 => c = 7 is the only possiblility.
=> bag (3,1) has 7, 8, 9 coins with average = 8.
Consider bag (2,1)
Median = 2 and 1 sack has more than 5 coins. Also ** => conditions i & iii should be satisfied.
=> 1, 2, 9 are the coins in bag (2,1) with average = 4
Consider bag (1,2)
Median = 9 and 2 elements are more than 5. Also * => (9 is present & 1 is not present)
=> c, 9, 9 are the coins in bag (1,2) and c is not equal to 1 and less than 5 => c = 3 for c + 18 to be a multiple of 3.
=> 3, 9, 9 are the coins in bag (1,2) with average = 7.
Capturing this info. in the table:
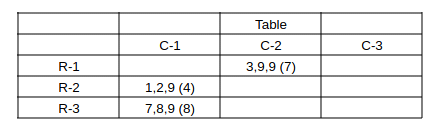
From (1), The average in bag (1,1) is 15 - 4 - 8 = 3.
From (1), The average in bag (1,3) is 15 - 3 - 7 = 5.
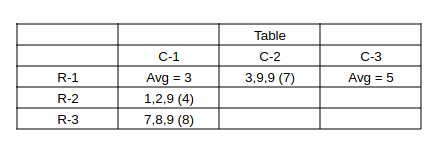
Consider bag (1,1)
Avg = 3, 1 sack has more than 5 and ** => 2 conditions are being satisfied. => (can't be condition-3 => 9 coins as the total sum of coins is it self 3*3 = 9)
=> bag (1,1) has 1, 1, 7 coins with average = 3.
Consider bag (1,3)
Avg. = 5 => Sum = 15.
Median = 6 and 2 sacks have more than 5 and * => (1 condition is satisfied)
Not condition ii as the median is 6 & Not condition iii as the sum of 2 sacks itself will become 6 + 9 = 15
=> 1, 6, c are the coins => For sum = 15 => c = 15 - 1 - 6 = 8
=> bag (1,3) has 1, 6, 8 coins with average = 5.
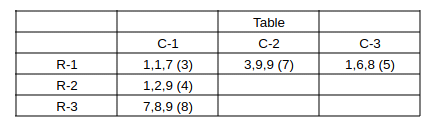
Consider bag (3,3)
0 sacks have more than 5 coins and ** => conditions i & ii are being satisfied.
=> 1,1,c are the coins. Now c = 1 or 2 or 3 or 4 => c = 1 or 4 for number of coins to be a multiple of 3.
But c = 1 as no other bag has the possibility to get avg. = 1 as bag (2,2) should have 1, b, c coins and b and c should be more than 1 as only 1*
=> bag (3,3) has 1, 1, 1 coins with average = 1.
Now, we can fill the averages in all the bags.
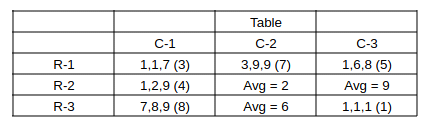
In bag (2,3) Avg. = 9 => 9, 9, 9 are the coins.
In bag (2,2) => Avg. = 2 => Sum = 6 and only 1* => smallest elemens=t should be 1.
=> 1, b, c are the coins where b + c = 5 and b,c can't be equal to 1 and less than 5 => 2 + 3 = 5 is the only possibility.
=> 1, 2, 3 are the coins with average = 2.
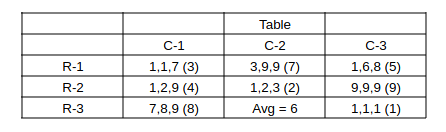
Considering bag (3,2)
Avg. = 6 => Sum = 18.
2 sacks more than 5 coins and ** => 2 sacks have 1 and 9 coins.
=> bag (3,2) has 1, c, 9 coins and c = 18 - 1 - 9 = 8
=> bag (3,2) has 1, 8, 9 coins with average = 6 coins.
==> Final required table, bracket number => average coins per sack in the bag.
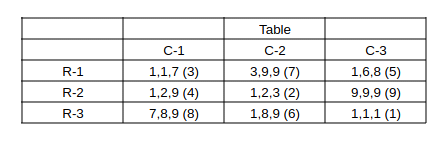
Bag (1,1) => 2 sacks with 1 coins, (2,1) => 1 sack, (2,2) => 1 sack, (3,2) => 1 sack, (1,3) => 1 sack, (3,3) => 3 sacks.
=> Total = 2 + 1 + 1 + 1 + 1 + 3 = 9 sacks.
Attention CAT Students!
To make sure you are not studying endlessly, EduRev has designed CAT study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in CAT.
|
Explore Courses for CAT exam
|
|
Similar CAT Doubts
Top Courses for CATView all
There are nine boxes arranged in a 3 × 3 array as shown in Tables 1 and 2. Each box contains three sacks. Each sack has a certain number of coins, between 1 and 9, both inclusive.The average number of coins per sack in the boxes are all distinct integers. The total number of coins in each row is the same. The total number of coins in each column is also the same.Table 1 gives information regarding the median of the numbers of coins in the three sacks in a box for some of the boxes. In Table 2 each box has a number which represents the number of sacks in that box having more than 5 coins. That number is followed by a * if the sacks in that box satisfy exactly one among the following three conditions, and it is followed by ** if two or more of these conditions are satisfied.(i) The minimum among the numbers of coins in the three sacks in the box is 1.(ii) The median of the numbers of coins in the three sacks is 1.(iii) The maximum among the numbers of coins in the three sacks in the box is 9.How many sacks have exactly one coin?Correct answer is '9'. Can you explain this answer?
Question Description
There are nine boxes arranged in a 3 × 3 array as shown in Tables 1 and 2. Each box contains three sacks. Each sack has a certain number of coins, between 1 and 9, both inclusive.The average number of coins per sack in the boxes are all distinct integers. The total number of coins in each row is the same. The total number of coins in each column is also the same.Table 1 gives information regarding the median of the numbers of coins in the three sacks in a box for some of the boxes. In Table 2 each box has a number which represents the number of sacks in that box having more than 5 coins. That number is followed by a * if the sacks in that box satisfy exactly one among the following three conditions, and it is followed by ** if two or more of these conditions are satisfied.(i) The minimum among the numbers of coins in the three sacks in the box is 1.(ii) The median of the numbers of coins in the three sacks is 1.(iii) The maximum among the numbers of coins in the three sacks in the box is 9.How many sacks have exactly one coin?Correct answer is '9'. Can you explain this answer? for CAT 2024 is part of CAT preparation. The Question and answers have been prepared according to the CAT exam syllabus. Information about There are nine boxes arranged in a 3 × 3 array as shown in Tables 1 and 2. Each box contains three sacks. Each sack has a certain number of coins, between 1 and 9, both inclusive.The average number of coins per sack in the boxes are all distinct integers. The total number of coins in each row is the same. The total number of coins in each column is also the same.Table 1 gives information regarding the median of the numbers of coins in the three sacks in a box for some of the boxes. In Table 2 each box has a number which represents the number of sacks in that box having more than 5 coins. That number is followed by a * if the sacks in that box satisfy exactly one among the following three conditions, and it is followed by ** if two or more of these conditions are satisfied.(i) The minimum among the numbers of coins in the three sacks in the box is 1.(ii) The median of the numbers of coins in the three sacks is 1.(iii) The maximum among the numbers of coins in the three sacks in the box is 9.How many sacks have exactly one coin?Correct answer is '9'. Can you explain this answer? covers all topics & solutions for CAT 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for There are nine boxes arranged in a 3 × 3 array as shown in Tables 1 and 2. Each box contains three sacks. Each sack has a certain number of coins, between 1 and 9, both inclusive.The average number of coins per sack in the boxes are all distinct integers. The total number of coins in each row is the same. The total number of coins in each column is also the same.Table 1 gives information regarding the median of the numbers of coins in the three sacks in a box for some of the boxes. In Table 2 each box has a number which represents the number of sacks in that box having more than 5 coins. That number is followed by a * if the sacks in that box satisfy exactly one among the following three conditions, and it is followed by ** if two or more of these conditions are satisfied.(i) The minimum among the numbers of coins in the three sacks in the box is 1.(ii) The median of the numbers of coins in the three sacks is 1.(iii) The maximum among the numbers of coins in the three sacks in the box is 9.How many sacks have exactly one coin?Correct answer is '9'. Can you explain this answer?.
There are nine boxes arranged in a 3 × 3 array as shown in Tables 1 and 2. Each box contains three sacks. Each sack has a certain number of coins, between 1 and 9, both inclusive.The average number of coins per sack in the boxes are all distinct integers. The total number of coins in each row is the same. The total number of coins in each column is also the same.Table 1 gives information regarding the median of the numbers of coins in the three sacks in a box for some of the boxes. In Table 2 each box has a number which represents the number of sacks in that box having more than 5 coins. That number is followed by a * if the sacks in that box satisfy exactly one among the following three conditions, and it is followed by ** if two or more of these conditions are satisfied.(i) The minimum among the numbers of coins in the three sacks in the box is 1.(ii) The median of the numbers of coins in the three sacks is 1.(iii) The maximum among the numbers of coins in the three sacks in the box is 9.How many sacks have exactly one coin?Correct answer is '9'. Can you explain this answer? for CAT 2024 is part of CAT preparation. The Question and answers have been prepared according to the CAT exam syllabus. Information about There are nine boxes arranged in a 3 × 3 array as shown in Tables 1 and 2. Each box contains three sacks. Each sack has a certain number of coins, between 1 and 9, both inclusive.The average number of coins per sack in the boxes are all distinct integers. The total number of coins in each row is the same. The total number of coins in each column is also the same.Table 1 gives information regarding the median of the numbers of coins in the three sacks in a box for some of the boxes. In Table 2 each box has a number which represents the number of sacks in that box having more than 5 coins. That number is followed by a * if the sacks in that box satisfy exactly one among the following three conditions, and it is followed by ** if two or more of these conditions are satisfied.(i) The minimum among the numbers of coins in the three sacks in the box is 1.(ii) The median of the numbers of coins in the three sacks is 1.(iii) The maximum among the numbers of coins in the three sacks in the box is 9.How many sacks have exactly one coin?Correct answer is '9'. Can you explain this answer? covers all topics & solutions for CAT 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for There are nine boxes arranged in a 3 × 3 array as shown in Tables 1 and 2. Each box contains three sacks. Each sack has a certain number of coins, between 1 and 9, both inclusive.The average number of coins per sack in the boxes are all distinct integers. The total number of coins in each row is the same. The total number of coins in each column is also the same.Table 1 gives information regarding the median of the numbers of coins in the three sacks in a box for some of the boxes. In Table 2 each box has a number which represents the number of sacks in that box having more than 5 coins. That number is followed by a * if the sacks in that box satisfy exactly one among the following three conditions, and it is followed by ** if two or more of these conditions are satisfied.(i) The minimum among the numbers of coins in the three sacks in the box is 1.(ii) The median of the numbers of coins in the three sacks is 1.(iii) The maximum among the numbers of coins in the three sacks in the box is 9.How many sacks have exactly one coin?Correct answer is '9'. Can you explain this answer?.
Solutions for There are nine boxes arranged in a 3 × 3 array as shown in Tables 1 and 2. Each box contains three sacks. Each sack has a certain number of coins, between 1 and 9, both inclusive.The average number of coins per sack in the boxes are all distinct integers. The total number of coins in each row is the same. The total number of coins in each column is also the same.Table 1 gives information regarding the median of the numbers of coins in the three sacks in a box for some of the boxes. In Table 2 each box has a number which represents the number of sacks in that box having more than 5 coins. That number is followed by a * if the sacks in that box satisfy exactly one among the following three conditions, and it is followed by ** if two or more of these conditions are satisfied.(i) The minimum among the numbers of coins in the three sacks in the box is 1.(ii) The median of the numbers of coins in the three sacks is 1.(iii) The maximum among the numbers of coins in the three sacks in the box is 9.How many sacks have exactly one coin?Correct answer is '9'. Can you explain this answer? in English & in Hindi are available as part of our courses for CAT.
Download more important topics, notes, lectures and mock test series for CAT Exam by signing up for free.
Here you can find the meaning of There are nine boxes arranged in a 3 × 3 array as shown in Tables 1 and 2. Each box contains three sacks. Each sack has a certain number of coins, between 1 and 9, both inclusive.The average number of coins per sack in the boxes are all distinct integers. The total number of coins in each row is the same. The total number of coins in each column is also the same.Table 1 gives information regarding the median of the numbers of coins in the three sacks in a box for some of the boxes. In Table 2 each box has a number which represents the number of sacks in that box having more than 5 coins. That number is followed by a * if the sacks in that box satisfy exactly one among the following three conditions, and it is followed by ** if two or more of these conditions are satisfied.(i) The minimum among the numbers of coins in the three sacks in the box is 1.(ii) The median of the numbers of coins in the three sacks is 1.(iii) The maximum among the numbers of coins in the three sacks in the box is 9.How many sacks have exactly one coin?Correct answer is '9'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
There are nine boxes arranged in a 3 × 3 array as shown in Tables 1 and 2. Each box contains three sacks. Each sack has a certain number of coins, between 1 and 9, both inclusive.The average number of coins per sack in the boxes are all distinct integers. The total number of coins in each row is the same. The total number of coins in each column is also the same.Table 1 gives information regarding the median of the numbers of coins in the three sacks in a box for some of the boxes. In Table 2 each box has a number which represents the number of sacks in that box having more than 5 coins. That number is followed by a * if the sacks in that box satisfy exactly one among the following three conditions, and it is followed by ** if two or more of these conditions are satisfied.(i) The minimum among the numbers of coins in the three sacks in the box is 1.(ii) The median of the numbers of coins in the three sacks is 1.(iii) The maximum among the numbers of coins in the three sacks in the box is 9.How many sacks have exactly one coin?Correct answer is '9'. Can you explain this answer?, a detailed solution for There are nine boxes arranged in a 3 × 3 array as shown in Tables 1 and 2. Each box contains three sacks. Each sack has a certain number of coins, between 1 and 9, both inclusive.The average number of coins per sack in the boxes are all distinct integers. The total number of coins in each row is the same. The total number of coins in each column is also the same.Table 1 gives information regarding the median of the numbers of coins in the three sacks in a box for some of the boxes. In Table 2 each box has a number which represents the number of sacks in that box having more than 5 coins. That number is followed by a * if the sacks in that box satisfy exactly one among the following three conditions, and it is followed by ** if two or more of these conditions are satisfied.(i) The minimum among the numbers of coins in the three sacks in the box is 1.(ii) The median of the numbers of coins in the three sacks is 1.(iii) The maximum among the numbers of coins in the three sacks in the box is 9.How many sacks have exactly one coin?Correct answer is '9'. Can you explain this answer? has been provided alongside types of There are nine boxes arranged in a 3 × 3 array as shown in Tables 1 and 2. Each box contains three sacks. Each sack has a certain number of coins, between 1 and 9, both inclusive.The average number of coins per sack in the boxes are all distinct integers. The total number of coins in each row is the same. The total number of coins in each column is also the same.Table 1 gives information regarding the median of the numbers of coins in the three sacks in a box for some of the boxes. In Table 2 each box has a number which represents the number of sacks in that box having more than 5 coins. That number is followed by a * if the sacks in that box satisfy exactly one among the following three conditions, and it is followed by ** if two or more of these conditions are satisfied.(i) The minimum among the numbers of coins in the three sacks in the box is 1.(ii) The median of the numbers of coins in the three sacks is 1.(iii) The maximum among the numbers of coins in the three sacks in the box is 9.How many sacks have exactly one coin?Correct answer is '9'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice There are nine boxes arranged in a 3 × 3 array as shown in Tables 1 and 2. Each box contains three sacks. Each sack has a certain number of coins, between 1 and 9, both inclusive.The average number of coins per sack in the boxes are all distinct integers. The total number of coins in each row is the same. The total number of coins in each column is also the same.Table 1 gives information regarding the median of the numbers of coins in the three sacks in a box for some of the boxes. In Table 2 each box has a number which represents the number of sacks in that box having more than 5 coins. That number is followed by a * if the sacks in that box satisfy exactly one among the following three conditions, and it is followed by ** if two or more of these conditions are satisfied.(i) The minimum among the numbers of coins in the three sacks in the box is 1.(ii) The median of the numbers of coins in the three sacks is 1.(iii) The maximum among the numbers of coins in the three sacks in the box is 9.How many sacks have exactly one coin?Correct answer is '9'. Can you explain this answer? tests, examples and also practice CAT tests.
|
|
Explore Courses for CAT exam
|
|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
Follow Us
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup