Class 11 Exam > Class 11 Questions > Use PMI : 1.1! +2.2! + 3.3! ___+ n.n! = (n+1)...
Start Learning for Free
Use PMI : 1.1! +2.2! + 3.3! ___+ n.n! = (n+1)! -1 ?
Most Upvoted Answer
Use PMI : 1.1! +2.2! + 3.3! ___+ n.n! = (n+1)! -1 ?
To prove any result through mathematical induction we have to follow three steps. If all the steps are satisfied we can say that it is proved through mathematical induction.
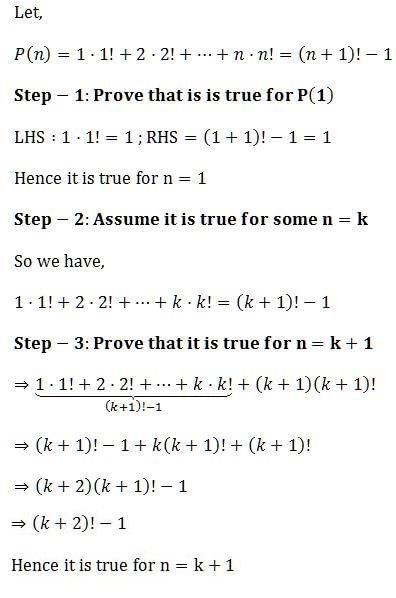

As all the steps are satisfied we have proved that 1.1! + 2.2! + ... + n.n! = (n + 1)! - 1 through mathematical induction.
Community Answer
Use PMI : 1.1! +2.2! + 3.3! ___+ n.n! = (n+1)! -1 ?
PMI and Factorials
PMI (Plus, Minus, Interesting) is a tool for critical thinking that helps to explore a statement or idea from different perspectives. In this case, we will use PMI to analyze the equation:
1.1! 2.2! 3.3! ___ n.n! = (n 1)! -1
Plus
The equation contains a pattern of factorials, which are mathematical functions that multiply a sequence of numbers.
Minus
The equation has a blank space that needs to be filled with a number that follows the pattern established by the sequence 1.1!, 2.2!, 3.3!, and n.n!.
Interesting
The equation relates the sum of a sequence of factorials to the difference between two factorials.
Solution
To solve the equation, we need to understand the pattern of the factorials in the sequence.
1.1! = 1
2.2! = 2 x 2 = 4
3.3! = 3 x 2 x 1 x 3 x 2 x 1 = 36
We notice that each factorial in the sequence is the product of a number and its corresponding decimal. For instance, 3.3! is the product of 3 x 2 x 1 x 0.3 x 0.2 x 0.1.
Therefore, the blank space in the equation should be filled with:
4.4! = 4 x 4 x 0.4 x 0.3 x 0.2 x 0.1 = 6.144
Now we can substitute the numbers in the equation and simplify it:
1! + 4! + 36 + 6.144 + n.n! = (n+1)! -1
We can simplify further by subtracting 1! from both sides:
4! + 36 + 6.144 + n.n! = (n+1)! -2
Finally, we can combine the terms on the left side and simplify the right side by factoring out (n+1):
40.144 + n.n! = (n+1)(n!) -2
Now we can solve for n by isolating the n! term and factoring out (n+1):
n.n! - (n+1)(n!) = -42.144
n!(n - (n+1)) = -42.144
n!(-1) = -42.144
n! = 42.144
However, this is not a valid solution because factorials are only defined for non-negative integers. Therefore, the equation has no solution.
Conclusion
Using PMI, we analyzed the equation 1.1! 2.2! 3.3! ___ n.n! = (n 1)! -1 and found that it had a pattern of factorials, a blank space that needed to be filled, and an interesting relationship between the sum of the sequence and the difference between two factorials. We solved the equation by understanding the pattern of the factorials and filling the blank space with 4.4!. We simplified the equation and found that
PMI (Plus, Minus, Interesting) is a tool for critical thinking that helps to explore a statement or idea from different perspectives. In this case, we will use PMI to analyze the equation:
1.1! 2.2! 3.3! ___ n.n! = (n 1)! -1
Plus
The equation contains a pattern of factorials, which are mathematical functions that multiply a sequence of numbers.
Minus
The equation has a blank space that needs to be filled with a number that follows the pattern established by the sequence 1.1!, 2.2!, 3.3!, and n.n!.
Interesting
The equation relates the sum of a sequence of factorials to the difference between two factorials.
Solution
To solve the equation, we need to understand the pattern of the factorials in the sequence.
1.1! = 1
2.2! = 2 x 2 = 4
3.3! = 3 x 2 x 1 x 3 x 2 x 1 = 36
We notice that each factorial in the sequence is the product of a number and its corresponding decimal. For instance, 3.3! is the product of 3 x 2 x 1 x 0.3 x 0.2 x 0.1.
Therefore, the blank space in the equation should be filled with:
4.4! = 4 x 4 x 0.4 x 0.3 x 0.2 x 0.1 = 6.144
Now we can substitute the numbers in the equation and simplify it:
1! + 4! + 36 + 6.144 + n.n! = (n+1)! -1
We can simplify further by subtracting 1! from both sides:
4! + 36 + 6.144 + n.n! = (n+1)! -2
Finally, we can combine the terms on the left side and simplify the right side by factoring out (n+1):
40.144 + n.n! = (n+1)(n!) -2
Now we can solve for n by isolating the n! term and factoring out (n+1):
n.n! - (n+1)(n!) = -42.144
n!(n - (n+1)) = -42.144
n!(-1) = -42.144
n! = 42.144
However, this is not a valid solution because factorials are only defined for non-negative integers. Therefore, the equation has no solution.
Conclusion
Using PMI, we analyzed the equation 1.1! 2.2! 3.3! ___ n.n! = (n 1)! -1 and found that it had a pattern of factorials, a blank space that needed to be filled, and an interesting relationship between the sum of the sequence and the difference between two factorials. We solved the equation by understanding the pattern of the factorials and filling the blank space with 4.4!. We simplified the equation and found that

|
Explore Courses for Class 11 exam
|

|
Similar Class 11 Doubts
Use PMI : 1.1! +2.2! + 3.3! ___+ n.n! = (n+1)! -1 ?
Question Description
Use PMI : 1.1! +2.2! + 3.3! ___+ n.n! = (n+1)! -1 ? for Class 11 2025 is part of Class 11 preparation. The Question and answers have been prepared according to the Class 11 exam syllabus. Information about Use PMI : 1.1! +2.2! + 3.3! ___+ n.n! = (n+1)! -1 ? covers all topics & solutions for Class 11 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Use PMI : 1.1! +2.2! + 3.3! ___+ n.n! = (n+1)! -1 ?.
Use PMI : 1.1! +2.2! + 3.3! ___+ n.n! = (n+1)! -1 ? for Class 11 2025 is part of Class 11 preparation. The Question and answers have been prepared according to the Class 11 exam syllabus. Information about Use PMI : 1.1! +2.2! + 3.3! ___+ n.n! = (n+1)! -1 ? covers all topics & solutions for Class 11 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Use PMI : 1.1! +2.2! + 3.3! ___+ n.n! = (n+1)! -1 ?.
Solutions for Use PMI : 1.1! +2.2! + 3.3! ___+ n.n! = (n+1)! -1 ? in English & in Hindi are available as part of our courses for Class 11.
Download more important topics, notes, lectures and mock test series for Class 11 Exam by signing up for free.
Here you can find the meaning of Use PMI : 1.1! +2.2! + 3.3! ___+ n.n! = (n+1)! -1 ? defined & explained in the simplest way possible. Besides giving the explanation of
Use PMI : 1.1! +2.2! + 3.3! ___+ n.n! = (n+1)! -1 ?, a detailed solution for Use PMI : 1.1! +2.2! + 3.3! ___+ n.n! = (n+1)! -1 ? has been provided alongside types of Use PMI : 1.1! +2.2! + 3.3! ___+ n.n! = (n+1)! -1 ? theory, EduRev gives you an
ample number of questions to practice Use PMI : 1.1! +2.2! + 3.3! ___+ n.n! = (n+1)! -1 ? tests, examples and also practice Class 11 tests.

|
Explore Courses for Class 11 exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.


























