Mechanical Engineering Exam > Mechanical Engineering Questions > In MLT θ system (T being time and θ...
Start Learning for Free
In MLT θ system (T being time and θ temperature), what is the dimension of thermal conductivity?
- a)ML−1T −1θ −3
- b)MLT −1θ −1
- c)MLθ −1T−3
- d)MLθ −1T−2
Correct answer is option 'C'. Can you explain this answer?
Verified Answer
In MLT θ system (T being time and θ temperature), what is th...
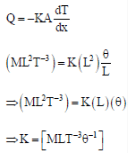
Most Upvoted Answer
In MLT θ system (T being time and θ temperature), what is th...
Thermal conductivity is a property that describes the ability of a material to conduct heat. It is defined as the amount of heat energy transferred through a unit area of a material per unit time, per unit temperature gradient. The MLT system is a system of units where M represents mass, L represents length, and T represents time.
The dimension of thermal conductivity can be determined by analyzing the equation that defines it. The equation for thermal conductivity is:
k = Q / (A * ΔT / Δx)
where:
- k is the thermal conductivity
- Q is the heat energy transferred
- A is the cross-sectional area of the material
- ΔT is the temperature difference across the material
- Δx is the thickness of the material
Breaking down the equation, we can determine the dimensions of each term:
- The dimension of heat energy transferred (Q) is ML2T-2. This can be determined by analyzing the equation for heat energy (Q = mcΔT), where m is mass, c is specific heat capacity, and ΔT is temperature difference. The dimension of specific heat capacity is L2T-2Θ-1.
- The dimension of cross-sectional area (A) is L2.
- The dimension of temperature difference (ΔT) is Θ.
- The dimension of thickness (Δx) is L.
By substituting the dimensions into the equation for thermal conductivity, we get:
k = (ML2T-2) / (L2 * Θ / L)
Simplifying the equation, we get:
k = ML1T-3
Therefore, the dimension of thermal conductivity in the MLT system is ML1T-3, which corresponds to option 'C' in the given choices.
The dimension of thermal conductivity can be determined by analyzing the equation that defines it. The equation for thermal conductivity is:
k = Q / (A * ΔT / Δx)
where:
- k is the thermal conductivity
- Q is the heat energy transferred
- A is the cross-sectional area of the material
- ΔT is the temperature difference across the material
- Δx is the thickness of the material
Breaking down the equation, we can determine the dimensions of each term:
- The dimension of heat energy transferred (Q) is ML2T-2. This can be determined by analyzing the equation for heat energy (Q = mcΔT), where m is mass, c is specific heat capacity, and ΔT is temperature difference. The dimension of specific heat capacity is L2T-2Θ-1.
- The dimension of cross-sectional area (A) is L2.
- The dimension of temperature difference (ΔT) is Θ.
- The dimension of thickness (Δx) is L.
By substituting the dimensions into the equation for thermal conductivity, we get:
k = (ML2T-2) / (L2 * Θ / L)
Simplifying the equation, we get:
k = ML1T-3
Therefore, the dimension of thermal conductivity in the MLT system is ML1T-3, which corresponds to option 'C' in the given choices.
Free Test
FREE
| Start Free Test |
Community Answer
In MLT θ system (T being time and θ temperature), what is th...
Unit of thermal conductivity is W/m*K watt is equal to joule per second joule is equal to Newton into metre so dimension of joule is M1L2T-2. SO DIMENSION OF WATT IS EQUAL TO M1L2T-3. THERMAL CONDUCTIVITY = M1L2T-3/((L1)*(theta1)) =. M1L1 K-1 T-3
|
Explore Courses for Mechanical Engineering exam
|
|
Similar Mechanical Engineering Doubts
Top Courses for Mechanical EngineeringView all
In MLT θ system (T being time and θ temperature), what is the dimension of thermal conductivity?a)ML−1T −1θ −3b)MLT −1θ −1c)MLθ −1T−3d)MLθ −1T−2Correct answer is option 'C'. Can you explain this answer?
Question Description
In MLT θ system (T being time and θ temperature), what is the dimension of thermal conductivity?a)ML−1T −1θ −3b)MLT −1θ −1c)MLθ −1T−3d)MLθ −1T−2Correct answer is option 'C'. Can you explain this answer? for Mechanical Engineering 2025 is part of Mechanical Engineering preparation. The Question and answers have been prepared according to the Mechanical Engineering exam syllabus. Information about In MLT θ system (T being time and θ temperature), what is the dimension of thermal conductivity?a)ML−1T −1θ −3b)MLT −1θ −1c)MLθ −1T−3d)MLθ −1T−2Correct answer is option 'C'. Can you explain this answer? covers all topics & solutions for Mechanical Engineering 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for In MLT θ system (T being time and θ temperature), what is the dimension of thermal conductivity?a)ML−1T −1θ −3b)MLT −1θ −1c)MLθ −1T−3d)MLθ −1T−2Correct answer is option 'C'. Can you explain this answer?.
In MLT θ system (T being time and θ temperature), what is the dimension of thermal conductivity?a)ML−1T −1θ −3b)MLT −1θ −1c)MLθ −1T−3d)MLθ −1T−2Correct answer is option 'C'. Can you explain this answer? for Mechanical Engineering 2025 is part of Mechanical Engineering preparation. The Question and answers have been prepared according to the Mechanical Engineering exam syllabus. Information about In MLT θ system (T being time and θ temperature), what is the dimension of thermal conductivity?a)ML−1T −1θ −3b)MLT −1θ −1c)MLθ −1T−3d)MLθ −1T−2Correct answer is option 'C'. Can you explain this answer? covers all topics & solutions for Mechanical Engineering 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for In MLT θ system (T being time and θ temperature), what is the dimension of thermal conductivity?a)ML−1T −1θ −3b)MLT −1θ −1c)MLθ −1T−3d)MLθ −1T−2Correct answer is option 'C'. Can you explain this answer?.
Solutions for In MLT θ system (T being time and θ temperature), what is the dimension of thermal conductivity?a)ML−1T −1θ −3b)MLT −1θ −1c)MLθ −1T−3d)MLθ −1T−2Correct answer is option 'C'. Can you explain this answer? in English & in Hindi are available as part of our courses for Mechanical Engineering.
Download more important topics, notes, lectures and mock test series for Mechanical Engineering Exam by signing up for free.
Here you can find the meaning of In MLT θ system (T being time and θ temperature), what is the dimension of thermal conductivity?a)ML−1T −1θ −3b)MLT −1θ −1c)MLθ −1T−3d)MLθ −1T−2Correct answer is option 'C'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
In MLT θ system (T being time and θ temperature), what is the dimension of thermal conductivity?a)ML−1T −1θ −3b)MLT −1θ −1c)MLθ −1T−3d)MLθ −1T−2Correct answer is option 'C'. Can you explain this answer?, a detailed solution for In MLT θ system (T being time and θ temperature), what is the dimension of thermal conductivity?a)ML−1T −1θ −3b)MLT −1θ −1c)MLθ −1T−3d)MLθ −1T−2Correct answer is option 'C'. Can you explain this answer? has been provided alongside types of In MLT θ system (T being time and θ temperature), what is the dimension of thermal conductivity?a)ML−1T −1θ −3b)MLT −1θ −1c)MLθ −1T−3d)MLθ −1T−2Correct answer is option 'C'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice In MLT θ system (T being time and θ temperature), what is the dimension of thermal conductivity?a)ML−1T −1θ −3b)MLT −1θ −1c)MLθ −1T−3d)MLθ −1T−2Correct answer is option 'C'. Can you explain this answer? tests, examples and also practice Mechanical Engineering tests.
|
|
Explore Courses for Mechanical Engineering exam
|
|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup