Chemistry Exam > Chemistry Questions > The cubic unit cell of aluminium (molar mass ...
Start Learning for Free
The cubic unit cell of aluminium (molar mass 27.0 g/mol) has an edge length of 405 pm. Its density is 2.70 g/cc. The type of unit cell is:
- a)Primitive
- b)Face centred
- c)Bod y centred
- d)End centred
Correct answer is option 'D'. Can you explain this answer?
Verified Answer
The cubic unit cell of aluminium (molar mass 27.0 g/mol) has an edge l...
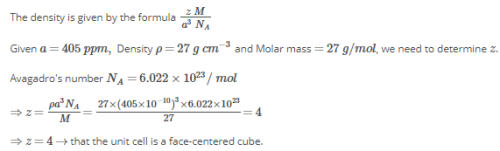
 This question is part of UPSC exam. View all Chemistry courses
This question is part of UPSC exam. View all Chemistry courses
Most Upvoted Answer
The cubic unit cell of aluminium (molar mass 27.0 g/mol) has an edge l...
Unit Cell and Density
A unit cell is the smallest repeating unit of a crystal lattice structure. It represents the arrangement of atoms or ions in a crystal. The density of a material is defined as its mass per unit volume.
Given Data:
- Molar mass of aluminium (Al) = 27.0 g/mol
- Edge length of cubic unit cell (a) = 405 pm = 405 × 10^(-12) m
- Density of aluminium (ρ) = 2.70 g/cc
Determining the Type of Unit Cell
To determine the type of unit cell for aluminium, we need to compare the edge length of the unit cell with the atomic radius of aluminium.
Lattice Parameter
The lattice parameter, denoted by 'a', is the distance between two adjacent lattice points in a crystal lattice. In a cubic unit cell, the edge length (a) is equal to the lattice parameter.
Atomic Radius
The atomic radius is the distance from the nucleus of an atom to its outermost electron shell. For aluminium, the atomic radius is approximately 143 pm.
Calculations:
1. Convert the edge length from picometers (pm) to meters (m):
405 pm = 405 × 10^(-12) m
2. Calculate the atomic radius of aluminium in meters:
143 pm = 143 × 10^(-12) m
3. Compare the atomic radius with the edge length of the unit cell:
If the edge length of the unit cell is greater than 4 times the atomic radius, it is an end-centered unit cell.
Calculating the Type of Unit Cell
The edge length of the unit cell (a) is given as 405 × 10^(-12) m, and the atomic radius of aluminium is approximately 143 × 10^(-12) m.
The edge length of the unit cell is greater than 4 times the atomic radius:
405 × 10^(-12) m > 4 * (143 × 10^(-12) m)
Therefore, the type of unit cell for aluminium is end-centered (d).
Conclusion
The cubic unit cell of aluminium, with an edge length of 405 pm, is an end-centered unit cell.
A unit cell is the smallest repeating unit of a crystal lattice structure. It represents the arrangement of atoms or ions in a crystal. The density of a material is defined as its mass per unit volume.
Given Data:
- Molar mass of aluminium (Al) = 27.0 g/mol
- Edge length of cubic unit cell (a) = 405 pm = 405 × 10^(-12) m
- Density of aluminium (ρ) = 2.70 g/cc
Determining the Type of Unit Cell
To determine the type of unit cell for aluminium, we need to compare the edge length of the unit cell with the atomic radius of aluminium.
Lattice Parameter
The lattice parameter, denoted by 'a', is the distance between two adjacent lattice points in a crystal lattice. In a cubic unit cell, the edge length (a) is equal to the lattice parameter.
Atomic Radius
The atomic radius is the distance from the nucleus of an atom to its outermost electron shell. For aluminium, the atomic radius is approximately 143 pm.
Calculations:
1. Convert the edge length from picometers (pm) to meters (m):
405 pm = 405 × 10^(-12) m
2. Calculate the atomic radius of aluminium in meters:
143 pm = 143 × 10^(-12) m
3. Compare the atomic radius with the edge length of the unit cell:
If the edge length of the unit cell is greater than 4 times the atomic radius, it is an end-centered unit cell.
Calculating the Type of Unit Cell
The edge length of the unit cell (a) is given as 405 × 10^(-12) m, and the atomic radius of aluminium is approximately 143 × 10^(-12) m.
The edge length of the unit cell is greater than 4 times the atomic radius:
405 × 10^(-12) m > 4 * (143 × 10^(-12) m)
Therefore, the type of unit cell for aluminium is end-centered (d).
Conclusion
The cubic unit cell of aluminium, with an edge length of 405 pm, is an end-centered unit cell.

|
Explore Courses for Chemistry exam
|

|
Similar Chemistry Doubts
The cubic unit cell of aluminium (molar mass 27.0 g/mol) has an edge length of 405 pm. Its density is 2.70 g/cc. The type of unit cell is:a)Primitiveb)Face centredc)Bod y centredd)End centredCorrect answer is option 'D'. Can you explain this answer?
Question Description
The cubic unit cell of aluminium (molar mass 27.0 g/mol) has an edge length of 405 pm. Its density is 2.70 g/cc. The type of unit cell is:a)Primitiveb)Face centredc)Bod y centredd)End centredCorrect answer is option 'D'. Can you explain this answer? for Chemistry 2024 is part of Chemistry preparation. The Question and answers have been prepared according to the Chemistry exam syllabus. Information about The cubic unit cell of aluminium (molar mass 27.0 g/mol) has an edge length of 405 pm. Its density is 2.70 g/cc. The type of unit cell is:a)Primitiveb)Face centredc)Bod y centredd)End centredCorrect answer is option 'D'. Can you explain this answer? covers all topics & solutions for Chemistry 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The cubic unit cell of aluminium (molar mass 27.0 g/mol) has an edge length of 405 pm. Its density is 2.70 g/cc. The type of unit cell is:a)Primitiveb)Face centredc)Bod y centredd)End centredCorrect answer is option 'D'. Can you explain this answer?.
The cubic unit cell of aluminium (molar mass 27.0 g/mol) has an edge length of 405 pm. Its density is 2.70 g/cc. The type of unit cell is:a)Primitiveb)Face centredc)Bod y centredd)End centredCorrect answer is option 'D'. Can you explain this answer? for Chemistry 2024 is part of Chemistry preparation. The Question and answers have been prepared according to the Chemistry exam syllabus. Information about The cubic unit cell of aluminium (molar mass 27.0 g/mol) has an edge length of 405 pm. Its density is 2.70 g/cc. The type of unit cell is:a)Primitiveb)Face centredc)Bod y centredd)End centredCorrect answer is option 'D'. Can you explain this answer? covers all topics & solutions for Chemistry 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for The cubic unit cell of aluminium (molar mass 27.0 g/mol) has an edge length of 405 pm. Its density is 2.70 g/cc. The type of unit cell is:a)Primitiveb)Face centredc)Bod y centredd)End centredCorrect answer is option 'D'. Can you explain this answer?.
Solutions for The cubic unit cell of aluminium (molar mass 27.0 g/mol) has an edge length of 405 pm. Its density is 2.70 g/cc. The type of unit cell is:a)Primitiveb)Face centredc)Bod y centredd)End centredCorrect answer is option 'D'. Can you explain this answer? in English & in Hindi are available as part of our courses for Chemistry.
Download more important topics, notes, lectures and mock test series for Chemistry Exam by signing up for free.
Here you can find the meaning of The cubic unit cell of aluminium (molar mass 27.0 g/mol) has an edge length of 405 pm. Its density is 2.70 g/cc. The type of unit cell is:a)Primitiveb)Face centredc)Bod y centredd)End centredCorrect answer is option 'D'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
The cubic unit cell of aluminium (molar mass 27.0 g/mol) has an edge length of 405 pm. Its density is 2.70 g/cc. The type of unit cell is:a)Primitiveb)Face centredc)Bod y centredd)End centredCorrect answer is option 'D'. Can you explain this answer?, a detailed solution for The cubic unit cell of aluminium (molar mass 27.0 g/mol) has an edge length of 405 pm. Its density is 2.70 g/cc. The type of unit cell is:a)Primitiveb)Face centredc)Bod y centredd)End centredCorrect answer is option 'D'. Can you explain this answer? has been provided alongside types of The cubic unit cell of aluminium (molar mass 27.0 g/mol) has an edge length of 405 pm. Its density is 2.70 g/cc. The type of unit cell is:a)Primitiveb)Face centredc)Bod y centredd)End centredCorrect answer is option 'D'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice The cubic unit cell of aluminium (molar mass 27.0 g/mol) has an edge length of 405 pm. Its density is 2.70 g/cc. The type of unit cell is:a)Primitiveb)Face centredc)Bod y centredd)End centredCorrect answer is option 'D'. Can you explain this answer? tests, examples and also practice Chemistry tests.

|
Explore Courses for Chemistry exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.



















