CA Foundation Exam > CA Foundation Questions > Indifference curves never intersect each othe...
Start Learning for Free
Indifference curves never intersect each other due to:
- a)Different levels of satisfaction
- b)Same levels of satisfaction
- c)Convex to origin
- d)Concave to origin
Correct answer is option 'A'. Can you explain this answer?
| FREE This question is part of | Download PDF Attempt this Test |
Verified Answer
Indifference curves never intersect each other due to:a)Different leve...
Indifference Curves cannot Intersect Each Other:
The indifference curves cannot intersect each other. It is because at the point of tangency, the higher curve will give as much as of the two commodities as is given by the lower indifference curve. This is absurd and impossible.
Diagram:
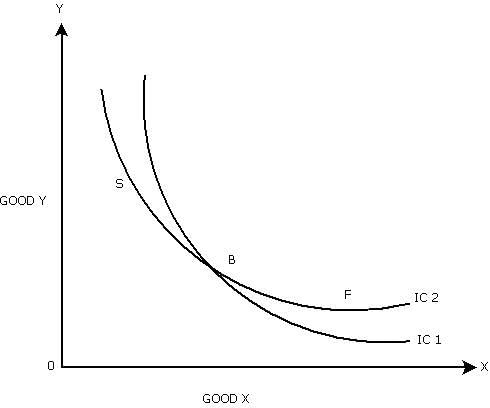
In the above diagram, two indifference curves are showing cutting each other at point B. The combinations represented by points B and F given equal satisfaction to the consumer because both lie on the same indifference curve IC2. Similarly the combinations shows by points B and E on indifference curve IC1 give equal satisfaction top the consumer.
If combination F is equal to combination B in terms of satisfaction and combination E is equal to combination B in satisfaction. It follows that the combination F will be equivalent to E in terms of satisfaction. This conclusion looks quite funny because combination F on IC2 contains more of good Y (wheat) than combination which gives more satisfaction to the consumer. We, therefore, conclude that indifference curves cannot cut each other.
Most Upvoted Answer
Indifference curves never intersect each other due to:a)Different leve...
Explanation:
Indifference curves represent the different combinations of two goods that provide the same level of satisfaction to a consumer. These curves are drawn on a graph with one good on the x-axis and the other good on the y-axis.
The following are the reasons why indifference curves never intersect each other:
Different levels of satisfaction: Each indifference curve represents a different level of satisfaction for the consumer. As we move away from the origin, the level of satisfaction increases. Therefore, it is not possible for two indifference curves to intersect because they represent different levels of satisfaction.
Convex to origin: Indifference curves are generally convex to the origin. This means that as we move away from the origin, the marginal rate of substitution (MRS) between the two goods changes. If two indifference curves intersected, it would mean that the MRS is the same at the point of intersection, which is not possible.
Concave to origin: If the indifference curves were concave to the origin, it would be possible for them to intersect. However, this is not the case, as most indifference curves are convex to the origin.
Therefore, the correct answer is option A, which states that indifference curves never intersect each other due to different levels of satisfaction.
Indifference curves represent the different combinations of two goods that provide the same level of satisfaction to a consumer. These curves are drawn on a graph with one good on the x-axis and the other good on the y-axis.
The following are the reasons why indifference curves never intersect each other:
Different levels of satisfaction: Each indifference curve represents a different level of satisfaction for the consumer. As we move away from the origin, the level of satisfaction increases. Therefore, it is not possible for two indifference curves to intersect because they represent different levels of satisfaction.
Convex to origin: Indifference curves are generally convex to the origin. This means that as we move away from the origin, the marginal rate of substitution (MRS) between the two goods changes. If two indifference curves intersected, it would mean that the MRS is the same at the point of intersection, which is not possible.
Concave to origin: If the indifference curves were concave to the origin, it would be possible for them to intersect. However, this is not the case, as most indifference curves are convex to the origin.
Therefore, the correct answer is option A, which states that indifference curves never intersect each other due to different levels of satisfaction.
Free Test
FREE
| Start Free Test |
Community Answer
Indifference curves never intersect each other due to:a)Different leve...
What do you think which opt is correct? and why
Attention CA Foundation Students!
To make sure you are not studying endlessly, EduRev has designed CA Foundation study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in CA Foundation.
|
Explore Courses for CA Foundation exam
|
|
Similar CA Foundation Doubts
Top Courses for CA FoundationView all
Indifference curves never intersect each other due to:a)Different levels of satisfactionb)Same levels of satisfactionc)Convex to origind)Concave to originCorrect answer is option 'A'. Can you explain this answer?
Question Description
Indifference curves never intersect each other due to:a)Different levels of satisfactionb)Same levels of satisfactionc)Convex to origind)Concave to originCorrect answer is option 'A'. Can you explain this answer? for CA Foundation 2024 is part of CA Foundation preparation. The Question and answers have been prepared according to the CA Foundation exam syllabus. Information about Indifference curves never intersect each other due to:a)Different levels of satisfactionb)Same levels of satisfactionc)Convex to origind)Concave to originCorrect answer is option 'A'. Can you explain this answer? covers all topics & solutions for CA Foundation 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Indifference curves never intersect each other due to:a)Different levels of satisfactionb)Same levels of satisfactionc)Convex to origind)Concave to originCorrect answer is option 'A'. Can you explain this answer?.
Indifference curves never intersect each other due to:a)Different levels of satisfactionb)Same levels of satisfactionc)Convex to origind)Concave to originCorrect answer is option 'A'. Can you explain this answer? for CA Foundation 2024 is part of CA Foundation preparation. The Question and answers have been prepared according to the CA Foundation exam syllabus. Information about Indifference curves never intersect each other due to:a)Different levels of satisfactionb)Same levels of satisfactionc)Convex to origind)Concave to originCorrect answer is option 'A'. Can you explain this answer? covers all topics & solutions for CA Foundation 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Indifference curves never intersect each other due to:a)Different levels of satisfactionb)Same levels of satisfactionc)Convex to origind)Concave to originCorrect answer is option 'A'. Can you explain this answer?.
Solutions for Indifference curves never intersect each other due to:a)Different levels of satisfactionb)Same levels of satisfactionc)Convex to origind)Concave to originCorrect answer is option 'A'. Can you explain this answer? in English & in Hindi are available as part of our courses for CA Foundation.
Download more important topics, notes, lectures and mock test series for CA Foundation Exam by signing up for free.
Here you can find the meaning of Indifference curves never intersect each other due to:a)Different levels of satisfactionb)Same levels of satisfactionc)Convex to origind)Concave to originCorrect answer is option 'A'. Can you explain this answer? defined & explained in the simplest way possible. Besides giving the explanation of
Indifference curves never intersect each other due to:a)Different levels of satisfactionb)Same levels of satisfactionc)Convex to origind)Concave to originCorrect answer is option 'A'. Can you explain this answer?, a detailed solution for Indifference curves never intersect each other due to:a)Different levels of satisfactionb)Same levels of satisfactionc)Convex to origind)Concave to originCorrect answer is option 'A'. Can you explain this answer? has been provided alongside types of Indifference curves never intersect each other due to:a)Different levels of satisfactionb)Same levels of satisfactionc)Convex to origind)Concave to originCorrect answer is option 'A'. Can you explain this answer? theory, EduRev gives you an
ample number of questions to practice Indifference curves never intersect each other due to:a)Different levels of satisfactionb)Same levels of satisfactionc)Convex to origind)Concave to originCorrect answer is option 'A'. Can you explain this answer? tests, examples and also practice CA Foundation tests.
|
|
Explore Courses for CA Foundation exam
|
|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.
|
© EduRev
|
Education Revolution
|
|
Signup to see your scores
go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup