Class 12 Exam > Class 12 Questions > A ball of mass 0.25 kg attached to the ends o...
Start Learning for Free
A ball of mass 0.25 kg attached to the ends of a string of length 1.96 m is rotating in a horizontal circle the string will break if tension is more than 25n. what is the maximum speed with which the ball can br rotated?
Verified Answer
A ball of mass 0.25 kg attached to the ends of a string of length 1.96...
Length of String, l = 1.96 m
Mass of Ball, m = 0.25 Kg
Maximum Tension of String, Tmax = 25 N
Let the speed of the ball is v m/s
In the horizontal circular motion of the ball tension in the string is balanced by the centrifugal force (mv^2/l) and hence the maximum tension in the string will be for the maximum speed of the ball (since m and l are fixed).
Therefore,
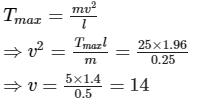
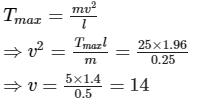
Therefore, maximum speed of the ball will be 14m/s
 This question is part of UPSC exam. View all Class 12 courses
This question is part of UPSC exam. View all Class 12 courses
Most Upvoted Answer
A ball of mass 0.25 kg attached to the ends of a string of length 1.96...
Problem:
A ball of mass 0.25 kg is attached to the ends of a string of length 1.96 m and is rotating in a horizontal circle. The string will break if the tension is more than 25 N. What is the maximum speed with which the ball can be rotated?
Solution:
To find the maximum speed at which the ball can be rotated, we need to determine the maximum tension in the string. We can use the centripetal force equation to relate the tension in the string with the mass, speed, and radius of the circular motion.
Centripetal Force:
The centripetal force is the force that keeps an object moving in a circular path. In this case, the tension in the string provides the centripetal force.
The centripetal force (F) can be calculated using the equation:
F = (m * v^2) / r
Where:
F - Centripetal force
m - Mass of the ball
v - Velocity of the ball
r - Radius of the circular path
Maximum Tension:
The tension in the string should not exceed 25 N, as the string will break if it surpasses this limit.
Therefore, we can set the maximum tension (Tmax) equal to 25 N and solve for the maximum velocity (vmax):
Tmax = (m * vmax^2) / r
Calculating the Maximum Speed:
Rearranging the equation, we can solve for vmax:
vmax^2 = (Tmax * r) / m
vmax = sqrt((Tmax * r) / m)
Substituting the given values:
Tmax = 25 N
r = 1.96 m
m = 0.25 kg
vmax = sqrt((25 * 1.96) / 0.25)
vmax = sqrt(490 / 0.25)
vmax = sqrt(1960)
vmax ≈ 44.27 m/s
Answer:
The maximum speed with which the ball can be rotated is approximately 44.27 m/s.
A ball of mass 0.25 kg is attached to the ends of a string of length 1.96 m and is rotating in a horizontal circle. The string will break if the tension is more than 25 N. What is the maximum speed with which the ball can be rotated?
Solution:
To find the maximum speed at which the ball can be rotated, we need to determine the maximum tension in the string. We can use the centripetal force equation to relate the tension in the string with the mass, speed, and radius of the circular motion.
Centripetal Force:
The centripetal force is the force that keeps an object moving in a circular path. In this case, the tension in the string provides the centripetal force.
The centripetal force (F) can be calculated using the equation:
F = (m * v^2) / r
Where:
F - Centripetal force
m - Mass of the ball
v - Velocity of the ball
r - Radius of the circular path
Maximum Tension:
The tension in the string should not exceed 25 N, as the string will break if it surpasses this limit.
Therefore, we can set the maximum tension (Tmax) equal to 25 N and solve for the maximum velocity (vmax):
Tmax = (m * vmax^2) / r
Calculating the Maximum Speed:
Rearranging the equation, we can solve for vmax:
vmax^2 = (Tmax * r) / m
vmax = sqrt((Tmax * r) / m)
Substituting the given values:
Tmax = 25 N
r = 1.96 m
m = 0.25 kg
vmax = sqrt((25 * 1.96) / 0.25)
vmax = sqrt(490 / 0.25)
vmax = sqrt(1960)
vmax ≈ 44.27 m/s
Answer:
The maximum speed with which the ball can be rotated is approximately 44.27 m/s.

|
Explore Courses for Class 12 exam
|

|
Similar Class 12 Doubts
A ball of mass 0.25 kg attached to the ends of a string of length 1.96 m is rotating in a horizontal circle the string will break if tension is more than 25n. what is the maximum speed with which the ball can br rotated?
Question Description
A ball of mass 0.25 kg attached to the ends of a string of length 1.96 m is rotating in a horizontal circle the string will break if tension is more than 25n. what is the maximum speed with which the ball can br rotated? for Class 12 2024 is part of Class 12 preparation. The Question and answers have been prepared according to the Class 12 exam syllabus. Information about A ball of mass 0.25 kg attached to the ends of a string of length 1.96 m is rotating in a horizontal circle the string will break if tension is more than 25n. what is the maximum speed with which the ball can br rotated? covers all topics & solutions for Class 12 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A ball of mass 0.25 kg attached to the ends of a string of length 1.96 m is rotating in a horizontal circle the string will break if tension is more than 25n. what is the maximum speed with which the ball can br rotated?.
A ball of mass 0.25 kg attached to the ends of a string of length 1.96 m is rotating in a horizontal circle the string will break if tension is more than 25n. what is the maximum speed with which the ball can br rotated? for Class 12 2024 is part of Class 12 preparation. The Question and answers have been prepared according to the Class 12 exam syllabus. Information about A ball of mass 0.25 kg attached to the ends of a string of length 1.96 m is rotating in a horizontal circle the string will break if tension is more than 25n. what is the maximum speed with which the ball can br rotated? covers all topics & solutions for Class 12 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for A ball of mass 0.25 kg attached to the ends of a string of length 1.96 m is rotating in a horizontal circle the string will break if tension is more than 25n. what is the maximum speed with which the ball can br rotated?.
Solutions for A ball of mass 0.25 kg attached to the ends of a string of length 1.96 m is rotating in a horizontal circle the string will break if tension is more than 25n. what is the maximum speed with which the ball can br rotated? in English & in Hindi are available as part of our courses for Class 12.
Download more important topics, notes, lectures and mock test series for Class 12 Exam by signing up for free.
Here you can find the meaning of A ball of mass 0.25 kg attached to the ends of a string of length 1.96 m is rotating in a horizontal circle the string will break if tension is more than 25n. what is the maximum speed with which the ball can br rotated? defined & explained in the simplest way possible. Besides giving the explanation of
A ball of mass 0.25 kg attached to the ends of a string of length 1.96 m is rotating in a horizontal circle the string will break if tension is more than 25n. what is the maximum speed with which the ball can br rotated?, a detailed solution for A ball of mass 0.25 kg attached to the ends of a string of length 1.96 m is rotating in a horizontal circle the string will break if tension is more than 25n. what is the maximum speed with which the ball can br rotated? has been provided alongside types of A ball of mass 0.25 kg attached to the ends of a string of length 1.96 m is rotating in a horizontal circle the string will break if tension is more than 25n. what is the maximum speed with which the ball can br rotated? theory, EduRev gives you an
ample number of questions to practice A ball of mass 0.25 kg attached to the ends of a string of length 1.96 m is rotating in a horizontal circle the string will break if tension is more than 25n. what is the maximum speed with which the ball can br rotated? tests, examples and also practice Class 12 tests.

|
Explore Courses for Class 12 exam
|

|
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.



















