Class 7 Exam > Class 7 Questions > but what is negative numbers Related: How ar...
Start Learning for Free
but what is negative numbers
Verified Answer
but what is negative numbers Related: How are Expressions Formed?- Va...
The first thing you should do is take a look at a number line.
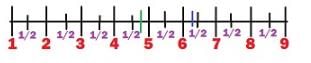

Less than zero.
(Positive means more than zero. Zero is neither negative nor positive.)
A negative number is written with a minus sign in front
 This question is part of UPSC exam. View all Class 7 courses
This question is part of UPSC exam. View all Class 7 courses
Most Upvoted Answer
but what is negative numbers Related: How are Expressions Formed?- Va...
The numbers less than zero and they have minus symbol in front of the number.......This is negative numbers
EX: -5, -10 , -1000, -3783, -838 and many more !!!
EX: -5, -10 , -1000, -3783, -838 and many more !!!
Community Answer
but what is negative numbers Related: How are Expressions Formed?- Va...
Negative numbers are an essential concept in mathematics that represents values less than zero. They are used to describe quantities that involve a decrease or a deficit. The concept of negative numbers extends the number line beyond zero, allowing for the representation of values in both directions.
Understanding Negative Numbers:
Negative numbers are introduced as a way to express values that are less than zero. On the number line, positive numbers are located to the right of zero, while negative numbers are located to the left. For example, -3 is a negative number, represented to the left of zero on the number line.
Significance of Negative Numbers:
Negative numbers play a crucial role in various mathematical operations, including addition, subtraction, multiplication, and division. They allow us to model real-life situations that involve debts, temperatures below zero, and other scenarios where quantities go below a reference point.
Representation:
Negative numbers are typically represented with a minus sign (-) placed before the number. For example, -5 represents a negative value of 5.
Operations with Negative Numbers:
1. Addition: When adding positive and negative numbers, we can think of it as combining quantities in opposite directions. For example, 3 + (-5) can be visualized as starting at 3 and moving 5 units to the left, resulting in -2.
2. Subtraction: Subtracting a negative number is equivalent to adding its positive counterpart. For example, 7 - (-3) can be rewritten as 7 + 3, resulting in 10.
3. Multiplication: When multiplying two negative numbers, the result is positive. For example, (-2) * (-4) equals 8. Multiplying a positive number by a negative number results in a negative value. For instance, 3 * (-6) equals -18.
4. Division: Dividing a negative number by a positive number yields a negative result. For example, (-12) ÷ 3 equals -4. Dividing a positive number by a negative number results in a negative quotient. For instance, 20 ÷ (-5) equals -4.
Applications:
Negative numbers have various real-world applications, such as:
- Representing temperatures below zero
- Describing debts or financial losses
- Calculating elevations above or below sea level
- Indicating a decrease in stock prices or temperature
Conclusion:
Understanding negative numbers is crucial for solving mathematical problems and representing real-life scenarios that involve values less than zero. They allow for the representation of quantities in both positive and negative directions, expanding the number line beyond zero. Negative numbers are used in various mathematical operations and have practical applications in fields such as finance, science, and weather forecasting.
Understanding Negative Numbers:
Negative numbers are introduced as a way to express values that are less than zero. On the number line, positive numbers are located to the right of zero, while negative numbers are located to the left. For example, -3 is a negative number, represented to the left of zero on the number line.
Significance of Negative Numbers:
Negative numbers play a crucial role in various mathematical operations, including addition, subtraction, multiplication, and division. They allow us to model real-life situations that involve debts, temperatures below zero, and other scenarios where quantities go below a reference point.
Representation:
Negative numbers are typically represented with a minus sign (-) placed before the number. For example, -5 represents a negative value of 5.
Operations with Negative Numbers:
1. Addition: When adding positive and negative numbers, we can think of it as combining quantities in opposite directions. For example, 3 + (-5) can be visualized as starting at 3 and moving 5 units to the left, resulting in -2.
2. Subtraction: Subtracting a negative number is equivalent to adding its positive counterpart. For example, 7 - (-3) can be rewritten as 7 + 3, resulting in 10.
3. Multiplication: When multiplying two negative numbers, the result is positive. For example, (-2) * (-4) equals 8. Multiplying a positive number by a negative number results in a negative value. For instance, 3 * (-6) equals -18.
4. Division: Dividing a negative number by a positive number yields a negative result. For example, (-12) ÷ 3 equals -4. Dividing a positive number by a negative number results in a negative quotient. For instance, 20 ÷ (-5) equals -4.
Applications:
Negative numbers have various real-world applications, such as:
- Representing temperatures below zero
- Describing debts or financial losses
- Calculating elevations above or below sea level
- Indicating a decrease in stock prices or temperature
Conclusion:
Understanding negative numbers is crucial for solving mathematical problems and representing real-life scenarios that involve values less than zero. They allow for the representation of quantities in both positive and negative directions, expanding the number line beyond zero. Negative numbers are used in various mathematical operations and have practical applications in fields such as finance, science, and weather forecasting.

|
Explore Courses for Class 7 exam
|

|
Similar Class 7 Doubts
Question Description
but what is negative numbers Related: How are Expressions Formed?- Variables and Terms, Algebraic Expression, Class 7 Math for Class 7 2025 is part of Class 7 preparation. The Question and answers have been prepared according to the Class 7 exam syllabus. Information about but what is negative numbers Related: How are Expressions Formed?- Variables and Terms, Algebraic Expression, Class 7 Math covers all topics & solutions for Class 7 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for but what is negative numbers Related: How are Expressions Formed?- Variables and Terms, Algebraic Expression, Class 7 Math.
but what is negative numbers Related: How are Expressions Formed?- Variables and Terms, Algebraic Expression, Class 7 Math for Class 7 2025 is part of Class 7 preparation. The Question and answers have been prepared according to the Class 7 exam syllabus. Information about but what is negative numbers Related: How are Expressions Formed?- Variables and Terms, Algebraic Expression, Class 7 Math covers all topics & solutions for Class 7 2025 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for but what is negative numbers Related: How are Expressions Formed?- Variables and Terms, Algebraic Expression, Class 7 Math.
Solutions for but what is negative numbers Related: How are Expressions Formed?- Variables and Terms, Algebraic Expression, Class 7 Math in English & in Hindi are available as part of our courses for Class 7.
Download more important topics, notes, lectures and mock test series for Class 7 Exam by signing up for free.
Here you can find the meaning of but what is negative numbers Related: How are Expressions Formed?- Variables and Terms, Algebraic Expression, Class 7 Math defined & explained in the simplest way possible. Besides giving the explanation of
but what is negative numbers Related: How are Expressions Formed?- Variables and Terms, Algebraic Expression, Class 7 Math, a detailed solution for but what is negative numbers Related: How are Expressions Formed?- Variables and Terms, Algebraic Expression, Class 7 Math has been provided alongside types of but what is negative numbers Related: How are Expressions Formed?- Variables and Terms, Algebraic Expression, Class 7 Math theory, EduRev gives you an
ample number of questions to practice but what is negative numbers Related: How are Expressions Formed?- Variables and Terms, Algebraic Expression, Class 7 Math tests, examples and also practice Class 7 tests.

|
Explore Courses for Class 7 exam
|

|
Signup to solve all Doubts
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.





















