Class 9 Exam > Class 9 Questions > Draw the graph for the following equation 5x-...
Start Learning for Free
Draw the graph for the following equation 5x-3y 10=0 and 5x 2y-15=0 shade the region bounded by these two lines and the x axis find the area of the shaded region.?
Most Upvoted Answer
Draw the graph for the following equation 5x-3y 10=0 and 5x 2y-15=0 sh...
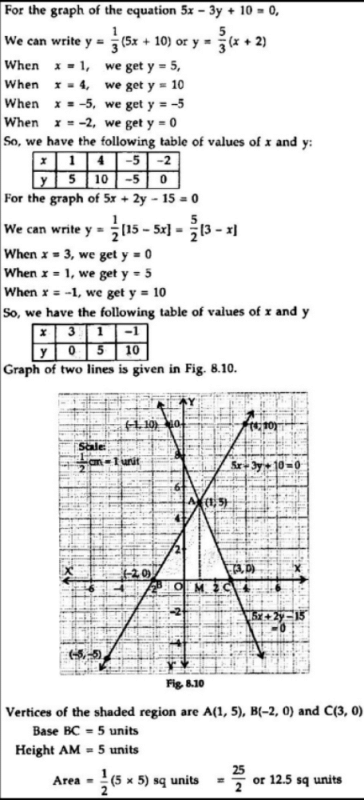
Community Answer
Draw the graph for the following equation 5x-3y 10=0 and 5x 2y-15=0 sh...
Graphing the Equations:
To graph the equations 5x - 3y + 10 = 0 and 5x + 2y - 15 = 0, we need to rearrange them in slope-intercept form, which is y = mx + b, where m is the slope and b is the y-intercept.
For the equation 5x - 3y + 10 = 0:
-3y = -5x - 10
y = (5/3)x + (10/3)
For the equation 5x + 2y - 15 = 0:
2y = -5x + 15
y = (-5/2)x + (15/2)
Now, we can graph these two lines on a coordinate plane.
Finding the Intersection Point:
To find the region bounded by these two lines and the x-axis, we need to find the intersection point of the two lines.
Setting the equations equal to each other:
(5/3)x + (10/3) = (-5/2)x + (15/2)
Multiplying both sides by 6 to eliminate fractions:
10x + 20 = -15x + 45
Adding 15x to both sides and subtracting 20 from both sides:
25x = 25
Dividing both sides by 25:
x = 1
Substituting the value of x into either of the equations, we find:
y = (5/3)(1) + (10/3)
y = 5/3 + 10/3
y = 15/3
y = 5
So the intersection point of the two lines is (1, 5).
Graphing the Shaded Region:
Now we can graph the lines and shade the region bounded by them and the x-axis.
Plotting the points (0, 10/3), (1, 5), and (3, 0), we can draw a line passing through these points for the equation 5x - 3y + 10 = 0. Similarly, plotting the points (0, 15/2), (1, 5), and (3, 0), we can draw a line for the equation 5x + 2y - 15 = 0.
The shaded region will be the area below the first line and above the second line, bounded by the x-axis.
Calculating the Area:
To find the area of the shaded region, we need to calculate the area of the trapezoid formed by the two lines and the x-axis.
The formula for the area of a trapezoid is A = (1/2)(b1 + b2)h, where b1 and b2 are the lengths of the two parallel bases and h is the height.
In this case, the length of the first base (b1) is 3 units (the horizontal distance between the points (0, 10/3) and (3, 0)), the length of the second base (b2) is also 3 units (the horizontal distance between the points (0, 15/2) and (3, 0)), and the height (h) is 5 units (the vertical distance between the points (0,
To graph the equations 5x - 3y + 10 = 0 and 5x + 2y - 15 = 0, we need to rearrange them in slope-intercept form, which is y = mx + b, where m is the slope and b is the y-intercept.
For the equation 5x - 3y + 10 = 0:
-3y = -5x - 10
y = (5/3)x + (10/3)
For the equation 5x + 2y - 15 = 0:
2y = -5x + 15
y = (-5/2)x + (15/2)
Now, we can graph these two lines on a coordinate plane.
Finding the Intersection Point:
To find the region bounded by these two lines and the x-axis, we need to find the intersection point of the two lines.
Setting the equations equal to each other:
(5/3)x + (10/3) = (-5/2)x + (15/2)
Multiplying both sides by 6 to eliminate fractions:
10x + 20 = -15x + 45
Adding 15x to both sides and subtracting 20 from both sides:
25x = 25
Dividing both sides by 25:
x = 1
Substituting the value of x into either of the equations, we find:
y = (5/3)(1) + (10/3)
y = 5/3 + 10/3
y = 15/3
y = 5
So the intersection point of the two lines is (1, 5).
Graphing the Shaded Region:
Now we can graph the lines and shade the region bounded by them and the x-axis.
Plotting the points (0, 10/3), (1, 5), and (3, 0), we can draw a line passing through these points for the equation 5x - 3y + 10 = 0. Similarly, plotting the points (0, 15/2), (1, 5), and (3, 0), we can draw a line for the equation 5x + 2y - 15 = 0.
The shaded region will be the area below the first line and above the second line, bounded by the x-axis.
Calculating the Area:
To find the area of the shaded region, we need to calculate the area of the trapezoid formed by the two lines and the x-axis.
The formula for the area of a trapezoid is A = (1/2)(b1 + b2)h, where b1 and b2 are the lengths of the two parallel bases and h is the height.
In this case, the length of the first base (b1) is 3 units (the horizontal distance between the points (0, 10/3) and (3, 0)), the length of the second base (b2) is also 3 units (the horizontal distance between the points (0, 15/2) and (3, 0)), and the height (h) is 5 units (the vertical distance between the points (0,
Attention Class 9 Students!
To make sure you are not studying endlessly, EduRev has designed Class 9 study material, with Structured Courses, Videos, & Test Series. Plus get personalized analysis, doubt solving and improvement plans to achieve a great score in Class 9.

|
Explore Courses for Class 9 exam
|

|
Similar Class 9 Doubts
Draw the graph for the following equation 5x-3y 10=0 and 5x 2y-15=0 shade the region bounded by these two lines and the x axis find the area of the shaded region.?
Question Description
Draw the graph for the following equation 5x-3y 10=0 and 5x 2y-15=0 shade the region bounded by these two lines and the x axis find the area of the shaded region.? for Class 9 2024 is part of Class 9 preparation. The Question and answers have been prepared according to the Class 9 exam syllabus. Information about Draw the graph for the following equation 5x-3y 10=0 and 5x 2y-15=0 shade the region bounded by these two lines and the x axis find the area of the shaded region.? covers all topics & solutions for Class 9 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Draw the graph for the following equation 5x-3y 10=0 and 5x 2y-15=0 shade the region bounded by these two lines and the x axis find the area of the shaded region.?.
Draw the graph for the following equation 5x-3y 10=0 and 5x 2y-15=0 shade the region bounded by these two lines and the x axis find the area of the shaded region.? for Class 9 2024 is part of Class 9 preparation. The Question and answers have been prepared according to the Class 9 exam syllabus. Information about Draw the graph for the following equation 5x-3y 10=0 and 5x 2y-15=0 shade the region bounded by these two lines and the x axis find the area of the shaded region.? covers all topics & solutions for Class 9 2024 Exam. Find important definitions, questions, meanings, examples, exercises and tests below for Draw the graph for the following equation 5x-3y 10=0 and 5x 2y-15=0 shade the region bounded by these two lines and the x axis find the area of the shaded region.?.
Solutions for Draw the graph for the following equation 5x-3y 10=0 and 5x 2y-15=0 shade the region bounded by these two lines and the x axis find the area of the shaded region.? in English & in Hindi are available as part of our courses for Class 9.
Download more important topics, notes, lectures and mock test series for Class 9 Exam by signing up for free.
Here you can find the meaning of Draw the graph for the following equation 5x-3y 10=0 and 5x 2y-15=0 shade the region bounded by these two lines and the x axis find the area of the shaded region.? defined & explained in the simplest way possible. Besides giving the explanation of
Draw the graph for the following equation 5x-3y 10=0 and 5x 2y-15=0 shade the region bounded by these two lines and the x axis find the area of the shaded region.?, a detailed solution for Draw the graph for the following equation 5x-3y 10=0 and 5x 2y-15=0 shade the region bounded by these two lines and the x axis find the area of the shaded region.? has been provided alongside types of Draw the graph for the following equation 5x-3y 10=0 and 5x 2y-15=0 shade the region bounded by these two lines and the x axis find the area of the shaded region.? theory, EduRev gives you an
ample number of questions to practice Draw the graph for the following equation 5x-3y 10=0 and 5x 2y-15=0 shade the region bounded by these two lines and the x axis find the area of the shaded region.? tests, examples and also practice Class 9 tests.

|
Explore Courses for Class 9 exam
|

|
Suggested Free Tests
Signup for Free!
Signup to see your scores go up within 7 days! Learn & Practice with 1000+ FREE Notes, Videos & Tests.

























