Class 10 Maths Chapter 12 Case Based Questions - Surface Area and Volumes
Case Study - 1
On a Sunday, your Parents took you to a fair. You could see lot of toys displayed, and you wanted them to buy a RUBIK’s cube and strawberry ice-cream for you.
Observe the figures and answer the questions: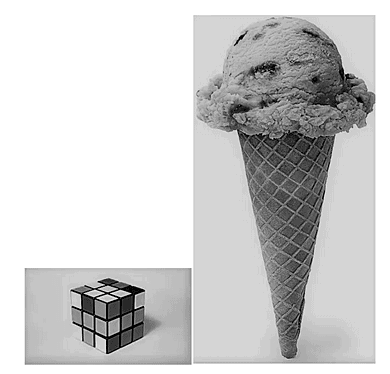 Q1: The total surface area of cone with hemispherical ice cream is
Q1: The total surface area of cone with hemispherical ice cream is
(a) 858 cm2
(b) 885 cm2
(c) 588 cm2
(d) 855 cm2
Ans: (a)
Explanation: The total surface area of a cone with hemispherical ice cream is given by the formula: TSA = CSA of the cone + CSA of the hemisphere. The Curved Surface Area (CSA) of a cone is given by the formula: πrl, where r is the radius and l is the slant height. The CSA of a hemisphere is 2πr2. Therefore, the TSA is πr(l+2r). Assuming the radius to be 7cm, and the slant height to be 25cm (as given in Q4), the TSA = π*7*(25 + 2*7) = 22/7*7*(25 + 14) = 858cm2.
Q2: What is the curved surface area of hemisphere (ice cream) if the base radius is 7cm?
(a) 309 cm2
(b) 308 cm2
(c) 803 cm2
(d) 903 cm2
Ans: (b)
Explanation: The curved surface area of a hemisphere is given by the formula: CSA = 2πr2. Here, the radius is given as 7cm. Therefore, the CSA = 2*22/7*7*7 = 308cm2.
Q3: The length of the diagonal if each edge measures 6cm is
(a) 3√ 3
(b) 3√6
(c) √12
(d) 6√ 3
Ans: (d)
Explanation: The length of the diagonal of a cube is given by the formula: √3*a, where a is the length of an edge. Given that each edge measures 6cm, the diagonal = √3*6 = 6√3.
Q4: Slant height of a cone if the radius is 7cm and the height is 24 cm
(a) 26cm
(b) 25 cm
(c) 52 cm
(d) 62cm
Ans: (b)
Explanation: The slant height of a cone can be found using the Pythagorean theorem: l = √(r2 + h2), where r is the radius and h is the height. Given that the radius is 7cm and the height is 24cm, the slant height = √(72 + 242) = 25cm.
Q5: Volume of the solid figure if the length of the edge is 7cm is
(a) 256 cm3
(b) 196 cm3
(c) 343 cm3
(d) 434 cm3
Ans: (c)
Explanation: The volume of a cube is given by the formula: V = a3, where a is the length of an edge. Given that each edge is 7cm, the volume = 73 = 343cm3.
Case Study - 2
Adventure camps are the perfect place for the children to practice decision making for themselves without parents and teachers guiding their every move. Some students of a school reached for adventure at Sakleshpur. At the camp, the waiters served some students with a welcome drink in a cylindrical glass and some students in a hemispherical cup whose dimensions are shown below. After that they went for a jungle trek. The jungle trek was enjoyable but tiring. As dusk fell, it was time to take shelter. Each group of four students was given a canvas of area 551m2. Each group had to make a conical tent to accommodate all the four students. Assuming that all the stitching and wasting incurred while cutting, would amount to 1m2, the students put the tents. The radius of the tent is 7m.

Q1: How much space on the ground is occupied by each student in the conical tent
(a) 54m2
(b) 38.5m2
(c) 86 m2
(d) 24 m2
Ans: (b)
Explanation: The area of the ground occupied by the conical tent is calculated using the formula for the area of a circle, πr².
Given the radius of the tent (r) is 7m, the area of the ground occupied by the tent is π * 7² = 154m².
Since each tent accommodates 4 students, the space on the ground occupied by each student is 154m² / 4 = 38.5m². Hence, the correct answer is option (b) 38.5m².
Q2: Which container had more juice and by how much?
(a) Hemispherical cup, 195 cm3
(b) Cylindrical glass, 207 cm3
(c) Hemispherical cup, 280.85 cm3
d) Cylindrical glass, 314.42 cm3
Ans: (d)
Explanation: The volume of the cylindrical glass is generally calculated by the formula πr²h and the volume of the hemispherical cup is calculated by the formula 2/3*πr³. By comparing these two volumes, we can determine which container had more juice.
Q3: The volume of cylindrical cup is
(a) 295.75 cm3
(b) 7415.5 cm3
(c) 384.88 cm3
(d) 404.25 cm3
Ans: (d)
Explanation: The volume of the cylindrical cup is calculated by the formula πr²h.
Q4: The volume of hemispherical cup is
(a) 179.67 cm3
(b) 89.83 cm3
(c) 172.25 cm3
(d) 210.60 cm3
Ans: (b)
Explanation: The volume of the hemispherical cup is calculated by the formula 2/3*πr³.
Q5: The height of the conical tent prepared to accommodate four students is
(a) 18m
(b) 10m
(c) 24m
(d) 14m
Ans: (b)
Explanation: The height of the conical tent can be calculated if we have the volume and the radius of the base of the tent, using the formula for the volume of a cone: V=1/3*πr²h. By rearranging the formula, we can solve for h: h=3V/πr².
|
29 videos|254 docs|56 tests
|
















