Class 10 Maths Chapter 1 Previous Year Questions - Real Numbers
| Table of contents |

|
| Previous Year Questions 2024 |

|
| Previous Year Questions 2023 |

|
| Previous Year Questions 2022 |

|
| Previous Year Questions 2021 |

|
| Previous Year Questions 2020 |

|
| Previous Year Questions 2019 |

|
Previous Year Questions 2024
Q1: The smallest irrational number by which √20 should be multipled so as to get a rational number, is: (2024)(a) √20
(b) √2
(c) 5
(d) √5
 View Answer
View AnswerAns: (d)
(a) 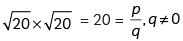
But  is not the smallest among all options.
is not the smallest among all options.
(b) 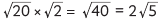 is irrational
is irrational
(c) 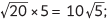 is irrational
is irrational
(d) 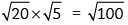
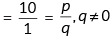
Hence, option (d) is correct.
Q2: The LCM of two prime numbers p and q (p > q) is 221. Then the value of 3p – q is: (2024)
(a) 4
(b) 28
(c) 38
(d) 48
 View Answer
View AnswerAns: (c)
The numbers p and q are prime numbers,
∴ HCF (p, q) = 1
Here, LCM(p, q) = 221
∴ As, p > q
p = 17, q = 13
(As p × q = 221)
Now, 3p – q = 3 × 17 – 13
= 51 – 13
= 38
Q3: A pair of irrational numbers whose product is a rational number is (2024)
(a) (√16, √4)
(b) (√5, √2)
(c) (√3, √27)
(d) (√36, √2)
 View Answer
View AnswerAns:(c)
Here √3 and √27 both are irrational numbers.
The product of 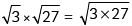
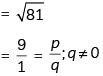
∴ 9 is a rational number.
Q4: Given HCF (2520, 6600) = 40, LCM (2520, 6600) = 252 × k, then the value of k is: (2024)
(a) 1650
(b) 1600
(c) 165
(d) 1625
 View Answer
View AnswerAns:(a)
HCF(2520, 6600) = 40
LCM(2520, 6600) = 252 × k
∴ HCF × LCM = Ist No. × IInd No.
∴ 40 × 252 × k = 2520 × 6600
⇒ 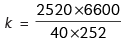
⇒ k = 1650
Q5: Teaching Mathematics through activities is a powerful approach that enhances students' understanding and engagement. Keeping this in mind, Ms. Mukta planned a prime number game for class 5 students. She announced the number 2 in her class and asked the first student to multiply it by a prime number and then pass it to the second student. The second student also multiplied it by a prime number and passed it to the third student. In this way by multiplying by a prime number, the last student got 173250.
Now, Mukta asked some questions as given below to the students: (2024)
(A) What is the least prime number used by students?
(B) How many students are in the class?
OR
What is the highest prime number used by students?
(C) Which prime number has been used maximum times?
 View Answer
View AnswerAns:
(A)
So least prime no. used by students = 3(because 2 is announced by the teacher, so the least number used by the students is 3)
(B)As the last student got 173250 = 2 × 3 × 3 × 5 × 5 × 5 × 7 × 11
there are 7 factors other than 2, which is announced by teacher. So, Number of student = 7
OR
Highest prime number used by student = 11
(C)Prime number 5 is used maximum times i.e., 3 times.
Previous Year Questions 2023
Q6: The ratio of HCF to LCM of the least composite number and the least prime number is (2023)(a) 1 : 2
(b) 2 : 1
(c) 1 : 1
(d) 1 : 3
 View Answer
View AnswerSol:Least composite number = 4
Least prime number = 2
∴ HCF = 2, LCM = 4
∴ Required ratio = HCF / LCM = 2/4
i.e. 1 : 2
Q7: Find the least number which when divided by 12, 16, and 24 leaves the remainder 7 in each case. (2023)
 View Answer
View AnswerAns:55
Given, least number which when divided by 12, 16 and 24 leaves remainder 7 in each case
∴ Least number = LCM(12, 16, 24) + 7
= 48 + 7
= 55
Q8: Two numbers are in the ratio 2 : 3 and their LCM is 180. What is the HCF of these numbers? (2023)
 View Answer
View AnswerAns:30
Let the two numbers be 2x and 3x
LCM of 2x and 3x = 6x, HCF(2x, 3x) = x
Now, 6x = 180
⇒ x = 180/6
x = 30
Q9: Prove that √3 is an irrational number. (2023)
 View Answer
View AnswerAns: Let us assume that √3 is a rational number.
Then √3 = a/b; where a and b ( ≠ 0) are co-prime positive integers.
Squaring on both sides, we get
3 = a2/b2
⇒ a2 = 3b2
⇒ 3 divides a2
⇒ 3 divides a _________(i)
= a = 3c, where c is an integer
Again, squaring on both sides, we get
a2 = 9c2
⇒3b2 = 9c2
⇒b2 = 3c2
⇒ 3 divides b2
⇒ 3 divides b _________(ii)
From (i) and (ii), we get 3 divides both a and b.
⇒ a and b are not co- prime integers.
This contradicts the fact that a and b are co-primes.
Hence, √3 is an irrational number.
Previous Year Questions 2022
Q10: Two positive numbers have their HCF as 12 and their product as 6336. The number of pairs possible for the numbers is (2022)(a) 2
(b) 3
(c) 4
(d) 1
 View Answer
View AnswerAns:(a)
Sol:Given, HCF = 12
Let two numbers be 12a and 12b
So. 12a x 12b = 6336
⇒ ab = 44
We can write 44 as product of two numbers in these ways:
ab = 1 x 44 = 2 x 22 = 4x 11
Here, we will take a = 1 and b = 44 ; a = 4 and b = 11.
We do not take ab = 2 x 22 because 2 and 22 are not co-prime to each other.
For a = 1 and b = 44, 1st no. = 12a = 12, 2nd no. = 12b = 528
For a = 4 and b = 11, 1st no. = 12a = 48, 2nd no. = 12b = 132
Hence, we get two pairs of numbers, (12, 528) and (48, 132).
Q11: If 'n' is any natural number, then (12)n cannot end with the digit (2022)
(a) 2
(b) 4
(c) 8
(d) 0
 View Answer
View AnswerAns: (d)
Sol: for n = 1, 2, 3, 4...
(12)n cannot end with 0.
Q12: The number 385 can be expressed as the product of prime factors as (2022)
(a) 5 x 11 x 13
(b) 5 x 7 x 11
(c) 5 x 7 x 13
(d) 5 x 11 x 17
 View Answer
View AnswerAns: (b)
Sol:We have,

∴ Prime factorisation of 385 = 5 x 7 x 11
Previous Year Questions 2021
Q13: Explain why 2 x 3 x 5 + 5 and 5 x 7 x 11 + 7 x 5 are composite numbers. (2021)
 View Answer
View AnswerAns: We have, 2 x 3 x 5 + 5 and 5 x 7 x 11 + 7 x 5.
We can write these numbers as:
2 x 3 x 5 + 5 = 5(2 x 3 + 1)
= 1 x 5 x 7
and 5 x 7 x 11 + 7 x 5 = 5 x 7(11 + 1)
= 5 x 7 x 12 = 1 x 5 x 7 x 12
Since, on simplifying. we find that both the numbers have more than two factors.
So. these are composite numbers.
Previous Year Questions 2020
Q14: The HCF and the LCM of 12, 21 and 15 respectively, are (2020)(a) 3, 140
(b) 12, 420
(c) 3, 420
(d) 420, 3
 View Answer
View AnswerAns: (c)
Sol:We have,
12 = 2 x 2 x 3 = 22 x 3
21= 3 x 7
15 = 3 x 5
∴ HCF (12, 21, 15) = 3
and LCM (12, 21 ,15 ) = 22 x 3 x 5 x 7
= 420
Q15: The LCM of two numbers is 182 and their HCF is 13. If one of the numbers is 26. find the other. (2020)
 View Answer
View AnswerAns: 91
Let the other number be x
As, HCF (a, b) x LCM (a, b) = a x b
⇒ 13 x 182= 26x
⇒ x = 13 x 182 / 26
= 91
Hence, other number is 91.
Q16: Given that HCF (135, 225) = 45, find the LCM (135, 225). (CBSE 2020)
 View Answer
View AnswerAns: We know that
LCM × HCF = Product of two numbers
∴ LCM (135, 225) = Product of 135 and 225 / HCF(135, 225)
= 135 x 225 / 45
= 675
So, LCM (135, 225) = 675
Previous Year Questions 2019
Q17: If HCF (336, 54) = 6. find LCM (336, 54). (2019) View Answer
View AnswerAns: 3024
Since. HCF (a, b) x LCM (a, b) = a x b
∴ HCF (336, 54) x LCM (336, 54) = 336 x 54
⇒ 6 x LCM(336, 54) = 18144
⇒ LCM (336, 54) = 18144 / 6
= 3024
Q18: The HCF of two numbers a and b is 5 and their LCM is 200. Find the product of ab. (2019)
 View Answer
View AnswerAns: 1000
We know that HCF (a, b) x LCM (a, b)=a x b
⇒ 5 x 200 = ab
⇒ ab = 1000
Q19: 1f HCF of 65 and 117 is expressible in the form 65n - 117, then find the value of n. (2019)
 View Answer
View AnswerAns: 2
Since, HCF (65 ,117) = 13
Given HCF ( 65, 117 ) = 65n - 117
13 = 65n - 117
⇒ 65n = 13 +117
⇒ n = 2
Q20: Find the HCF of 612 and 1314 using prime factorization. (2019)
 View Answer
View AnswerAns:18
Prime factorisation of 612 and 1314 are
612 = 2 x 2 x 3 x 3 x 17
1314 = 2 x 3 x 3 x 73
∴ HCF (612, 1314) = 2 x 3 x 3
= 18
Q21: Prove that √5 is an irrational number. (2019)
 View Answer
View AnswerAns: Let us assume that √5 is a rational number.
Then √5 = a/b where a and b (≠ 0} are co-prime integers,
if Squaring on both sides, we get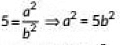
⇒ 5 divides a2
⇒ 5 divides a ----------(i)
⇒ a = 5c, where c is an integer
Again, squaring on both sides, we get
a2 = 25c2
⇒ 5b2 = 25c2
⇒ b2 = 5c2
⇒ 5 divides b2 ----------(ii)
⇒ 5 divides b
From (i) and {ii), we get 5 divides both a and b.
⇒ a and b are not co-prime integers.
Hence, our supposition is wrong.
Thus, √5 is an irrational number.
Q22: Prove that √2 is an irrational number. (2019)
 View Answer
View AnswerAns:Let us assume √2 be a rational number.
Then, √2 = p/q where p, q (q ≠ 0) are integers and co-prime. ;
On squaring both sides. we get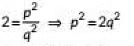 ------------(i)
------------(i)
⇒ 2 divides p2
⇒ 2 divides p -----------(ii)
So, p = 2a, where a is some integer.
Again squaring on both sides, we get
p2 = 4a2
⇒ 2q2 = 4a2 (using (i))
⇒ q2 = 2a2
⇒ 2 divides q2
⇒ 2 divides q -----------(iii)
From (ii) and (iii), we get
2 divides both p and q.
∴ p and q are not co-prime integers.
Hence, our assumption is wrong.
Thus √2 is an irrational number.
Q23: Prove that 2 + 5√3 is an irrational number given that √3 is an irrational number. (2019)
 View Answer
View AnswerAns:Suppose 2 + 5√3 is a rational number.
We can find two integers a, b (b ≠ 0) such that
2 + 5√3 = a/b, where a and b are co -prime integers.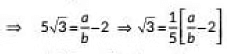
⇒ √3 is a rational number.
[ ∵ a, b are integers, so 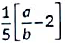 is a rational number]
is a rational number]
But this contradicts the fact that √3 is an irrational number.
Hence, our assumption is wrong.
Thus, 2 + 5√3 is an irrational number.
Q24: Write the smallest number which is divisible by both 306 and 657. (CBSE 2019)
 View Answer
View AnswerAns:Given numbers are 306 and 657.
The smallest number divisible by 306 and 657 = LCM(306, 657)
Prime factors of 306 = 2 × 3 × 3 × 17
Prime factors of 657 = 3 × 3 × 73
LCM of (306, 657) = 2 × 3 × 3 × 17 × 73
= 22338
Hence, the smallest number divisible by 306 and 657 is 22,338.
|
126 videos|457 docs|75 tests
|
FAQs on Class 10 Maths Chapter 1 Previous Year Questions - Real Numbers
| 1. What are real numbers in mathematics? |  |
| 2. How can I represent real numbers on a number line? |  |
| 3. What is the difference between rational and irrational numbers? |  |
| 4. How do I simplify a fraction involving real numbers? |  |
| 5. What are some common properties of real numbers? |  |

|
Explore Courses for Class 10 exam
|

|


















