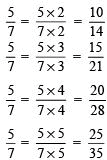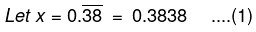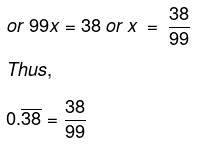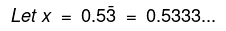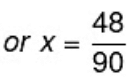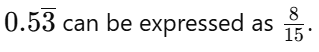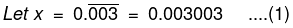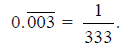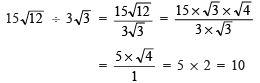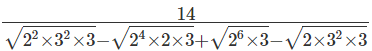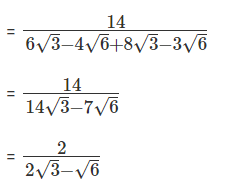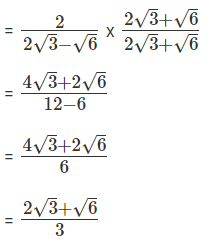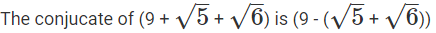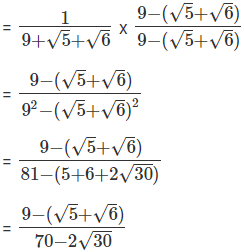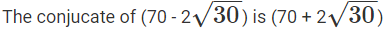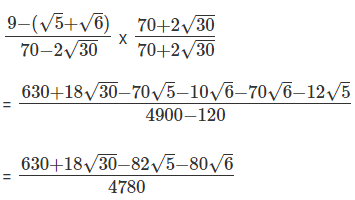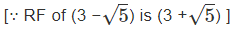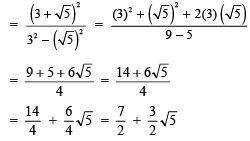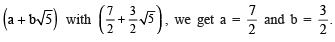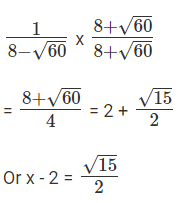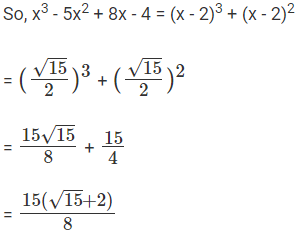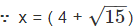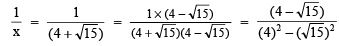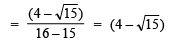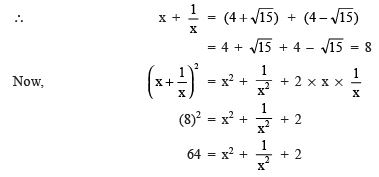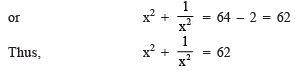Class 9 Maths Question Answers - Number System
Q1. Write four rational numbers equivalent to 5/7.
We have,
Four rational numbers equivalent to 5/7 are 10/14, 15/21, 20/28, 25/35.
Q2. Find nine rational numbers between 0.1 and 0.2.
Let x = 0.1, y = 0.2 and n = 9.
The nine rational numbers between x and y are:
(x + d), (x + 2d), (x + 3d), (x + 4d), (x + 5d), (x + 6d), (x + 7d), (x + 8d) and (x + 9d).
Nine rational numbers between 0.1 and 0.2 are:
(0.1 + 0.01); (0.1 + 0.02); (0.1 + 0.03); (0.1 + 0.04); (0.1 + 0.05); (0.1 + 0.06); (0.1 + 0.07); (0.1 + 0.08) and (0.1 + 0.09)
The nine rational numbers between 0.1 and 0.2 are 0.11, 0.12, 0.13, 0.14, 0.15, 0.16, 0.17, 0.18 and 0.19.
Q3. Express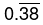 as a rational number in simplest form.
as a rational number in simplest form.
⇒ 100x = 100 x (0.3838…)
⇒ 100x = 38.3838...(2)
Subtracting (1) from (2),
We have, 100x – x = (38.3838…) – (0.3838…)
Q4. Express 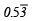 in the form of
in the form of 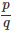 .
.
∴ 10x = 10 x (0.5353…)
or 10x = 5.333... ...(1)
Also, 100x = 53.333... ...(2)
Subtracting (1) from (2),
⇒ 100x – 10x = (53.333…) – (5.333…)
⇒ 90x = 48
Q5. Express 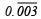 is the form of p/q in the simplest form.
is the form of p/q in the simplest form.
∴ 1000x = 1000 x (0.003003…)
or 1000x = 3.003003... …(2)
Subtracting (1) from (2),
We have 1000x – x = (3.003003…) – (0.003003…)
⇒ 999x = 3
⇒ x = 3/999 = 1/333
Thus,
Q6. Find the sum of (3√3 +7√2) and (√3 - 5√2)
We have (3√3 +7√2) + (√3 - 5√2)
⇒ √3 3+7√2 + √3 - 5√2
⇒ (3√3+√3) + 7√2 - 5√2)
⇒ √3(3+1) + √2(7-2)
⇒ √3(4) + √2(5) = (4√3 + 2√2)
Q7. Divide 15 √12 by 3√3.
Q8. Rationalize the denominator of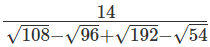
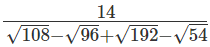
⇒(Prime factorize the numbers under the root in the denominator)
⇒
Q9. Rationalize the denominator of 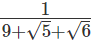
Q10. If ‘a’ and ‘b’ are rational numbers and 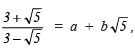 find the values of ‘a’ and ‘b’.
find the values of ‘a’ and ‘b’.
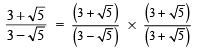
Comparing
Q11. If 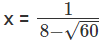 , what is the value of x3 - 5x2 + 8x - 4?
, what is the value of x3 - 5x2 + 8x - 4?
(x - 2)3 = x3 - 6x2 + 12x - 8 ...(1)
(x - 2)2 = x2 - 4x + 4 ... (2)
(1) + (2) = x3 - 5x2 + 8x - 4
Q12. Find the value of 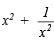 when
when 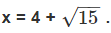
∵
Q13. Rationalise the denominator of 1/[7+3√3].
1/(7 + 3√3)
By rationalizing the denominator,
= [1/(7 + 3√3)] [(7 – 3√3)/(7 – 3√3)]
= (7 – 3√3)/[(7)2 – (3√3)2]
= (7 – 3√3)/(49 – 27)
= (7 – 3√3)/22
|
40 videos|471 docs|57 tests
|
FAQs on Class 9 Maths Question Answers - Number System
| 1. What are the different types of number systems? |  |
| 2. How do you convert a decimal number to a binary number? |  |
| 3. What is the significance of the base in number systems? |  |
| 4. How do you convert a binary number to a decimal number? |  |
| 5. Why is the hexadecimal system commonly used in computer programming? |  |