Co­ordinate Geometry Exercise 14.1 (Part-11) | Extra Documents, Videos & Tests for Class 10 PDF Download

Question 7: Prove that the lines joining the middle points of the opposite sides of a quadrilateral and the join of the middle points of its diagonals meet in a point and bisect one another.

Answer : 

Let us consider a Cartesian plane having a parallelogram OABC in which O is the origin.

We have to prove that middle point of the opposite sides of a quadrilateral and the join of the mid-points of its diagonals meet in a point and bisect each other.

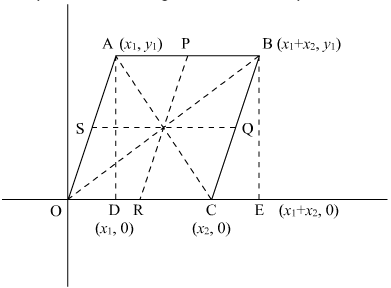
Let the co-ordinate of A be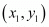. So the coordinates of other vertices of the quadrilateral are- O (0, 0); B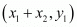; C

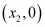
Let P, Q, R and S be the mid-points of the sides AB, BC, CD, DA respectively.

In general to find the mid-point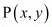 of two points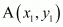and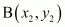 we use section formula as,

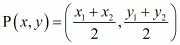
So co-ordinate of point P,

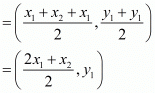
Similarly co-ordinate of point Q,

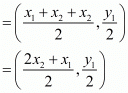
Similarly co-ordinate of point R,

Similarly co-ordinate of point S,

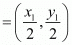
Let us find the co-ordinates of mid-point of PR as,

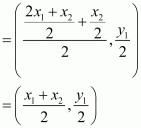
Similarly co-ordinates of mid-point of QS as,

Now the mid-point of diagonal AC,

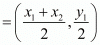
Similarly the mid−point of diagonal OA,

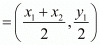
Hence the mid-points of PR, QS, AC and OA coincide.

Thus, middle point of the opposite sides of a quadrilateral and the join of the mid-points of its diagonals meet in a point and bisect each other.

Question 8: If G be the centroid of a triangle ABC and P be any other point in the plane, prove that PA² + PB² + PC² = GA² + GB² + GC² + 3GP². 

Answer : 

Letbe any triangle whose coordinates are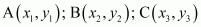. Let P be the origin and G be the centroid of the triangle.

We have to prove that,

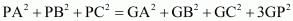 …… (1)

We know that the co-ordinates of the centroid G of a triangle whose vertices are 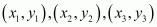 is−

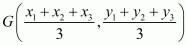
In general, the distance between A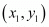 and B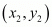 is given by,

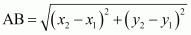
So,

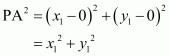
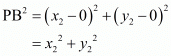
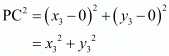
Now,

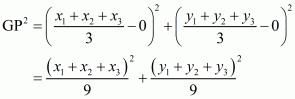
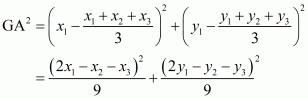
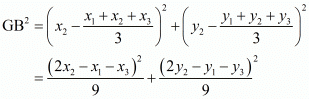
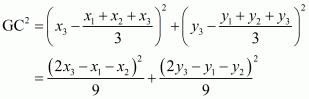
So we get the value of left hand side of equation (1) as,

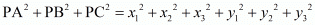
Similarly we get the value of right hand side of equation (1) as,

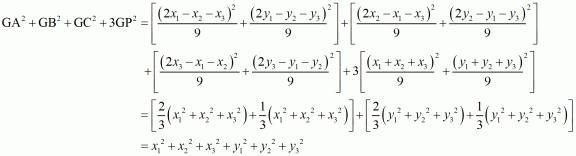
Hence,

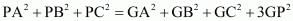
Question 9: If G be the centroid of a triangle ABC, prove that:
AB² + BC² + CA² = 3 (GA² + GB² + GC²)

Answer : 

Let A(x1,y1); B(x2,y2); C(x3,y3) be the coordinates of the vertices of ΔABC.Let us assume that centroid of the ΔABC is at the origin G.So, the coordinates of G are G(0,0).Now,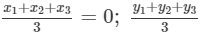=0so, x1+x2+x3=0   .......(1)y1+y2+y3=0        ........(2)Squaring (1) and (2), we getx12+x22+x32+2x1x2+2x2x3+2x3x1=0     .......(3)y12+y22+y32+2y1y2+2y2y3+2y3y1=0      ......(4)LHS=AB2+BC2+CA2

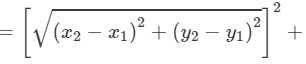
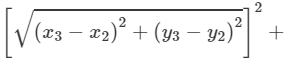
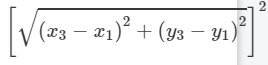
=(x2−x1)2+(y2−y1)2+(x3−x2)2+(y3−y2)2+(x3−x1)2+(y3−y1)2=x12+x22−2x1x2+y12+y22−2y1y2+x22+x32−2x2x3+y22+y32−2y2y3+x12+x32−2x1x3+y12+y32−2y1y3=2(x12+x22+x32)+2(y12+y22+y32)−(2x1x2+2x2x3+2x3x1)−(2y1y2+2y2y3+2y3y1)=2(x12+x22+x32)+2(y12+y22+y32)+(x12+x22+x32)+(y12+y22+y32)=3(x12+x22+x32+y12+y22+y32)

RHS=3(GA²+GB²+GC²) 

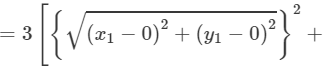
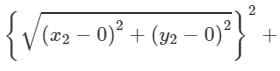
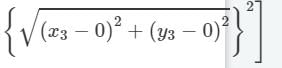
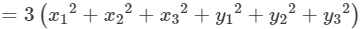
Hence, AB²+BC²+CA²=3(GA²+GB²+GC²) 

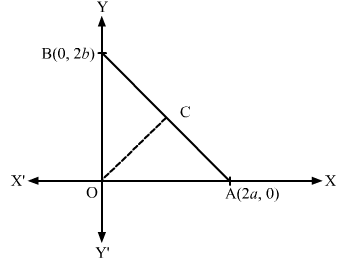
Question 10: In Fig. 14.36, a right triangle BOA is given C is the mid-point of the hypotenuse AB. Show that it is equidistant from the vertices O, A  and B.

Answer : 

We have a right angled triangle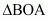, right angled at O. Co-ordinates are B (0,2b); A (2a, 0) and C (0, 0).

We have to prove that mid-point C of hypotenuse AB is equidistant from the vertices.

In general to find the mid-point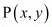 of two points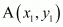and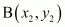 we use section formula as,

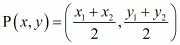
So co-ordinates of C is,

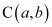
In general, the distance between A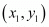 and B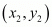 is given by,

So,

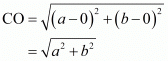
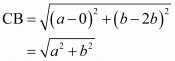
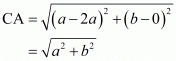
Hence, mid−point C of hypotenuse AB is equidistant from the vertices.