Important Formulas: Arithmetic Progressions | Mathematics (Maths) Class 10 PDF Download

The chapter on "Arithmetic Progression" is crucial for understanding the patterns and principles behind sequences of numbers, forming the basis for solving a wide range of mathematical problems in various fields. 

This document provides Class 10 Mathematics Formulas for Arithmetic Progressions that can help you succeed in board exams and in future competitive exams.

Important Definitions and Terms

1. Arithmetic Progressions

An arithmetic progression, often abbreviated as AP, is a sequence of numbers in which the difference between any two consecutive terms is constant. This constant difference is known as the "common difference." Arithmetic progressions are an essential concept in mathematics and have various applications in real-world scenarios.

In Arithmetic Progression (AP), there are some key terms that we need to get familiar with so as to understand formulas better. 

• First term (a)
• Common difference (d)
• nth Term (a_n)
• Sum of the first n terms (S_n)

2. First Term of an A.P.

The AP can also be written in terms of common differences, as follows ;
a, a + d, a + 2d, a + 3d, a + 4d, ………., a + (n – 1) d
where “a” is the first term of the progression.

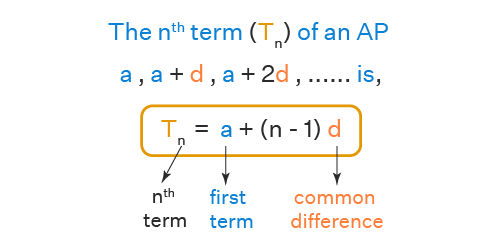
Example 1:

Consider an arithmetic progression with a common difference (d) of 3. If we have the following terms in the AP:

a, a + 3, a + 6, a + 9, a + 12, ...

Sol: In this case, the first term (a) is the initial value of the progression. For example, if a = 2, the first term of the AP would be 2.

Example 2:

Let's take another example of an arithmetic progression with a common difference (d) of -2. The terms of the AP are given by:

a, a - 2, a - 4, a - 6, a - 8, ...

Sol: Again, the first term (a) represents the starting value of the progression. For instance, if a = 10, the first term of the AP would be 10.

In both examples, "a" denotes the first term of the arithmetic progression.

3. Common Difference

In an arithmetic progression, the terms are identified by their position (first term, second term, etc.), the
common difference between consecutive terms, and the nth term. If the series is denoted by a₁, a₂, a₃, ……………., a_n, then the common difference "d" can be determined by:
d = a₂ – a₁ = a₃ – a₂ = ……. = a_n – a_n – 1

Here are two examples of the common difference in an arithmetic progression (AP):

Example 1: Consider the following arithmetic progression: 3, 7, 11, 15, 19, ...

Sol: To find the common difference (d), we can take the difference between any two consecutive terms. Let's calculate:

d = 7 - 3 = 4

In this case, the common difference is 4. Each term in the progression is obtained by adding 4 to the previous term.

Example 2: Let's take another arithmetic progression: -2, -5, -8, -11, -14, ...

Sol: To determine the common difference (d), we can subtract any two consecutive terms:

d = -5 - (-2) = -3

In this example, the common difference is -3. Each term in the progression is obtained by subtracting 3 from the previous term.

In both cases, the common difference (d) represents the constant value by which consecutive terms in the arithmetic progression differ from each other.

Important Formulas and related Examples

When it comes to Arithmetic Progression, there are two essential formulas that we need to know like the back of our hand:

• The nth term of AP
• Sum of the first n terms

Here we will understand both formulas along with some examples.

1. nth Term of an AP

The formula for finding the nth term of an AP is:

Example 1: Find the 12th term of an arithmetic progression with a first term of 8 and a common
difference of 5.

Sol:
a₁₂ = a₁ + (n-1)d
a₁₂ = 8 + (12-1)*5
a₁₂ = 8 + 55
a₁₂ = 63

Example 2: If the 5th term of an arithmetic progression is 17 and the common difference is -3, find the first term.

Sol:
a₅ = a₁ + (n-1)d
17 = a₁ + (5-1)*(-3)
17 = a₁ - 12
a₁ = 29

2. Sum of n Terms of AP

The formula for finding the sum of the first n terms of an arithmetic progression can be determined if you know the first term, common difference, and the number of terms. This formula helps in calculating the total sum of an AP series.

Example 1: Find the sum of the first 20 terms of an arithmetic progression where the first term is 3 and the common difference is 4.

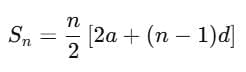Sol:
To find the sum of the first 20 terms of an AP, we can use the formula:
S_n = (n/2) x [2a + (n-1)d]
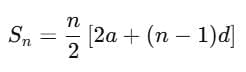
where S_n is the sum of the first n terms, a is the first term, d is the common difference, and n is the number of terms.
Substituting the given values, we get:
S₂₀ = (20/2) x [2(3) + (20-1)(4)]
S₂₀ = 10 x [6 + 76]
S₂₀ = 820
Therefore, the sum of the first 20 terms of the AP is 820.

Example 2: Find the sum of the first 20 terms of an AP where the first term is 10 and the common difference is 5.

Sol:
Here, the first term (a) = 10, common difference (d) = 5 and n (total number of terms) = 20.
Using the formula for the sum of n terms of an AP, we have:
S₂₀ = (20/2) * [2(10) + (20 - 1)(5)]
S₂₀ = 10 * [20 + 95]
S₂₀ = 10 * 115
S₂₀ = 1150

3. Sum of AP when the Last Term is Given

Formula to find the sum of AP when first and last terms are given as follows:

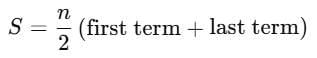Example 1: Find the sum of an arithmetic progression if the last term is 35, the first term is 5, and there are 8 terms in total.
Sol:
Using the formula 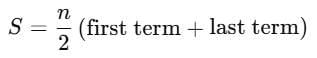S = n/2 (a + l), we have:
S = 8/2 (5 + 35)
S = 4 x 40
S = 160
Therefore, the sum of the given arithmetic progression is 160.