JEE Advanced (Fill in the Blanks): Heat & Thermodynamics | Chapter-wise Tests for JEE Main & Advanced PDF Download

Q.1. One mole of a mono-atomic ideal gas is mixed with one mole of a diatomic ideal gas. The molar specific heat of the mixture at constant volume is .......

Ans. 4 cal.

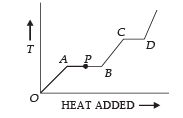
Solution. 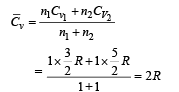Q.2. The variation of temperature of a material as heat is given to it at a constant rate is shown in the figure. The material is in solid state at the point O. The state of the material at the point P is ........

Ans. Partly solid and partly liquid

Solution.  

Q.3. During an experiment, an ideal gas is found to obey an additional law VP² =constant, The gas is initially at a temperature T, and volume V. When it expands to a volume 2V, the temperature becomes..........

Ans. √2T

Solution. PV = RT (Ideal gas equation)

Given that VP² = const ... (ii) 

From (i) and (ii)

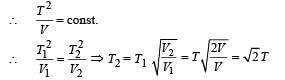
Q.4. 300 grams of water at 25° C is added to 100 grams of ice at 0°C. The final temperature of the mixture is .....°C.

Ans. 0°C

Solution. The heat required for 100 g of ice at 0° C to change into water at 0°C = mL = 100 × 80 ×4.2 = 33,600 J          ... (i) 

The heat released by 300g of water at 25°C to change its temperature to 0°C = mcΔT = 300 × 4.2 × 25 = 31,500 J  ... (ii) 

Since the energy in eq. (ii) is less than of eq. (i) therefore the final temperature will be 0°C.

Q.5. The earth receives at its surface radiation from the sun at the rate of 1400 W m^–2. The distance of the centre of the sun from the surface of the earth is 1.5 × 10¹¹ m and the radius of the sun is 7 × 10⁸ m. Treating the sun as a black body, it follows from the above data that its surface temperature is...........K.

Ans. 5803K

Solution. The energy received per second per unit area from Sun at a distance of 1.5 × 10¹¹ m is 1400 J/sm². The total energy released by Sun/per second.

= 1400 × 4π × (1.5 × 10¹¹)².

∴ The total energy released per second per unit surface area of the Sun

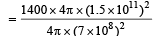
This energy E is also equal to E = σT⁴

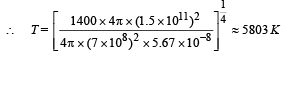
Q.6. A solid copper sphere (density r and specific heat c) of radius r at an initial temperature 200 K is suspended inside a chamber whose walls are at almost 0K. The time required for the temperature of the sphere to drop to 100K is ....................

Ans. 1.71ρrc

Solution.The energy emitted per second when the temperature of the copper sphere is T and the surrounding temperature T₀

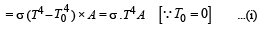
_{We know that}

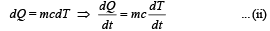
From (i) and (ii)

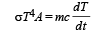
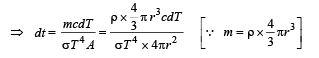
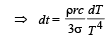
Integrating both sides

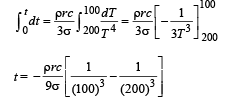
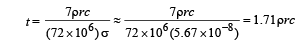
Q.7. A point source of heat of power P is placed at the centre of a spherical shell of mean radius R. The material of the shell has thermal conductivity K. If the temperature difference between the outer and inner surface of the shell in not to exceed T, the thickness of the shell should not be less than ............

Ans. 

Solution. 

KEY CONCEPT :

When the spherical shell is thin, t << R. In this case, The rate of flow of heat from the sphere to the surroundings

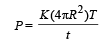
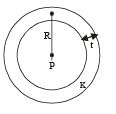
where T is the temperature difference and t is the thickness of steel then

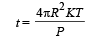
Q.8. A substance of mass M kg requires a power input of P watts to remain in the molten state at its melting point. When the power source is turned off, the sample completely solidifies in time t seconds. The latent heat of fusion of the substance is ....................

Ans. 

Solution. Since P joules per second of heat is supplied to keep the substance in molten state, it means that the substance in the molten state at its melting point releases P Joule of heat in one second.

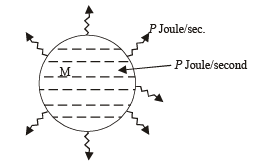
The power is turned off then the heat input becomes zero.

But heat output continues. It takes t seconds for the substance to solidify (given). Therefore total heat released in t seconds = P × t = mL_fusion

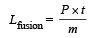
Q.9. A container of volume 1m³ is divided into two equal parts by a partition. One part has an ideal gas at 300K and the other part is vacuum. The whole system is thermally isolated from the surroundings. When the partition is removed, the gas expands to occupy the whole volume. Its temperature will now be.......

Ans. 300 K

Solution. In this expansion, no work is done because the gas expands in vacuum. Therefore ΔW = 0 

As the process is a adiabatic, Q = 0. From first law of thermodynamics, ΔU = 0 i.e. temperature remains constant.

Q.10. An ideal gas with pressure P, volume V and temperature T is expanded isothermally to a volume 2V and a final pressure P_i. If the same gas is expanded adiabatically to a volume 2V, the final pressure is P_a. The ratio of the specific heats of the gas is 1.67 . The ratio Pa/R is ...

Ans. 0.628

Solution. For isothermal expansion

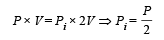
For adiabatic expansion

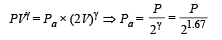
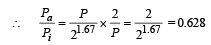
Q.11. Two metal cubes A and B of same size are arranged as shown in Figure. The extreme ends of the combination are maintained at the indicated temperatures. The arrangement is thermally insulated. The coefficients of thermal conductivity of A and B are 300 W/m °C and 200 W/m°C, respectively. After steady state is reached the temperature t of the interface will be .....

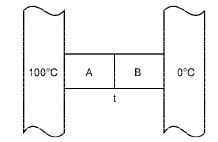
Ans. 60°C Solution.

Solution. The heat transferred through A per second

 Q₁= K₁ A (100 – t) 

The heat transferred through B per second 

Q₂= K₂ A (t – 0) 

At steady state K₁ A (100 – t) = K₂A (t – 0)

 ⇒ 300 (100 – t) = 200 (t – 0) ⇒ 300 – 3t = 2t ⇒ t = 60° C

Q.12. A ring shaped tube contains two ideal gases with equal masses and relative molar masses M₁= 32 and M₂ = 28. The gases are separated by one fixed partition and another movable stopper S which can move freely without friction inside the ring. The angle a as shown in the figure is ........... degrees.

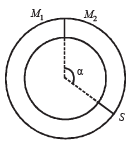
Ans. 192º

Solution. The movable stopper will adjust to a position with equal pressure on either sides. Applying ideal gas equation to the two gases, we get

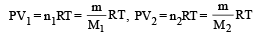
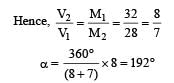
Q.13. Earth receives 1400 W/m ² of solar power. If all the solar energy falling on a lens of area 0.2 m² is focused on to a block of ice of mass 280 grams, the time taken to melt the ice will be... minutes. (Latent heat of fusion of ice = 3.3 × 10⁵J/ kg.

Ans. 5.5 min.

Solution.  Solar power received by earth = 1400 W/m² 

Solar power received by 0.2 m² area 

= (1400 W/m²) (0.2 m²) = 280 W 

Mass of ice = 280 g = 0.280 kg 

Heat required to meltice .

= (0.280) (3.3 × 105) = 9.24 × 104 J