JEE Advanced (Subjective Type Questions): Properties of Triangle - 1 | Chapter-wise Tests for JEE Main & Advanced PDF Download
Q. 1. A triangle ABC has sides AB = AC = 5 cm and BC = 6 cm Triangle A'B'C' is the reflection of the triangle ABC in a line parallel to AB placed at a distance 2 cm from AB, outside the triangle ABC. Triangle A"B"C" is the reflection of the triangle A'B'C' in a line parallel to B'C' placed at a distance of 2 cm from B'C' outside the triangle A'B'C'. Find the distance between A and A".
Ans. 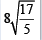
Solution.
Let L be the line parallel to side AB of ΔABC, at a distance of 2 cm from AB, in which the first reflection ΔA' B' C' is obtained.
Let L' be the second line parallel to B' C', at a distance of 2 cm from B' C', in which reflection of ΔA' B' C' is taken as ΔA"B"C".
In figure, size of ΔA"B"C" is same to the size of ΔA'B'C'.
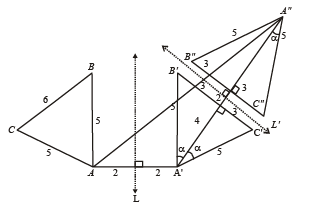
From figure AA' = 4 cm and A'A" = 12 cm. So to find AA" it suffices to know ∠AA'A", clearly
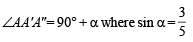
⇒ cos ∠AA'A" = cos (90° + α) = – sin α = 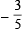 and hence
and hence
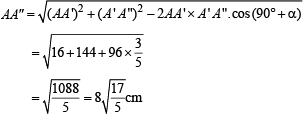
Q. 2. (a) If a circle is inscribed in a right angled triangle ABC with the right angle at B, show that the diameter of the circle is equal to AB + BC – AC.
(b) If a triangle is inscribed in a circle, then the product of any two sides of the triangle is equal to the product of the diameter and the perpendicular distance of the third side from the opposite vertex. Prove the above statement.
Solution. (a) Inradius of the circle is given by
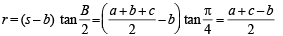
2r = a + c – b ⇒ Diameter = BC + AB – AC
(b) Given a ΔABC in which AD ⊥ BC, AE is diameter of circumcircle of ΔABC.
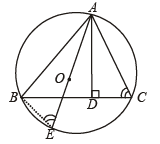
To prove :
AB × AC = AE × AD
Construction : Join BE
Proof : ABE = 90° (∠ in a semi circle)
Now in Δ's ABE and ADC
∠ABE = ∠ADC (each 90°)
∠AEB = ∠ACD (∠’s in the same segment)
∴ ΔABE ~ ΔADC (by AA similarity)
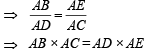 (Proved)
(Proved)
Q. 3. (a) A balloon is observed simultaneously from three points A, B and C on a straight road directly beneath it. The angular elevation at B is twice that at A and the angular elevation at C is thrice that at A. If the distance between A and B is a and the distance between B and C is b, find the height of the balloon in terms of a and b.
(b) Find the area of the smaller part of a disc of radius 10 cm, cut off by a chord AB which subtends an angle of 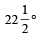 at the circumference.
at the circumference.
Ans. 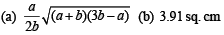
Solution. By exterior angle theorem, in the adjacent fig.
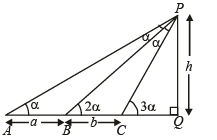
∠APB = ∠BPC = α
Also in ΔABP, ∠BAP = ∠APB = a
⇒ AB = PB = a
Applying sine law in ΔPBC, we get
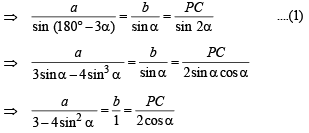
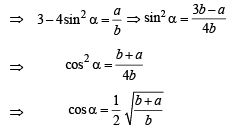
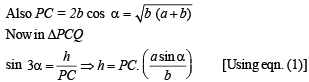
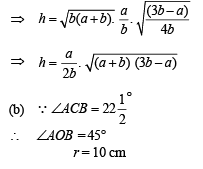
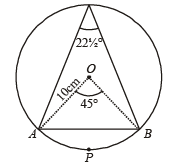
Area of the segment APB = Area of the sector AOB – area of ΔAOB
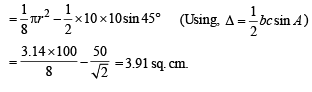
Q. 4. ABC is a triangle. D is the middle point of BC. If AD is perpendicular to AC, then prove that 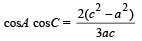
Solution.
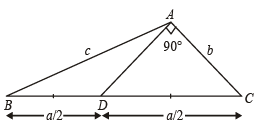
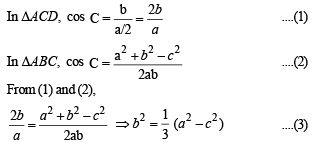
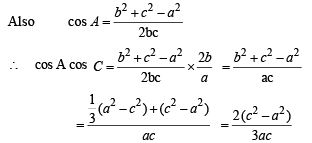
Q. 5. ABC is a triangle with AB = AC. D is any point on the side BC. E and F are points on the side AB and AC, respectively, such that DE is parallel to AC, and DF is parallel to AB.
Prove that
DF + FA + AE + ED = AB + AC
Solution. Given that AB = AC
∴ ∠1 = ∠2 ....(1)
But AB || DF (given) and BC is transversal
∴ ∠1 = ∠3 ....(2)
From equations (1) and (2)
∠2 = ∠3
⇒ DF = CF ....(3)
Similaraly we can prove DE = BE
Now, DF + FA + AE + ED = CF + FA + AE + BE
= AC + AB [using equation (3) and (4)]
Q. 6. (i) PQ is a vertical tower. P is the foot and Q is the top of the tower. A, B, C are three points in the horizontal plane through P. The angles of elevation of Q from A, B, C are equal, and each is equal to θ. The sides of the triangle ABC are a, b, c; and the area of the triangle ABC is D. Show that the height of the tower is 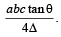
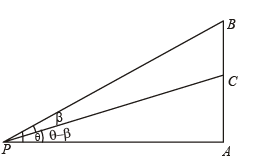
(ii) AB is a vertical pole. The end A is on the level ground. C is the middle point of AB. P is a point on the level ground. The portion CB subtends an angle β at P. If
AP = n AB, then show that 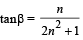
Solution. (i) Let h be the height of tower PQ.
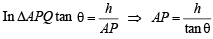
Similarly in Δ's BPQ and CPQ we obtain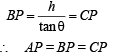
⇒ P is the circumcentre of
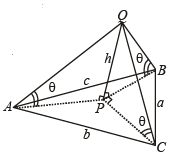
ΔABC with circum radius as 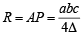
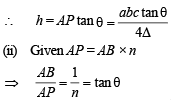
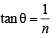
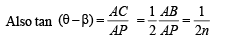
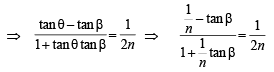
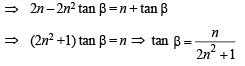
Q. 7. Let the angles A, B, C of a triangle ABC be in A.P. and let 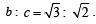 Find the angle A.
Find the angle A.
Solution. As the angles A, B, C of ΔABC are in AP
∴ Let A = x – d, B = x, C = x + d
But A + B + C = 180° (∠Sum prop. of Δ)
∴ x – d + x + x + d = 180°
⇒ 3x = 180° ⇒ x = 60° ∴ ∠B = 60°
Now by sine law in ΔABC, we have
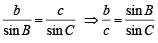
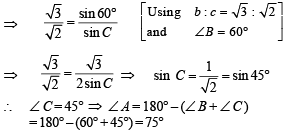
Q. 8. A vertical pole stands at a point Q on a horizontal ground. A and B are points on the ground, d meters apart. The pole subtends angles α and β at A and B respectively. AB subtends an angle γ at Q. Find the height of the pole.
Solution. Let ht. of pole PQ be h.
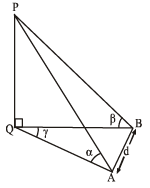
In ΔAPQ, 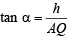
⇒ 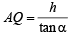 ....(1)
....(1)
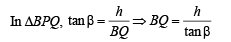 ....(2)
....(2)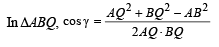
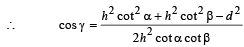
∴ – 2h2 cot α cot β cos γ + h2 cot2 α + h2 cot2 β = d2
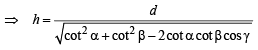
Q. 9. Four ships A, B, C and D are at sea in the following relative positions : B is on the straight line segment AC, B is due North of D and D is due west of C. The distance between B and D is 2 km. ∠BDA = 40°, ∠BCD = 25°. What is the distance between A and D? [Take sin25° = 0.423]
Solution. According to question figure is as follows
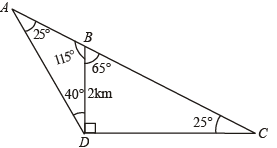
Here, ∠BDC = 90°, BD = 2 km
∠BDA = 40°, ⇒ ∠ADC = 130°,
∴ ∠DAC = 180° – (25° +130°) = 25°
From the figure, in Δ ABD, using sine law
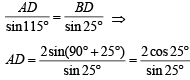
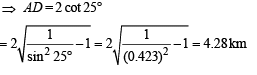
Q. 10. The ex-radii r1, r2, r3 of Δ ABC are in H.P. Show that its sides a, b, c are in A.P.
Solution. KEY CONCEPT :
Ex-radii of a DABC are 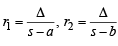
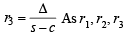 are in HP.
are in HP. 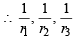 are in AP
are in AP
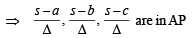
⇒ s – a, s – b, s – c are in AP
⇒ – a, – b, – c are in AP.
⇒ a, b, c are in A.P.
Q. 11. For a triangle ABC it is given that cosA + cosB + cosC= 3/2. Prove that the triangle is equilateral.
Solution. Given that CosA + cosB + cosC= 3/2. in ΔABC
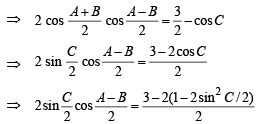
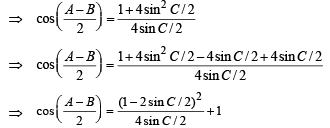
Which is possible only when
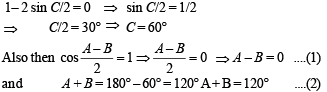
From (1) and (2) A = B = 60° Thus we get A = B = C = 60°
∴ ΔABC is an equilateral Δ.
Q. 12. With usual notation, if in a triangle ABC; 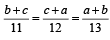 then prove that
then prove that 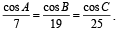
Solution. Given that, in Δ ABC,
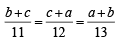
where a, b, c are the lengths of sides BC, CA and AB respectively.
Let 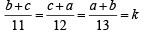
⇒ b + c = 11 k ....(1)
c + a = 12 k ....(2)
a + b = 13 k ....(3)
Adding the above three eqs. we get
2 (a + b + c) = 36 k
⇒ a + b + c = 18 k ....(4)
Solving each of (1), (2) and (3) with (4), we get
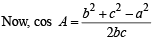
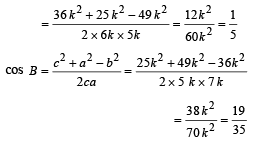
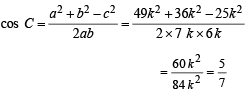
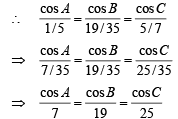
Q. 13. A ladder rests against a wall at an angle a to the horizontal. Its foot is pulled away from the wall through a distance a, so that it slides a distance b down the wall making an angle b with the horizon tal. Show that 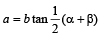
Solution. Let ℓ be the length of the ladder, then
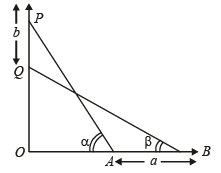
In ΔOQB, 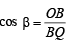
⇒ 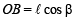 ....(1)
....(1)
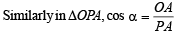
⇒ OA = ℓ cos α ....(2)
Now a = OB – OA = ℓ (cos β – cos α) ....(3)
Also from ΔOAP, OP = ℓ sin α
and in OQB ; OQ = ℓ sin β
∴ b = OP – OQ = ℓ (sin α – sin β) ....(4)
Dividing eq. (3) by (4) we get
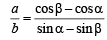
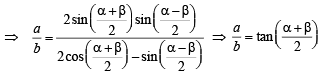
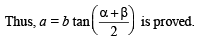
Q. 14. In a triangle ABC, t he median to th e side BC is of length 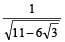 and it divides the angle A into angles 30° and 45°. Find the length of the side BC.
and it divides the angle A into angles 30° and 45°. Find the length of the side BC.
Solution. Let AD be the median in ΔABC.
Let ∠B = q then ∠C = 105°– θ
In ΔABD, using sine law, we get
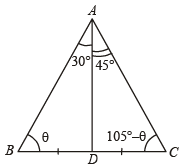

In ΔACD, using sine law, we get
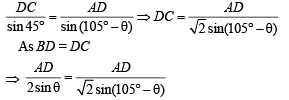
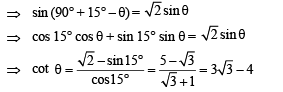
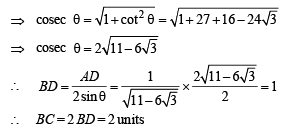
Q. 15. If in a triangle ABC, cos A cos B + sin A sin B sin C = 1, Show that a : b : c = 1 : 1 : √2
Solution. We are given that in ΔABC cos A cos B + sin A sin B sin C = 1
⇒ sin A sin B sin C = 1 – cos A cos B
⇒ 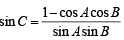
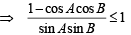 [∴sin C < 1]
[∴sin C < 1]
⇒ 1 - cos A cos B < sin A sinB
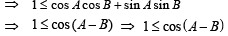
But we know cos (A – B) < 1
∴ We must have cos (A – B) = 1
⇒ A – B = 0
⇒ A = B
∴ cosA cos A + sin A sin A sin C = 1 [For A = B]
⇒ cos2 A + sin2 A sin C = 1
⇒ sin2 A sin C = 1 – cos2 A
⇒ sin2 A sin C = sin2 A ⇒ sin2 A (sin C – 1) = 0
⇒ sin A = 0 or sin C = 1
The only possibility is sin C = 1 ⇒ C = π/2
∴ A + B = π/2
But A = B ⇒ A = B = π/4
∴ By Sine law in ΔABC,
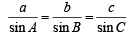
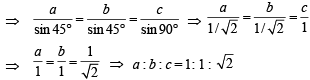
Hence proved the result.
Q. 16. A sign-post in the form of an isosceles triangle ABC is mounted on a pole of height h fixed to the ground. The base BC of the triangle is parallel to the ground. A man standing on the ground at a distance d from the sign-post finds that the top vertex A of the triangle subtends an angle β and either of the other two vertices subtends the same angle α at his feet. Find the area of the triangle.
Solution. Let ABC be the isosceles triangular sign board with BC horizontal. DE be the pole of height h. Let the man be standing at P such that PE = d
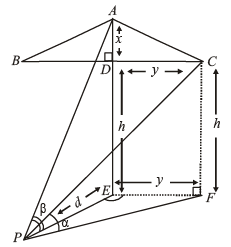
Also ATQ, ∠APE = β
∠CPF = α
Let AD = x be altitude of ΔABC.
As ΔABC is isosceles with AB = AC
∴ D is mid pt of BC.
Hence BC = 2y.
Now in ΔAPE,
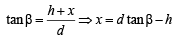 ....(1)
....(1)
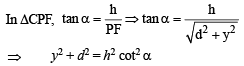
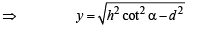 ....(2)
....(2)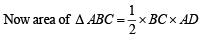
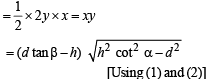
|
481 docs|964 tests
|
















