Class 10 Maths Chapter 3 Question Answers - Pair of Linear Equations in Two Variables

Q1: For what value of p, the pair of linear equations px = 2y; 2x - y + 5 = 0 has unique solution?
Sol: We have: px = 2y
⇒ p - 2y = 0
2x = y + 5
⇒ 2x - y = - 5
Here, a₁ = p, b₁ = - 2,
c₁ = 0
a₂ = 2, b₂ = - 1,
c₂ = - 5
For a unique solution,

a₁a₂ ≠ b₁b₂

Substitute the values:

p2 ≠ -2-1

p2 ≠ 2

p ≠ 4 

Q2: In a cyclic quadrilateral PQRS, ∠P = (2x + 4)°, ∠Q = (y + 3)°, ∠R = (2y + 10)° and ∠S = (4x - 5)°. Find its four angles. 
Sol: In a cyclic quadrilateral, the sum of opposite angles is 180°.
From ∠P + ∠R = 180°:
(2x + 4) + (2y + 10) = 180 → 2x + 2y = 166 (Equation 1)
From ∠Q + ∠S = 180°:
(y + 3) + (4x - 5) = 180 → 4x + y = 182 (Equation 2)
Solving the equations:
From Equation 1: y = 83 - x
Substitute into Equation 2:
4x + (83 - x) = 182 → 3x + 83 = 182 → x = 33
Find y:
y = 83 - 33 = 50
Calculate the angles:

• ∠P = 2x + 4 = 70°
• ∠Q = y + 3 = 53°
• ∠R = 2y + 10 = 110°
• ∠S = 4x - 5 = 127°

Therefore,  ∠P = 70°, ∠Q = 53°, ∠R = 110°, ∠S = 127°

Q3: Solve:
23x + 35y = 209
35x + 23y = 197
Sol: We have:
23x + 35y = 209   ...(1)
35x + 23y = 197    ...(2)
58x + 58y = 406 [Adding (1) and (2)]
⇒ x + y = 7 ...(3) [Dividing by whole equation by 58]
Subtracting (1) from (2),
We get, 
12x - 12y = - 12
⇒ x - y = 1 ...(4) [Dividing whole equation  by 12]
Adding (3) and (4),We get, 
x + y + x - y = 7 + 1
⇒ 2x = 8 ⇒ x = 4
Putting the value of x = 4 in (3) 
We get,
x + y = 7 ⇒ 4 + y = 7
⇒ y = 3
So, x = 4 and y = 3.

Q4: Solve:
3x + 5y = 70   ...(1)
7x - 3y = 60   ...(2)
Sol: To solve the equations:
3x + 5y = 70
7x - 3y = 60
Step 1: Eliminate one variable
Multiply Equation (1) by 3 and Equation (2) by 5:
(9x + 15y = 210)
(35x - 15y = 300)
Add the two equations:
(44x = 510)
x = 51044 = 25522

Step 2: Substitute x into Equation (1)
Substitute x = 25522 into 3x + 5y = 70:
3 x 25522 + 5y = 70
76522 + 5y = 70
5y = 70 - 76522
5y = 154022 - 76522
5y = 77522
y = 775110 = 15522
Therefore, x = 25522, y = 15522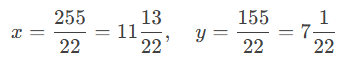

Q5: Without drawing the graphs, state whether the following pair of linear equations will represent intersecting lines, coinciding lines or parallel lines:
6x - 3y + 10 = 0
2x - y + 9 = 0
Sol: Here, the given set of equations is:
6x - 3y + 10 = 0   ...(1)
2x - y + 9 = 0    ...(2)
From (1) and (2), we have:
a₁ = 6, b₁ = - 3, c₁ = 10
a₂ = 2, b₂ = - 1, c₂ = 9

Now , a₁a₂ = 62 = 3

∴ We have: a₁a₂ = b₁b₂ ≠ c₁c₂

This condition represents parallel lines. Hence, the given pair represents parallel lines.

Q6: Check graphically whether the pair of equations 

3x - 2y + 2 = 0
32 x - y + 3 = 0 
is consistent. Also find the co-ordinates of the points where the graphs of the equations meet the y-axis.
Sol: 3x − 2y + 2 = 0 ⇒ y = 3x − 22 ... (1)

Also, 0 = y = 32 x − y + 3 = ... (2)
0 ⇒ y = 32 x + 3

Plotting the points (0, 1), (2, 4), (- 2, - 2) and (0, 3), (2, 6), (–2, 0) we get two straight lines l₁ and l₂ which are parallel.
∴ The given equations are inconsistent.
From the graph, we observe that line l₁ meets the y-axis at (0, 1) and line l₂ meets the y-axis at (0, 3).

Q7: A fraction becomes 1/3 if 2 is added to both numerator and denominator. If 3 is added to both numerator and denominator, it becomes 2/5. Find the fraction.

Sol:  Let the fraction be: xy
From the first condition: x + 2y + 2 = 13
Cross-multiplying:
3(x + 2) = y + 2
Simplify:
3x - y = -4   (1)
From the second condition: x + 3y + 3 = 25
Cross-multiplying:
5(x + 3) = 2(y + 3)
Simplify:
5x - 2y = -9   (2)
From Equation (1):
y = 3x + 4   (3)
Substitute y = 3x + 4 into Equation (2):
5x - 2(3x + 4) = -9
Simplify:
-x = -1 , x = 1
Substitute x = 1 into Equation (3):
y = 3(1) + 4 = 7
Thus, the fraction is: 17

Q8: Check graphically whether the pair of equations
3x + 5y = 15
x - y = 5
is consistent. Also, find the coordinates of the points where the graphs of equations meet the y-axis.
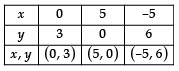
Sol: We have

3x + 5y = 15

⇒ y =  15 - 3x5

∴

And from x - y = 5

⇒ y = x - 5

Plotting the above two sets of points we get two straight lines l1 and l2 which intersect at the point (5, 0).

Thus, the given system is consistent.

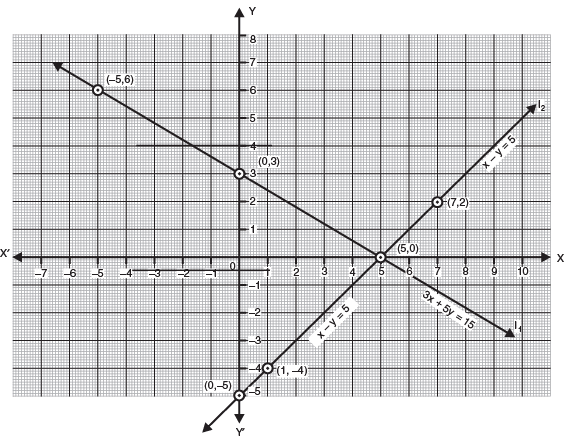
Obviously, line l₁ meets the y-axis at (0, 3) and line l₂ meets the y-axis at (0, - 5).

Q9: Places A and B are 160 km apart on the highway. One car starts from A and another from B at the same time. If the cars travel in the same direction at different speeds, they meet in 8 hours, but if they travel towards each other, they meet in 2 hours. What are the speeds of the two cars? 

Sol: Let the car-I and car-II start from A and B at x km/hr and y km/hr respectively.

Case-I: [Cars are moving in the same direction]

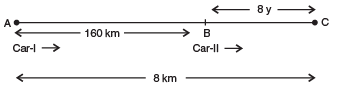
Let the two cars meet at C after 8 hours.
Distance covered:
by car-I = AC = 8x km
by car-II = BC = 8y km
∴ AB = AC - BC
⇒ 160 = 8x - 8y
⇒ x - y = 20   ...(1)
Case-II: [Cars are moving in opposite directions]

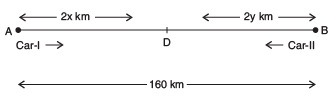
Let, after 2 hours, the cars meet at D.
∴ Distance cover after 2 hours,
by car-I = AD = 2x km
by car-II = BD = 2y km
⇒ AB = AD + BD
⇒ 160 = 2x + 2y
⇒ 80 = x + y
⇒ x + y = 80 ...(2)
Adding (1) and (2), we get
x + y = 80
x - y = 20
2x = 100
⇒ x = 100/2 = 50
⇒ Substituting x = 50 in (1), we get
x - y = 20 ⇒ 50 - y = 20
⇒ y = 50 - 20 = 30
⇒ Speed of car-I = 50 km/hr
Speed of car-II = 30 km/hr.

Q10: Solve for x and y:

axb - bya =  a + b 

ax - by = 2ab 
Sol: We have:
= axb - bya = a + b ...(1)
ax − by = 2ab    ...(2)
Dividing (2) by a, we have:

x -  bya = 2b 

⇒  bya =  x - 2b  ... (3)

From (1) and (3), we have:

axb - (x - 2b) =  a + b 

⇒ axb -  x + 2b - a - b = 0 

⇒ axb - x + b - a = 0

⇒ x ( ab  - 1 ) = - (b - a)

⇒ x ( a - bb ) = - (b - a)

⇒ x = - (b - a) x  b(a - b)

 ⇒ x = - (b - a) x  b- (b - a) = b
From (2),
ab - by = 2ab
⇒ - by = 2ab - ab = ab
⇒ -y =  abb =  a 
y = - a
hus, x = b and y = - a.

Q11: The sum of two numbers is 8. Determine the numbers if the sum of their reciprocals is 8/15.

Sol: Let the two numbers be x and y.
According to the conditions:
x + y = 8 ...(1)
=  1x + 1y = 815 ... (2)
From (1), x = (8 - y)
Substituting x = (8 - y) in (2),

1(8 - y) + 1y = 815

⇒ y + 8 - y(8 - y)y = 815

⇒ 8 × 15 = 8 × y (8 - y)
⇒ 64y - 8y² - 120 = 0
⇒- y² + 8y - 15 = 0
⇒ y² - 8y + 15 = 0
⇒ y² - 5y - 3y + 15 = 0
⇒ y (y - 5) - 3 (y - 5) = 0
⇒ (y - 5) (y - 3) = 0
⇒ If y - 5 = 0 then y = 5
or if y - 3 = 0, then y = 3
Since x = 8 - y
⇒ when y = 5,
then x = 8 - 5 = 3
when y = 3, then
x = 8 - 3 = 5
⇒ The required numbers are (3, 5) or (5, 3).

Q12: Solve the following pair of equations:

5x - 1 + 1y - 2 =  2 

6x - 1 - 3y - 2 =  1 

Sol: Let p = 1x - 1  and q = 1y - 2  

⇒The given system of equations becomes:
5p + q = 2 ...(1)
6p - 3q = 1 ...(2)
Multiplying (1) by 3 and adding to (2),

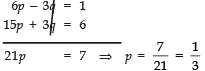
 ⇒21p = 7 

⇒p = 721 = 13 

⇒ p =  13

and 5p + q = 2 

 ⇒ 5(13) + q = 2

⇒  53 + q = 2

⇒ q = 2 - 53 = 13
⇒ q =  13 
Since, p = 1x - 1
therefore 1x - 1 = 13  ⇒  x - 1 = 3
⇒ x = 4
Also, q = 1y - 2
⇒ y - 2 = 3 ⇒ y = 5

Q13: Solve the following pair of equations:

10x + y + 2x − y = 4

15x + y − 5x − y = −2

Sol: Let 1x + y = p and 1x − y = q
⇒ The given pair of equation is expressed as
10p + 2q = 4 ⇒ 5p + q = 2   ...(1)
15p - 5q = - 2 ...(2)
Multiplying (1) by 5 and adding to (2)

From (1), 5 ( 51 ) + q = 2

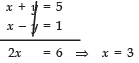
From (3), 3 + y = 5 ⇒ y = 2
Thus, x = 3 and y = 2.

Q14: Solve for x and y:
37x + 43y = 123
43x + 37y = 117

Sol: We have:
37x + 43y = 123 ...(1)
43x + 37y = 117 ...(2)
Adding (1) and (2)

Dividing both sides by 80, we get
x + y = 3 ...(3)
Subtracting (2) from (1),
- 6x + 6y = 6 ⇒ - x + y = 1 ...(4)
Adding:

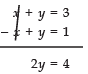
⇒ y = 4/2 = 2
Putting y = 2 in x + y = 3, we get
x + 2 = 3 ⇒ x = 3 - 2 = 1
Thus, x = 1 and y = 2.

Ques 15: Solve for ‘x’ and ‘y’:
(a - b) x + (a + b) y = a² - 2ab - b²
(a + b) (x + y) = a² + b²

Sol: We have:
(a - b) x + (a + b) y = a² - 2ab - b² ...(1)
(a + b) x + (a + b) y = a² + b²   ...(2)

− 2b x = − 2ab − b

⇒ (− 2b) x = − 2b (a + b)
⇒  x =  -2b(a + b)-2b = (a + b)
From (2),
(a + b) (a + b) + (a + b) y = a² + b²
⇒(a + b)2 + (a + b) y = (a² + b²)
⇒ (a + b) y = (a² + b²) - (a + b)²
⇒ (a + b) y = a² + b² - (a² + b² + 2ab)
⇒ (a + b) y = a² + b² - a² - b² - 2ab
⇒ (a + b) y = - 2ab
⇒ y = -2ab(a + b)
Thus, x = (a + b) and
y = -2ab(a + b)

Q16: Represent the following pair of equations graphically and write the co-ordinates of points where the lines intersect y-axis:
x + 3y = 6, 2x - 3y = 12
Sol: We have:
x + 3y = 6

And 2x - 3y = 12:

Plotting the above points, we get two straight lines l₁ and l₂ such that they intersect at (6, 0) as shown below:

Obviously,
The line l₁ meets the y-axis at (0, 2).
The line l₂ meets the y-axis at (0, - 4).

Q17: Solve for x and y:

5x - 1 + 1y - 2 = 2

6x - 1 - 3y - 2 = 1

Sol: Given equations:

5x - 1 + 1y - 2 = 2

6x - 1 - 3y - 2 = 1

Substituting u = 1x - 1 and v = 1y - 2, we get:
5u + v = 2
6u - 3v = 1
Solving these equations:
From 5u + v = 2:
v = 2 - 5u
Substituting v = 2 - 5u into 6u - 3v = 1:
6u - 3(2 - 5u) = 1
6u - 6 + 15u = 1
21u = 7
u = 13
Finding v:
v = 2 - 5 × 13 = 13
Back-substituting to find x and y:
u = 1x - 1 ⇒ x - 1 = 3 ⇒ x = 4
v = 1y - 2 ⇒ y - 2 = 3 ⇒ y = 5
Solution:
x = 4, y = 5

Q18: For what values of ‘a’ and ‘b’ does the following pair of equations have an infinite number of solutions?
2x + 3y = 7
a (x + y) - b (x - y) = 3a + b - 2
Sol: We have:
2x + 3y = 7   ...(1)
a (x + y) - b (x - y) = 3a + b - 2   ...(2)
From (2), we have:
a (x + y) - b (x - y) = 3a + b - 2
⇒ ax + ay - bx + by = 3a + b - 2
⇒ ax - bx + ay + by = 3a + b - 2
⇒ (a - b) x + (a + b) y = 3a + b - 2
Now, A₁ = 2, B₁= 3,
C₁ = - 7
A₂ = (a - b), B₂
= (a + b),
C₂ = - [3a + b - 2]
For infinite number of solutions,
A₁A₂ = B₁B₂ = C₁C₂
i.e., 2a - b = 3a + b = 73a + b - 2
∴ 2a - b = 3a + b
⇒ 2(a + b) = 3(a - b)
⇒ 2a + 2b = 3a - 3b
⇒ -a + 5b = 0
⇒ a = 5b ...(3)
Also, 3a + b = 73a + b - 2

⇒ 3 (3a + b - 2) = 7 (a + b)
⇒ 9a + 3b - 6 = 7a + 7b
⇒ 9a - 7a + 3b - 7b = 6
⇒ 2a - 4b = 6
⇒ a - 2b = 3    ...(4)
From (3) and (4),
5b - 2b = 3
⇒ 3b = 3 ⇒ b = 1
Thus, a = 5 × b
⇒ a = 5 × 1 = 5
i.e., a = 5 and b = 1.

Q19: Solve the following pairs of equations for x and y:

15x - y + 22x + y = 5

40x - y + 55x + y = 13 [x ≠ y and x ≠ -y]

Sol:  We are given the equations:

15x - y + 22x + y = 5

40x - y + 55x + y = 13

Let us substitute:
u = 1x - y, v = 1x + y
The equations become:
15u + 22v = 5
40u + 55v = 13
From the first equation:
u = 5 - 22v15
Substitute this value into the second equation:
40 × 5 - 22v15 + 55v = 13
Multiply through by 15:
200 - 880v + 825v = 195
Combine like terms:
200 - 55v = 195
Simplify:
-55v = -5 → v = 111
Substitute v back into the first equation:
15u + 22 × 111 = 5
Simplify:
15u + 2 = 5 → 15u = 3 → u = 15
Now back-substitute:
x - y = 5, x + y = 11
Add the equations:
2x = 16 → x = 8
Subtract the equations:
2y = 6 → y = 3
Final Answer: x = 8, y = 3

Q20: Draw the graph of the pair of linear equations x – y + 2 = 0 and 4x – y – 4 = 0. Calculate the area of triangle formed by the lines so drawn and the x-axis. 
Sol: To draw the graph of the given pair of equations, we have the table of ordered pairs
x - y + 2 = 0

4x - y - 4 = 0

Plot the points A(0, 2), B(–2, 0); C(0, –4) and D(1, 0) on the graph paper and join the points to form the lines AB and CD :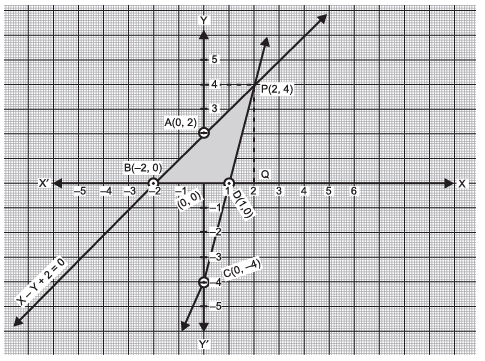

From the graph, we find that the points P(2, 4) is common to both the lines AB and CD.
These lines meet the x-axis at B(–2, 0) and D(1, 0).
Thus, the triangle BDP is formed by the lines and the x-axis.
The vertices of this Δ are
B(–2, 0), D(1, 0)  and    P(2, 4)
Now, the base of ΔBDP = BD
= (BO + OD)
= (2 + 1) units
= 3 units
Altitude of the ΔBDP = PQ
= 4 units
∴ Area of ΔBDP = 12 × base × altitude
= 12 × BD × PQ
= 12 × 3 × 4 units
= 6 sq. units

Q21: It can take 12 hours to fill a swimming pool using two pipes. If the pipe of larger diameter is used for 4 hours and the pipe of smaller diameter for 9 hours, only half the pool can be filled. How long would it take for each pipe to fill the pool separately?
Sol: Let the time taken by the pipe of larger diameter to fill the pool separately = x hours.
The time taken by the pipe of smaller diameter to fill the pool separately = y hours.
∴ Part of the pool fill by a pipe of larger diameter in 1 hour = 1/x.
Part of fool filled by the pipe of larger diameter in 4 hours = 4/x.
Similarly, Part of the pool filled by the pipe of smaller diameter in 9 hours = 9/y.
∴ We have 4x + 9y = 12 ...(1)
Since the pool is filled by both the pipes together in 12 hours.
∴ 12x + 12y = 1 ...(2)
To solve (1) and (2), multiplying (1) by 3 and subtracting (2) from it, we have
3[ 4x + 9y] − [ 12x + 12y] = 1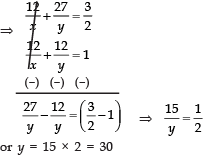

Substituting, y = 30 in (2), we have

12x + 1230 = 1
⇒ 12x = 1 − 25 = 1830 = 120
∴ x = 20
⇒ Required time taken by pipe of larger diameter = 20 hours
⇒ Required time taken by pipe of smaller diameter = 30 hours