MCQs with Solutions: Quadrilaterals - 2 | Mathematics (Maths) Class 9 PDF Download
Q1: Two parallelogram stand on equal bases and between the same parallels. The ratio of their areas is
(a) It is 3:1
(b) It is 1:2
(c) It is 2:1
(d) It is 1:1
Ans: d
Q2: In quadrilateral ABCD, if ∠A = 60∘ and ∠B : ∠C : ∠D = 2:3:7, then ∠D is :
(a) 25∘
(b) 175∘
(c) 180∘
(d) 50∘
Ans: b
Q3: D and E are the mid-points of the sides AB and AC res. Of △ABC. DE is produced to F. To prove that CF is equal and parallel to DA, we need an additional information which is:
(a) AE = EF
(b) ∠ADE = ∠ECF
(c) ∠DAE = ∠EFC
(d) DE = EF
Ans: d
Q4: P, Q, R are the mid- points of AB, BC, AC res, If AB = 10cm, BC = 8cm, AC = 12cm, Find the perimeter of △PQR.
(a) 15.5cm
(b) 14cm
(c) 15cm
(d) 13cm
Ans: c
Q5: In Triangle ABC which is right angled at B. Given that AB = 9cm, AC = 15cm and D, E are the mid-points of the sides AB and AC res. Find the length of BC?
(a) 12cm
(b) 13cm
(c) 13.5cm
(d) 15cm
Ans: a
Q6: Three Statements are given below:
(I) In a, Parallelogram the angle bisectors of 2 adjacent angles enclose a right angle.
(II) The angle bisector of a Parallelogram form a Rectangle.
(III) The Triangle formed by joining the mid-points of the sides of an isosceles triangle is not necessarily an isosceles triangle. Which is True?
(a) II
(b) I and II
(c) I and III
(d) I
Ans: b
Q7: If APB and CQD are 2 parallel lines, then the bisectors of the angles APQ, BPQ, CQP and PQD form, square only if
(a) ABCD is a Rhombus
(b) ABCD are equal
(c) Diagonals of ABCD are equal
(d) None of these
Ans: d
Sol: Line APB is parallel to CQD
when we join PQ it will be transversal
then angle BPQ=angle CQP (alternate angles)
angle APQ=angle PQD
when we will draw bisectors
then the figure formed will have opposite angles equal
which means that it is a parallelogram
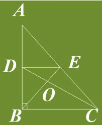
Q8: If bisectors of ∠A and ∠B of a quadrilateral ABCD intersect each other at P, of ∠B and ∠C at Q, of ∠C and ∠D at R and of ∠D and ∠A at S, then PQRS is a
(a) Rectangle
(b) Quadrilateral whose opposite angles are supplementary
(c) Parallelogram
(d) Rhombus
Ans: b
Sol: To show: ∠PSR + ∠PQR = 180°
∠SPQ + ∠SRQ = 180°
In △DSA,
∠DAS + ∠ADS + ∠DSA = 180° (angle sum property)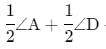
+ ∠ SA = 180° (since RD and AP are bisectors of ∠D and ∠A)
∠DSA = 180°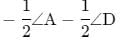
∠PSR = 180°−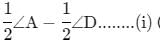
(∵ ∠DSA = ∠PSR are vertically opposite angles)
Similarly,
∠PQR = 180°− 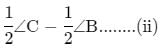
Adding (i) and (ii), we get, ∠PSR + ∠PQR = 180°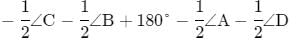
=360° − 1/2 × (∠A + ∠B + ∠C + ∠D)
=360°− 1/2 × 360° = 180° ∴ ∠PSR + ∠PQR = 180°
In quadrilateral PQRS,
∠SPQ + ∠SRQ + ∠PSR + ∠PQR = 360°
=> ∠SPQ + ∠SRQ + 180° = 360°
=> ∠SPQ + ∠SRQ = 180°
Hence, showed that opposite angles of PQRS are supplementary.
Q9: The Diagonals AC and BD of a Parallelogram ABCD intersect each other at the point O such that ∠DAC = 30∘ and ∠AOB = 70∘. Then, ∠DBC?
(a) 30∘
(b) 45∘
(c) 35∘
(d) 40∘
Ans: d
Q10: In Parallelogram ABCD, bisectors of angles A and B intersect each other at O. The measure of ∠AOB is
(a) 120∘
(b) 60∘
(c) 90∘
(d) 30∘
Ans: c
Q11: Three statements are given below:
(I) In a Rectangle ABCD, the diagonals AC bisects ∠A as well as ∠C.
(II) In a Square ABCD, the diagonals AC bisects ∠A as well as ∠C.
(III) In rhombus ABCD, the diagonals AC bisects ∠Aas well as ∠C.
Which is True?
(a) III
(b) I
(c) II and III
(d) II
Ans: c
Q12: D and E are the mid-points of the sides AB and AC of △ABC and O is any point on the side BC, O is joined to A. If P and Q are the mid-points of OB and OC res, Then DEQP is
(a) A Rectangle
(b) A Triangle
(c) A Rhombus
(d) A Parallelogram
Ans: d
Q13: Given Rectangle ABCD and P, Q, R and S are the mid-points of the sides AB, BC, CD and DA res. If length of a diagonal of Rectangle is 8cm, then the quadrilateral PQRS is a
(a) Rectangle with one side 4cm
(b) Parallelogram with one side 4cm
(c) Square with one side 4 cm
(d) Rhombus with each side 4cm
Ans: d
Q14: In the given figure, ABCD is a Rhombus. Then,
(a) AC2+ BD2 = 4AB2
(b) AC2+ BD2 = 2AB2
(c) (AC2+ BD2) = 3AB2
(d) AC2+ BD2 = AB2
Ans: a
Q15: D and E are the mid-points of the sides AB and AC. Of △ABC. If BC = 5.6cm, find DE.
(a) 3cm
(b) 2.5cm
(c) 2.9cm
(d) 2.8cm
Ans: d
Q16: In a triangle P, Q and R are the mid-points of the sides BC, CA and AB res. If AC = 21cm, BC = 29cm and AB = 30cm, find the perimeter of the quadrilateral ARPQ?
(a) 52cm
(b) 51cm
(c) 80cm
(d) 20cm
Ans: b
Q17: The bisectors of the angles of a Parallelogram enclose a
(a) Rhombus
(b) Square
(c) Parallelogram
(d) Rectangle
Ans: d
Q18: Opposite angles of a Quadrilateral ABCD are equal. If AB = 4cm, find the length of CD.
(a) 4cm
(b) 3cm
(c) 5cm
(d) 2cm
Ans: a
Q19: In a Trapezium ABCD, if AB ║ CD, then (AC2+ BD2) = ?
(a) BC2+ AD2+ 2AB.CD
(b) BC2+ AD2+ 2BC.AD
(c) AB2+ CD2+ 2AB.CD
(d) AB2+ CD2+ 2AD.BC
Ans: a
Q20: In quadrilateral ABCD, ∠B=90∘, ∠C−∠D = 60∘ and ∠A−∠C−∠D = 10∘. Find ∠A, ∠C and ∠D.
(a) 140∘, 95∘, 35∘
(b) 145∘, 55∘, 20∘
(c) 150∘, 60∘, 80∘
(d) None of these
Ans: a
Q21: If a Quadrilateral ABCD,∠A = 90∘ and AB = BC = CD = DA, Then ABCD is a
(a) Rectangle
(b) Parallelogram
(c) Square
(d) Triangle
Ans: c
Q22: Rhombus is a quadrilateral
(a) in which diagonals are at right angle
(b) in which diagonals are inclines at an angle of 60∘
(c) in which diagonals bisect each other
(d) in which diagonals bisect opposite angles
Ans: d
Q23: In △ABC, EF is the line segment joining the mid-points of the sides AB and AC. BC = 7.2cm, Find EF.
(a) 3.6cm
(b) 3.4cm
(c) 2.6cm
(d) 3.5cm
Ans: a
Q24: In the figure, ABCD is a rhombus, whose diagonals meet at 0. Find the values of x and y. 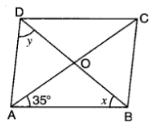
(a) 55° and 55°
(b) 35 and 35
(c) 37 and 37
(d) 45 and 45
Ans: a
Sol:
Since diagonals of a rhombus bisect each other at right angle .
∴ In △AOB , we have
∠OAB + ∠x + 90° = 180°
∠x = 180° - 90° - 35° [∵ ∠OAB = 35°]
= 55°
Also, ∠DAO = ∠BAO = 35°
∴ ∠y + ∠DAO + ∠BAO + ∠x = 180°
⇒ ∠y + 35° + 35° + 55° = 180°
⇒ ∠y = 180° - 125° = 55°
Hence the values of x and y are x = 55°, y = 55°.
Q25: The diagonals AC and BD of a parallelogram ABCD intersect each other at the point O. If ∠DAC = 32∘ and ∠AOB = 70∘ then, ∠DBC is equal to
(a) 24∘
(b) 38∘
(c) 40∘
(d) 86∘
Ans: b
|
40 videos|471 docs|57 tests
|
FAQs on MCQs with Solutions: Quadrilaterals - 2 - Mathematics (Maths) Class 9
| 1. What are the properties of a quadrilateral? |  |
| 2. How many types of quadrilaterals are there? |  |
| 3. What is the difference between a square and a rectangle? |  |
| 4. How can you determine if a quadrilateral is a parallelogram? |  |
| 5. What are the properties of a rhombus? |  |





















