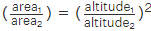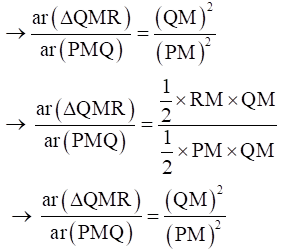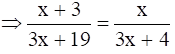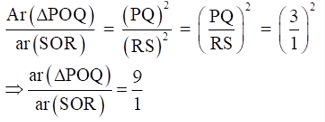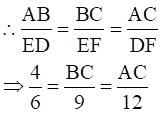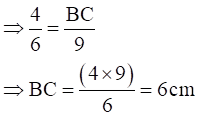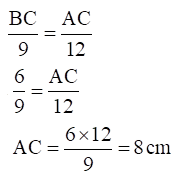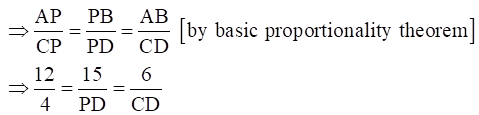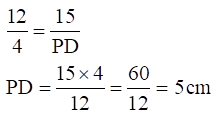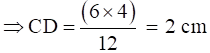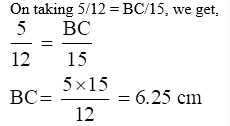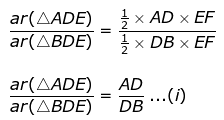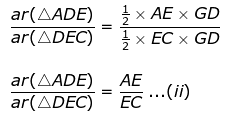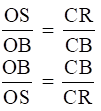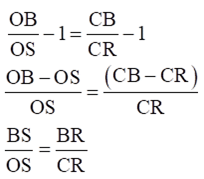NCERT Exemplar: Triangles | Mathematics (Maths) Class 10 PDF Download
| Table of contents |

|
| Exercise 6.1 |

|
| Exercise 6.2 |

|
| Exercise 6.3 |

|
| Exercise 6.4 |

|
Exercise 6.1
Q.1. In figure, if ∠BAC =90° and AD⊥BC. Then,
(a) BD.CD = BZC²
(b) AB.AC = BC²
(c) BD.CD=AD²
(d) AB.AC =AD²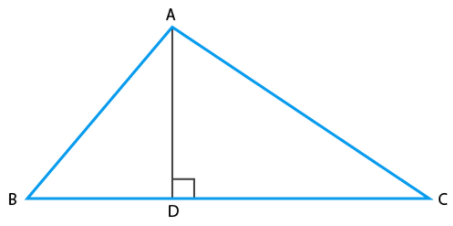
Correct Answer is option (c)
From ∆ADB and ∆ADC,
According to the question, we have,
∠D = ∠D = 90° (∵ AD ⊥ BC)
∠DBA = ∠DAC [each angle = 90°- ∠C]
Using AAA similarity criteria,
∆ADB ∼ ∆ADC
BD/AD = AD/CD
BD.CD = AD2
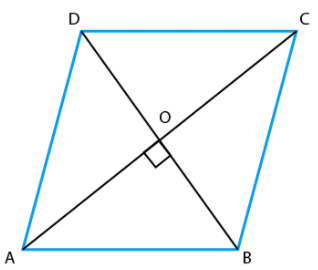
Q.2. If the lengths of the diagonals of rhombus are 16 cm and 12 cm. Then, the length of the sides of the rhombus is
(a) 9 cm
(b) 10 cm
(c) 8 cm
(d) 20 cm
Correct Answer is option (b)
We know that,
A rhombus is a simple quadrilateral whose four sides are of same length and diagonals are perpendicular bisector of each other.
According to the question, we get,
According to the question,
AC = 16 cm and BD = 12 cm
∠AOB = 90°
∵ AC and BD bisects each other
AO = ½ AC and BO = ½ BD
Then, we get,
AO = 8 cm and BO = 6 cm
Now, in right angled ∆AOB,
Using the Pythagoras theorem,
We have,
AB2 = AO2 + OB2
AB2 = 82 + 62 = 64 + 36 = 100
∴ AB = √100 = 10 cm
We know that the four sides of a rhombus are equal.
Therefore, we get, one side of rhombus = 10 cm.
Q.3. If ΔABC ~ ΔEDF and ΔABC is not similar to ΔDEF, then which of the following is not true?
(a) BC · EF = AC · FD
(b) AB · EF = AC · DE
(c) BC · DE = AB · EF
(d) BC · DE = AB · FD
Correct Answer is option (c)
We know that,
If sides of one triangle are proportional to the side of the other triangle, and the corresponding angles are also equal, then the triangles are similar by SSS similarity.
So, ∆ABC ∼ ∆EDF
Using similarity property,
AB/ED = BC/DF = AC/EF
Taking AB/ED = BC/DF, we get
AB/ED = BC/DF
AB.DF = ED.BC
So, option (d) BC · DE = AB · FD is true
Taking BC/DF = AC/EF, we get
BC/DF = AC/EF
⇒ BC.EF = AC.DF
So, option (a) BC · EF = AC · FD is true
Taking AB/ED = AC/EF, we get,
AB/ED = AC/EF
AB.EF = ED.AC
So, option (b) AB · EF = AC · DE is true.
Q.4. If in two Δ PQR,AB/QR = BC/PR = CA/PQ, then
(a) Δ PQR~Δ CAB
(b) Δ PQR ~ Δ ABC
(c) Δ CBA ~ Δ PQR
(d) Δ BCA ~ Δ PQR
Correct Answer is option (a)
From ∆ABC and ∆PQR, we have,
AB/QR = BC/PR = CA/PQ
If sides of one triangle are proportional to the side of the other triangle, and their corresponding angles are also equal, then both the triangles are similar by SSS similarity.
Therefore, we have,
Δ PQR~Δ CAB
Q.5. In figure, two line segments AC and BD intersect each other at the point P such that PA = 6 cm, PB = 3 cm, PC = 2.5 cm, PD = 5 cm, ∠APB = 50° and ∠CDP = 30°. Then, ∠PBA is equal to
(a) 50°
(b) 30°
(c) 60°
(d) 100°
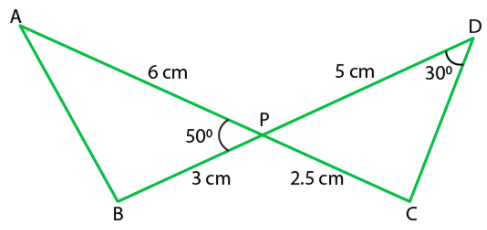
Correct Answer is option (d)
From ∆APB and ∆CPD,
∠APB = ∠CPD = 50° (since they are vertically opposite angles)
AP/PD = 6/5 … (i)
Also, BP/CP = 3/2.5
Or BP/CP = 6/5 … (ii)
From equations (i) and (ii),
We get,
AP/PD = BP/CP
So, ∆APB ∼ ∆DPC [using SAS similarity criterion]
∴ ∠A = ∠D = 30° [since, corresponding angles of similar triangles]
Since, Sum of angles of a triangle = 180°,
In ∆APB,
∠A + ∠B + ∠APB = 180°
So, 30° + ∠B + 50° = 180°
Then, ∠B = 180° – (50° + 30°)
∠B = 180 – 80° = 100°
Therefore, ∠PBA = 100°
Q.6. If in two triangles DEF and PQR, ∠D = ∠Q and ∠R = ∠E, then which of the following is not true?
(a) EF/PR = DF/PQ
(b) DE/PQ = EF/RP
(c) DE/QR = DF/PQ
(d) EF/RP = DE/QR
Correct Answer is option (b)
Given, the two triangles DEF and PQR.
Also, ∠D = ∠Q and ∠R = ∠E
We have to find the true statement from the options.As ∠D = ∠Q and ∠R = ∠E
It is clear that the corresponding angles are equal. So, the third angle will also be equal.
AAA criterion states that if in two triangles, corresponding angles are equal, then their corresponding sides are in the same ratio and hence the two triangles are similar.
By AAA criterion,
The triangles DEF and PQR are similar.
The corresponding sides will be
DF/PQ = DE/QR = EF/PR
Taking DF/PQ = DE/QR
Therefore, option C is true.
Taking DE/QR = EF/PR
Therefore, option D is true
Taking DF/PQ = EF/PR
Therefore, option A is true.
Q.7. In triangles ABC and DEF, ∠B = ∠E, ∠F = ∠C and AB = 3 DE. Then, the two triangles are
(a) congruent but not similar
(b) similar but not congruent
(c) neither congruent nor similar
(d) congruent as well as similar
Correct Answer is option (b)
Given, in the triangles ABC and DEF, ∠B = ∠E, ∠F = ∠C
Also, AB = 3DE
We have to find if the triangles are similar and congruent or not.
AAA criterion states that if two angles of a triangle are respectively equal to two angles of another triangle, then by the angle sum property of a triangle their third angle will also be equal.
By AAA criterion,
As ∠B = ∠E and ∠F = ∠C, the third angle will also be equal.
i.e. ∠D = ∠A
Therefore, the triangles ABC and DEF are similar.In triangles ABC and DEF, ∠B = ∠E, ∠F = ∠C and AB = 3 DE. Then, the two triangles areIt is given that AB = 3DEIt is clear that the sides AB and DE are not equal So, the triangles are not congruent. Therefore, the triangles ABC and DEF are similar but not congruent.
Q.8. It is given that △ABC ~ △PQR, with BC/QR = 1/3. Then ar(PRQ)/ar(BCA) is equal to
(a) 9
(b) 3
(c) 1/3
(d) 1/9
Correct Answer is option (a)
Given, the triangles ABC and PQR are similar.
BC/QR = 1/3
We have to find the ratio of the area of the triangles PQR and BCA.
We know that the ratio of the areas of two similar triangles is equal to the ratio of the squares of their corresponding sides.
So, area of PQR/area of BCA = QR2/BC2
= (3)2/1
= 9/1
Therefore, ar(PRQ)/ar(BCA) = 9
Q.9. It is given that ΔABC ~ ΔDFE, ∠A =30°, ∠C = 50°, AB = 5 cm, AC = 8 cm and DF= 7.5 cm. Then, the following is true:
(a) DE = 12 cm, ∠F = 50°
(b) DE = 12 cm, ∠F = 100°
(c) EF = 12 cm, ∠D = 100°
(d) EF = 12 cm, ∠D = 30°
Correct Answer is option (b)
Given, the triangles ABC and DEF are similar.
Also, ∠A =30° and ∠C = 50°
The length of the sides
AB = 5 cm
AC = 8 cm
DF = 7.5 cm
We have to determine the correct solution from the given options.Since the two triangles are similar, the corresponding sides will be
AB/DF = AC/DE = BC/EF
5/7.5 = 8/DE = BC/EF
Taking 5/7.5 = 8/DE
On cross multiplication,
5(DE) = 8(7.5)
DE = 60/5
DE = 12 cm
In triangle ABC,
∠A + ∠B + ∠C = 180°
30° + ∠B + 50° = 180°
∠B = 180° - 80°
∠B = 100°
As the triangles are similar, the corresponding angles will be equal.
So, ∠B = ∠F = 100°
Therefore, DE = 12 cm and ∠F = 100°
Q.10. If in triangles ABC and DEF, AB/DE = BC/FD, then they will be similar, when
(a) ∠B = ∠E
(b) ∠A = ∠D
(c) ∠B = ∠D
(d) ∠A = ∠F
Correct Answer is option (c)
Given, the triangles ABC and DEF
Also, AB/DE = BC/FD
We have to choose the correct condition so the triangles will be similar.SSS criterion states that if three sides of one triangle are proportional to three sides of another triangle, then the triangles are similar.
By SSS criterion,
AB/DE = BC/DF = AC/EF
We know that similar triangles have congruent corresponding angles and the corresponding sides are in proportion.
So, ∠A = ∠E
∠B = ∠D
∠C = ∠F
So, option C is true.
Therefore, the triangles to be similar when ∠B = ∠D.
Q.11. If △ABC ~ △QRP, ar(ABC)/ar(PQR) = 9/4, AB = 18 cm and BC = 15 cm, then PR is equal to
(a)10 cm
(b) 12 cm
(c) 20/3 cm
(d) 8 cm
Correct Answer is option (a)
Given, the triangles ABC and PQR are similar.
Area of ABC/Area of PQR = 9/4
The length of the sides
AB = 18 cm
BC = 15 cm
We have to find the length of PR.We know that the ratio of the areas of two similar triangles is equal to the ratio of the squares of their corresponding sides.
So, area of ABC/area of PQR = BC2/RP2
9/4 = (15)2/RP2
Taking square root,
3/2 = 15/RP
On cross multiplication,
3(RP) = 15(2)
RP = 30/3
RP = 10 cm
Therefore, the length of PR is 10 cm.
Q.12. If S is a point on side PQ of a △PQR such that PS = QS = RS, then
(a) PR . QR = RS2
(b) QS2 + RS2 = QR2
(c) PR2 + QR2 = PQ2
(d) PS2 + RS2 = PR2
Correct Answer is option (c)
Given, S is a point on side PQ of a triangle PQR.
Also, PS = QS = RSIn triangle PSR,
Given, PS = RS
We know that angles opposite to equal sides in a triangle are equal
∠P = ∠R
So, ∠P = ∠1
Similarly, in triangle RSQ
Given, RS = QS
∠R = ∠Q
So, ∠Q = 2
Considering triangle PQR,
We know that the sum of all three interior angles of a triangle is always equal to 180 degrees.
So, ∠P + ∠Q + ∠PRQ = 180°
∠1 + ∠2 + ∠PRQ = 180°
AAA criterion states that if two angles of a triangle are respectively equal to two angles of another triangle, then by the angle sum property of a triangle their third angle will also be equal.
So, ∠PRQ = ∠1 + ∠2
Now, ∠1 + ∠2 + ∠1 + ∠2 = 180°
2(∠1 + ∠2) = 180°
∠1 + ∠2 = 180°/2
∠1 + ∠2 = 90°
∠PRQ = 90°
So PRQ is a right triangle with right angle at R.
By Pythagoras theorem,
PQ2 = PR2 + QR2
Therefore, option C is true.
Exercise 6.2
Q.1. Is the triangle with sides 25 cm, 5 cm and 24 cm a right triangle? Give reason for your answer.
False
According to the question,
Let us assume that,
A = 25 cm
B = 5 cm
C = 24 cm
Now, Using Pythagoras Theorem,
We have,
A2 = B2 + C2
B2 + C2 = (5)2 + (24)2
B2 + C2 = 25 + 576
B2 + C2 = 601
A2 = 625
625 ≠ 601
A2 ≠ B2 + C2
Since the sides does not satisfy the property of Pythagoras theorem, triangle with sides 25cm, 5cm and 24cm is not a right triangle.
Q.2. It is given that ΔDEF ~ ΔRPQ. Is it true to say that ∠D = ∠R and ∠F = ∠P ? Why?
False
We know that,
Corresponding angles are equal in similar triangles.
So, we get,
∠D = ∠R
∠E = ∠P
∠F = ∠Q
Q.3. A and B are respectively the points on the sides PQ and PR of a ΔPQR such that PQ = 12.5 cm, PA = 5 cm, BR = 6 cm and PB = 4 cm. Is AB || QR? Give reason for your answer.
True
According to the question,PQ = 12.5 cm
PA = 5 cm
BR = 6 cm
PB = 4 cm
Then,
QA = QP – PA = 12.5 – 5 = 7.5 cm
So,
PA/AQ = 5/7.5 = 50/75 = 2/3 … (i)
PB/BR = 4/6 = 2/3 … (ii)
Form Equations (i) and (ii).
PA/AQ = PB/BR
We know that, if a line divides any two sides of a triangle in the same ratio, then the line is
parallel to the third side.
Therefore,
AB || QR.
Q.4. In figure, BD and CE intersect each other at the point P. Is ΔPBC ~ ΔPDE? Why?
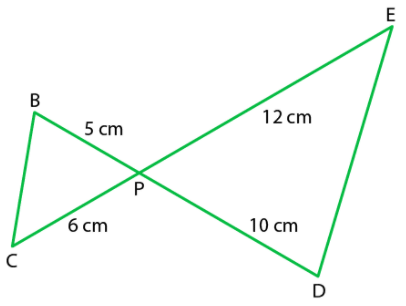
True
In ∆PBC and ∆PDE,
∠BPC = ∠EPD [vertically opposite angles]
PB/PD = 5/10 = ½ … (i)
PC/PE = 6/12 = ½ … (ii)
From equation (i) and (ii),
We get,
PB/PD = PC/PE
Since, ∠BPC of ∆PBC = ∠EPD of ∆PDE and the sides including these.
Then, by SAS similarity criteria
∆PBC ∼ ∆PDE
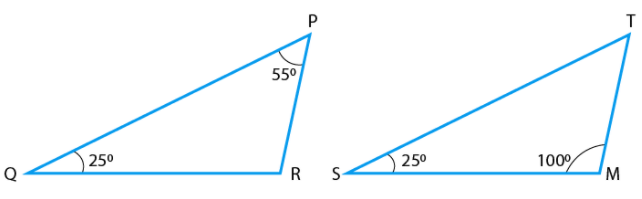
Q.5. In ΔPQR and ΔMST, ∠P = 55°, ∠Q =25°, ∠M = 100° and ∠S = 25°. Is ΔQPR ~ ΔTSM? Why
We know that,
Sum of the three angles of a triangle = 180°.
Then, from ∆PQR,
∠P + ∠Q + ∠R = 180°
55° + 25° + ∠R = 180°
So, we get,
∠R = 180° – (55° + 25°) = 180°- 80° = 100°
Similarly, from ∆TSM,
∠T + ∠S + ∠M = 180°
∠T + ∠25° + 100° = 180°
So, we get,
∠T = 180°- (∠25° + 100°)
∠T = 180° – 125° = 55°
In ∆PQR and ∆TSM,
We have,
∠P = ∠T,
∠Q = ∠S
∠R = ∠M
Hence, ∆PQR ∼ ∆TSM
Since, all corresponding angles are equal,
∆QPR is similar to ∆TSM,
Q.6. Is the following statement true? Why?
“Two quadrilaterals are similar, if their corresponding angles are equal”.
False
Two quadrilaterals cannot be similar, if only their corresponding angles are equal.
Q.7. Two sides and the perimeter of one triangle are respectively three times the corresponding sides and the perimeter of the other triangle. Are the two triangles similar? Why?
Given, the two sides and the perimeter of one triangle are three times the corresponding sides and the perimeter of the other triangle.
We have to determine if the two triangles are similar.
The perimeter of a triangle is the sum of all three sides of a triangle.
Let us consider the first triangle be ABC and the second triangle be PQR
The sides of the first triangle ABC are x, y, and z.
So, the sides of the second triangle PQR will be 3x, 3y and 3z.
Perimeter of the triangle ABC = x + y + z
Perimeter of the triangle PQR = 3x + 3y + 3z = 3(x + y + z)
It is obvious that the sides are increased by a factor 3.
The SSS similarity criterion states that if the three sides of one triangle are proportional to the three sides of another, then the two triangles are similar.
Here, the perimeter and the two sides of a triangle are in proportion.
So, the third side will also be proportional.
Therefore, the two triangles are similar.
Q.8 . If in two right triangles, one of the acute angles of one triangle is equal to an acute angle of the other triangle, can you say that the two triangles will be similar? Why?
Given, in two right triangles, one of the acute angles of one triangle is equal to an acute angle of the other triangle.
We have to check if the two triangles are similar.
Let the two right triangles be △ABC and △DEFFrom the above figure,
∠B = ∠E = 90°
Acute angles, ∠A = ∠D
We know that the sum of all the three interior angles of a triangle is always equal to 180°
In △ABC,
∠A + ∠B + ∠C = 180°
∠A + 90° + ∠C = 180°
∠A + ∠C = 180°-90°
∠C = 90° - ∠A
In △DEF,
∠D + ∠E + ∠F = 180°
∠D + 90° + ∠F = 180°
∠D + ∠F = 180°- 90°
∠F = 90° - ∠D
AAA criterion states that if in two triangles, corresponding angles are equal, then their corresponding sides are in the same ratio and hence the two triangles are similar
As, ∠A = ∠D
∠E = 90° - ∠A
So, ∠E = ∠C
It is clear that the corresponding angles are equal and the corresponding sides are equal.
Therefore, the two right triangles are similar.
Q.9 . The ratio of the corresponding altitudes of two similar triangles is 3/5. Is it correct to say that ratio of their areas is 6/5 ? Why?
Given, the ratio of the corresponding altitudes of two similar triangles is 3/5.
We have to determine if the ratio of their areas is 6/5.
We know that,
Similar triangles have congruent corresponding angles and the corresponding sides are in proportion.
Similar triangles have the same shape, but not the same size.
By the property of similar triangles,
LHS : area₁/area₂
= 6/5
= (3/5)2
= 9/25
LHS ≠ RHS
The ratio of areas is not equal to the ratio of the squares of the altitude.
Therefore, the ratio of the areas is not equal to 6/5.
Q.10. 1 0 . D is a point on side QR of ΔPQR such that PD ⊥ QR. Will it be correct to say that ΔPQD ~ ΔRPD? Why?
Given, D is a point on the side QR of △PQR.
PD⟂QR
We have to determine if the triangles PQD and RPD are similar.
We know that similar triangles have congruent corresponding angles and the corresponding sides are in proportion.From the above figure,
PD is the side common to both triangles PQD and RPD.
Since, PD⟂QR we have ∠PDQ = ∠RDP = 90°
Also, PD does not bisect ∠P.
∠QPD ≠ ∠RPD
∠PQD ≠ ∠PRD
This implies that ∠Q ≠ ∠R, so PR ≠ PQ
We observe that the ratio of the corresponding sides are not equal and the corresponding angles are not equal.
Therefore, the triangles PQD and RPD are not similar.
Q. 11 . In Figure below, if ∠D = ∠C, then is it true that ΔADE ~ ΔACB? Why?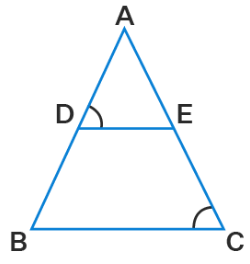
Given, in the triangles ADE and ACB ∠D = ∠C
We have to determine if the triangles ADE and ACB are similar.
From the figure,
It is clear that A is the common angle.
AAA criterion states that if two angles of a triangle are respectively equal to two angles of another triangle, then by the angle sum property of a triangle their third angle will also be equal.
By AAA criterion,
The third angle must be equal.
∠B = ∠E
So, △ADE ~ △ACB
Therefore, the triangles ADE and ACB are similar.
Q.12 . Is it true to say that if in two triangles, an angle of one triangle is equal to an angle of another triangle and two sides of one triangle are proportional to the two sides of the other triangle, then the triangles are similar? Give reasons for your answer.
Given, in two triangles an angle of one triangle is equal to an angle of another triangle.
Also, two sides of one triangle are proportional to the two sides of the other triangle.
We have to check if the triangles are similar.
SAS Similarity Theorem states that if two sides in one triangle are proportional to two sides in another triangle and the included angle in both are congruent, then the two triangles are similar.
Here, two sides of the triangles are equal but these sides do not include the equal angle.
Therefore, the two triangles cannot be similar.
Exercise 6.3
Q.1. In a ΔPQR, PR2 – PQ2 = QR2 and M is a point on side PR such that QM ⊥ PR.
Prove that QM2 =PM × MR.
According to the question,
In ∆PQR,
PR2 = QR2 and QM⊥PR
Using Pythagoras theorem, we have,
PR2 = PQ2 + QR2
∆PQR is right angled triangle at Q.
From ∆QMR and ∆PMQ, we have,
∠M = ∠M
∠MQR = ∠QPM [= 90°-∠R]
So, using the AAA similarity criteria,
We have,
∆QMR ∼ ∆PMQ
Also, we know that,
Area of triangles = ½ × base × height
So, by property of area of similar triangles,
QM2 = PM × RM
Hence proved.
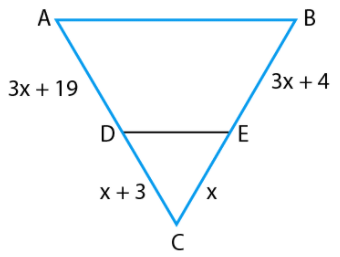
Q.2. Find the value of x for which DE||AB in given figure.
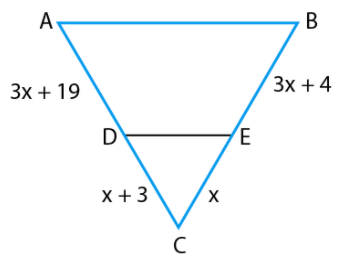
According to the question,
DE || AB
Using basic proportionality theorem,
CD/AD = CE/BE
∴ If a line is drawn parallel to one side of a triangle such that it intersects the other sides at distinct points, then, the other two sides are divided in the same ratio.
Hence, we can conclude that, the line drawn is equal to the third side of the triangle.
(x + 3) (3x + 4) = x (3x + 19)
3x2 + 4x + 9x + 12 = 3x2 + 19x
19x – 13x = 12
6x = 12
∴ x = 12/6 = 2
Q.3. In figure, if ∠1 =∠2 and ΔNSQ = ΔMTR, then prove that ΔPTS ~ ΔPRQ.
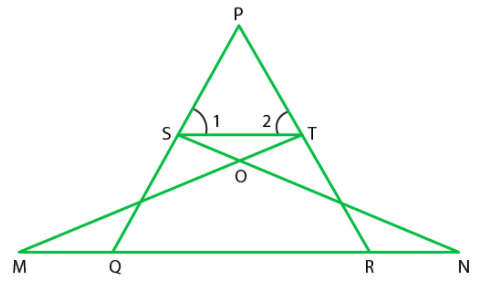
According to the question,
∆ NSQ ≅ ∆MTR
∠1 = ∠2
Since,
∆NSQ = ∆MTR
So,
SQ = TR ….(i)
Also,
∠1 = ∠2 ⇒ PT = PS….(ii)
[Since, sides opposite to equal angles are also equal]
From Equation (i) and (ii).
PS/SQ = PT/TR
⇒ ST || QR
By converse of basic proportionality theorem, If a line is drawn parallel to one side of a triangle to intersect the other sides in distinct points, the other two sides are divided in the same ratio.
∴ ∠1 = PQR
And
∠2 = ∠PRQ
In ∆PTS and ∆PRQ.
∠P = ∠P [Common angles]
∠1 = ∠PQR (proved)
∠2 = ∠PRQ (proved)
∴ ∆PTS – ∆PRQ
[By AAA similarity criteria]
Hence proved.
Q.4. Diagonals of a trapezium PQRS intersect each other at the point 0, PQ || RS and PQ = 3 RS. Find the ratio of the areas of Δ POQ and Δ ROS.
According to the question,
PQRS is a trapezium in which PQ‖RS and PQ = 3RS
PQ/RS = 3/1 = 3 …(i)In ∆POQ and ∆ROS,
∠SOR = ∠QOP [vertically opposite angles]
∠SRP = ∠RPQ [alternate angles]
∴ ∆POQ ∼ ∆ROS [by AAA similarity criterion]
By property of area of similar triangle,
Therefore, the required ratio = 9:1.
Q.5. In figure, if AB || DC and AC, PQ intersect each other at the point O. Prove that OA.CQ = OC.AP.
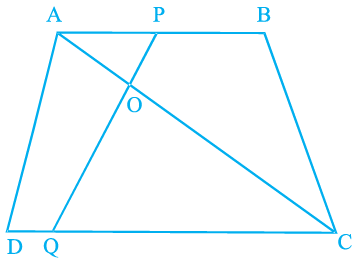
According to the question,
AC and PQ intersect each other at the point O and AB||DC.
From ∆AOP and ∆COQ,
∠AOP = ∠COQ [Since they are vertically opposite angles]
∠APO = ∠CQO [since, AB||DC and PQ is transversal, the angles are alternate angles]
∴ ∆AOP ∼ ∆COQ [using AAA similarity criterion]
Then, since, corresponding sides are proportional
We have,
OA/OC = AP/CQ
OA × CQ = OC × AP
Hence Proved.
Q.6. Find the altitude of an equilateral triangle of side 8 cm.
Let ABC be an equilateral triangle of side 8 cm
AB = BC = CA = 8 cm. (all sides of an equilateral triangle is equal)Draw altitude AD which is perpendicular to BC.
Then, D is the mid-point of BC.
∴ BD = CD = ½
BC = 8/2 = 4 cm
Now, by Pythagoras theorem
AB2 = AD2 + BD2
⇒ (8)2 = AD2 + (4)2
⇒ 64 = AD2 + 16
⇒ AD = √48 = 4√3 cm.
Hence, altitude of an equilateral triangle is 4√3 cm.
Q.7. If ∆ABC ∼ ∆DEF, AB = 4 cm, DE = 6, EF = 9 cm and FD = 12 cm, then find the perimeter of ∆ABC.
According to the question,
AB = 4 cm,
DE = 6 cm
EF = 9 cm
FD = 12 cm
Also,
∆ABC ∼ ∆DEF
We have,
By taking first two terms, we have
And by taking last two terms, we have,
Now,
Perimeter of ∆ABC = AB + BC + AC = 4 + 6 + 8 = 18 cm
Thus, the perimeter of the triangle is 18 cm.
Q.8. In Figure below, if DE||BC, find the ratio of ar (△ADE) and ar (△DECB)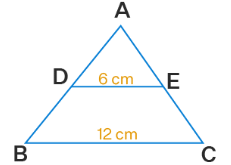
Given, DE||BC
We have to find the ratio of ar (△ADE) and ar (△DECB).
From the figure,
DE = 6 cm
BC = 12 cm
In △ADE and △ABC,
∠A = ∠A = common angle
By the property of similar triangles,
The corresponding angles are equal.
∠ABC = ∠ADE
∠BCA = ∠AED
AAA criterion states that if two angles of a triangle are respectively equal to two angles of another triangle, then by the angle sum property of a triangle their third angle will also be equal.
By AAA criterion, the triangles ABC and ADE are similar.
We know that the ratio of the areas of two similar triangles is equal to the ratio of the squares of their corresponding sides.
Area of △ADE/Area of △ABC = DE²/BC²
= (6)²/(12)²
= 1/4
Let the area of △ADE = a
So, the area of △ABC = 4a
Area of quadrilateral DECB = area of △ABC - area of △ADE
So, area of quadrilateral DECB = 4a - a = 3a
Now, Area of △ADE/Area of quadrilateral DECB = a/3a
= 1/3
Therefore, the ratio of the area of △ADE to the area of quadrilateral DECB is 1:3
Q.9. ABCD is a trapezium in which AB||DC and P and Q are points on AD and BC, respectively such that PQ||DC. If PD = 18 cm, BQ = 35 cm and QC = 15 cm, find AD.
Given, ABCD is a trapezium in which AB||DC
P and Q are the points on AD and BC such that PQ||DC
We have to find the length of AD.Given, PD = 18 cm
BQ = 35 cm
QC = 15cm
Also, AB||PQ||DC
Basic Proportionality Theorem states that if a line is drawn parallel to one side of a triangle to intersect the other two sides in distinct points, the other two sides are divided in the same ratio.
In △ABD,
Given, AB||PQ
So, PO||AB
By BPT, DP/AP = OD/OB -------------- (1)
In △BDC,
Given, DC||PQ
So, OQ||DC
By BPT, OB/OD = BQ/QC
On rearranging,
OD/OB = QC/BQ ------------ (2)
Equating (1) and (2),
DP/AP = QC/BQ
So, 18/AP = 15/35
AP(15) = 35(18)
AP = 7(18)/3
AP = 42 cm
We know, AD = DP + AP
AD = 18 + 42
AD = 60 cm
Therefore, the length of AD is 60 cm.
Q.10. Corresponding sides of two similar triangles are in the ratio of 2 : 3. If the area of the smaller triangle is 48 cm², find the area of the larger triangle
Given, the ratio of corresponding sides of two similar triangles = 2:3
Area of the smaller triangle = 48 square cm.
We have to find the area of the larger triangle.
We know that the ratio of the areas of two similar triangles is equal to the ratio of the squares of their corresponding sides.
So, area of smaller triangle/area of larger triangle = (2/3)²
48/area of larger triangle = 4/9
48(9) = 4(area of larger triangle)
12(9) = area of larger triangle.
Area of larger triangle = 108 square cm.
Therefore, the area of the larger triangle is 108 square cm.
Q.11. In a triangle PQR, N is a point on PR such that QN ⊥ PR . If PN. NR = QN², prove that ∠PQR = 90°.
Given, N is a point on PR of a triangle PQR such that QN⊥PR
Also, PN . NR = QN²
We have to prove that ∠PQR = 90°
PN . NR = QN² can be written as
PN/QN = QN/NRFrom △PNQ and △RNQ,
PN/QN = QN/NR
∠PNQ = ∠RNQ = 90°
SAS Similarity Theorem states that if two sides in one triangle are proportional to two sides in another triangle and the included angle in both are congruent, then the two triangles are similar.
By SAS criterion, the triangles PNQ and RNQ are similar.
By the property of similar triangles,
∠PQN = ∠ QRN -------------- (1)
∠RQN = ∠QPN --------------- (2)
Adding (1) and (2),
∠PQN + ∠RQN = ∠QRN + ∠QPN
From the figure,
∠PQR = ∠PQN + ∠RQN ----------- (3)
So, ∠PQR = ∠QRN + ∠QPN --- (4)
We know that the sum of all the three interior angles of a triangle is always equal to 180°
In △QNR,
∠QNR + ∠NRQ + ∠RQN = 180°
90°+ ∠NRQ + ∠RQN= 180°
∠NRQ + ∠RQN = 180° - 90°
∠NRQ + ∠QPN = 90° --- [From (2)]
∠PQR = 90° [From (4)]
Therefore, it is proved that ∠PQR = 90°
Q.12. Areas of two similar triangles are 36 cm² and 100 cm² . If the length of a side of the larger triangle is 20 cm, find the length of the corresponding side of the smaller triangle.
Given, area of two similar triangles are 36cm² and 100cm²
The length of a side of the larger triangle is 20 cm
We have to find the corresponding side of the smaller triangle.
Let the corresponding side of the smaller triangle be x.
Area of the larger triangle be 100cm²
Area of the smaller triangle be 36cm²
We know that the ratio of the areas of two similar triangles is equal to the ratio of the squares of their corresponding sides.
So, area of larger triangle/area of smaller triangle = (side of larger triangle)²/(side of smaller triangle)²
100/36 = (20)²/x²
Taking square root,
10/6 = 20/x
On cross multiplication,
10x = 6(20)
10x = 120
x = 120/10
x = 12 cm
Therefore, the corresponding side of the smaller triangle is 12 cm.
Q.13. In Fig. below, if ∠ACB = ∠CDA, AC = 8 cm and AD = 3 cm, find BD.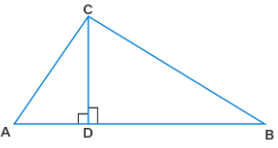
Given, ∠ACB = ∠CDA
Also, AC = 8 cm
AD = 3 cm
We have find BD.
In △ADC and △ACB,
Given, ∠ACB = ∠CDA
∠CAD = ∠BAC = common angle
AAA criterion states that if two angles of a triangle are respectively equal to two angles of another triangle, then by the angle sum property of a triangle their third angle will also be equal.
By AAA criterion, the third angle will be equal.
Therefore, the triangles ADC and ACB are similar.
By the property of similar triangles,
The corresponding sides are proportional.
So, AC/AD = AB/AC
8/3 = AB/8
On cross multiplication,
8(8) = 3AB
AB = 64/3
Now, BD = AB - AD
= 64/3 - 3
= (64 - 9)/3
= 55/3
BD = 18.33 cm
Therefore, the length of BD is 18.33 cm
Q.14. A 15 metres high tower casts a shadow 24 metres long at a certain time and at the same time, a telephone pole casts a shadow 16 metres long. Find the height of the telephone pole.
Given, a 15 m high tower casts a shadow of 24 m long.
A telephone pole casts a shadow of 16 m long.
We have to find the height of the telephone poleLet h be the height of the telephone pole.
In triangle ADC,
By Pythagorean theorem,
tan θ = opposite / adjacent
So, tan θ = CD/AC
tan θ = 15/24 ------------------- (1)
In triangle ABE,
By Pythagorean theorem,
tan θ = EB/AB
tan θ = h/16 ---------------------- (2)
Equating (1) and (2),
h/16 = 15/24
h/16 = 5/8
h = 16(5)/8
h = 2(5)
h = 10 cm
Therefore, the height of the telephone pole is 10 cm.
Q.15. Foot of a 10 m long ladder leaning against a vertical wall is 6 m away from the base of the wall. Find the height of the point on the wall where the top of the ladder reaches.
Given, the length of the ladder is 10 m
The foot of the ladder leaning against a vertical wall is 6 m away from the base of the wall.
We have to find the point on the wall where the top of the ladder reaches.In the figure,
PR is the ladder = 10 m
RQ is the distance from the base of the wall to the foot of the ladder = 6 m
PQ is the height of the point on the wall where the top of the ladder reaches.
By using Pythagorean theorem,
PR² = PQ² + RQ²
(10)² = PQ² + (6)²
100 = PQ² + 36
PQ² = 100 - 36
PQ² = 64
Taking square root,
PQ = 8 m
Therefore, the height of the point on the wall where the top of the ladder reaches is 8 m.
Exercise 6.4
Q.1. In the given Figure, if ∠A = ∠C, AB = 6 cm, BP = 15 cm, AP = 12 cm and CP = 4 cm, then find the lengths of PD and CD.
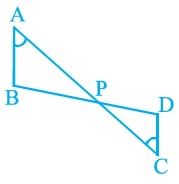
According to the question,
∠A = ∠C,
AB = 6 cm, BP = 15 cm,
AP = 12 cm
CP = 4 cm
From ∆APB and ∆CPD,
∠A = ∠C
∠APB = ∠CPD [vertically opposite angles]
∴ By AAA similarity criteria,
∆APD ∼ ∆CPD
Using basic proportionality theorem,
Considering AP/CP = PB/PD, we get,
Considering, AP/CP = AB/CD
Therefore,
Length of PD = 5 cm
Length of CD = 2 cm
Q.2. It is given that ∆ ABC ~ ∆ EDF such that AB = 5 cm, AC = 7 cm, DF= 15 cm and DE = 12 cm. Find the lengths of the remaining sides of the triangles.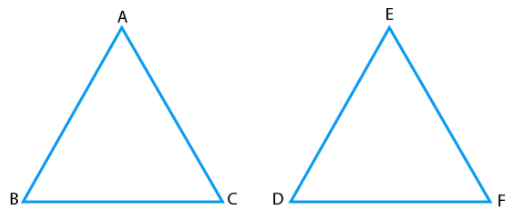
According to the question,
∆ABC ∼ ∆EDF
From property of similar triangle,
We know that, corresponding sides of ∆ABC and ∆EDF are in the same ratio.
AB/ED = AC/EF = BC/DF ….(i)
According to the question,
AB = 5cm, AC = 7cm
DF = 15cm and DE = 12cm
Substituting these values in Equation (i), we get,.
Hence, lengths of the remaining sides of the triangles are EF = 16.8 cm and BC = 6.25 cm
Q.3. Prove that if a line is drawn parallel to one side of a triangle to intersect the other two sides, then the two sides are divided in the same ratio.
Let a ∆ABC in which a line DE parallel to BC intersects AB at D and AC at E.
To prove DE divides the two sides in the same ratio.
AD/DB = AE/ECConstruction:
Join BE, CD
Draw EF ⊥ AB and DG ⊥ AC.
We know that,
Area of triangle = ½ × base × height
Then,
Since,
∆BDE and ∆DEC lie between the same parallel DE and BC and are on the same base DE.
We have,
area (∆BDE) = area(∆DEC) …..(iii)
From Equation (i), (ii) and (iii),
We get,
AD/DB = AE/EC
Hence proved.
Q.4. In the given Figure, if PQRS is a parallelogram and AB||PS, then prove that OC||SR.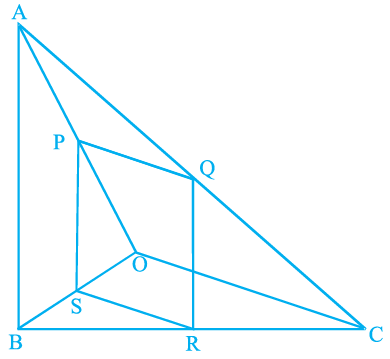
According to the question,
PQRS is a parallelogram,
Therefore, PQ || SR and PS || QR.
Also given, AB || PS.
To prove:
OC || SR
From ∆OPS and OAB,
PS||AB
∠POS = ∠AOB [common angle]
∠OSP = ∠OBA [corresponding angles]
∆OPS ∼ ∆OAB [by AAA similarity criteria]
Then,
Using basic proportionality theorem,
We get,
PS/AB = OS/OB …(i)
From ∆CQR and ∆CAB,
QR || PS || AB
∠QCR = ∠ACB [common angle]
∠CRQ = ∠CBA [corresponding angles]
∆CQR ∼ ∆CAB
Then, by basic proportionality theorem
[PS ≅ QR Since, PQRS is a parallelogram,]
From Equation (i) and (ii),
Subtracting 1 from L.H.S and R.H.S, we get,
SR || OC [By converse of basic proportionality theorem]
Hence proved.
Q.5. A 5 m long ladder is placed leaning towards a vertical wall such that it reaches the wall at a point 4 m high. If the foot of the ladder is moved 1.6 m towards the wall, then find the distance by which the top of the ladder would slide upwards on the wall.
Let the length of the ladder = AC = 5 m
Let the height of the wall on which ladder is placed = BC = 4m.From right angled ∆EBD,
Using the Pythagoras Theorem,
ED2 = EB2 + BD2
(5)2 = (EB)2 + (14)2 [ BD = 1.4]
25 = (EB)2 + 1.96
(EB)2 = 25 –1.96 = 23.04
EB = √23.04 = 4.8
Now, we have,
EC = EB – BC = 4.8 – 4 = 0.8
Hence, the top of the ladder would slide upwards on the wall by a distance of 0.8 m.
Q.6. For going to a city B from city A, there is a route via city C such that AC⊥CB, AC = 2 x km and CB = 2 (x + 7) km. It is proposed to construct a 26 km highway which directly connects the two cities A and B. Find how much distance will be saved in reaching city B from city A after the construction of the highway.
According to the question,
AC⊥CB,
AC = 2x km,
CB = 2 (x + 7) km and AB = 26 km
Thus, we get ∆ ACB right angled at C.
Now, from ∆ACB,
Using Pythagoras Theorem,
AB2 = AC2 + BC2
⇒ (26)2 = (2x)2 + {2(x + 7)}2
⇒ 676 = 4x2 + 4(x2 + 196 + 14x)
⇒ 676 = 4x2 + 4x2 + 196 + 56x
⇒ 676 = 82 + 56x + 196
⇒ 8x2 + 56x – 480 = 0Dividing the equation by 8, we get,
x2 + 7x – 60 = 0
x2 + 12x – 5x – 60 = 0
x(x + 12)-5(x + 12) = 0
(x + 12)(x – 5) = 0
∴ x = -12 or x = 5
Since the distance can’t be negative, we neglect x = -12
∴ x = 5
Now,
AC = 2x = 10km
BC = 2(x + 7) = 2(5 + 7) = 24 km
Thus, the distance covered to city B from city A via city C = AC + BC
AC + BC = 10 + 24 = 34 km
Distance covered to city B from city A after the highway was constructed = BA = 26 km
Therefore, the distance saved = 34 – 26 = 8 km.
Q.7. A flag pole 18 m high casts a shadow 9.6 m long. Find the distance of the top of the pole from the far end of the shadow.
Let MN = 18 m be the flag pole and its shadow be LM = 9.6 m.
The distance of the top of the pole, N from the far end, L of the shadow is LN.
In right angled ∆LMN,
LN2 = LM2 + MN2 [by Pythagoras theorem]
⇒ LN2 = (9.6)2 + (18)2
⇒ LN2 = 9.216 + 324
⇒ LN2 = 416.16
∴ LN = √416.16 = 20.4 m
Hence, the required distance is 20.4 m
Q.8. A street light bulb is fixed on a pole 6 m above the level of the street. If a woman of height 1.5 m casts a shadow of 3m, find how far she is away from the base of the pole.
Let A be the position of the street bulb fixed on a pole AB=6 m and CD=1.5 m be the height of a woman and her shadow be ED=3 m.
Let distance between pole and woman be x m.
Here, woman and pole both are standing vertically.So,CD∥ABIn △CDE and △ABE,∠E=∠E(common angle)
⇒ ∠ABE=∠CDE (each equal to 90∘ )
⇒ ∠BAE=∠ECD (Corresponding angles)
∴ △CDE∼△ABE by AAA similarity criterion
Then, EB/ED = AB/CD
⇒ 3/ 3+x = 1.5/6
⇒ 3 × 6=1.5(3 + x)
⇒ 18 = 4.5 + 1.5x
⇒ 1.5x = 18 − 4.5 = 13.5
⇒ x= 13.5 / 1.5
=9m
Q.9. In Fig. below, ABC is a triangle right angled at B and BD ⊥ AC. If AD = 4 cm, and CD = 5 cm, find BD and AB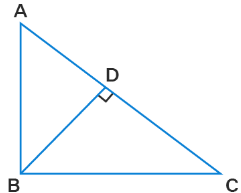
Given, ABC is a right triangle with B at right angle.
Also, BD ⊥ AC
The length of the sides
AD = 4 cm
CD = 5 cm
We have to find the length of the sides BD and AB.
In △ADB and △CDB,
∠BDA = ∠BDC = 90°
∠BAD = ∠CBD = 90° - ∠C
AAA criterion states that if two angles of a triangle are respectively equal to two angles of another triangle, then by the angle sum property of a triangle their third angle will also be equal.
By AAA, △ADB ⩬ △CDB
By the property of similarity,
The corresponding sides are proportional.
So, BD/AD = CD/BD = AB/BC
Considering BD/AD = CD/BD
BD2 = AD . CD
BD2 = 4 . 5
BD2 = 20
Taking square root,
BD = 2√5 cm
In △BDC,
By pythagoras theorem,
CB2 = BD2 + CD2
CB2 = (2√5)2 + (5)2
CB2 = 20 + 25
CB2 = 45
Taking square root,
CB = 3√5 cm
Considering BD/CD = AB/BC,
2√5/5 = AB/3√5
5AB = 2√5(3√5)
5AB = 6(5)
AB = 30/5
AB = 6 cm
Therefore, the length of sides AB and BD is 6 cm and 2√5 cm.
Q. 10. In Fig. below, PQR is a right triangle right angled at Q and QS ⊥ PR . If PQ = 6 cm and PS = 4 cm, find QS, RS and QR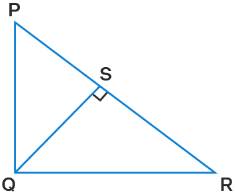
Given, PQR is a right triangle with Q at right angle.
Also, QS ⊥ PR
The length of the sides
PQ = 6 cm
PS = 4 cm
We have to find the length of QS, RS and QR.
In △PSQ,
PQ2 = PS2 + SQ2
(6)2 = (4)2 + SQ2
36 = 16 + SQ2
SQ2 = 36 - 16
SQ2 = 20
Taking square root,
SQ = 2√5 cm
We know that a perpendicular drawn from the vertex of the right angle of a right triangle to its hypotenuse divides the triangle into two triangles which are similar to the whole triangle and to each other.
So, SQ2 = PS × SR
(2√5)2 = 4 × SR
4(5) = 4SR
SR = 5 cm
In △QSR,
Given, QS⊥PR
So, ∠QSR = 90°
By pythagoras theorem,
QR2 = SQ2 + RS2
QR2 = (2√5)2 + (5)2
QR2 = 4(5) + 25
QR2 = 20 + 25
QR2 = 45
Taking square root,
QR = 3√5 cm
Therefore, the length of QS, RS and QR are 2√5 cm, 5 cm and 3√5 cm.
Q.11. In ∆ PQR, PD ⊥ QR such that D lies on QR . If PQ = a, PR = b, QD = c and DR = d, prove that (a + b) (a - b) = (c + d) (c – d)
Given, in triangle PQR, PD ⊥ QR such that D lies on QR.
Also, PQ = a
PR = b
QD = c
DR = d
We have to prove that (a + b)(a - b) = (c + d)(c - d)In △PDQ,
D = 90°
By pythagoras theorem,
PQ2 = PD2 + DQ2
a2 = PD2 + c2
PD2 = a2 - c2 ------------- (1)
In △PDR,
D = 90°
By pythagoras theorem,
PR2 = PD2 + DR2
b2 = PD2 + d2
PD2 = b2 - d2 -------------- (2)
From (1) and (2),
a2 - c2 = b2 - d2
On rearranging.
a2 - b2 = c2 - d2
By using algebraic identity,
(x2 - y2) = (x + y)(x - y)
So, (a2 - b2) = (a + b)(a - b)
c2 - d2 = (c + d)(c - d)
(a + b)(a - b) = (c + d)(c - d)
Therefore, it is proved that (a + b)(a - b) = (c + d)(c - d)
Q.12. In a quadrilateral ABCD, ∠A + ∠D = 90°. Prove that AC2 + BD2 = AD2 + BC2 [Hint: Produce AB and DC to meet at E.]
Given, ABCD is a quadrilateral
Also, ∠A + ∠D = 90°
We have to prove that AC2 + BD2 = AD2 + BC2.We know that the sum of all the three interior angles of a triangle is always equal to 180°
In △AED,
∠A + ∠D + ∠E = 180°
∠E + 90° = 180°
∠E = 180° - 90°
∠E = 90°
In △ADE,
By pythagoras theorem,
AD2 = AE2 + DE2 --------------------- (1)
In △BEC,
By pythagoras theorem,
BC2 = BE2 + CE2 --------------------- (2)
Adding (1) and (2),
AD2 + BC2 = AE2 + DE2 + BE2 + CE2
AD2 + BC2 = AE2 + CE2 + BE2 + DE2 --------------- (3)
In △AEC,
By pythagoras theorem,
AC2 = AE2 + CE2 ------------ (4)
In △BED,
By pythagoras theorem,
BD2 = BE2 + DE2 ------------- (5)
Substituting (4) and (5) in (3),
AD2 + BC2 = AC2 + BD2
Therefore, it is proved that AC2 + BD2 = AD2 + BC2
Q.13. In fig. below, l||m and line segments AB, CD and EF are concurrent at point P. Prove that AE/BF = AC/BD = CE/FD.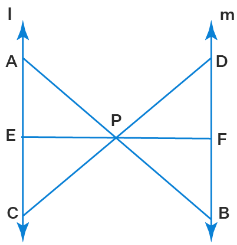
Given, l || m
The line segments AB, CD and EF are concurrent at point P.
We have to prove that AE/BF = AC/BD = CE/FD
The points A, E and C lie on l.
The points D, F and B lie on m.
In △APC and △PDB,
Vertically opposite angles are equal. i.e.,∠APC = ∠DP
Alternate angles are equal i.e., ∠PAC = ∠PBD
AAA criterion states that if two angles of a triangle are respectively equal to two angles of another triangle, then by the angle sum property of a triangle their third angle will also be equal.
By AAA criterion, △APC ⩬ △PDB
By the property of similarity,
The corresponding sides are proportional.
AP/PB = PC/PD = AC/DB -------------- (1)
In △APE and △PBF,
Vertically opposite angles are equal. i.e.,∠APE = ∠BPF
Alternate angles are equal i.e., ∠PAE = ∠PBF
AAA criterion states that if two angles of a triangle are respectively equal to two angles of another triangle, then by the angle sum property of a triangle their third angle will also be equal.
By AAA criterion, △APE ⩬ △BPF
By the property of similarity,
The corresponding sides are proportional.
AP/PB = AE/FB = EP/FP ------------------------ (2)
In △CPE and △PDF,
Vertically opposite angles are equal. i.e.,∠CPE = ∠DPF
Alternate angles are equal i.e., ∠PCE = ∠PDF
AAA criterion states that if two angles of a triangle are respectively equal to two angles of another triangle, then by the angle sum property of a triangle their third angle will also be equal.
By AAA criterion, △CPE ⩬ △PDF
By the property of similarity,
The corresponding sides are proportional.
So, EP/FP = PC/PD = CE/DF ----------------- (3)
Equating (1), (2) and (3), we get,
AP/PB = PC/PD = AC/DB = AP/PB = AE/FB = EP/FP = EP/FP = PC/PD = CE/DF
Cancelling out common terms,
AC/DB = AP/PB = AE/FB = CE/DF = EP/FP ------------------------- (4)
From (4), AE/BF = AC/BD = CE/FD
Therefore, it is proved that AE/BF = AC/BD = CE/FD
Q.14. In Fig. below, PA, QB, RC and SD are all perpendiculars to a line l, AB = 6 cm, BC = 9 cm, CD = 12 cm and SP = 36 cm. Find PQ, QR and RS.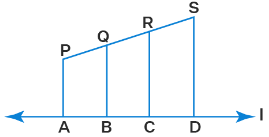
Given, PA, QB, RC and SD are all perpendicular to a line l.
Also, AB = 6 cm
BC = 9 cm
CD = 12 cm
SP = 36 cm
We have to find PQ, QR and RS.
From the figure, the lines PA, QB, RC and SD are parallel to each other.
The lines PA, QB, RC and SD are perpendicular to line l.
The Intercept theorem provides the ratios between the line segments created when two parallel lines are intercepted by two intersecting lines.
By Intercept theorem,
PQ/AB = QR/BC = RS/CD = PS/AD
AD = AB + BC + CD = 6 + 9 + 12 = 27 cm
So, PQ/6 = QR/9 = RS/12 = 36/2
Considering PQ/6 = 36/27
PQ/6 = 4/3
On cross multiplication,
3PQ = 6(4)
PQ = 24/3
PQ = 8 cm
Considering QR/9 = 36/27
QR/9 = 4/3
On cross multiplication,
3QR = 9(4)
QR = 36/3
QR = 12 cm
Considering RS/12 = 36/27
RS/12 = 4/3
On cross multiplication,
3RS = 12(4)
RS = 48/3
RS = 16 cm
Therefore, the lengths of PQ, QR and RS are 8 cm, 12 cm and 16 cm respectively.
Q.15. O is the point of intersection of the diagonals AC and BD of a trapezium ABCD with AB ║ DC. Through O, a line segment PQ is drawn parallel to AB meeting AD in P and BC in Q. Prove that PO = QO.
Given, ABCD is a trapezium with AB || DC.
O is the point of intersection of the diagonals AC and BD.
A line segment PQ is drawn parallel to AB through O.
P and Q intersect at AD and BC.
We have to prove that PO = QO.In △ADC and △POA,
∠A = ∠A = common angle
The corresponding angles are equal. i.e.,∠ACD = ∠POA
AAA criterion states that if two angles of a triangle are respectively equal to two angles of another triangle, then by the angle sum property of a triangle their third angle will also be equal.
By AAA criterion, △ADC ⩬ △POA
By the property of similarity,
The corresponding sides are proportional.
So, PO/DC = AP/AD ------------------ (1)
In △BDC and △QOB,
∠B = ∠B = common angle
The corresponding angles are equal. i.e.,∠ACD = ∠QOB
By AAA criterion, △BDC ⩬ △QOB
By the property of similarity,
The corresponding sides are proportional.
So, OQ/DC = QB/BC -------------------- (2)
Now, in △ADB,
OP||AB
Basic Proportionality Theorem states that if a line is drawn parallel to one side of a triangle to intersect the other two sides in distinct points, the other two sides are divided in the same ratio.
By basic proportionality theorem,
DP/AP = DO/OB --------------------- (3)
Now, in △CDB,
OQ||CD
By basic proportionality theorem,
CQ/QB = DO/OB --------------------- (4)
From (3) and (4),
DP/AP = CQ/QB
Adding 1 on both sides,
DP/AP + 1 = CQ/QB + 1
DP+AP / AP = CQ+QB / QB
From the figure,
DP + AP = AD
CQ + QB = BC
So, AD/AP = BC/QB
On rearranging,
AP/AD = QB/BC
From (1), PO/DC = QB/BC
From (2), PO/DC = OQ/DC
Therefore, it is clear that PO = OQ.
Q.16. In Fig. below, line segment DF intersect the side AC of a triangle ABC at the point E such that E is the mid-point of CA and ∠AEF = ∠AFE . Prove that BD/CD = BF/CE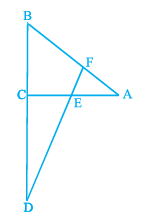
Given, line segment DF intersects the side AC of a triangle ABC at E.
E is the midpoint of CA
Also, ∠AEF = ∠AFE
We have to prove that BD/CD = BF/CE
Since E is the midpoint of CA, then
CE = AE --------------- (1)
Given, ∠AEF = ∠AFE ---------------- (2)
So, AE = AF ------------------- (3)
From (1) and (3),
CE = AE = AF --------------- (4)
Now, draw CG parallel to DF,From the figure,
The corresponding angles are equal. i.e., ∠AEF = ∠ACG ------------ (5)
Also, ∠AFE = ∠AGC ---------------- (6)
From (2), (5) and (6),
∠AFE = ∠AEF = ∠ACG = ∠AGC
We know that sides opposite to equal angles are equal.
So, AC = AG ---------------- (7)
We know, AC = CE + AE
AG = AF + GF
So, (7) becomes CE + AE = AF + GF
From (4) and (7),
AE = CE = AF = GF
CE = AF = GF ----------------- (8)
Basic Proportionality Theorem(BPT) states that if a line is drawn parallel to one side of a triangle to intersect the other two sides in distinct points, the other two sides are divided in the same ratio.
By BPT,
CB/DC = BG/GF
Adding 1 on both sides,
CB/DC + 1 = BG/GF + 1
CB+DC / DC = BG+GF / GF
From the figure,
CB + CD = BD
BG + GF = BF
So, BD/DC = BF/GF
Therefore, it is proven that BD/DC = BF/GF
Q.17. Prove that the area of the semicircle drawn on the hypotenuse of a right angled triangle is equal to the sum of the areas of the semicircles drawn on the other two sides of the triangle.
Given, a semicircle is drawn on the hypotenuse of a right angled triangle.
Also, semicircles are drawn on the other two sides of the triangle.
We have to prove that the area of the semicircle drawn on the hypotenuse of a right triangle is equal to the sum of the areas of the semicircles drawn on the other two sides of the triangle.Let ABC be a right triangle with B at right angle.
Let AB = y and BC = x
A semicircle is drawn on the hypotenuse with AC as the diameter.
Two semicircles are drawn on the other two sides with AB and BC as the diameter.
Let A₁ be the area of the semicircle drawn on the hypotenuse
A₂ and A₃ the area of the semicircles drawn on the other two sides
By pythagoras theorem,
AC2 = AB2 + BC2
AC2 = y2 + x2
Taking square root,
AC = √(x2 + y2)
We know that, area of semicircle = πr2/2
Area of semicircle drawn on side AC = π(AC/2)2 = πAC2/8
A₁ = π/8(√(x2 + y2))2
= π(x2 + y2)/8 --------------------- (1)
Area of semicircle drawn on AB = π(AB/2)2 = πAB2/8
A₂ = πy2/8
Area of semicircle drawn on BC = π(BC/2)2 = πBC2/8
A₃ = πx2/8
Sum of areas of semicircle drawn on AB and BC = A₂ + A₃
= πy2/8 + πx2/8
= π(x2 + y2)/8 ------------------ (2)
From (1) and (2),
A₁ = A₂ + A₃
Therefore, the area of a semicircle drawn on the hypotenuse is equal to the sum of the area of semicircles drawn on AB and BC.
Q.18. Prove that the area of the equilateral triangle drawn on the hypotenuse of a right angled triangle is equal to the sum of the areas of the equilateral triangles drawn on the other two sides of the triangle.
Given, an equilateral triangle is drawn on the hypotenuse of a right triangle.
Two equilateral triangles are drawn on the other two sides of the triangle.
We have to prove that the area of the equilateral triangle drawn on the hypotenuse of a right angled triangle is equal to the sum of the areas of the equilateral triangles drawn on the other two sides of the triangle.Let PQU be an equilateral triangle drawn with the hypotenuse PQ
Let PRS and QRT be the equilateral triangles drawn on the sides PR and RQ respectively.
Let PR = b an RQ = a
Let x3 be the area of the equilateral triangle drawn on the hypotenuse
x2 and x1 be the area of the equilateral triangles drawn on the other two sides
In △PRQ,
By pythagoras theorem,
PQ2 = PR2 + RQ2
PQ2 = b2 + a2
Taking square root,
PQ = √(a2 + b2
We know that, area of the equilateral triangle = √3/4(side)2
Area of △PQU = x3
= √3/4(√(a2 + b2))2
x3 =√3/4(√(a2 + b2))2
x3 =√3/4 (a2 + b2)------------------ (1)
Area of △QRT= x1
x1= √3/4(PQ)2
x1 = √3/4(a2)
Area of △PRS = x2
= √3/4(PR)2
x2 = √3/4(b2)
Sum of the area of the equilateral triangles drawn on side RQ and PR = x1 + x2
= √3/4(a2) + √3/4(b2)
= √3/4(a2 + b2) ----------------------- (2)
From (1) and (2),
x3 = x1 + x2
Therefore, the area of the equilateral triangle drawn on the hypotenuse of a right angled triangle is equal to the sum of the areas of the equilateral triangles drawn on the other two sides of the triangle.
|
127 videos|584 docs|79 tests
|
FAQs on NCERT Exemplar: Triangles - Mathematics (Maths) Class 10
| 1. What are the properties of a triangle? |  |
| 2. What are the different types of triangles based on their angles? |  |
| 3. What are the different types of triangles based on their sides? |  |
| 4. How can we find the area of a triangle? |  |
| 5. How do we determine if two triangles are similar? |  |