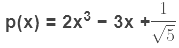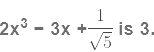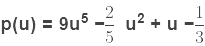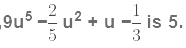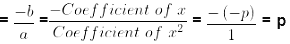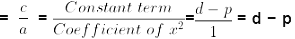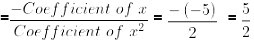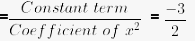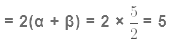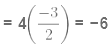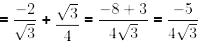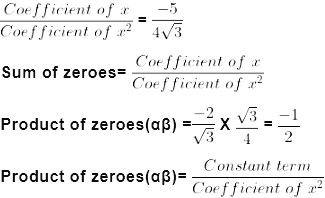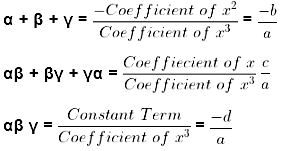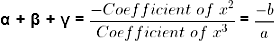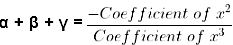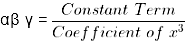Polynomials Class 10 Notes Maths Chapter 2
Algebraic Expressions
An algebraic expression is a combination of numbers, letters (representing variables), and symbols used for mathematical operations.
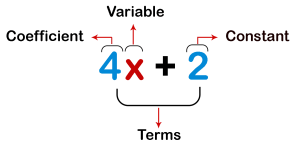 Algebraic Expressions
Algebraic Expressions
Polynomial
An expression using mathematical operations such as addition, subtraction, multiplication, and division can include fractional exponents.
On the other hand, a polynomial is a mathematical expression consisting of variables and coefficients where the exponent of each variable is a non-negative integer.
Degree of a Polynomial
For a polynomial in one variable – the highest exponent on the variable in a polynomial is the degree of the polynomial.
 Degree of a Polynomial
Degree of a Polynomial
Types of Polynomials
a) Monomial – A polynomial with just one term. Example: 2x, 6x2, 9xy
b) Binomial – A polynomial with two unlike terms. Example: 4x2+x, 5x+4
c) Trinomial – A polynomial with three unlike terms. Example: x2+3x+4
Example 1: Write the degree of the following Polynomials.
i)
Sol: As the highest power of x in p(x) is 3. The degree of polynomial,
ii)
Sol: As the highest power of u in p(u) is 5. The degree of the polynomial,
Example 2: Identify the type of the Polynomials given below (on the basis of degree)
i) 2y + 6
Sol: Here, the highest power of y in the given polynomial is 1, so it is a Linear Polynomial
ii) √2 + y2 + y
Sol: Here, the highest power of y in the given polynomial is 2, so it is a Quadratic Polynomial.
iii) y3 + 4y2 + 2y + 1
Sol: Here, the highest power of y in the given polynomial is 3, so it is a Cubic Polynomial.
Zeroes of a Polynomial
If p(x) is a polynomial in x and k is any real number, then the value obtained by replacing x by k in p(x), is called the value of p(x) at x = k, and is denoted by p(k).
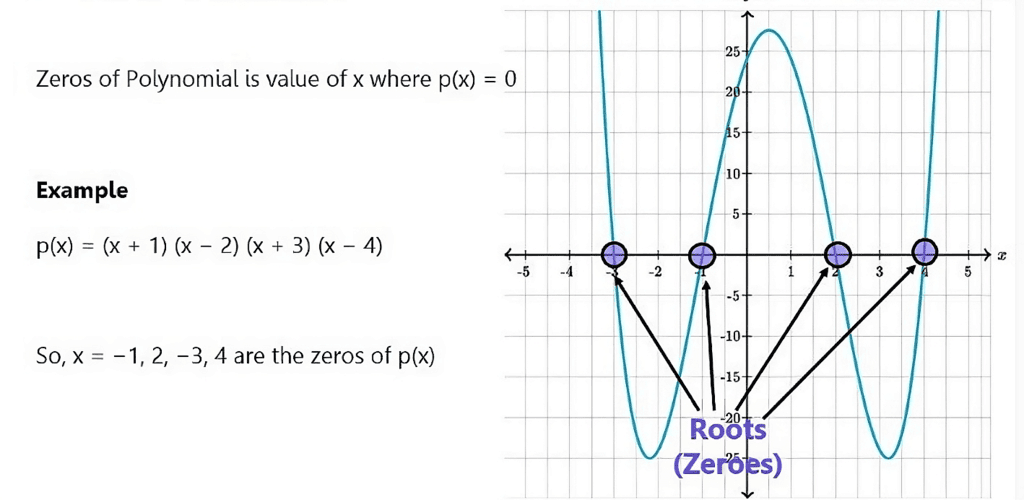 Zeroes of a Polynomial
Zeroes of a Polynomial
Let us consider the polynomial p(x) = 2x3 − 3x2 + 1
If we put x = 2 in the polynomial, that is, we replace x by 2 in the given polynomial, we get,
p(2) = 2(2)3 − 3(2)2 + 1 = 16 − 12 + 1 = 5
Therefore, the value of the polynomial p(x) at x = 2 is 5, that is, p(2) = 5
Similarly, we can find the value of p(x) at x = 0,
p(0) = 2(0)3 − 3(0)2 + 1 = 0 − 0 + 1 = 1
The value of p(x) at x = 0 is 1, that is, p(0) = 1
Now consider another case where we find the value of p(x) at x = 1
p(1) = 2(1)3 − 3(1)2 + 1 = 2 − 3 + 1 = 3 − 3 = 0
Here, we see that the value of the polynomial p(x) is 0 at x = 1
As p(1) = 0, 1 is called a zero of the polynomial
p(x) = 2x3 − 3x2 + 1
A real number k is said to be a zero of the polynomial p(x) if p(k) = 0.
Now consider a linear polynomial,p(x) = 2x + 1,
If k is a zero of p(x), then p(k) = 0
2k + 1 = 0
2k = −1
k = -1/2
If k is a zero of a polynomial, p(x) = ax + b, then,
p(k) = ak + b = 0
k = -b/a
Therefore, the zero of the polynomial, ax + b = -b/a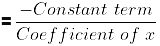
Example 3: If 3 is a zero of polynomial,p(x) = 2x2 + 3x − 9a, then find the value of a.
Sol:
If 3 is a zero of the polynomial, p(x) = 2x2 + 3x − 9a then p(3) = 0
Now, p(x) = 2x2 + 3x − 9a
p(3) = 2(3)2 + 3(3) − 9a
Now we will equate p(3) to 0.
2(3)2 + 3(3) − 9a = 0
18 + 9 − 9a = 0
27 − 9a = 0
27 = 9a
a = 3
∴ The value of a = 3.
Example 4: For what value of k, -2 is a zero of the polynomial 3x2 + 4x + 2k.
Sol:
If -2 is a zero of the polynomial, p(x) = 3x2 + 4x + 2k then p(−2) = 0
Now, p(x) = 3x2 + 4x + 2k
As -2 is a zero of p(x), we will replace x by -2 in the given polynomial and equate it to 0.
p(−2) = 3(−2)2 + 4(−2) + 2k = 0
12 − 8 + 2k = 0
2k = −4
k = −2
Example 5: If 1 and 2 are zeroes of a polynomial p(x) = 2x2 − kx + 2m, then find the value of k and m.
Sol:
Here, 1 and 2 are zeroes of the polynomial,
p(x) = 2x2 − kx + 2m
So, p(1) = 2(1)2 − k(1) + 2m = 0
2 − k + 2m = 0
k = 2m + 2 →(i)
Now p(2) = 2(22)− k(2) + 2m = 0
8 − 2k + 2m = 0
Putting the value of k in the above equation, we get
8 − 2(2m + 2) + 2m = 0
8 − 4m − 4 + 2m = 0
4 − 2m = 0
2m = 4
m = 2
We will now find the value of k by putting m = 2 in equation (i)
k = 2(2) + 2
k = 6
∴ k = 6 and m = 2
Geometrical Meaning of Zeroes of Polynomial
We know that a real number k is a zero of the polynomial p(x) if p(k) = 0.
A linear polynomial is of the form ax + b where a ≠ 0.
We will first study the graph of a linear polynomial, y = x + 3
If we put x = 2 in the above equation, we get
y = 2 + 3 = 5
Similarly, we can find more values of y by putting different values of x.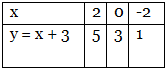
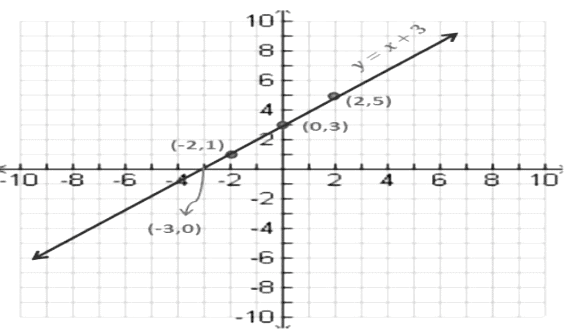 Here, we can see that the graph of a linear polynomial is a straight line.
Here, we can see that the graph of a linear polynomial is a straight line.
The graph of y = x + 3 intersects the x − axis at x = −3
Thus, −3 is the zero of the linear polynomial, y = x + 3.
Therefore, the zero of the polynomial, x + 3 is the x −coordinate of the point where the graph of y = x + 3 intersects the x-axis.
- For a linear polynomial ax + b, where a ≠ 0, the graph of y = ax + b is a straight line that intersects the x-axis at exactly one point, that is, (-b/a, 0)
- Therefore, the linear polynomial ax + b has exactly one zero, namely the x −coordinate of the point where the graph of y = ax + b intersects the x-axis.
Next, we will study the geometrical meaning of a zero of a quadratic polynomial.
Consider a quadratic polynomial, x2 − 4
First, we will find some values of y = x2 − 4 corresponding to some values of x.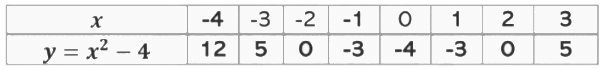 If we plot these points on a graph, this is how the graph will look like.
If we plot these points on a graph, this is how the graph will look like.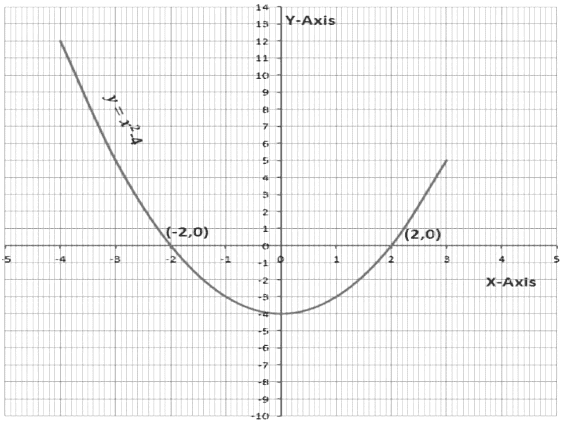
For that matter, any quadratic polynomial y = ax2 + bx + c, where a ≠ 0 the graph will have either one of the two shapes depending on the value of a
i) If a > 0, then the shape is open upwards. ii) If a < 0, then the shape is open downwards
ii) If a < 0, then the shape is open downwards These curves are called parabolas.
These curves are called parabolas.
If we see the graph, then -2 and 2 are the points on the x- axis where the graph of y = x2 − 4 intersects the x-axis.
Therefore -2 and 2 are the zeroes of the polynomial x2 − 4.
The zeroes of a quadratic polynomial ax2 + bx + c, a ≠ 0 are the x − coordinates of the point, where the parabola representing y = ax2 + bx + c intersects the x − axis.
According to the shape of the graph of y = ax2 + bx + c, the following cases may arise.
Case 1:
In this case, the graph intersects the x-axis at two distinct points A and A′, then the x- coordinates of A and A′are the two zeroes of the quadratic polynomial ax2 + bx + c.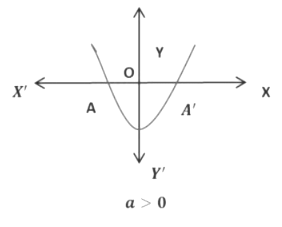
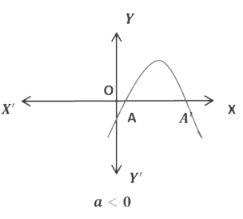
Case 2:
In this case, the graph cuts the x − axis at exactly one point. Therefore, the two points A and A′of Case 1 coincide here to become one point A.
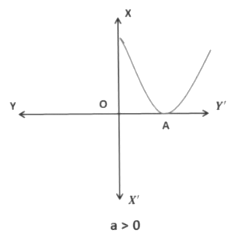
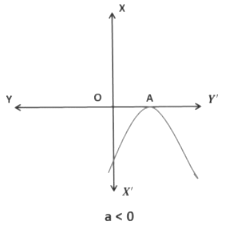 Here, the x- coordinate of A is the only zero for the quadratic polynomial, ax2 + bx + c.
Here, the x- coordinate of A is the only zero for the quadratic polynomial, ax2 + bx + c.
Case 3:
In the third case, the graph is either completely above x-axis or completely below x-axis. Therefore, the graph does not intersect the x-axis at any point. Thus, the quadratic polynomial has no zero.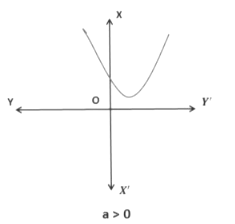
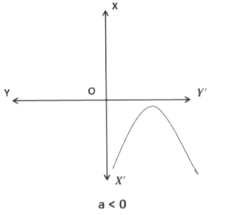
So, after studying all the cases we can see that a quadratic polynomial can have,
- Two distinct zeroes ( shown in case 1)
- Two equal zeroes or one zero ( shown in case 2)
- No zero (shown in case 3)
Therefore, geometrically we can see that a quadratic polynomial can have either two distinct zeroes or two equal zeroes that are one zero or no zero. Thus a quadratic polynomial of degree 2 has at most 2 zeroes.
Now we will study the geometrical meaning of the zeroes of a cubic polynomial. Consider a cubic polynomial x3 − 4x. First, we will find some values of y corresponding to a few values of x.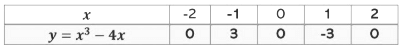 If we plot these points on the graph, the graph will look like this,
If we plot these points on the graph, the graph will look like this,
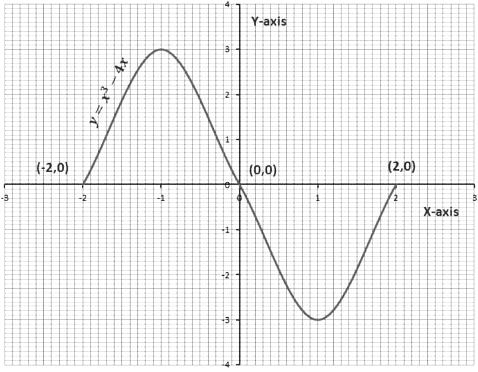 If we observe the graph, we see that -2, 0 and 2 are the x-coordinate of the points where the graph of y = x3 − 4x intersect the x-axis. Thus -2, 0 and 2 are the zeroes of the cubic polynomial, y = x3 − 4x.
If we observe the graph, we see that -2, 0 and 2 are the x-coordinate of the points where the graph of y = x3 − 4x intersect the x-axis. Thus -2, 0 and 2 are the zeroes of the cubic polynomial, y = x3 − 4x.
We will draw the graphs of a few more cubic polynomials.
Let us first consider the cubic polynomial, y = x3. We will find a few values of x and y.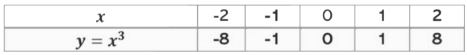
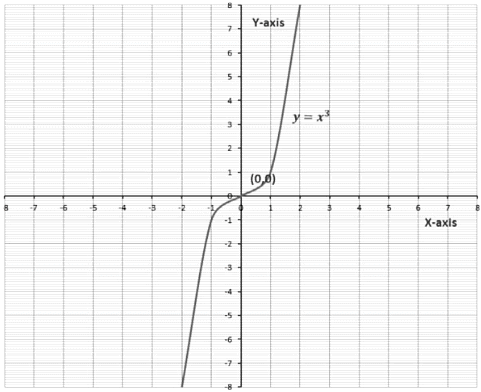 If we observe the graph we see that 0 is the only zero of the cubic polynomial, y = x3 as its graph intersects the x-axis at the origin only.
If we observe the graph we see that 0 is the only zero of the cubic polynomial, y = x3 as its graph intersects the x-axis at the origin only.
Now consider one more cubic polynomial, y = x3 − x. We will again find a few values of x and y.
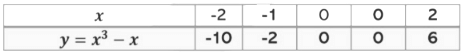
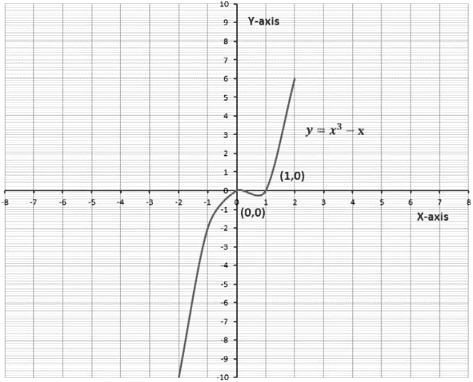 Now, we can see in the graph that 0 and 1 are the two zeroes of the cubic polynomial, y = x3 − x as its graph is intersecting the x-axis at (0, 0) and (1, 0).
Now, we can see in the graph that 0 and 1 are the two zeroes of the cubic polynomial, y = x3 − x as its graph is intersecting the x-axis at (0, 0) and (1, 0).
Therefore, any cubic polynomial can have at most 3 zeroes.
Example 6: The graph of y = p(x) is given, for some polynomials p(x). Find the number of zeroes of p(x) in each case.
Sol:
(a)
This is a graph of a quadratic polynomial. As the graph of y = p(x) does not intersect the x – axis at any point. Thus, it has no zero.
(b)This is again a graph of a quadratic polynomial. Here, the graph of y = p(x) intersects the x- axis at two points. Hence the number of zeroes is 2.
(c)
This is a graph of a linear polynomial. The number of zeroes is 1. As the graph of y = p(x) is intersecting the x-axis at one point only.
(d)The given graph is of a cubic polynomial. Here the polynomial y = p(x) is intersecting the x – axis at 3 points. Therefore, the number of zeroes is 3.
Example 7: Which of the following is not the graph of a cubic polynomial?
Sol:
Option d) is not the graph of a cubic polynomial. It is a graph of a quadratic polynomial, as the graph is in the shape of a parabola which is opening downwards.
Relationship between Zeroes and Coefficients of a Polynomial
We know that a quadratic polynomial is of the form ax2 + bx + c. A quadratic polynomial can have at the most two zeroes.
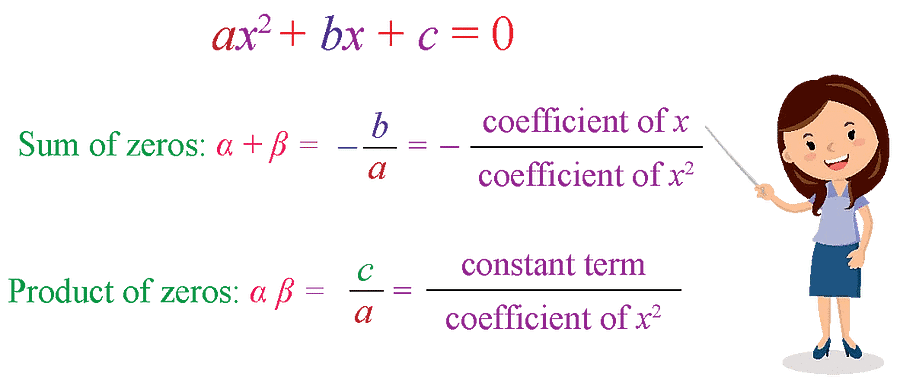 Sum and Product of ZeroesIn general, if α and β are the zeroes of the quadratic polynomial p(x) = ax2 + bx + c, a ≠ 0, then x − α and x − β are the actors of the p(x). Therefore,
Sum and Product of ZeroesIn general, if α and β are the zeroes of the quadratic polynomial p(x) = ax2 + bx + c, a ≠ 0, then x − α and x − β are the actors of the p(x). Therefore,
ax2 + bx + c = k (x - a) (x - β)
= k(x2 - xβ - ax + aβ)
= [x2 - (β + a)x + aβ)
= kx2 - k (β + a)x + kaβ
Now, comparing the coefficients of x2, x and constant terms on both sides, we get
a = k, b = -k(β + a), c = kaβ
(a + β) = -b/k
Now k = a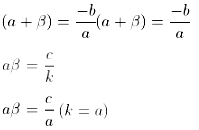
Sum of zeroes(α + β)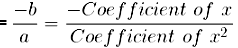
Product of zeroes(αβ)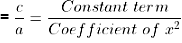
Example 8: If one zero of 2x2 − 3x + k is reciprocal to the other, then find the value of k.
Sol:
Let one zero be α, then the other zero will be 1/a
We know that, Product of zeroes(αβ) = c/a = Constant term / Coefficient of x2
= c/a = k/2
K= 2
Example 9: If α and β are zeroes of the polynomial x2 − p(x + 1) + d such that (α + 1)(β + 1) = 0, then find the value of d.
Sol:
The given polynomial is x2 − p(x + 1) + d
x2 − px − p + d
Comparing the above equation with ax2 + bx + c, we get
a = 1, b = −p, c = −p + d
α and β are the zeroes of the polynomial x2 − px − p + d
Sum of zeroes(α + β)
Product of zeroes(αβ)
(α + 1)(β + 1) = 0 (given)
αβ + α + β + 1 = 0
Putting (α + β) = p and (αβ) = d − p in the above equation we get,
d − p + p + 1 = 0
d + 1 = 0
d = −1
Example 10: Form a quadratic polynomial, whose one zero is 7 and the product of zeroes is -56.
Sol:
Let the zeroes be α and β.
It is given that the value of one zero is 7, then let us assume that α = 7
Product of zeroes = αβ = 7β
Now, 7β = −56
β = −8
∴ α = 7 and β = −8
(α + β) = 7 − 8 = −1
(αβ) = 7 × (−8) = −56
We know that a quadratic polynomial isx2 −(sum of zeroes)x + product of zeroes
x2 − (α + β)x + (αβ)
Putting (α + β) = −1 and (αβ) = −56
x2 + x − 56
Example 11: If the zeroes of the polynomial x2 + px + q are double in value of the zeroes of 2x2 − 5x − 3, then find the value of p and q.
Sol:
Let α and β be the zeroes of 2x2 − 5x − 3.
Sum of zeroes(α + β) =−ba
Product of zeroes(αβ) = c/a =
As the zeroes of x2 + px + q are double in value. Therefore, 2α and 2β are the zeroes of x2 + px + q
Sum of zeroes(2α + 2β) = 2(α + β)
Product of zeroes(2α × 2β) = 4αβ
We know that a quadratic polynomial is x2 −(sum of zeroes)x + product of zeroes
∴ x2 − (2α + 2β)x + (2α × 2β)
x2 − 5x − 6
Comparing the above equation with x2 + px + q, we get
p = −5, q = −6
Example 12: Find the zeroes of the quadratic polynomial, 4√3x2 + 5x − 2√3 and verify the relationship between the zeroes and the coefficients.
Sol:
Letp(x) = 4√3x2 + 5x − 2√3
By splitting the middle term we get,
p(x) = 4√3x2 + 5x − 2√3
p(x) = 4√3x2 + (8 − 3)x − 2√3
p(x) = 4√3x2 + 8x − 3x − 2√3
p(x) = 4x(√3x + 2) − √3(√3x + 2)
p(x) = (√3x + 2)(4x − √3)
The value of 4√3x2 + 5x − 2√3 is zero, when x =-2/√3 and x = √3/4
Therefore, the zeroes of 4√3x2 + 5x − 2√3 are x = -2/√3 and x = √3/4
Sum of zeroes(α + β)
Let α, β, γ be the zeroes of a cubic polynomial
ax3 + bx2 + cx + d
x3 −(sum of zeroes)x2 + (sum of the product of zeroes taking two at a time)x – product of zeroes
x3 +( α + β + γ)x2 + (αβ + βγ + γα)x – αβ γ
Example 13: If two zeroes of the polynomial f(x) = x3 − 4x2 − 3x + 12 are √3 and −√3, then find its third zero.
Sol:
Let α and β be the two roots of the polynomial,
x3 − 4x2 − 3x + 12
Then α = √3 and β = - √3,
√3 - √3 + y = -(4)/1 = 4
γ = 4
Therefore the third zero is 4.
Example 14: If zeroes of the polynomial f(x) = x3 − 3x2 + x + 1 are a − b, a and a + b, then find a and b.
Sol:
Now, a − b, a, and a + b are zeroes of the cubic polynomial
Let α = a − b, β = a and γ = a + b
We know that,
a − b + a + a + b = -(3)/1 = 3
3a = 3 , a = 1
(a − b)a(a + b) = -(1)/1 = 1
a(a2 − b2) = −1
Putting a = 1 in the above equation
1(1 − b2) = −1
(1 − b2) = −1
b2 = 2
b = ±√2
|
127 videos|584 docs|79 tests
|
FAQs on Polynomials Class 10 Notes Maths Chapter 2
| 1. What is a polynomial and how is it defined ? |  |
| 2. How do you determine the degree of a polynomial ? |  |
| 3. What are the different types of polynomials based on their degrees ? |  |
| 4. What are the zeroes of a polynomial and how are they found ? |  |
| 5. What is the geometrical meaning of the zeroes of a polynomial ? |  |