Ex-5.2 Trigonometric Ratios (Part - 2), Class 10, Maths RD Sharma Solutions | Extra Documents, Videos & Tests for Class 10 PDF Download
Q26) If θ = 30°,verify(i)Tan2θ = 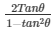
Solution:
Tan2θ = 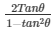 …..(i)
…..(i)
Substitute θ = 30° in equation (i)
LHS = Tan 60° = √3
RHS = 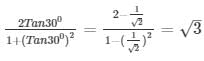
Therefore, LHS = RHS
(ii) sinθ =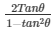
Substitute θ = 30°
sin60° = 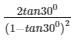
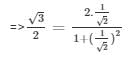
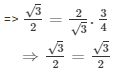
Therefore, LHS = RHS.
(iii) cos2θ =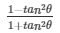
Substitute θ = 30°
LHS = cosecθ RHS = 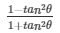
= cos 2(30°) =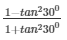
Cos 60° = 1/2 = 
Therefore, LHS = RHS
(iv) cos3θ = 4cos3θ − 3cosθ
Solution:
LHS = cos3θ
Substitute θ = 30°
= cos 3 (30°) = cos 900
= 0
RHS = 4cos3θ − 3cosθ
= 4cos330° − 3cos30°
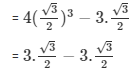
= 0
Therefore, LHS = RHS.
Q27) If A = B = 60°. Verify (i) Cos (A – B) = Cos A Cos B + Sin A Sin B
Solution:
Cos (A – B) = Cos A Cos B + Sin A Sin B…….(i)
Substitute A and B in (i)
⇒ cos (60° – 60°) = cos 60° cos 60° + sin 60° sin 60°
⇒ cos 00 = (1/2)2 + (√3/2)2
⇒ 1 = 1/4 + 3/4
⇒ 1 = 1
Therefore, LHS = RHS
(ii) Substitute A and B in (i)
⇒ sin (60° – 60°) = sin 60° cos 60° – cos 60° sin 60°
⇒ sin 00 = 0
⇒ 0 = 0
Therefore, LHS = RHS
(iii) Tan(A − B) = 
A = 60°, B = 60° we get,
Tan(60° − 60°) = 
Tan 00 = 0
0 = 0
Therefore, LHS = RHS
Q28 ) If A = 30°, B = 60° verify:
(i) Sin (A + B) = Sin A Cos B + Cos A Sin B
Solution:
A = 30°, B = 60° we get
Sin (30° + 60°) = Sin 30° Cos 60° + Cos 30° Sin 60°
Sin (900) = 1/2.1/2 + √3/2.√3/2
Sin (900) = 1 ⇒ 1 = 1
Therefore, LHS = RHS
(ii) Cos (A + B) = Cos A Cos B – Sin A Sin B
A = 30°, B = 60° we get
Cos (30° + 60°) = Cos 30° Cos 60° – Sin 30° Sin 60°
Cos (900) = 1/2.3√2 − √3/2.1/2
0 = 0
Therefore, LHS = RHS
Q29. If sin(A + B) = 1 and cos(A-B) = 1, 0°<A + B≤90°, A≥B find A and B.
Sol:
Given,
sin(A + B) = 1 this can be written as sin(A + B) = sin(90°)
cos(A-B) = 1 this can be written as cos(A-B) = cos(0°)
⇒ A + B = 90°
A – B = 0°
2A = 90°
A = 90°/2
A = 45°
Substitute A value in A – B = 0°
45° – B = 0°
B = 45°
Hence, the value of A = 45° and B = 45°
Q30. If tan(A-B) = 1/√3 and tan(A + B) = √3, 0°<A + B≤90°, A>B find A and B
Solution:
Given,
tan(A-B) = 1/√3
A – B = tan − 1(1/√3)
A – B = 30° ——- 1
tan(A + B) = √3
A + B = tan − 1√3
A + B = 60° ——- 2
Solve equations 1 and 2
A + B = 30°
A – B = 60°
2A = 90°
A = 90°/2
A = 45°
Substitute the value of A in equation 1
45° + B = 30°
B = 30° – 45°
B = 15°
The value of A = 45° and B = 15°
Q31. If sin(A-B) = 1/2 and cos(A + B) = 1/2, 0°<A + B≤90°, A<B find A and B.
Solution:
Given,
sin(A-B) = 1/2
A – B = sin − 1(1/2)
A – B = 30° ——- 1
cos(A + B) = 1/2
A + B = cos − 1(1/2)
A + B = 60° ——- 2
Solve equations 1 and 2
A + B = 60°
A – B = 30°
2A = 90°
A = 90°/2
A = 45°
Substitute the value of A in equation 2
45° + B = 60°
B = 60° – 45°
B = 15°
The value of A = 45° and B = 15°
Q32. In a Δ ABC right angled triangle at B, ∠A = ∠C.Find the values of:
1. sinAcosC + cosAsinC
Solution:
since, it is given as ∠A = ∠C
the value of A and C is 45°, the value of angle B is 90°
because the sum of angles of triangle is 180°
⇒ sin(45°)cos(45°) + cos(45°)sin(45°)
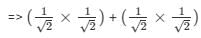
⇒ 1/2 + 1/2
⇒ 1
The value of sinAcosC + cosAsinC is 1
2. sinAsinB + cosAcosB
Solution:
since, it is given as ∠A = ∠C
the value of A and C is 45°, the value of angle B is 90°
because the sum of angles of triangle is 180°
⇒ sin(45°)sin(90°) + cos(45°)sin(90°)
⇒ 1/√2(1) + 1/√2(0)
⇒ 1/√2 + 0
⇒ 1/√2
The value of sinAsinB + cosAcosB is 1/√2
Q33. Find the acute angle A and B, if sin(A + 2B) = √3/2 and cos(A + 4B) = 0, A>B.
Solution:
Given,
sin(A + 2B) = √3/2
A + 2B = sin − 1√3/2
A + 2B = 60° —— – 1
Cos(A + 4B) = 0
A + 4B = sin − 1(90)
A + 4B = 90° —— – 2
Solve equations 1 and 2
A + 2B = 60°
A + 4B = 90°
(-) (-) (-)
-2B = – 30°
2B = 30°
B = 30°/2
B = 15°
Substitute B value in eq 2
A + 4B = 90°
A + 4(15°) = 90°
A + 60° = 90°
A = 90° – 60°
A = 30°
The value of A = 30° and B = 15°
Q 34. In ΔPQR, right angled at Q, PQ = 3 cm and PR = 6 cm. Determine ∠ P and ∠ R.
Solution:
Given,
In ΔPQR, right angled at Q, PQ = 3 cm and PR = 6 cm
By Pythagoras theorem,
PR2 = PQ2 + QR2
⇒ 62 = 32 + QR2
⇒ QR2 = 36 – 9
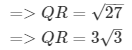
sin R = 3/6 = 1/2 = sin30°
∠R = 30°
As we know, Sum of angles in a triangle = 180
∠P + ∠Q + ∠R = 180°
⇒ ∠P + 90° + 30° = 180°
⇒ ∠P = 180° – 120°
⇒ ∠P = 60°
Therefore, ∠R = 30°
And, ∠P = 60°
Q35. If sin(A – B) = sin A cos B – cos A sin B and cos (A – B) = cos A cos B + sin A sin B, find the values of sin 15 and cos 15.
Solution:
Given,
sin(A – B) = sin A cos B – cos A sin B
And, cos (A – B) = cos A cos B + sin A sin B
We need to find, sin 15 and cos 15.
Let A = 45 and B = 30
sin 15 = sin (45- 30) = sin 45 cos 30 – cos 45 sin 30
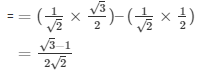
cos 15 = cos (45- 30) = cos 45 cos 30 – sin 45 sin 30
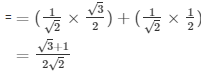
Q36. In a right triangle ABC, right angled at C, if ∠B = 60° and AB = 15 units. Find the remaining angles and sides.
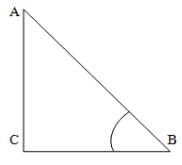
Solution:
sin60° = x/15
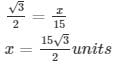
cos60° = x/15
1/2 = x/15
x = 15/2
x = 7.5units
Q37. In ΔABC is a right triangle such that ∠C = 90°, ∠A = 45° and BC = 7 units. Find the remaining angles and sides.
Solution:
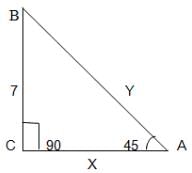
Here, ∠C = 90° and ∠A = 45°
We know that,
∠A + ∠B + ∠C = 180°
⇒ 45° + 90° + ∠C = 180°
⇒ 135° + ∠C = 180°
⇒ ∠C = 180° – 135°
⇒ ∠C = 45°
The value of the remaining angle C is 45°
Now, we need to find the sides x and y
here,
cos(45) = BC/AB
1/√2 = 7/y
y = 7√2 units
sin(45) = AC/AB

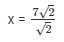
x = 7 units
the value of x = 7 units and y = 7√2 units
Q 38 . In a rectangle ABCD , AB = 20 cm , ∠BAC = 60° , calculate side BC and diagonals AC and BD .
Solution:
Let AC = x cm and CB = y cm
Since , cosθ = base/hypotenuse
Therefore , cos60° = 20/x
⇒1/2 = 20/x [since,cos60° = 1/2]
⇒ x = 40 cm = AC
Similarly BD = 40 cm
Now ,
Since , sinθ = perpendicular/hypotenuse
Therefore , sin60° = BC/AC
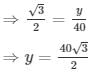
⇒y = 20√3 cm .
Q39:If A & B are acute angles such that tanA = 1/2 tanB = 1/3 and tan(A + B) = 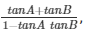 find A + B.
find A + B.
Solution:
Tan(A + B) = 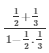
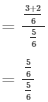
Tan(A + B) = 5/6 × 6/5
(A + B) = Tan − 1(1)
(A + B) = 45°
Q 40: Prove that : (√3 − 1)(3 − cot30°) = tan360 − 2sin60°
Ans:
L.H.S ⇒ (√3 + 1)(3 − cot30°)
= (√3 + 1)(3 − √3) ∵ cot30° = √3
= (√3 + 1)(√3 − 1)√3
= ((√3)2 − (1)2)√3
= 2√3
R.H.S ⇒ tan36° − 2sin60°
= (√3)3 − 2×√3/2
= 3√3 − √3
= 2√3
L.H.S = R.H.S
Hence proved.
|
5 videos|292 docs|59 tests
|
|
5 videos|292 docs|59 tests
|

|
Explore Courses for Class 10 exam
|

|
















