Ex-8.3 Quadratic Equations (Part - 2), Class 10, Maths RD Sharma Solutions | Extra Documents, Videos & Tests for Class 10 PDF Download
Question 19: Find the roots of the equation 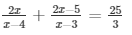
Sol:
The given equation is 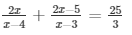
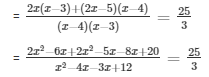

= 3(4x2 - 19x + 20) = 25(x2 - 7x + 12)
= 12x2 - 57x + 60 = 25x2 – 175x + 300
= 13x2 - 78x - 40x + 240 = 0
= 13x2 - 118x + 240 = 0
= 13x2 - 78x - 40x + 240 = 0
= 13x(x - 6) - 40(x - 6) = 0
= (x - 6)(13x - 40) = 0
Either x - 6 = 0 therefore x = 6
Or , 13x - 40 = 0 therefore x = 40/13
The roots of the above mentioned quadratic equation are 6 and 40/13 respectively.
Question 20: Find the roots of the quadratic equation 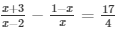
Sol:
The given equation is 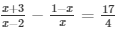
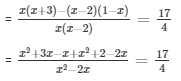
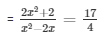
= 4(2x2 + 2) = 17(x2 - 2x)
= 8x2 + 8 = 17x2 - 34x
= 9x2 - 34x - 8 = 0
= 9x2 - 36x + 2x - 8 = 0
= 9x(x - 4) + 2(x - 4) = 0
= (9x + 2)(x - 4) = 0
Either 9x + 2 = 0 therefore x = −2/9
Or, x - 4 = 0 therefore x = 4
The roots of the above mentioned quadratic equation are x = −2/9 and 4 respectively.
Question 21: Find the roots of the quadratic equation 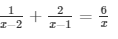
Sol:
The equation is 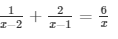
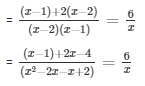

= x(3x - 5) = 6(x2 - 3x + 2)
= 3x2 - 5x = 6x2 - 18x + 12
= 3x2 - 13x + 12 = 0
= 3x2 - 9x - 4x + 12 = 0
= 3x(x - 3) - 4(x - 3) = 0
= (x - 3)(3x - 4) = 0
Either x - 3 = 0 therefore x = 3
Or, 3x - 4 = 0 therefore 4/3
The roots of the above mentioned quadratic equation are 3 and 4/3 respectively.
Question 22: Find the roots of the quadratic equation 
Sol:
The equation is 
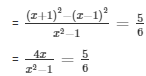
= 6(4x) = 5(x2 - 1)
= 24x = 5x2 - 5
= 5x2 - 24x - 5 = 0
= 5x2 - 25x + x - 5 = 0
= 5x(x - 5) + 1(x - 5) = 0
= (5x + 1)(x - 5) = 0
Either x - 5 = 0
Therefore x = 5
Or, 5x + 1 = 0
Therefore x = −1/5
The roots of the above mentioned quadratic equation are x = −1/5 and 5 respectively.
Question 23: Find the roots of the quadratic equation 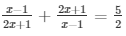
Sol:
The equation is 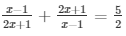
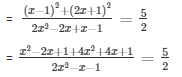
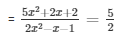
= 2(5x2 + 2x + 2) = 5(2x2 - x - 1)
= 10x2 + 4x + 4 = 10x2 - 5x - 5
Cancelling out the equal terms on both sides of the equation. We get,
= 4x + 5x + 4 + 5 = 0
= 9x + 9 = 0
= 9x = - 9
X = - 1
X = - 1 is the only root of the given equation.
Question 24: Find the roots of the quadratic equation  = 1−2x
= 1−2x
The given equation is  = 1−2x
= 1−2x
=  = 1−2x
= 1−2x
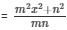 = 1−2x
= 1−2x
= m2x2 + 2mnx + (n2 - mn) = 0
Now we solve the above quadratic equation using factorization method
Therefore
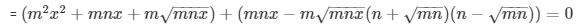
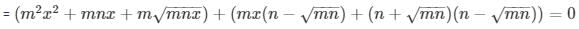
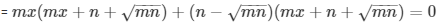
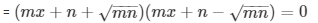
Now, one of the products must be equal to zero for the whole product to be zero for the whole product to be zero. Hence, we equate both the products to zero in order to find the value of x .
Therefore,
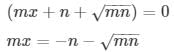
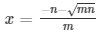
Or
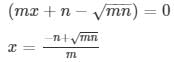
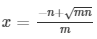
The roots of the above mentioned quadratic equation are  respectively.
respectively.
Question 25: Find the roots of the quadratic equation 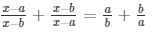
Sol:
The given equation is 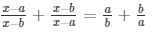
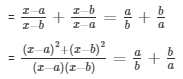
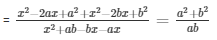
= (2x2 - 2x(a + b) + a2 + b2)ab = (a2 + b2)(x2 - (a + b)x + ab)
= (2abx2 - 2abx(a + b) + ab(a2 + b2)) = (a2 + b2)(x2 - (a2 + b2)(a + b)x + (a2 + b2)(ab)
= (a2 + b2 - 2ab)x - (a + b)(a2 + b2 - 2ab)x = 0
= (a - b)2x2 - (a + b)(a + b)2x2 = 0
= x(a - b)2(x - (a + b)) = 0
= x(x - (a + b)) = 0
Either x = 0
Or, (x - (a + b)) = 0
Therefore x = a + b
The roots of the above mentioned quadratic equation are 0 and a + b respectively.
Question 26: Find the roots of the quadratic equation 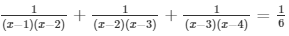
Sol:
The given equation is 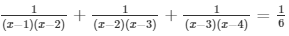
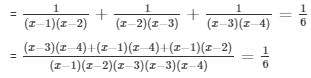

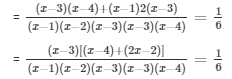
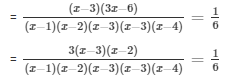
Cancelling out the like terms on both the sides of numerator and denominator. We get,
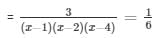
= (x - 1)(x - 4) = 18
= x2 - 4x - x + 4 = 18
= x2 - 5x - 14 = 0
= x2 - 7x + 2x - 14 = 0
= x(x - 7) + 2(x - 7) = 0
= (x - 7)(x + 2) = 0
Either x - 7 = 0
Therefore x = 7
Or, x + 2 = 0
Therefore x = - 2
The roots of the above mentioned quadratic equation are 7 and - 2 respectively.
Question 27: Find the roots of the quadratic equation 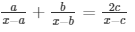
Sol:
The given equation is 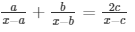
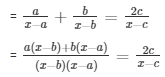
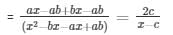
= (x - c)(ax - 2ab + bx) = 2c(x2 - bx - ax + ab)
= (a + b)x2 - 2abx - (a + b)cx + 2abc = 2cx2 - 2c(a + b)x + 2abc
Question 28: Find the roots of the Question x2 + 2ab = (2a + b)x
Sol:
The given equation is x2 + 2ab = (2a + b)x
= x2 + 2ab = (2a + b)x
= x2 - (2a + b)x + 2ab = 0
= x2 - 2ax - bx + 2ab = 0
= x(x - 2a) - b(x - 2a) = 0
= (x - 2a)(x - b) = 0
Either x - 2a = 0
Therefore x = 2a
Or, x - b = 0
Therefore x = b
The roots of the above mentioned quadratic equation are 2a and b respectively.
Question 29: Find the roots of the quadratic equation (a + b)2x2 - 4abx - (a - b)2 = 0
Sol:
The given equation is (a + b)2x2 - 4abx - (a - b)2 = 0
= (a + b)2x2 - 4abx - (a - b)2 = 0
= (a + b)2x2 - ((a + b)2 –(a - b)2 )x - (a - b)2 = 0
= (a + b)2x2 - (a + b)2 x + (a - b)2 x - (a - b)2 = 0
= (a + b)2x(x - 1) (a + b)2 (x - 1) = 0
= (x - 1) (a + b)2x + (a + b)2) = 0
Either x - 1 = 0
Therefore x = 1
Or, (a + b)2x + (a + b)2) = 0
Therefore 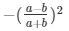
The roots of the above mentioned quadratic equation are 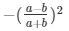 and 1 respectively .
and 1 respectively .
Question 30: Find the roots of the quadratic equation a(x2 + 1) - x(a2 + 1) = 0
Sol:
The given equation is a(x2 + 1) - x(a2 + 1) = 0
= a(x2 + 1) - x(a2 + 1) = 0
= ax2 + a - a2x - x = 0
= ax(x - a) - 1(x - a) = 0
= (x - a)(ax - 1) = 0
Either x - a = 0
Therefore x = a
Or, ax - 1 = 0
Therefore x = 1/a
The roots of the above mentioned quadratic equation are ( a) and x = 1/a respectively.
Question 31: Find the roots of the quadratic equation x2 + (a + 1/a)x + 1 = 0.
Sol:
The given equation is x2 + (a + 1/a)x + 1 = 0
= x2 + (a + 1/a)x + 1 = 0
= x2 + ax + x/a + (a×1/a) = 0
= x(x + a) + 1/a(x + a) = 0
= (x + a)(x + 1/a) = 0
Either x + a = 0
Therefore x = - a
Or , (x + 1/a) = 0
Therefore x = 1/a
The roots of the above mentioned quadratic equation are x = 1/a and –a respectively.
Question 32: Find the roots of the quadratic equation abx2 + (b2 - ac)x - bc = 0
Sol:
The given equation is abx2 + (b2 - ac)x - bc = 0
= abx2 + (b2 - ac)x - bc = 0
= abx2 + b2x - acx - bc = 0
= bx (ax + b) - c (ax + b) = 0
= (ax + b)(bx - c) = 0
Either, ax + b = 0
Therefore x = −b/a
Or, bx - c = 0
Therefore x = c/b
The roots of the above mentioned quadratic equation are x = c/b and x = −b/a respectively.
Question 33: Find the roots of the quadratic equation a2b2x2 + b2x - a2x - 1 = 0
Sol:
The given equation is a2b2x2 + b2x - a2x - 1 = 0
= a2b2x2 + b2x - a2x - 1 = 0
= b2x(a2x + 1) - 1(a2x + 1)
= (a2x + 1)( b2x - 1) = 0
Either (a2x + 1) = 0
Therefore x = 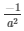
Or, ( b2x - 1) = 0
Therefore x = 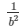
The roots of the above mentioned quadratic equation are x = 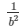 and x =
and x = 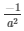 respectively.
respectively.
|
5 videos|292 docs|59 tests
|
FAQs on Ex-8.3 Quadratic Equations (Part - 2), Class 10, Maths RD Sharma Solutions - Extra Documents, Videos & Tests for Class 10
| 1. What are the steps to solve a quadratic equation? |  |
| 2. How can I determine the nature of the roots of a quadratic equation? |  |
| 3. Can a quadratic equation have more than two roots? |  |
| 4. Are there any alternative methods to solve quadratic equations? |  |
| 5. Can a quadratic equation have irrational roots? |  |
|
5 videos|292 docs|59 tests
|

|
Explore Courses for Class 10 exam
|

|
















