Quadrilaterals Class 9 Notes Maths Chapter 8
| Table of contents |

|
| What is Quadrilateral? |

|
| Angle Sum Property of a Quadrilateral |

|
| Types of Quadrilaterals |

|
| Properties of a Parallelogram |

|
| The Mid-point Theorem |

|
What is Quadrilateral?
Any closed polygon with four sides, four angles and four vertices are called Quadrilateral. It could be regular or irregular.
Angle Sum Property of a Quadrilateral
- The sum of the four angles of a quadrilateral is 360°
- If we draw a diagonal in the quadrilateral, it divides it into two triangles.
- And we know the angle sum property of a triangle i.e. the sum of all the three angles of a triangle is 180°.
- The sum of angles of ∆ADC = 180°.
- The sum of angles of ∆ABC = 180°.
- By adding both we get ∠A + ∠B + ∠C + ∠D = 360°
- Hence, the sum of the four angles of a quadrilateral is 360°.
Example: Find ∠A and ∠D, if BC∥ AD and ∠B = 52° and ∠C = 60° in the quadrilateral ABCD.
Solution: Given BC ∥ AD, so ∠A and ∠B are consecutive interior angles.
So ∠A + ∠B = 180° (Sum of consecutive interior angles is 180°).
∠B = 52°
∠A = 180°- 52° = 128°
∠A + ∠B + ∠C + ∠D = 360° (Sum of the four angles of a quadrilateral is 360°).
∠C = 60°
128° + 52° + 60° + ∠D = 360°
∠D = 120°
∴ ∠A = 128° and ∠D = 120 °.
Types of Quadrilaterals
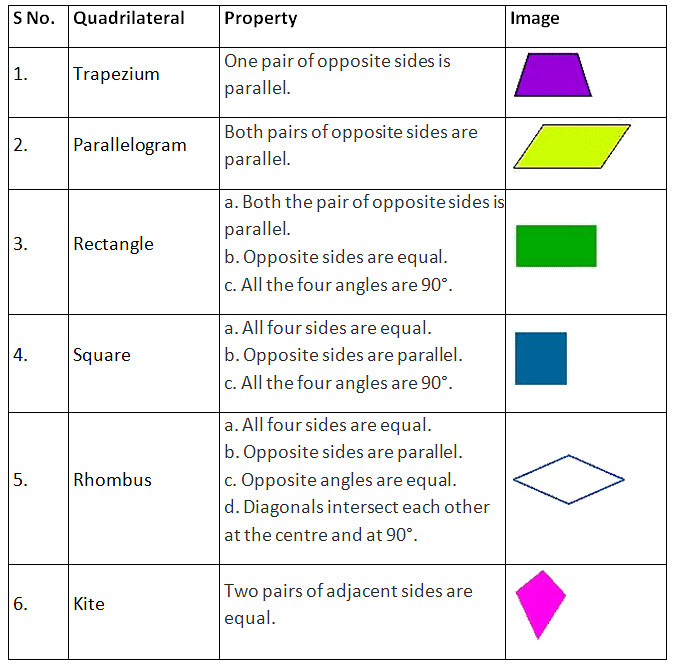
Remark: A square, Rectangle and Rhombus are also a parallelogram.
Properties of a Parallelogram
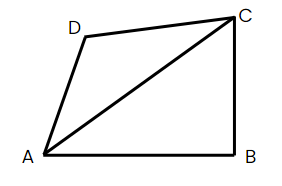
Theorem 1: When we divide a parallelogram into two parts diagonally then it divides it into two congruent triangles.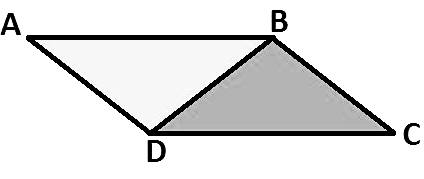
∆ABD ≅ ∆CDB
Theorem 2: In a parallelogram, opposite sides will always be equal.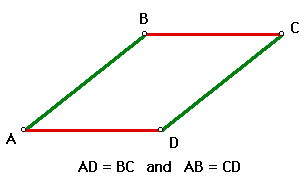
Theorem 3: A quadrilateral will be a parallelogram if each pair of its opposite sides will be equal.
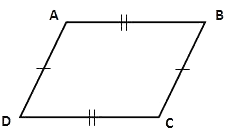
Here, AD = BC and AB = DC
Then ABCD is a parallelogram.
Theorem 4: In a parallelogram, opposite angles are equal.
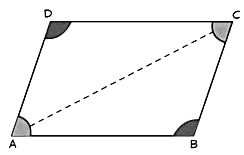
In ABCD, ∠A = ∠C and ∠B = ∠D
Theorem 5: In a quadrilateral, if each pair of opposite angles is equal, then it is said to be a parallelogram. This is the reverse of Theorem 4.
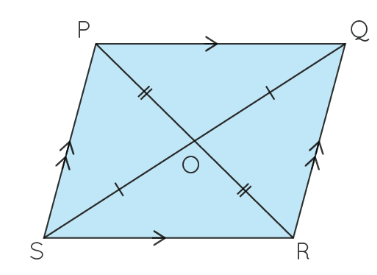
Theorem 6: The diagonals of a parallelogram bisect each other.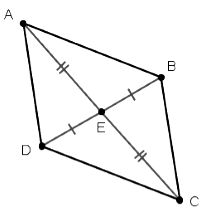
Here, AC and BD are the diagonals of the parallelogram ABCD.
So the bisect each other at the centre.
DE = EB and AE = EC
Theorem 7: When the diagonals of the given quadrilateral bisect each other, then it is a parallelogram.
This is the reverse of the theorem 6.
The Mid-point Theorem
1. If a line segment joins the midpoints of the two sides of the triangle then it will be parallel to the third side of the triangle.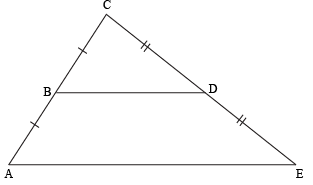
If AB = BC and CD = DE then BD ∥ AE.
2. If a line starts from the midpoint of one line and that line is parallel to the third line then it will intersect the midpoint of the third line.
If D is the midpoint of AB and DE∥ BC then E is the midpoint of AC.
Example: Prove that C is the midpoint of BF if ABFE is a trapezium and AB ∥ EF.D is the midpoint of AE and EF∥ DC.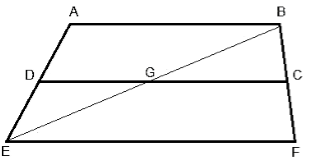
Solution: Let BE cut DC at a point G.
Now in ∆AEB, D is the midpoint of AE and DG ∥ AB.
By midpoint theorem, G is the midpoint of EB.
Again in ∆BEF, G is the midpoint of BE and GC∥ EF.
So, by midpoint theorem C is the midpoint of BF.
Hence proved.
|
40 videos|471 docs|57 tests
|




















